"

35 Thin Airfoil Theory

Introduction

Airfoil theory is one of the cornerstones of aerodynamics, which can be used to predict how wing-like shapes or airfoils interact with airflows to generate lift and other aerodynamic effects. Many different types of airfoils can be designed in terms of their shape to optimize performance for specific tasks, such as maximizing lift, minimizing drag, or controlling pitching moments. The study of airfoil aerodynamics often encompasses the effects of their geometric properties, including the camber and thickness distribution, as they affect the aerodynamic characteristics, such as lift, drag, and pitching moment coefficients.

The geometry of an airfoil is described in terms of a profile shape that defines its upper and lower surfaces, i.e., an envelope, as shown in the figure below. The key dimension of an airfoil profile is defined in terms of its length or chord, i.e., the distance measured from the leading edge to the trailing edge. The camber of the airfoil profile is a measure of its curvature, which will give the airfoil different upper and lower surface shapes. The amount of camber and the chordwise position of the maximum camber strongly influence the airfoil’s aerodynamic characteristics.

 

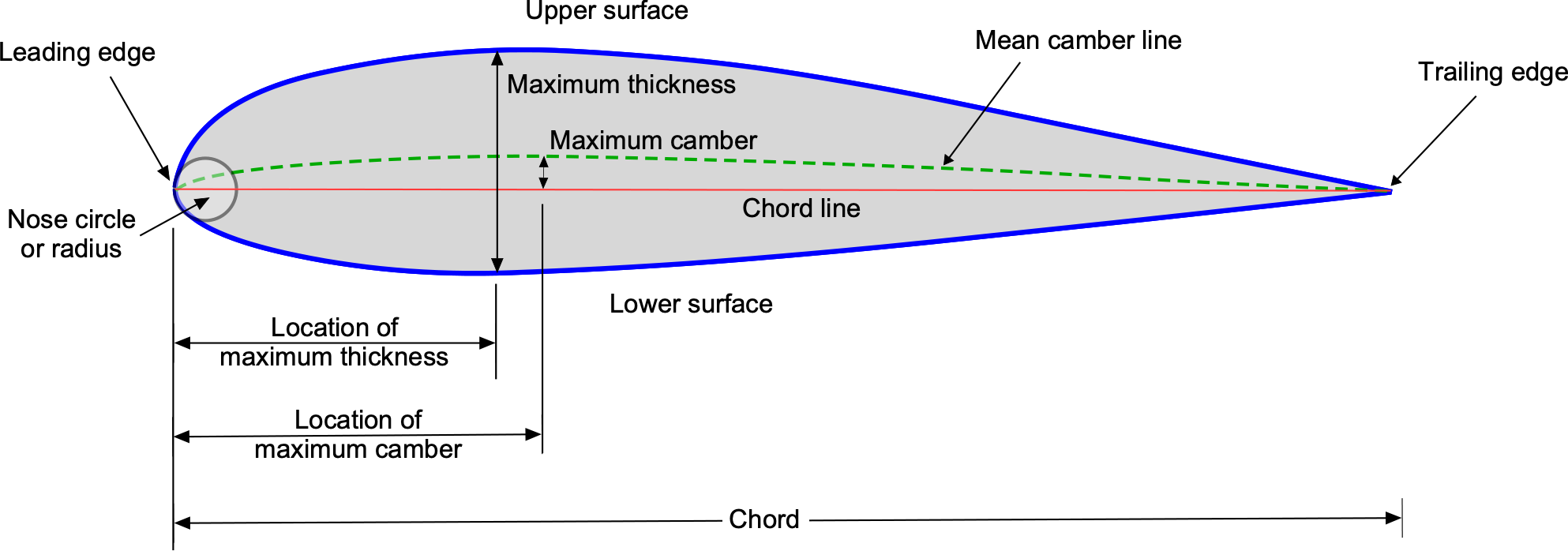
The geometry of an airfoil can be described in terms of its mean camberline and thickness distribution over the chord.

Understanding airfoil characteristics began with observations and measurements of wings placed in the wind tunnel, some of which were made by the Wright brothers. The Wrights were surprised that no aerodynamic theory existed by 1900, but the theory would soon catch up by the 1930s and evolve into increasingly more sophisticated predictive capabilities. Among the most influential analytical models was the classic thin airfoil theory, which provided a simplified yet theoretically exact framework for predicting the aerodynamic behavior of airfoils. Thin airfoil theory is a linearized analysis of airfoil flows with slight camber and at small angles of attack below stall. It is also a potential flow theory that assumes an inviscid and incompressible flow about the airfoil but can also be extended to linearized compressible and supersonic flows. Despite its simplicity, the thin airfoil theory yields key insights into airfoil behavior and is a foundation for more complex aerodynamic analyses. All students of aerodynamics learn the basics of the thin airfoil theory and how to use it as an essential part of their education and training.

Learning Objectives

  • Understand the principles of vortex sheets and their use in aerodynamic theory.
  • Appreciate the assumptions and simplifications underlying thin airfoil theory.
  • Derive and interpret the key equations for aerodynamic performance, such as lift and pitching moments.
  • Use the thin airfoil theory to predict the aerodynamics of a defined camberline shape.
  • Know about the advantages and limitations of thin airfoil theory.

History

Contributions from several aerodynamic pioneers shaped the development of the thin airfoil theory. Ludwig Prandtl, often regarded as the father of modern aerodynamics, laid the groundwork for understanding flows over airfoils. His work on aerodynamic theory and boundary layers paved the way for rapid advances in aerodynamic understanding and theoretical approaches. The mathematical formulation of airfoil theory was further advanced by Prandtl’s Ph.D. student, Max Munk, who introduced the concept of representing airfoil lift using circulation and vortex sheets. Munk’s contribution[1] established the connection between the lift force and the shape of the camberline of an airfoil, which is central to thin airfoil theory. The extract shown in the figure below from Munk’s dissertation in 1909 sets down what is known today as the fundamental equation of thin airfoil theory using the principles of vortex sheets and the Biot-Savart law.

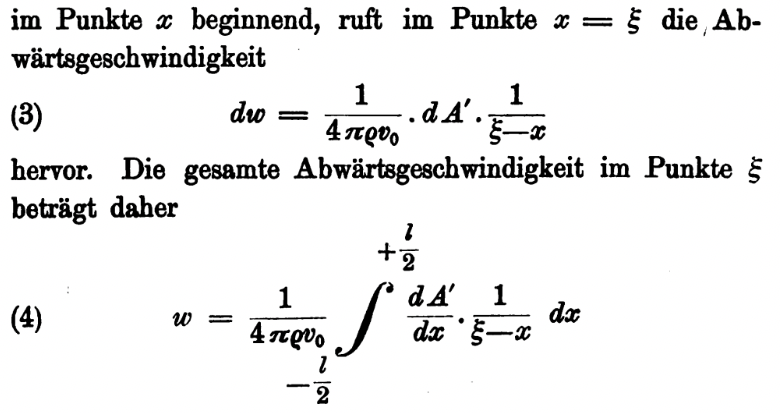
An extract from Max Monk’s Ph.D. dissertation showing the idea of a thin airfoil solution in aerodynamic theory.

Later mathematical developments and refinements by Hermann Glauert[2] made the thin airfoil theory more practical for routine use by using a general Fourier series approach to represent an arbitrary chordwise aerodynamic loading. Munk’s analysis, as Glauert stated, “is not quite free from errors,” so Glauert presented a more careful analysis with both mathematical and practical rigor. The aerodynamics of airfoils with arbitrary camber can then be solved. In the 1930s, Theodore Theodorsen aimed to extend the thin airfoil theory to unsteady aerodynamic problems, spawning the development of other aerodynamic theories. The NACA family of airfoil shapes, which became iconic in airplane design, used the principles underlying the thin airfoil theory and highlighted the lasting impact of this theory on aeronautical engineering.

Thin Airfoil Assumption

Many aerodynamic properties of airfoil sections with finite thickness can be closely approximated by their mean camberline. The mean camberline is defined as the locus of points situated halfway between the upper and lower surfaces of the section measured normal (perpendicular) to the mean line, as shown in the figure below. Airfoils without camber are called symmetric airfoils, with the same camber on the upper and lower surfaces.

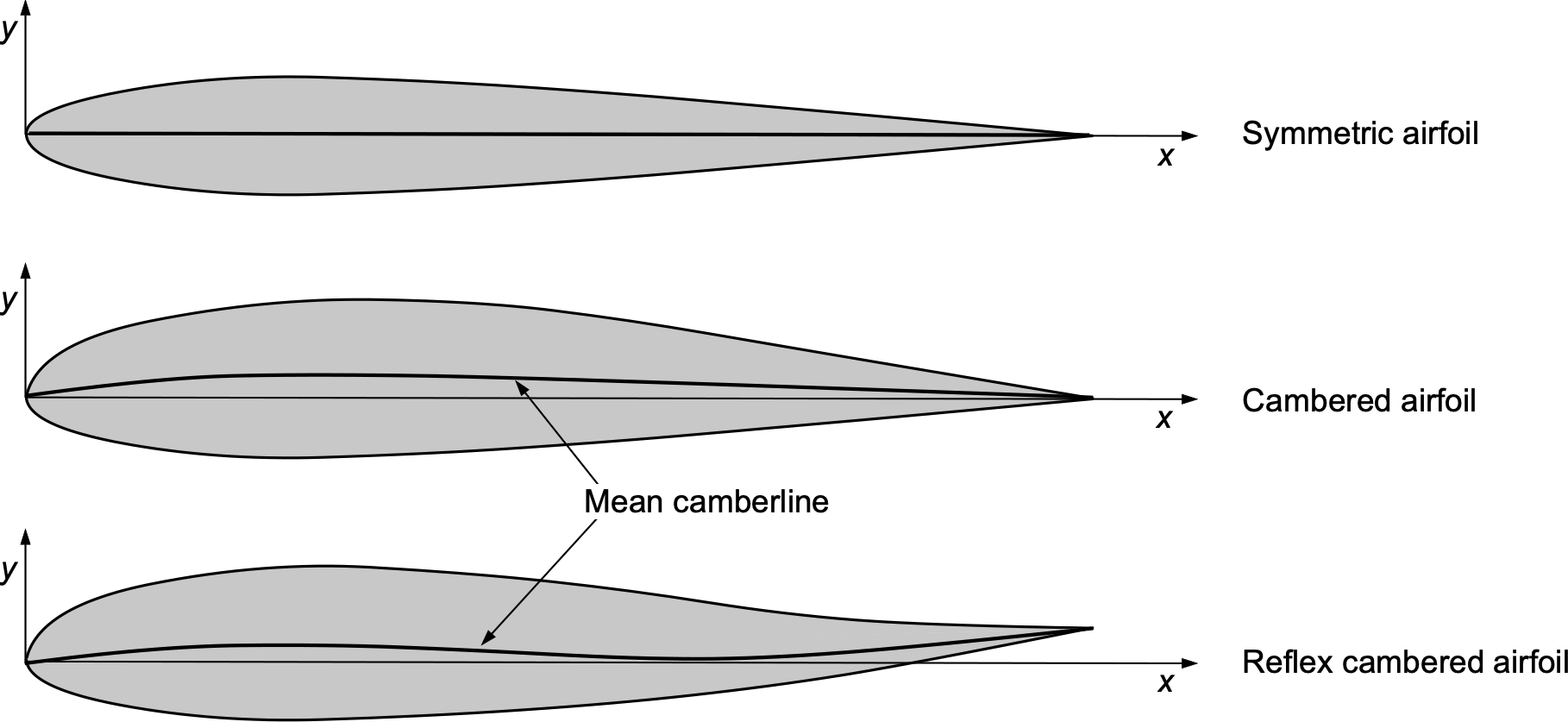
Airfoils come in many shapes, but most are positively cambered and reflexed airfoils.

Using some camber, especially over the airfoil’s leading edge, improves aerodynamic characteristics, especially at higher angles of attack, e.g., increasing the maximum lift coefficient. However, this capability is usually accompanied by increased pitching moments, which may be undesirable. In this regard, reflex camber, a form of upward camber at the trailing edge, provides many of the aerodynamic benefits of positive camber but with reduced pitching moments.

In the thin airfoil theory, the camberline itself becomes the airfoil shape on which to perform the aerodynamic analysis, as shown in the figure below. In other words, the aerodynamic effects of thickness are removed and ignored aerodynamically. Indeed, while the thickness distribution affects the pressure distribution around the airfoil, the effects are symmetrically disposed between the upper and lower surfaces. So, to first order, they cancel out their impacts on the integrated properties, such as lift and pitching moment.

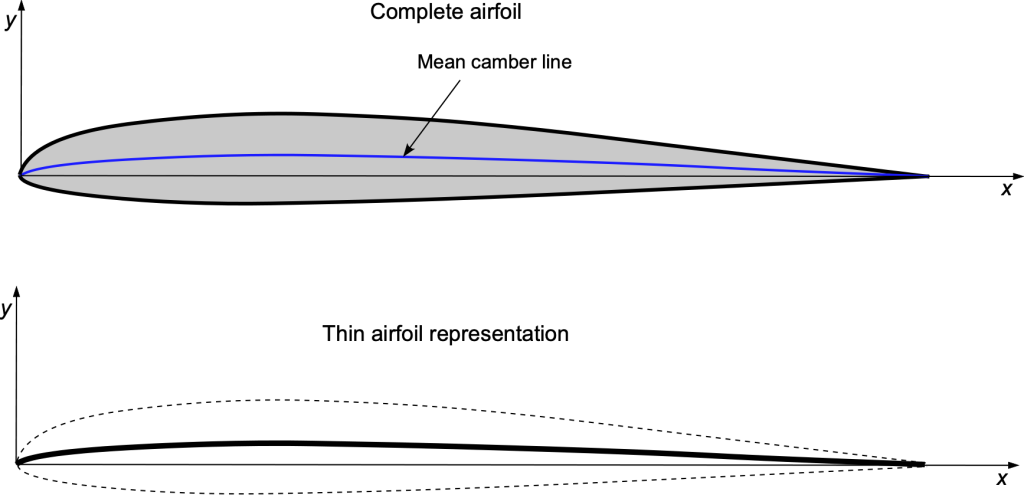
In the thin airfoil theory, the airfoil shape is replaced by its mean camberline.

The prominent aerodynamic properties that are primarily associated with the shape of the camberline include:

  • The chordwise loading distribution, i.e., the velocity and pressure distributions across the chord.
  • The magnitude of the lift coefficient, C_l, and the slope of the lift curve, i.e., the lift curve slope, C_{l_{\alpha}}.
  • The angle of attack for zero-lift, \alpha_0, i.e., the angle to which a cambered airfoil must be depressed to generate no lift.
  • The pitching-moment coefficient about some convenient reference axis, such as 1/4 chord, i.e., C_{m_{1/4}}.
  • Location of the center of pressure, x_{\rm cp}, and aerodynamic center, x_{\rm ac}.

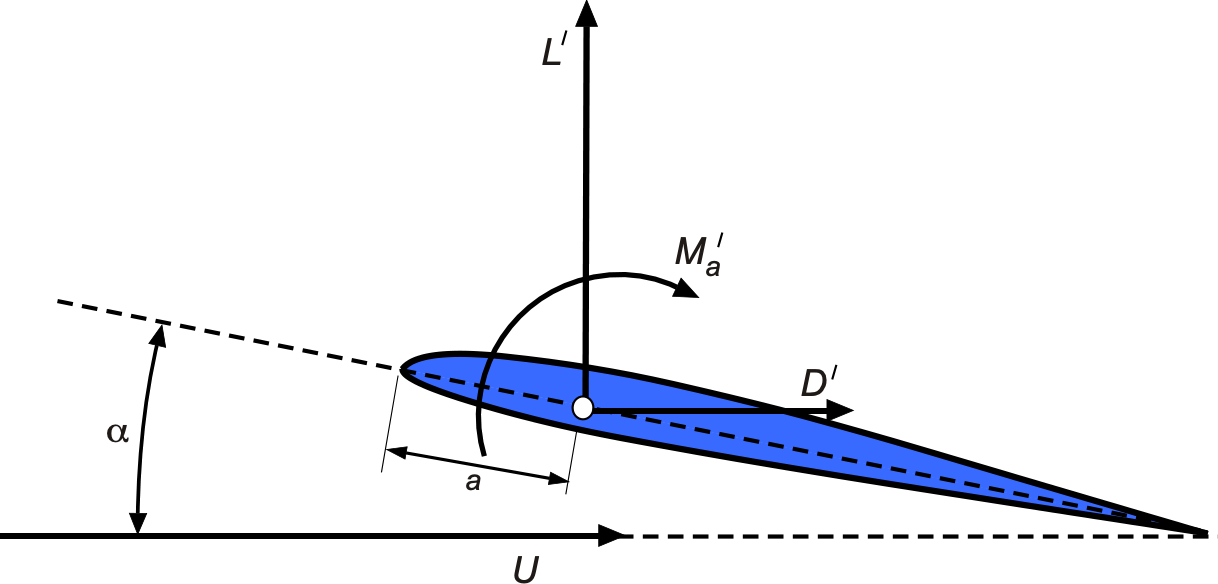
Force & Moment Conventions

A reminder of the aerodynamic coefficients acting on an airfoil section at an angle of attack \alpha is appropriate. The lift force per unit length (span), L', acts in a direction perpendicular to the free-stream velocity, V_{\infty}, or U in the convention of the classic thin airfoil theory, as shown in the figure below. The corresponding drag per unit span, D', is parallel to U, and there is also a moment M_a' about the point where the lift and drag are assumed to act. In a potential flow, the drag is zero, a result known as D’Alembert’s paradox.

Forces and moment acting on a two-dimensional airfoil section.

The dimensionless force coefficients are defined using the free-stream dynamic pressure, q_{\infty}, and chord, c, as a reference, i.e., the lift coefficient, C_l, and the drag coefficient, C_d, are given by[3]

(1)   \begin{equation*} C_{l} = \dfrac{L'}{q_{\infty} \, c} \quad \text{and} \quad C_{d} = \dfrac{D'}{q_{\infty} \, c} \end{equation*}

where the free stream dynamic pressure is q_{\infty} = \dfrac{1}{2} \varrho_{\infty} U^2. For an incompressible flow, \varrho = \varrho_{\infty} at all points, so the {\infty} subscript can be dropped.

Pitching moments on an airfoil can be resolved to any convenient reference point, as shown in the figure below. The dimensionless moment coefficient for an airfoil section can be defined as

(2)   \begin{equation*} C_{m_{a}} = \dfrac{M'_a}{q_{\infty} \, c^2} \end{equation*}

where a is some moment axis location. The 1/4-chord axis position is the most common, so that

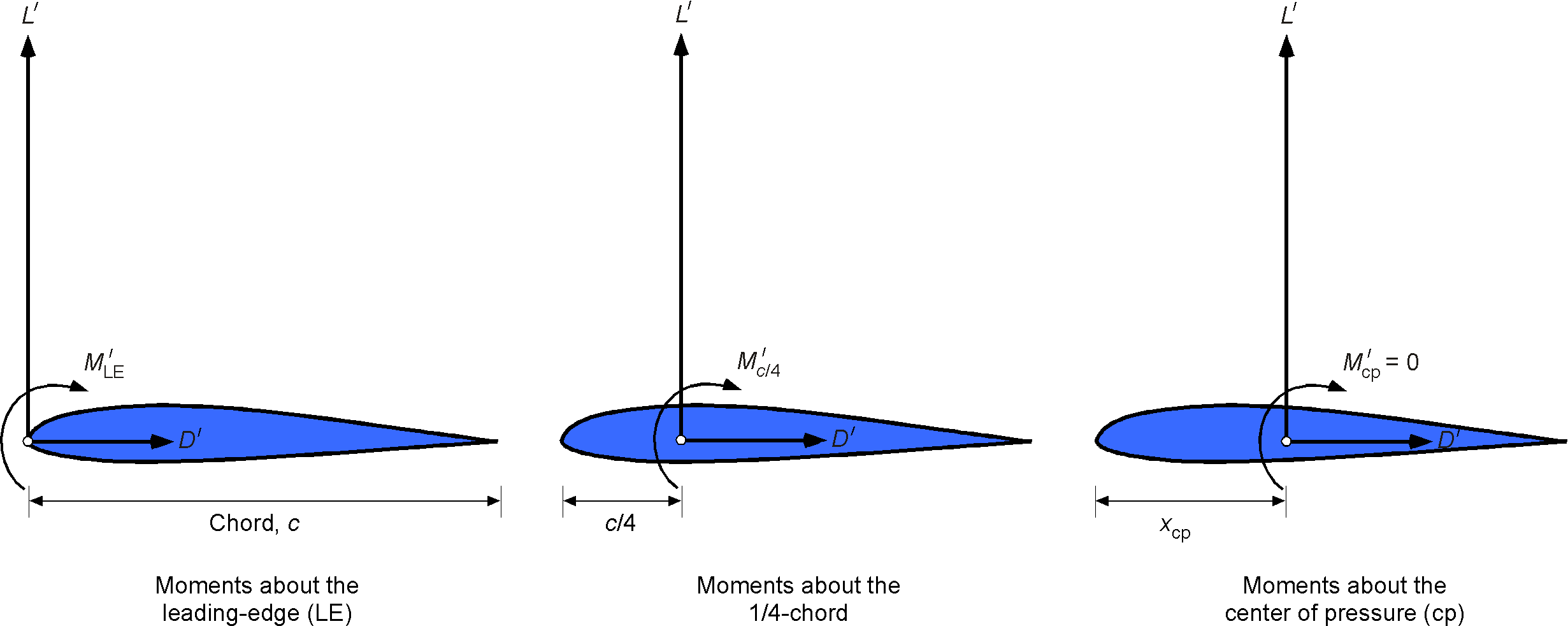
(3)   \begin{equation*} C_{m_{1/4}} = \dfrac{M'_{1/4}}{q_{\infty} \, c^2} \end{equation*}

Pitching moments may be taken about any axis, but the 1/4-chord is the most common. However, the moment about any axis point can be obtained using the principles of statics. In practice, one should double-check which axis the moments are calculated about.

Theory of Vortex Sheets

The theory of vortex sheets is fundamental in aerodynamics, particularly in the thin airfoil theory and surface singularity or “panel” methods. It provides a mathematical framework for modeling the flow velocity and pressure distribution around an airfoil and predicting its aerodynamic performance. The figure below shows the idea of a vortex sheet.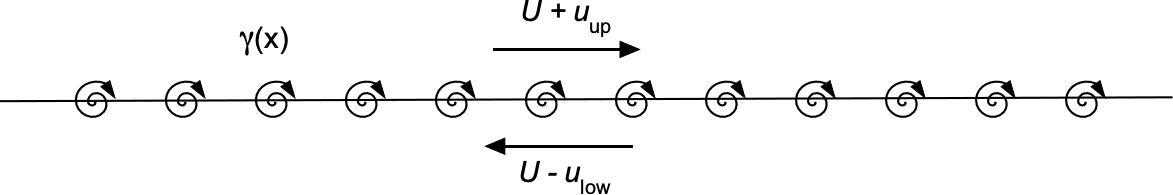

A vortex sheet singularity has a jump in velocity and pressure over its surface. The vortex sheet strength, \gamma(x), which can vary along the length of the sheet, represents the jump in tangential velocity across the sheet, i.e.,

(4)   \begin{equation*} \gamma(x) = u_\text{up} - u_\text{low} \end{equation*}

The jump in tangential velocity also leads to a pressure jump, \Delta p, i.e.,

(5)   \begin{equation*} \Delta p = \varrho \, u_\text{avg} \, \gamma(x) \end{equation*}

where u_\text{avg} = \dfrac{u_\text{up} + u_\text{low}}{2} is the average velocity. Integrating this pressure jump across the sheet generates a lifting force given by

(6)   \begin{equation*} L' = \int \Delta p \, dx \end{equation*}

Alternatively, because the total circulation is given by

(7)   \begin{equation*} \Gamma = \int \gamma(x) \, dx \end{equation*}

then, using the Kutta-Joukowski theorem, the lift is given by

(8)   \begin{equation*} L' = \varrho \, U \, \Gamma \end{equation*}

Thin Airfoil Theory

With the groundwork now done, the thin airfoil theory can be exposed. Vortex sheets play a crucial role in modeling the flow around an airfoil. The present treatment follows Glauert’s approach. The key idea is to represent the flow over the airfoil by placing a vortex sheet of unknown strength \gamma (x) along the camberline, as shown in the figure below. Because the camber is assumed to be small, the problem can then be linearized without much loss of accuracy by placing the vortex sheet along the chord line. Then, the approach is to calculate the strength of the vortex sheet on the chordline so that the flow is tangential to the camberline, i.e., the no penetration condition. In addition, the Kutta condition, which is an empirical observation, must be satisfied in that the flow must leave the trailing edge smoothly.

 

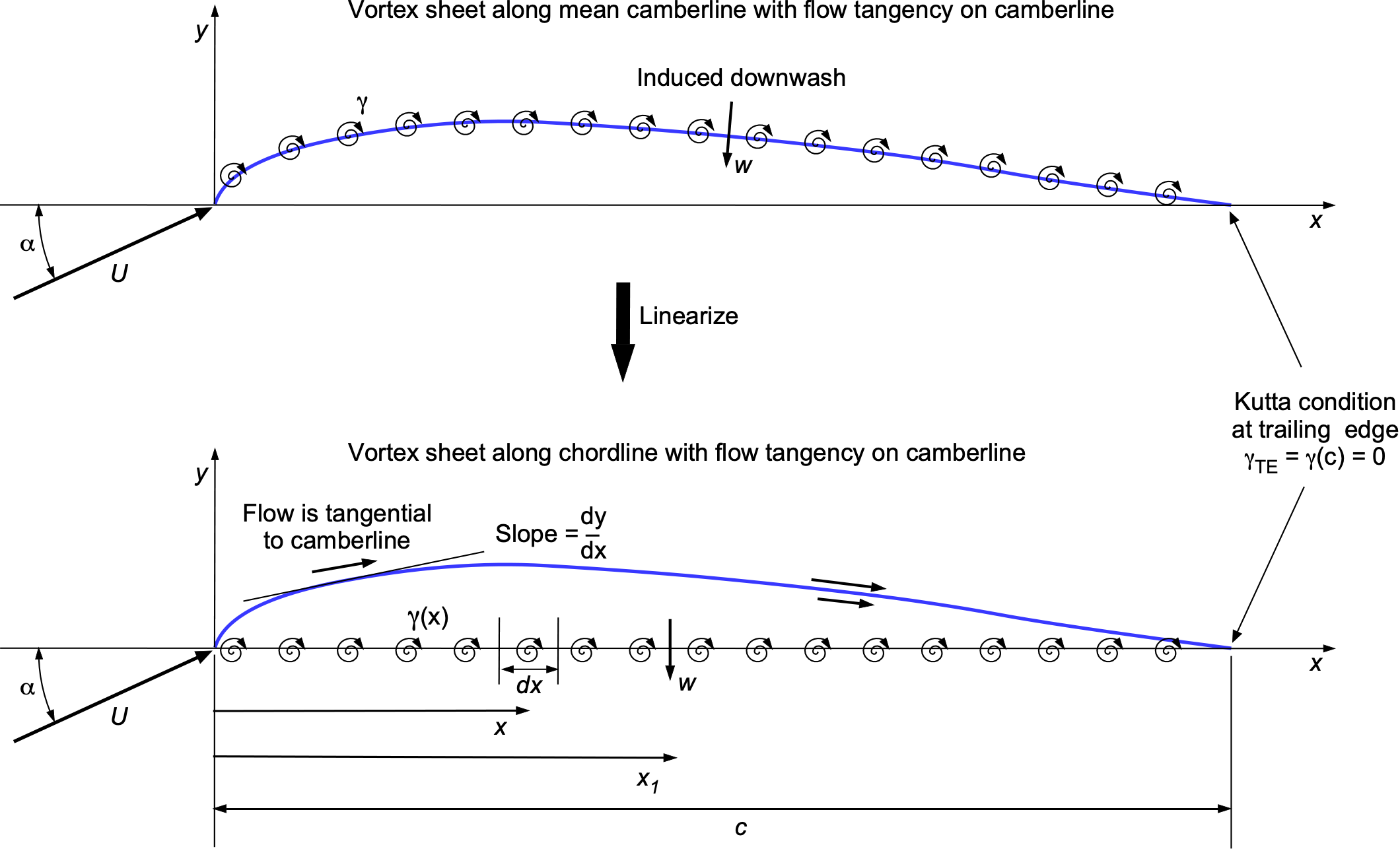
In the linearized thin airfoil theory, the vortex sheet is placed on the chordline, but flow tangency is still enforced on the camberline.

The strength of the sheet, \gamma (x), is partially determined using the tangential flow (no-penetration) boundary condition, i.e., it will be apparent from the figure below that

(9)   \begin{equation*} U \alpha - U \frac{dy}{dx} = w' \approx w \end{equation*}

and so to a small angle assumption

(10)   \begin{equation*} \frac{w}{U} = \alpha - \frac{dy}{dx} \end{equation*}

This latter equation is a formal statement of the no-penetration or flow tangency requirement on the camberline.

The auxiliary boundary condition is at the trailing edge, where the vortex sheet strength must go to zero to satisfy the Kutta condition, i.e., there is a smooth flow with no jump in velocity, which means that

(11)   \begin{equation*} u_\text{up}(TE) - u_\text{low}(TE) = \gamma({\rm TE}) = \gamma_{\rm TE} = 0 \end{equation*}

If the sheet strength is then suitably determined according to these boundary conditions, the total circulation around the airfoil section, \Gamma, is

(12)   \begin{equation*} \Gamma = \int_0^c \gamma (x) \, dx \end{equation*}

This leads to the pressure distribution over the vortex sheet and the integrated quantities on the airfoil, such as lift, per the Kutta-Joukowski theorem, i.e.,

(13)   \begin{equation*} L' = \varrho \, U \, \Gamma \end{equation*}

Now, more carefully consider the downwash velocity caused by an element of the vortex sheet, as shown in the figure below. Using the Biot-Savart law, the downwash, dw, at a point x_1 caused by an element at x will be

(14)   \begin{equation*} dw (x_1) = \frac{\gamma(x) \, dx}{2\pi (x_1 - x)} \end{equation*}

The flow model used to develop the thin airfoil theory.

Therefore, the total downwash from the sheet at any point x_1 is

(15)   \begin{equation*} w(x_1) = \int_0^c \frac{\gamma \, dx}{2\pi (x - x_1)} \end{equation*}

Recall that the boundary condition for flow tangency is

(16)   \begin{equation*} \frac{w}{U} = \alpha - \frac{dy}{dx} \end{equation*}

which leads to

(17)   \begin{equation*} \frac{1}{2 \pi \, U} \int_0^c \frac{\gamma (x)}{x - x_1} \, dx = \alpha - \frac{dy}{dx} \end{equation*}

This latter equation is called the fundamental or governing equation of thin airfoil theory. Again, the sheet strength \gamma(x) is unknown, which must now be solved subject to the boundary conditions of flow tangency on the camberline as well as the Kutta condition at the trailing edge.

Kutta Condition

The Kutta condition is based on an empirical observation that the flow leaves the trailing edge of an airfoil smoothly, avoiding singular characteristics in flow velocity and static pressure. As an empirical observation, it must be mathematically implemented in the thin airfoil theory. This condition was demonstrated in the early experiments of Prandtl and Tijens, who, about 1910, visualized the flow around an impulsively started airfoil at an angle of attack, as shown below. Watch the historical film here. Since then, many investigators have conducted confirmatory experiments at higher flow speeds and Mach numbers.

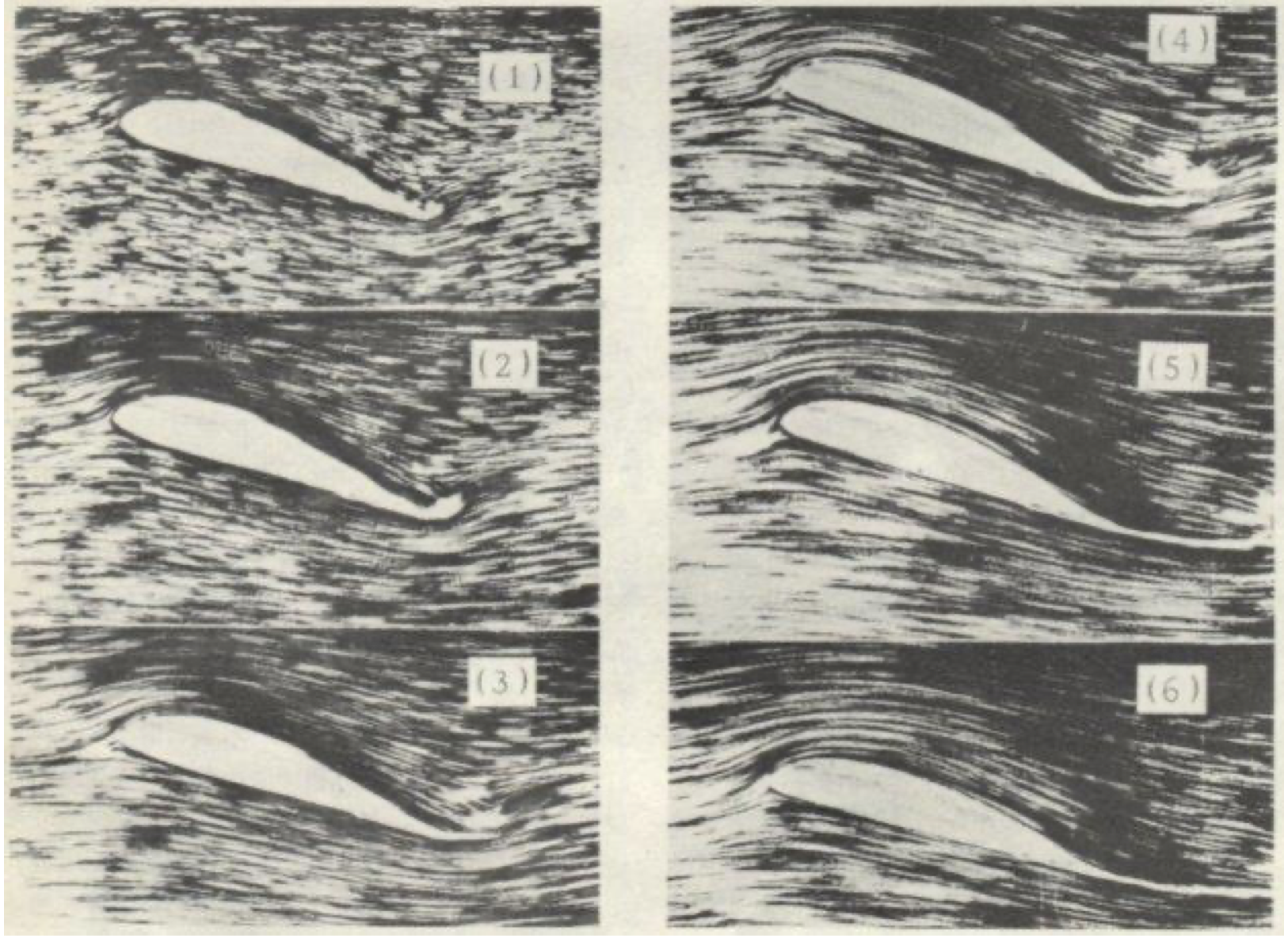
Time-history of flow developments around an impulsively started airfoil. (From Prandtl and Tijens.)

As frame (1) shows, the initial flow establishes itself, causing a local vortex flow and circulation around the trailing edge toward the upper surface. Still, no significant circulation or lift is generated on the airfoil at this stage. As the flow first curls around the trailing edge, locally high velocities and low pressure are obtained[4],which are not sustained for very long. Frames (2) through (5) then depict the temporal development of a starting vortex, which forms and is shed from the trailing edge as the flow further evolves. During this time, the circulation increases, and the lift builds.

In frame (6), the steady-state flow is becoming fully established, leaving the trailing edge smoothly in accordance with the Kutta condition, and the full lift is established. The starting vortex is convected asymptotically further downstream. It will eventually not influence the flow at the airfoil so that it can be disregarded in any steady-state flow analysis[5].

The Kutta condition, which forms the second boundary condition after flow tangency on the camberline, is expressed as

(18)   \begin{equation*} \gamma(x = c) = \gamma(TE) = u_{\rm up}(x) - u_{\rm low}(x) = 0 \end{equation*}

where u_{\rm up} and u_{\rm low} are the tangential velocities on the upper and lower surfaces, respectively, as shown in the figure below. Three types of trailing edges are encountered with practical airfoils: a convex trailing edge, a trailing edge with a finite enclosed angle, and a trailing edge forming a cusp.

 

Regardless of the trailing edge shape, the Kutta condition must be implemented to ensure the physical nature of the flow that it leaves the trailing edge smoothly.

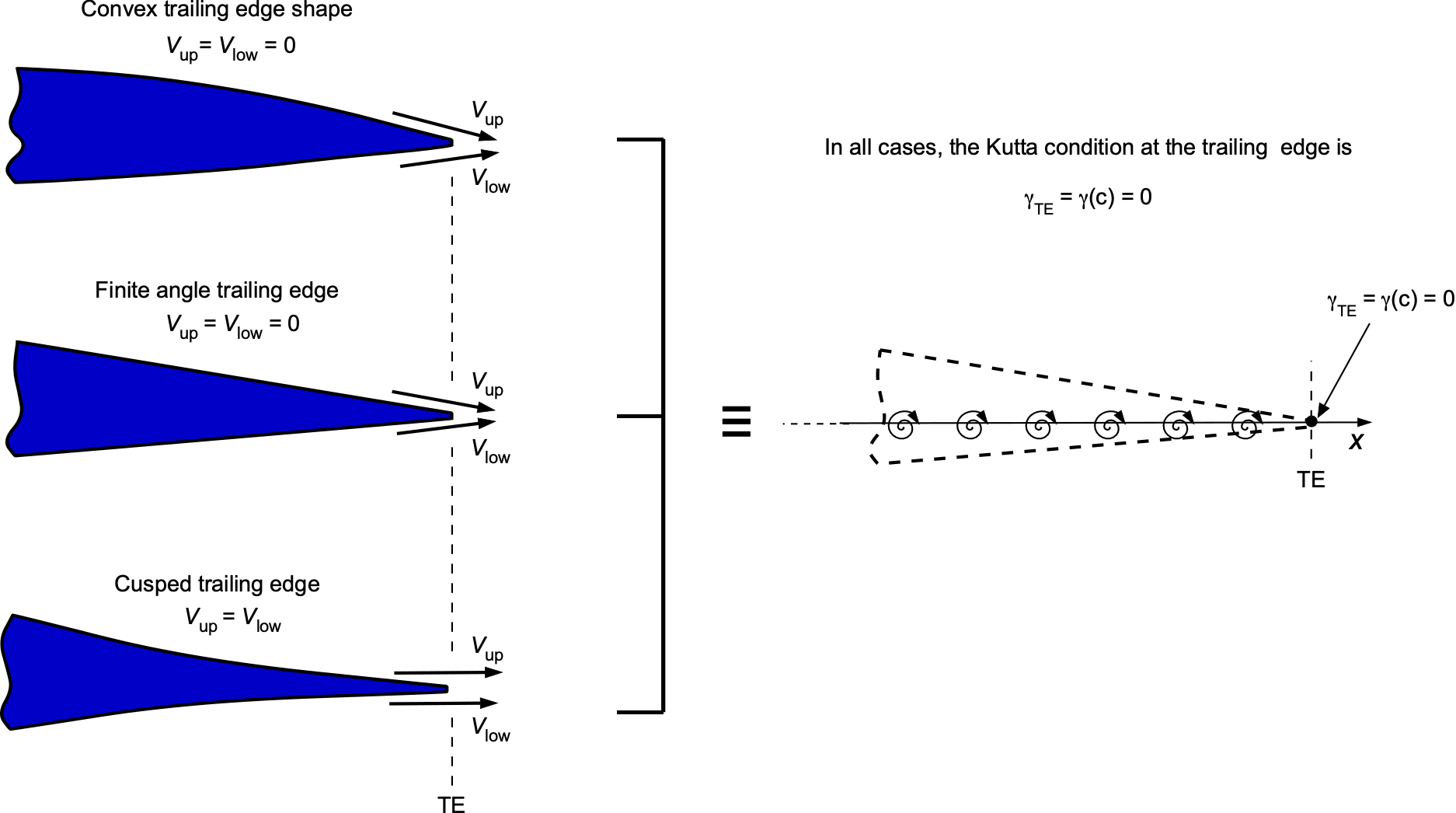
A convex trailing edge is characterized by a rounded trailing edge with finite curvature. Here, the tangential velocities u_{\rm up} and u_{\rm low} must have the same finite value as the flow leaves the trailing edge, so the only solution is that u_{\rm up} = u_{\rm low} = 0, i.e., a stagnation point. Therefore, \gamma(TE) = 0. For a finite-angle trailing edge, the upper and lower surfaces meet at a sharp point with a nonzero included angle. Again, the tangential velocities u_{\rm up} and u_{\rm low} must have the same finite value as the flow leaves the trailing edge, so the only solution is that u_{\rm up} = u_{\rm low} = 0, i.e., a stagnation point. Therefore, \gamma(TE) = 0 in this case also.

A cusped trailing edge is characterized by the upper and lower surfaces meeting tangentially at an infinitely sharp point. In this case, u_{\rm up} = u_{\rm low}, but it is not a stagnation point. Nevertheless, the theory of vortex sheets again ensures that \gamma(TE) = 0. In all cases, the Kutta condition ensures smooth, physically consistent flow at the airfoil’s trailing edge, enabling accurate lift predictions and overall aerodynamic performance.

Starting Vortex & Kelvin’s Theorem

Kelvin’s circulation theorem is closely connected to the concept of the Kutta condition. This theorem states that circulation in a potential flow is conserved. The condition can be described mathematically as

(19)   \begin{equation*} \frac{d\Gamma}{dt} = 0 \end{equation*}

Understanding the concept of circulation requires some thought, along with the connection of circulation to lift generation on a body in motion. Consider an airfoil initially at rest. If U = 0, the circulation \Gamma about the circuit around the airfoil must also be zero. When the airfoil is set into motion, it develops a nonzero circulation -\Gamma_1, as shown in the figure below. Observations of this flow show that a starting vortex with a counter circulation \Gamma_2 is shed from the trailing edge. This shedding also satisfies the Kutta condition at every instant in time. The vortex is swept downstream as observed in the standard reference frame moving with the airfoil, and in the steady state, it is convected to infinity with zero influence.

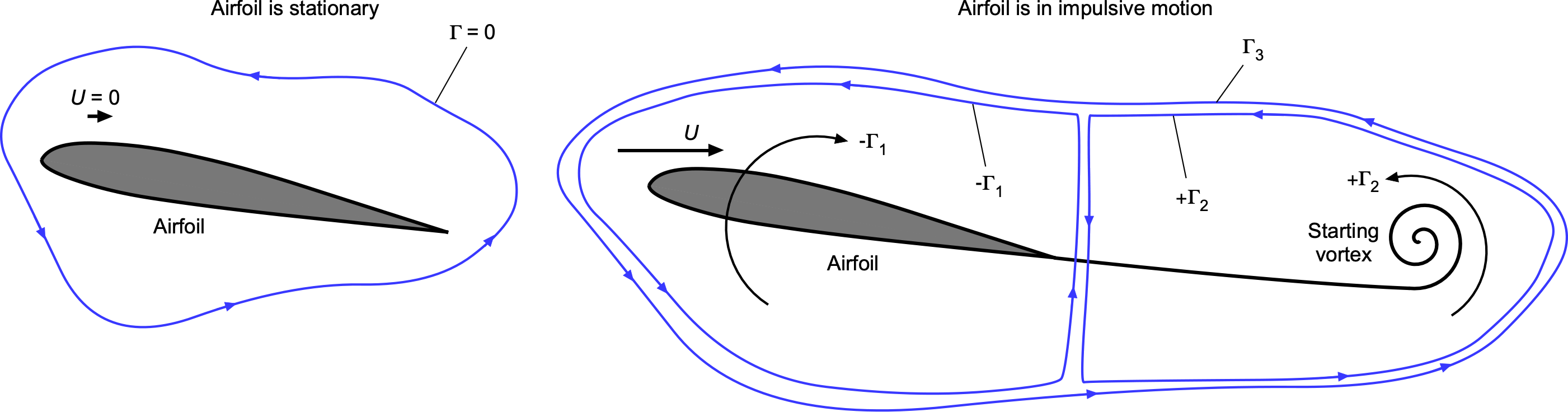
The shedding of a startup vortex and the creation of circulation is consistent with Kelvin’s circulation and the Kutta condition.

Following Kelvin’s theorem, the overall circulation \Gamma_3 must be zero and so equal the sum of the airfoil circulation and the shed vortex circulation, i.e.,

(20)   \begin{equation*} \Gamma_3 = -\Gamma_1 + \Gamma_2 = 0 \end{equation*}

Therefore, and regardless of the sign convention, the airfoil and the starting vortex must have equal and opposite values of circulation, i.e.,

(21)   \begin{equation*} \Gamma_2 = -\Gamma_1 \end{equation*}

As previously noted, a longer time[6] after the start of motion, the starting vortex is far downstream behind the airfoil and has no influence on the flow field around the airfoil. Therefore, the shed starting vortex can be disregarded when considering steady 2-dimensional airfoil flow. However, the continuous shedding of circulation into the near wake must still be considered in unsteady airfoil flows because this will create an induced velocity that affects the circulation around the airfoil and, hence, its lift.

Fourier Representation of Vortex Sheet Strength

Returning to the thin airfoil problem, the significance of the foregoing concepts can be understood and appreciated. While Munk did not take the thin airfoil theory to a practical conclusion, Glauert realized that it had to be expressed in a readily solvable form for it to be helpful. To this end, Glauert was to follow the work of Bairnbaum[7]to use a Fourier series representation of vortex sheet strength to simplify the mathematical treatment of predicting the distribution of aerodynamic loads along an airfoil. Using a Fourier series provided an elegant and systematic way to solve the fundamental equation of thin airfoil theory.

This approach has several advantages. First, using a Fourier series to represent the vortex sheet strength reduces the integral equation into a set of algebraic equations, making it easier to solve. Second, the Fourier series expansion allows the vortex sheet strength to be decomposed into independent modes, which can be combined using the principle of superposition. By expressing the loading as a sum of sine modes, the mathematics becomes more straightforward to analyze because trigonometric functions are well understood. Each mode corresponds to specific aerodynamic effects, such as overall lift or variations in chordwise loading. Finally, the coefficients of the Fourier series can be assigned direct physical interpretations, as will be shown.

Glauert Transformation

To solve the thin airfoil problem in a very general way, Glauert first used a linear to polar coordinate transformation as given by

(22)   \begin{equation*} x = \frac{c}{2} (1 - \cos \theta) \end{equation*}

where each x point on the chord is mapped to an equivalent \theta value, as shown in the figure below. This was a transformation that he also used in the development of the lifting-line theory.

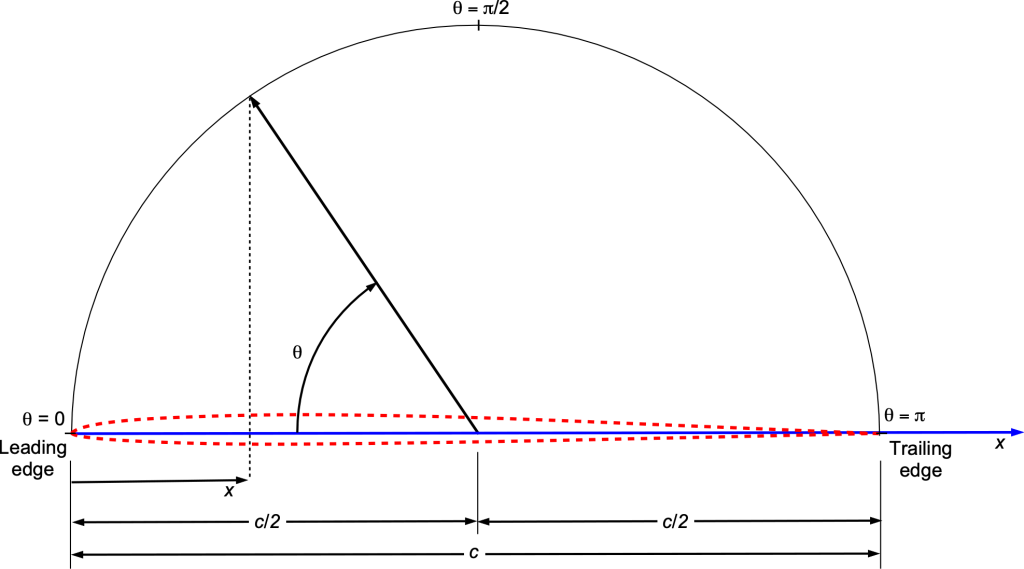
The Glauert transformation maps the linear airfoil coordinate in x to an angular coordinate in \theta.

In terms of the \theta coordinate, the fundamental or governing equation of thin airfoil theory is now

(23)   \begin{equation*} \left(\alpha - \frac{dy}{dx} \right) - \frac{1}{2\pi \, U} \int_0^\pi \frac{\gamma(\theta) \sin \theta}{\cos \theta - \cos \theta_1} \, d\theta = 0 \end{equation*}

where x maps to \theta and x_1 maps to \theta_1. The solution for the vortex strength \gamma (\theta) is then expressed as

(24)   \begin{equation*} \gamma (\theta) = 2 U \left( A_0 \cot \frac{\theta}{2} + \sum_{n=1}^\infty A_n \sin n\theta \right) \end{equation*}

where A_0 depends only on \alpha, and A_n depends on the shape of the mean camberline.

Fourier Loading Modes

Notice that the primary loading term, A_0, is given by

(25)   \begin{equation*} \cot \frac{\theta}{2} = \frac{1 + \cos \theta}{\sin \theta} \end{equation*}

the shape of which is shown in the figure below in terms of the x coordinate and the \theta coordinate.

 

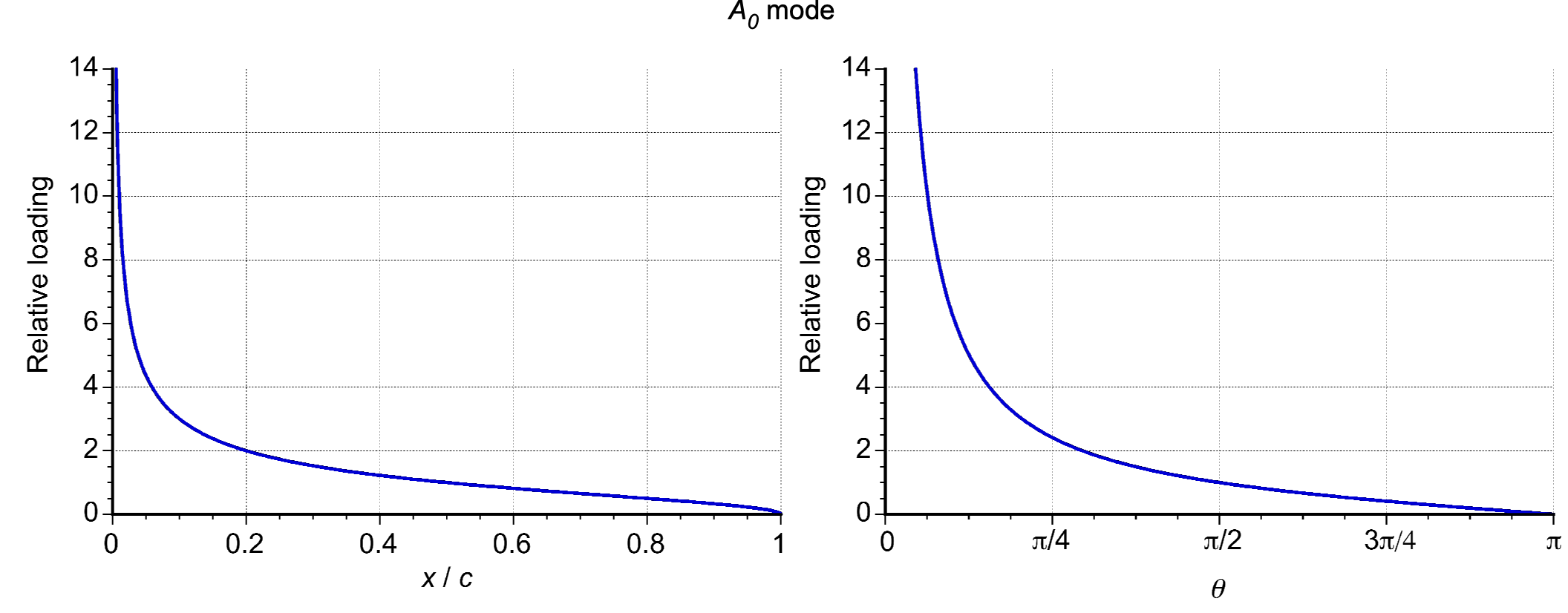
The primary A_0 Fourier loading mode mimics the chordwise differential pressure on a subsonic airfoil.

This A_0 term is significant because it mimics the angle of attack loading on a low-speed airfoil, an example of which is shown in the figure below. However, the leading edge singularity of the function should be noted as \theta \rightarrow 0. In thin airfoil theory, the pressure coefficient difference between the upper and lower surfaces, \Delta C_p, is given by

(26)   \begin{equation*} \Delta C_p(x) = \frac{2 \gamma(x)}{U} \end{equation*}

in accordance with the principles of vortex sheets. This pressure loading can also be written in terms of the x coordinate as

(27)   \begin{equation*} \Delta C_p(\bar{x}) = 4 \alpha \sqrt{ \dfrac{1 - \bar{x}}{ \bar{x} } } \end{equation*}

where \bar{x} = x/c and \alpha is the angle of attack in radians. In practice, the leading edge suction value is less than the theoretical maximum, which tends to infinity. This result is of some consequence in calculating the pressure drag, which is considered later in this chapter.

 

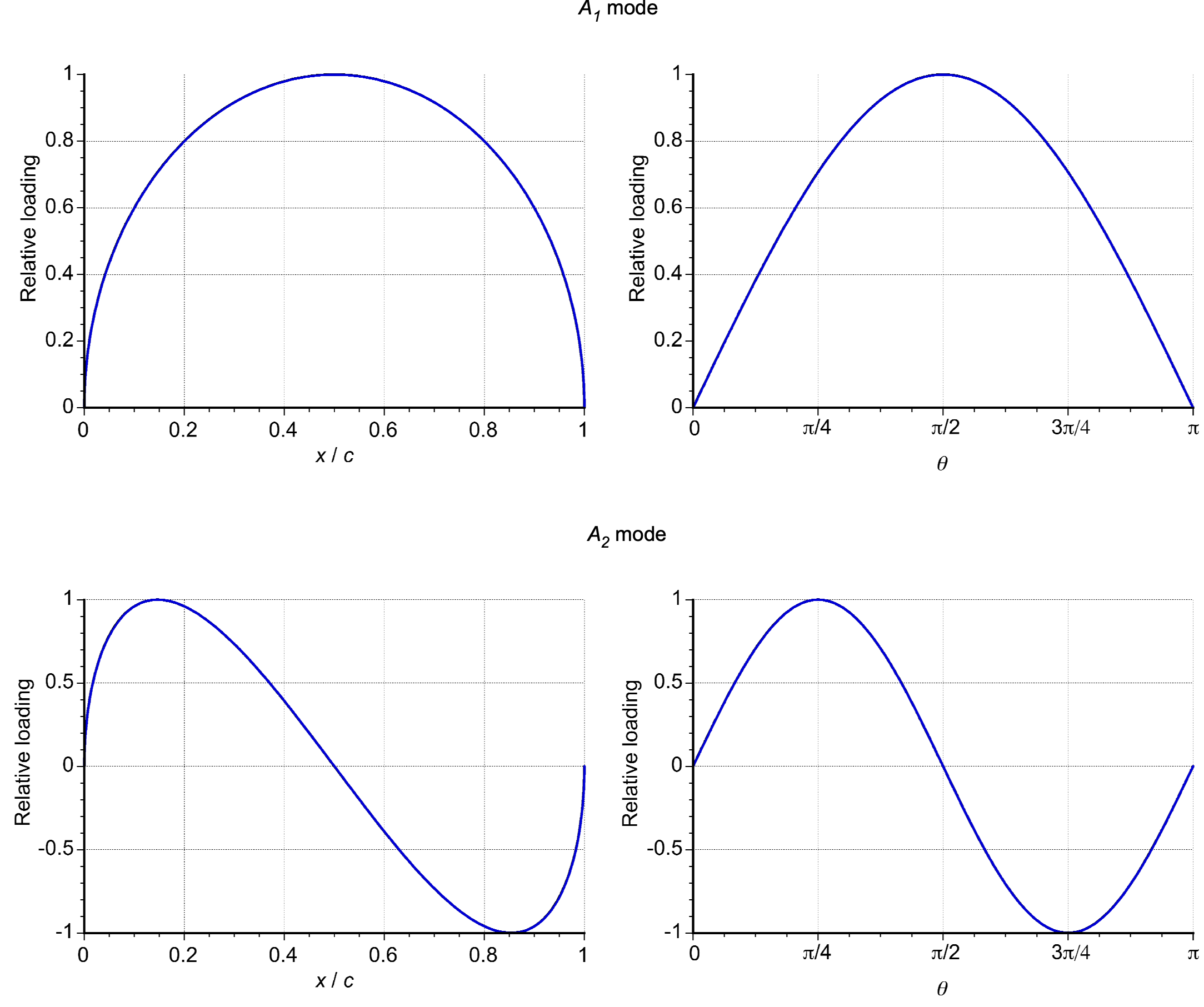
Representative pressure distribution on a thin airfoil at low speeds exhibits a high leading edge suction pressure.

The A_1 \sin \theta mode represents the first harmonic of the chordwise loading distribution, as shown in the figure below. It has an asymmetric variation that will affect the lift and the pitching moment. The A_2 \sin 2\theta mode represents the second harmonic of the loading distribution. This mode is symmetric about the mid-chord and will not affect the lift, but it will affect the moments.

 

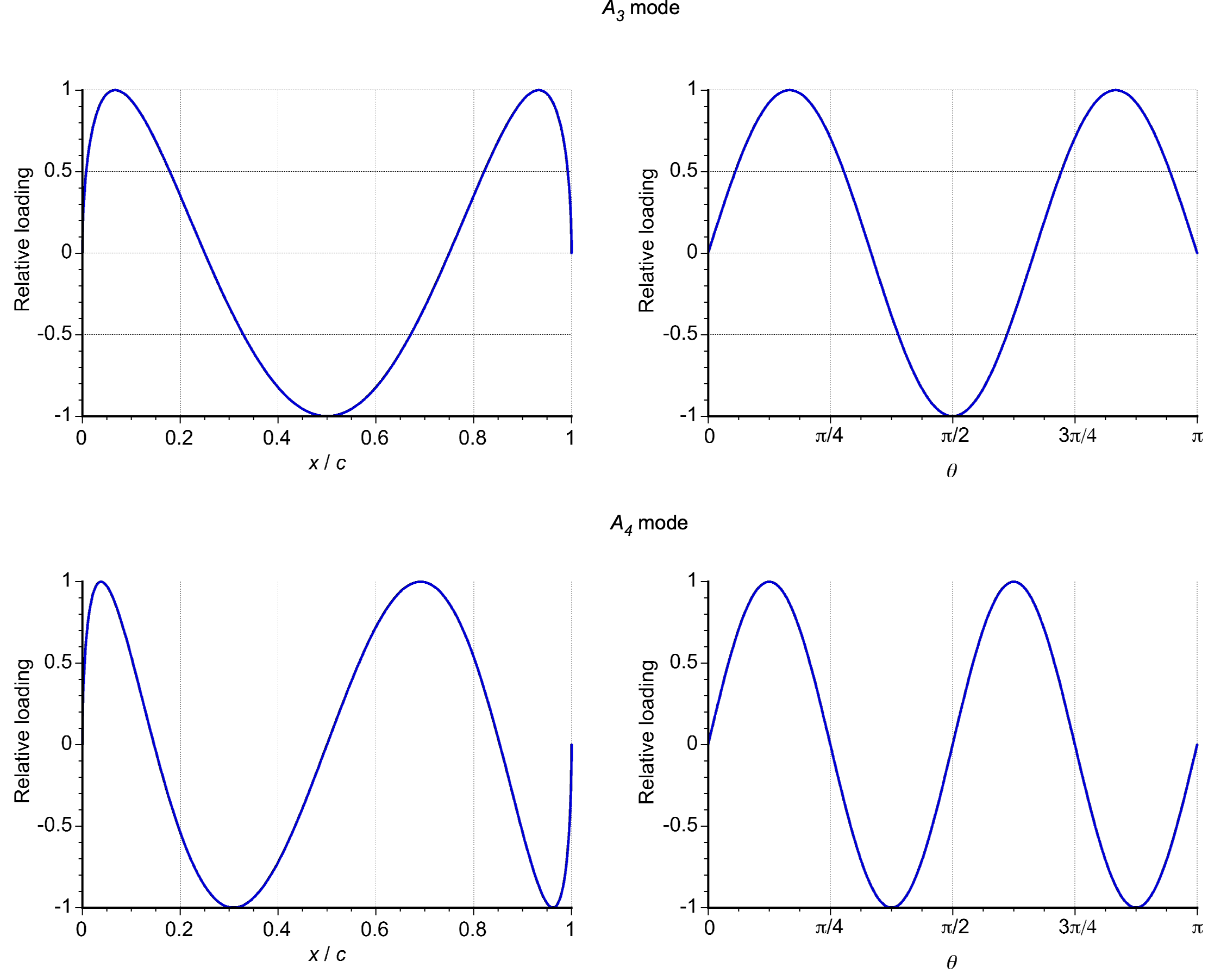
The A_1 \sin \theta and A_2 \sin 2\theta modes will contribute to both the lift and the pitching moments.

The A_3 \sin 3\theta, A_4 \sin 4\theta, and higher modes also have no symmetry about the mid-chord, indicating none of these modes will affect the lift or the pitching moments. This outcome will be apparent from an examination of the A_3 \sin 3\theta and A_4 \sin 4\theta modes, as shown in the figure below, where their integrals from 0 to \pi are all zero. These modes, however, do affect the pressure distribution. If the prediction of the pressure distribution is a desired outcome, they will have to be calculated using a sufficiently high number of modes.

 

The A_3 \sin 3\theta and higher modes do not affect the lift or the pitching moments.

Determining the Loading Coefficients

The primary loading coefficient A_0 is determined by evaluating

(28)   \begin{equation*} A_0 = \alpha - \frac{1}{\pi} \int_0^\pi \frac{dy}{dx} d\theta \end{equation*}

where \dfrac{dy}{dx} is the slope of the camberine. If the camberline is known in terms of some simple polynomial or other easily differentiable function, then this integral can be evaluated analytically.

Likewise, the coefficients A_n for n \geq 1 are determined using

(29)   \begin{equation*} A_n = \frac{2}{\pi} \int_0^\pi \frac{dy}{dx} \cos n\theta \, d\theta \end{equation*}

It will be noted that for a symmetric airfoil, y(x) = 0, then A_0 = \alpha, A_n = 0 \quad \text{for all } n \geq 1. However, for a cambered airfoil where y(x) \neq 0, A_0 and the A_n value must be calculated based on the specific camberline, y(x).

Lift & Lift Coefficient

The lift per unit span, L', is obtained by integrating the chordwise circulation distribution, \gamma(x), over the chord, i.e.,

(30)   \begin{equation*} L' = \int_0^c \varrho \, U \, \gamma \, dx \end{equation*}

Because the circulation distribution \gamma(x) is given using the Fourier series expansion

(31)   \begin{equation*} \gamma(\theta) = 2U \bigg( A_0 (1 + \cos\theta) + \sum_{n=1}^\infty A_n \sin n\theta \, \sin\theta \bigg) \end{equation*}

substituting this into the prior equation for the lift gives

(32)   \begin{equation*} L' = \int_0^\pi 2 \varrho \, U^2 \bigg( A_0 (1 + \cos\theta) + \sum_{n=1}^\infty A_n \sin n\theta \sin\theta \bigg) d\theta \end{equation*}

Separating the terms gives

(33)   \begin{equation*} L' = \int_0^\pi 2\varrho \, U^2 A_0 (1 + \cos\theta) \, d\theta + \int_0^\pi 2\varrho \, U^2 \left( \sum_{n=1}^\infty A_n \sin n\theta \sin\theta \right) d\theta \end{equation*}

Evaluating the first term gives

(34)   \begin{equation*} \int_0^\pi (1 + \cos\theta) \, d\theta = \pi \end{equation*}

and the second term (for n = 1) is

(35)   \begin{equation*} \int_0^\pi \sin\theta \sin\theta \, d\theta = \frac{\pi}{2} \end{equation*}

It will be apparent that the higher-order terms (n > 1) do not contribute to the lift.

Therefore, after evaluating the integrals, then

(36)   \begin{equation*} L' = \pi c \varrho \, U^2 \left(A_0 + \dfrac{A_1}{2}\right) \end{equation*}

This shows that lift depends only on the first two modes, A_0 and A_1, because of the orthogonality of trigonometric functions.

The lift coefficient is defined conventionally as

(37)   \begin{equation*} C_l = \frac{L'}{\frac{1}{2} \varrho \, U^2 c} \end{equation*}

Substituting the result for L' gives

(38)   \begin{equation*} C_l = \frac{\pi c \varrho \, U^2 \left(A_0 + \dfrac{A_1}{2}\right)}{\frac{1}{2} \varrho \, U^2 c} \end{equation*}

and simplifying gives

(39)   \begin{equation*} C_l = 2\pi \left(A_0 + \dfrac{A_1}{2}\right) = C_{l_{\alpha}} \left(A_0 + \dfrac{A_1}{2}\right) \end{equation*}

Notice that the lift-curve-slope is C_{l_{\alpha}} = 2\pi per radian angle of attack, independent of camber. This result is obtained because

(40)   \begin{equation*} C_{l_{\alpha}} = \frac{dC_l}{d\alpha} = 2\pi \left( A_0 \, \alpha + \dfrac{A_1}{2} \right) \end{equation*}

The A_1 term is a constant for a given airfoil because it depends only on the camberline, not the angle of attack. Differentiating with respect to \alpha gives

(41)   \begin{equation*} \frac{dC_l}{d\alpha} = 2\pi \left( \frac{dA_0}{d\alpha} \right) \end{equation*}

Therefore, the lift-curve slope C_{l_{\alpha}} = 2\pi is determined solely by the A_0 term, which includes the linear dependence on \alpha. The A_1 term affects the value of C_l at a specific \alpha, but it does not affect the slope of the lift curve.

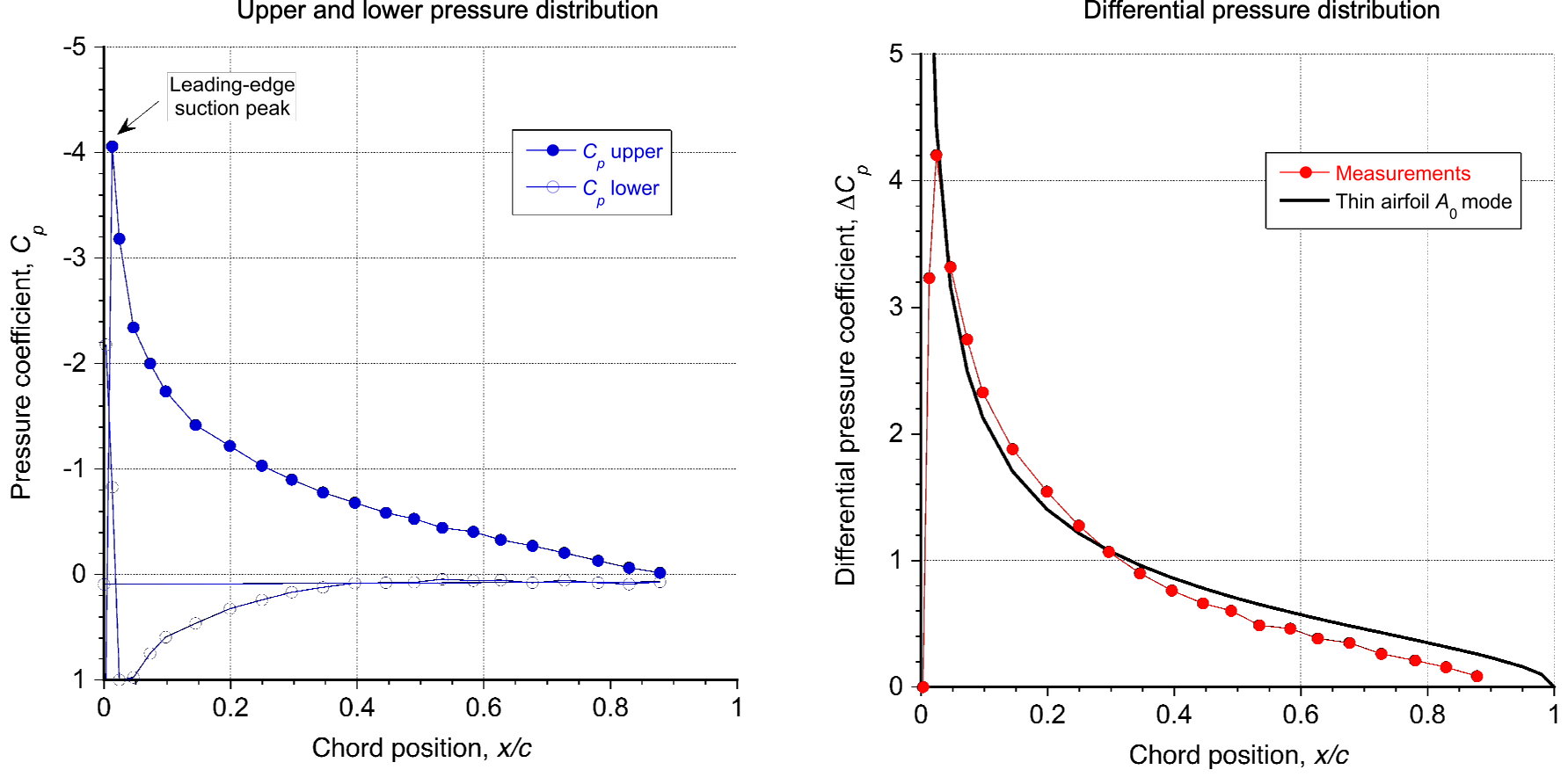
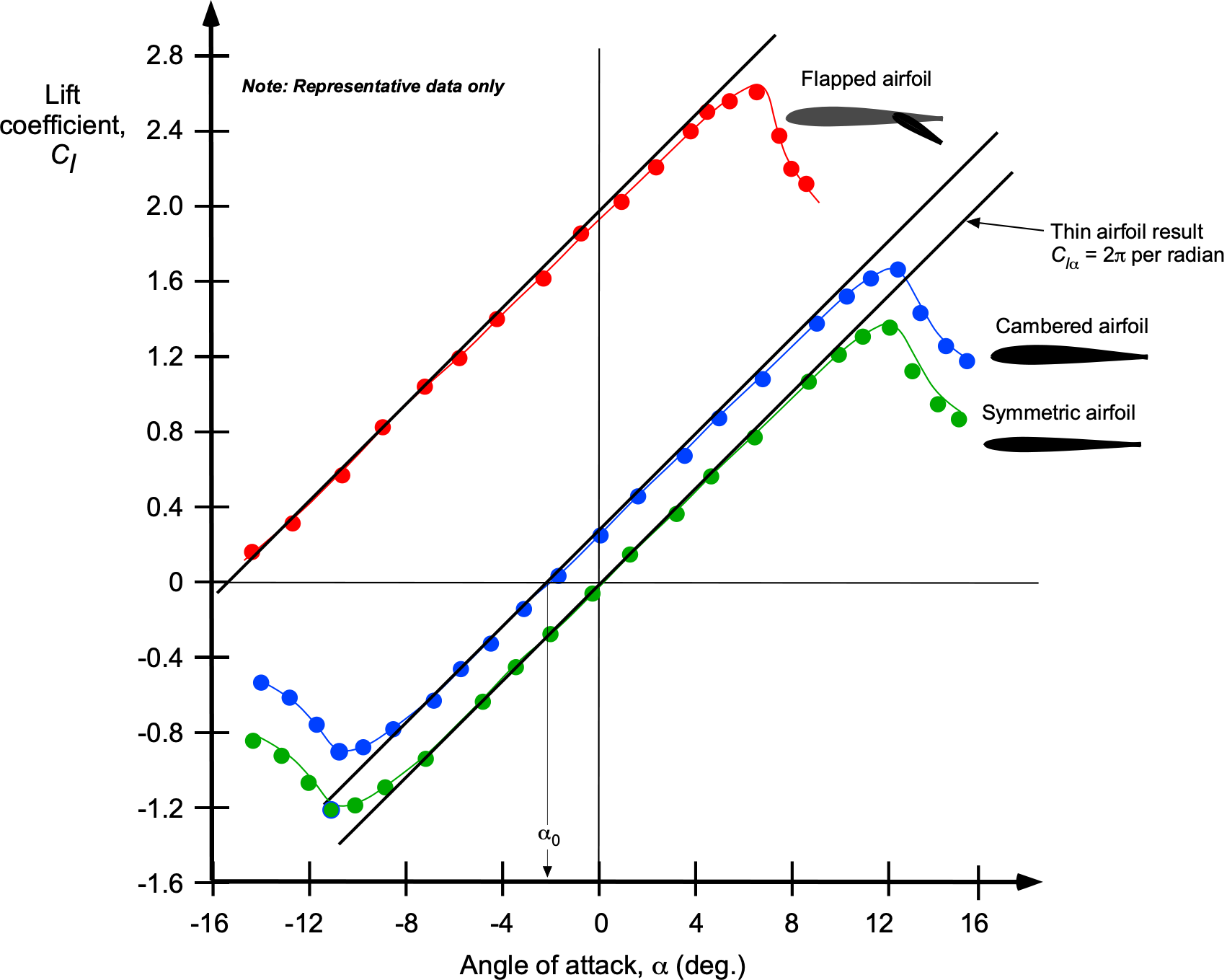
Comparison with Lift Measurements

One of the strengths of thin airfoil theory is its ability to accurately predict the linear relationship between the lift coefficient, C_l,  and angle of attack, \alpha, at mall angles and for moderately cambered airfoils. For example, the relationship

(42)   \begin{equation*} C_l = 2 \pi \left( \alpha - \alpha_0 \right) \end{equation*}

matches measured data well for thin airfoils with low thickness and fully attached flow, as shown in the figure below. It is particularly effective for explaining aerodynamic trends, such as the impact of camber on the zero-lift angle of attack, \alpha_0, and zero-lift pitching moment, C_{m_{0}}.

Comparison of thin airfoil theory with lift coefficient measurements for a cambered airfoil section.

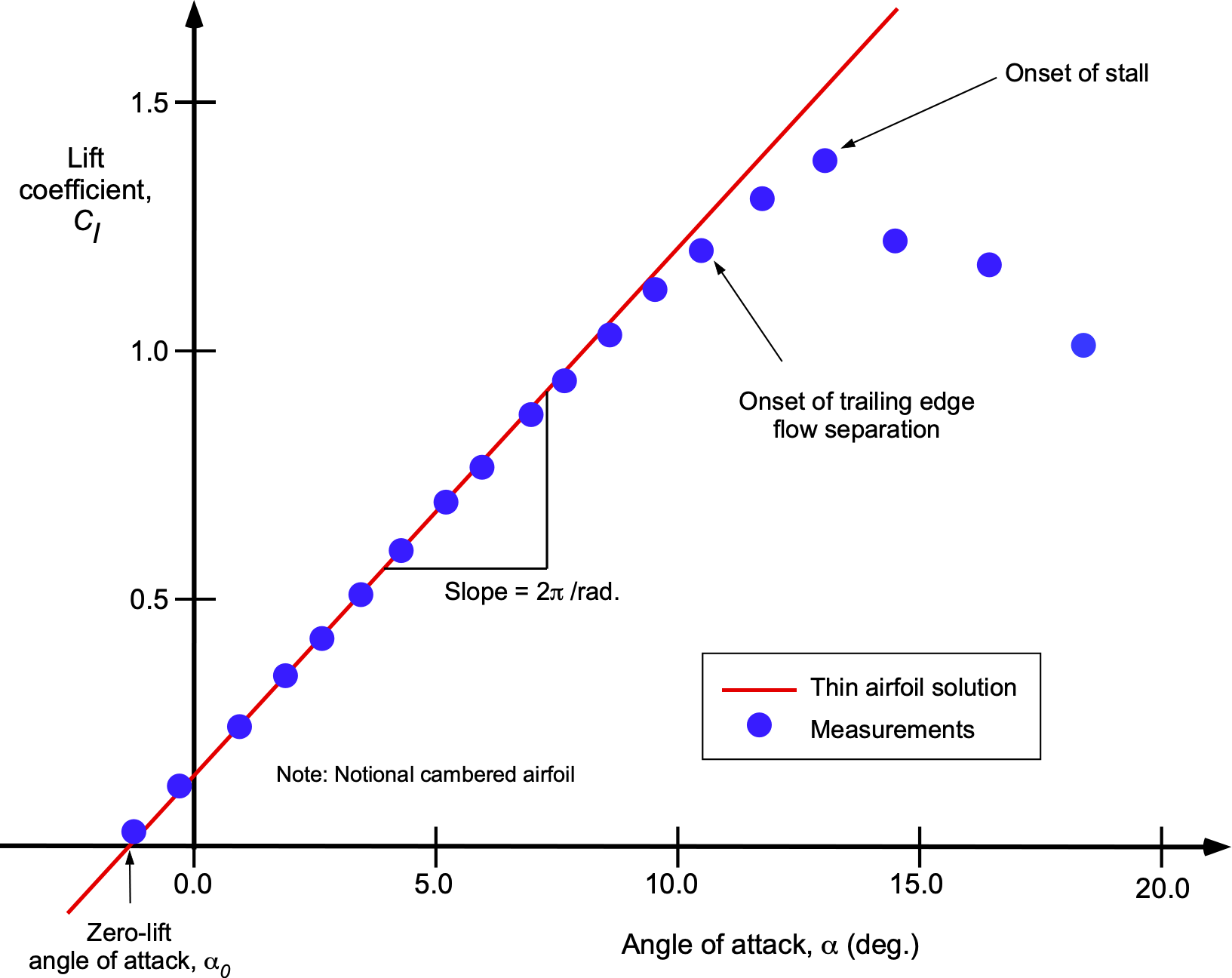
Compared with measured data, thin airfoil theory accurately predicts the lift curve slope 2\pi per radian for thin, symmetric airfoils under subsonic conditions. However, measurements for most airfoils show slightly lower slopes because of thickness and viscous effects. The theory does not predict the sharp drop lift near the stall because it cannot model flow separation. Overall, thin airfoil theory works best for thin, low-camber airfoils operating at low to moderate angles of attack and under subsonic flow conditions.

One of its primary assumptions is that the airfoil has zero thickness, which means it cannot account for the effects of finite thickness, such as increased drag, boundary layer separation, or compressibility at high speeds. It also assumes fully attached flow, which makes it invalid near stall or at high angles of attack where flow separation occurs. Furthermore, because it ignores viscosity, the theory cannot predict drag or account for Reynolds number effects, especially below 10^6. At higher angles of attack, for airfoils with large camber, at higher flow speeds where compressibility effects manifest, the linear assumptions of the theory break down, leading to significant deviations from measurements.

Pitching Moments

The expression for the pitching moment per unit span about the leading edge is given by

(43)   \begin{equation*} M'_{\rm LE} = -\int_0^c \varrho \, U \, \gamma \, x \, dx \end{equation*}

Using the chordwise loading distribution, then the pitching moment becomes

(44)   \begin{equation*} M'_{\rm LE} = -\frac{\varrho c^2 U^2}{2} \int_0^\pi \bigg( A_0(1 - \cos\theta) + \sum_{n=1}^\infty A_n \sin n\theta \left(\sin\theta - \frac{1}{2} \sin 2\theta\right)\bigg) d\theta \end{equation*}

Using the trigonometric simplification

    \[ \sin\theta(1 - \cos\theta) = \sin\theta - \frac{1}{2}\sin 2\theta \]

and performing the integration loads to

    \[ \int_0^\pi A_0(1 - \cos\theta)\sin\theta \, d\theta = \frac{\pi}{2} \, A_0 \]

and

    \[ \int_0^\pi A_1 \sin\theta \sin\theta \, d\theta = \frac{\pi}{2} \, A_1 \]

and

    \[ \int_0^\pi A_2 \sin 2\theta \left(-\frac{1}{2}\sin 2\theta\right) \, d\theta = -\frac{\pi}{4} \, A_2 \]

Notice that higher-order terms (n > 2) vanish because of the orthogonality of trigonometric functions.

The final expression for the pitching moment about the leading edge is

(45)   \begin{equation*} M'_{\rm LE} = -\frac{\pi}{2} c^2 \varrho \, U^2 \left(A_0 + A_1 - \frac{A_2}{2}\right) \end{equation*}

and the pitching moment coefficient about the leading edge is then

(46)   \begin{equation*} C_{m_{\rm LE}} = \dfrac{M'_{\rm LE}}{\dfrac{1}{2} \varrho \, U^2 c^2} = -\pi \left(A_0 + A_1 - \frac{A_2}{2}\right) \end{equation*}

Aerodynamic Center

The aerodynamic center (x_{\text{ac}}) is the point along the chord of the airfoil where the pitching moment coefficient, C_m, is constant and does not change with the angle of attack, \alpha. For all thin airfoils, including both symmetric and cambered airfoils, the aerodynamic center is located at 1/4-chord, i.e.,

(47)   \begin{equation*} x_{\text{ac}} = \frac{c}{4} \quad \text{or} \quad \frac{x_{\text{ac}}}{c} = \overline{x}_{\text{ac}} = \frac{1}{4} \end{equation*}

where c is the chord of the airfoil. In practice, it is found from measurements that the aerodynamic center of most airfoils in low-speed flows is close to the 1/4-chord.

Center of Pressure

The center of pressure (x_{\text{cp}}) is the point along the chord of the airfoil where the resultant aerodynamic force acts. At this point, there is no pitching moment about the center of pressure. The location of the center of pressure is a moving point and depends on the lift coefficient, C_l, and the moment coefficient about the leading edge, C_{m,\text{LE}}, i.e.,

(48)   \begin{equation*} x_{\text{cp}} = -\frac{C_{m_{\rm LE}}}{C_l} \end{equation*}

Be aware that the center of pressure may lie off the chord at low values of lift coefficient. This is another reason it is not the most convenient point, as it is a moving point, as shown in the figure below. Notice that as the angle of attack and lift coefficient increase, the center of pressure approaches the aerodynamic center.

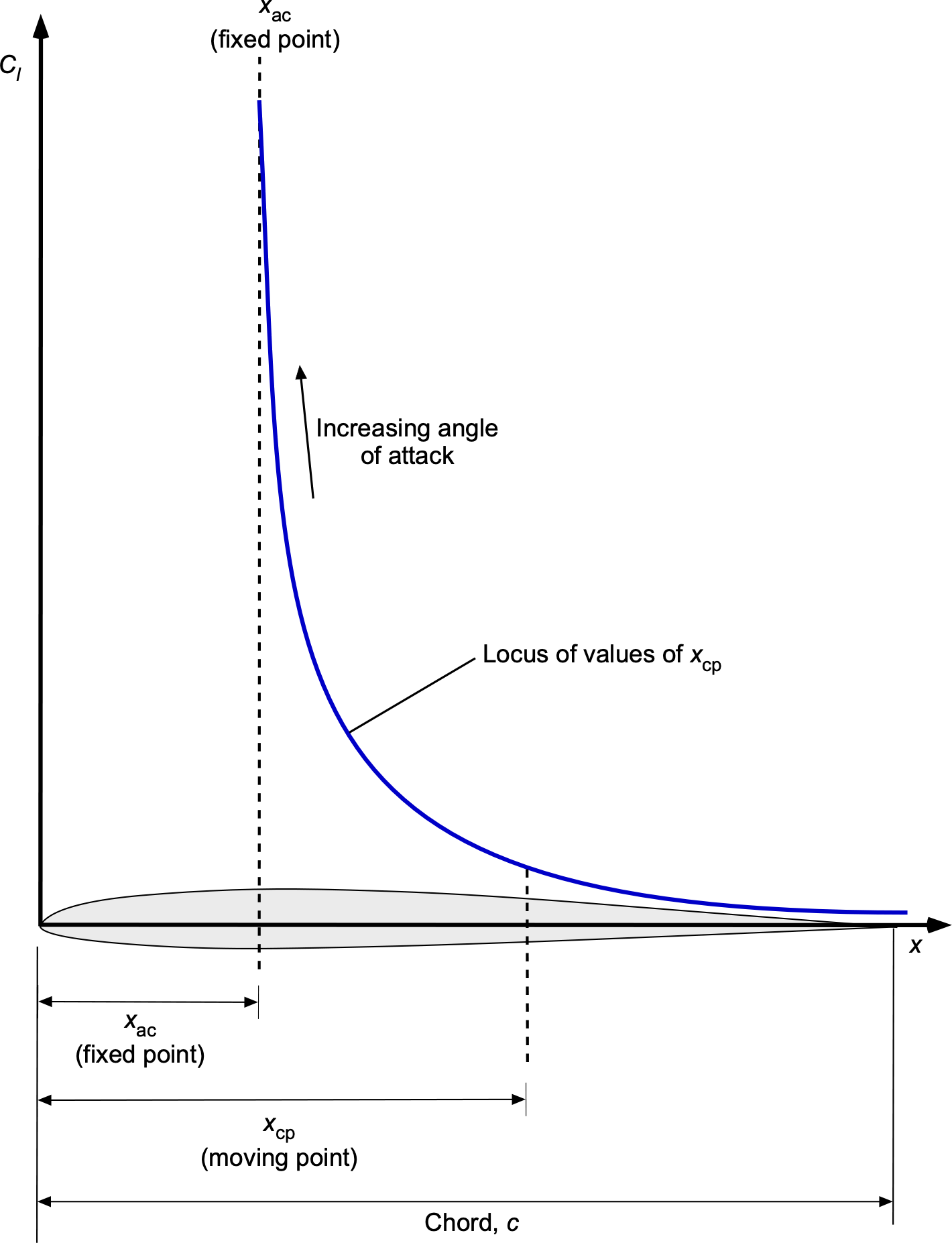
While the center of pressure is generally a moving point, it approaches the aerodynamic center at higher angles of attack.

For a symmetric airfoil, then

(49)   \begin{equation*} x_{\text{cp}} = x_{\text{ac}} = \frac{c}{4} \quad \text{or} \quad \frac{x_{\text{cp}}}{c} = \overline{x}_{\text{cp}}= \frac{1}{4} \end{equation*}

so for a symmetric airfoil, the aerodynamic center and the center of pressure coincide. For a cambered airfoil, then

(50)   \begin{equation*} \overline{x}_{\text{ac}} = \frac{1}{4} \end{equation*}

so the aerodynamic center remains fixed at the 1/4-chord. However, for a cambered airfoil x_{\text{cp}} varies with \alpha according to Eq. 48.

Comparison with Moment Measurements

Airfoil measurements are usually presented about the 1/4-chord. In this regard, the pitching moment about the 1/4-chord can be obtained using the principles of statics, i.e.,

(51)   \begin{equation*} C_{m_{\rm LE}} = C_{m_{1/4}} - \dfrac{C_l}{4} \end{equation*}

noting that moments are measured positive nose-up. Therefore,

(52)   \begin{equation*} C_{m_{1/4}} = C_{m_{\rm LE}} + \dfrac{1}{4} \, C_l = C_{m_{\rm LE}} + \overline{x}_{\text{ac}} \, C_l \end{equation*}

which is plotted below. Notice that the thin airfoil results tend to follow a slightly positive slope.

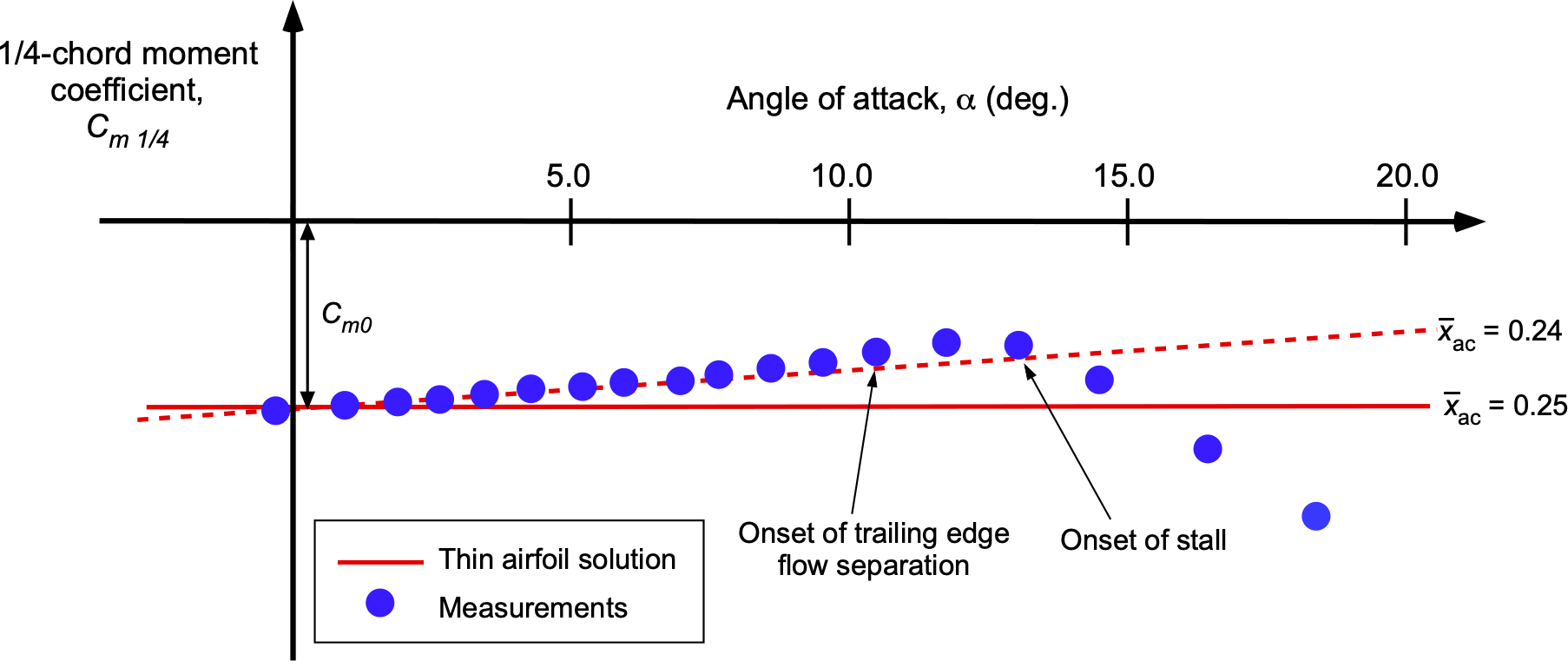
Comparison of thin airfoil theory with 1/4-chord pitching moment coefficient measurements for a cambered airfoil section.

The pitching moment coefficient can also be written as

(53)   \begin{equation*} C_{m_{1/4}} = C_{m_{0}} + \left(\dfrac{1}{4} - \overline{x}_{\text{ac}} \right) \, C_l \end{equation*}

where C_{m_{0}} is the moment coefficient at zero-lift and \overline{x}_{\text{ac}} now becomes the actual position of the aerodynamic center other than at 1/4-chord. For these particular data, it would seem that the actual aerodynamic center is just slightly forward of 1/4-chord. In general, the use of thin airfoil theory aligns well with the measurement of the zero-lift moment. However, minor deviations as a function of the angle of attack (or lift coefficient) indicate that the aerodynamic center is not perfectly at 1/4-chord for most airfoils. This outcome is because of the effects of viscosity and the formation of boundary layers on the upper and lower surfaces of the airfoil.

Worked Example #1 – Parabolic Camberline

Determine the aerodynamic characteristics of a thin airfoil of unit chord where the camberline is given by

    \[ y(x) = 4y_m x \left( 1 - x \right) \]

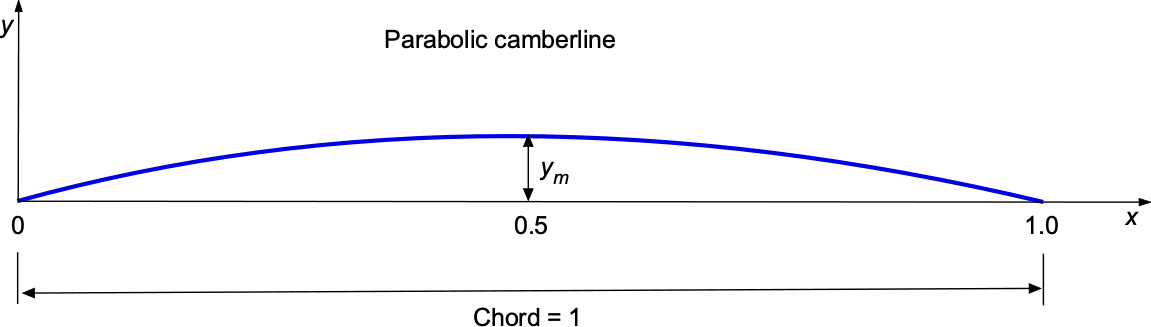
Show solution/hide solution.

The slope of the camberline is

    \[ \frac{dy}{dx} = 4y_m \left(1 - 2x\right) \]

Substituting x = \dfrac{1}{2}(1 - \cos\theta) gives

    \[ \frac{dy}{dx} = 4y_m \left( 1 - 2\left(\frac{1}{2}(1 - \cos\theta)\right) \right) = 4y_m \cos\theta \]

The A_0 term is given by

    \[ A_0 = \alpha - \frac{1}{\pi} \int_0^\pi \frac{dy}{dx} \, d\theta = \alpha - \frac{1}{\pi} \int_0^\pi 4y_m \cos\theta \, d\theta = \alpha \]

The A_1 term is given by

    \[ A_1 = \frac{2}{\pi} \int_0^\pi \frac{dy}{dx} \cos\theta \, d\theta = \frac{2}{\pi} \int_0^\pi 4y_m \cos^2\theta \, d\theta = \frac{2}{\pi} 4y_m \int_0^\pi \frac{1 + \cos(2\theta)}{2} \, d\theta \]

Separating the integral gives

    \[ A_1 = \frac{2}{\pi} 4y_m \left( \int_0^\pi \frac{1}{2} \, d\theta + \int_0^\pi \frac{\cos(2\theta)}{2} \, d\theta \right) \]

Because \displaystyle{ \int_0^\pi \cos(2\theta) \, d\theta = 0 }, it simplifies to

    \[ A_1 = \frac{2}{\pi} 4y_m \left( \frac{\pi}{2} \right) = 4y_m \]

The lift coefficient is given by

    \[ C_l = 2\pi \left( A_0 + \dfrac{A_1}{2} \right) = 2\pi \left( \alpha + 2y_m \right) \]

The moment about the leading edge (LE) is given by

    \[ C_{m,\text{LE}} = -\frac{\pi}{2} \left( A_0 + A_1 \right) = -\frac{\pi}{2} \left( \alpha + 4y_m \right) = -\frac{\pi}{2} \alpha - 2\pi y_m \]

The moment about the quarter-chord (c/4) is given by

    \[ C_{m,\frac{c}{4}} = -\frac{\pi}{4} A_1 = -\frac{\pi}{4} 4y_m = -\pi y_m \]

Worked Example #2 – Two Part Camberline

Consider a NACA 2415 airfoil  two-part camberline given by

    \[ \frac{y_c}{c} = \begin{cases} \dfrac{2m}{p^2} \left(p - \dfrac{x}{c}\right) \dfrac{x}{c}, & 0 \leq \dfrac{x}{c} \leq p \\[16pt] \dfrac{2m}{(1-p)^2} \left(\dfrac{x}{c} - p\right) \left(1 - \dfrac{x}{c}\right), & p \leq \dfrac{x}{c} \leq 1 \end{cases} \]

where m = 0.02 (maximum camber), p = 0.4 (location of maximum camber as a fraction of chord), c = 1.5 \, \mathrm{m} (chord length). The airfoil operates at an angle of attack \alpha = 5^\circ. Using the thin airfoil theory:

  1. Calculate the lift coefficient, C_l.
  2. Determine the moment coefficient about the aerodynamic center, C_{m,\text{ac}}.
  3. Find the moment coefficient about the leading edge, C_{m,\text{LE}}.
Show solution/hide solution.

The slope of the camberline is obtained by differentiating y_c with respect to x/c. For the two segments:

    \begin{align*} \text{\small For } 0 \leq \frac{x}{c} \leq p: & \quad \frac{dy_c}{dx} = \frac{2m}{p^2} \left(p - 2\frac{x}{c}\right), \\[12pt] \text{\small For } p \leq \frac{x}{c} \leq 1: & \quad \frac{dy_c}{dx} = \frac{2m}{(1-p)^2} \left(2\frac{x}{c} - p - 1\right). \end{align*}

Substituting m = 0.02 and p = 0.4, gives

    \begin{align*} \text{\small First segment: } & \quad \frac{dy_c}{dx} = 0.25 \left(0.4 - 2\frac{x}{c}\right) \\[12pt] \text{\small Second segment: } & \quad \frac{dy_c}{dx} = 0.555 \left(2\frac{x}{c} - 1.4\right) \end{align*}

The lift coefficient is given by

    \[ C_l = 2\pi \alpha + 2\pi \left(\frac{m}{p} - \frac{m}{1-p}\right) \]

Substituting the known values gives

    \[ C_l = 2\pi (0.0873) + 2\pi \left( \frac{0.02}{0.4} - \frac{0.02}{0.6}\right) = 0.653 \]

The moment coefficient about the aerodynamic center is

    \[ C_{m,\text{ac}} = -\pi \left( \frac{m}{p} - \frac{m}{1-p} \right) = -\pi \left( 0.05 - 0.0333 \right) = -0.0524 \]

The moment coefficient about the leading edge is

    \[ C_{m,\text{LE}} = C_{m,\text{ac}} + \frac{1}{4}C_l = -0.0524 + \frac{1}{4}(0.653) = 0.1106 \]

Zero-Lift Angle of Attack

The lift coefficient of a cambered airfoil is given by

(54)   \begin{equation*} C_l = 2 \pi \left( \alpha - \alpha_0 \right) = 2 \pi \left( A_0 + \dfrac{A_1}{2} \right) \end{equation*}

where \alpha_0 is called the zero-lift angle of attack. For a symmetric airfoil, \alpha_0 = 0, and for a cambered airfoil the thin airfoil theory gives

(55)   \begin{equation*} \alpha_0 = -\frac{1}{\pi} \int_0^\pi \frac{dy}{dx} \cos\theta \, d\theta \end{equation*}

The zero-lift angle is usually a small negative number for most airfoils, as shown in the figure below. The camber shifts the lift curve upward on the plot, meaning that a cambered airfoil must have its angle of attack depressed to a lower value for no lift generation.

Adding camber or a flap shifts the angle of attack for zero lift to a negative value. A flap is very effective in increasing the maximum lift coefficient of an airfoil section.

Airfoil with a Flap

An airfoil with a flap is a standard aerodynamic configuration that controls lift and pitching moments. The geometry of this configuration is shown in the figure below. The value of x_f represents the non-dimensional hinge point of the flap. It is the location along the chord line, measured as a fraction of the chord length, where the flap is hinged. The flap deflection angle, \eta_f, is small and measured in radians. Positive values of \eta_f indicate a downward deflection of the flap, increasing the camber of the airfoil.

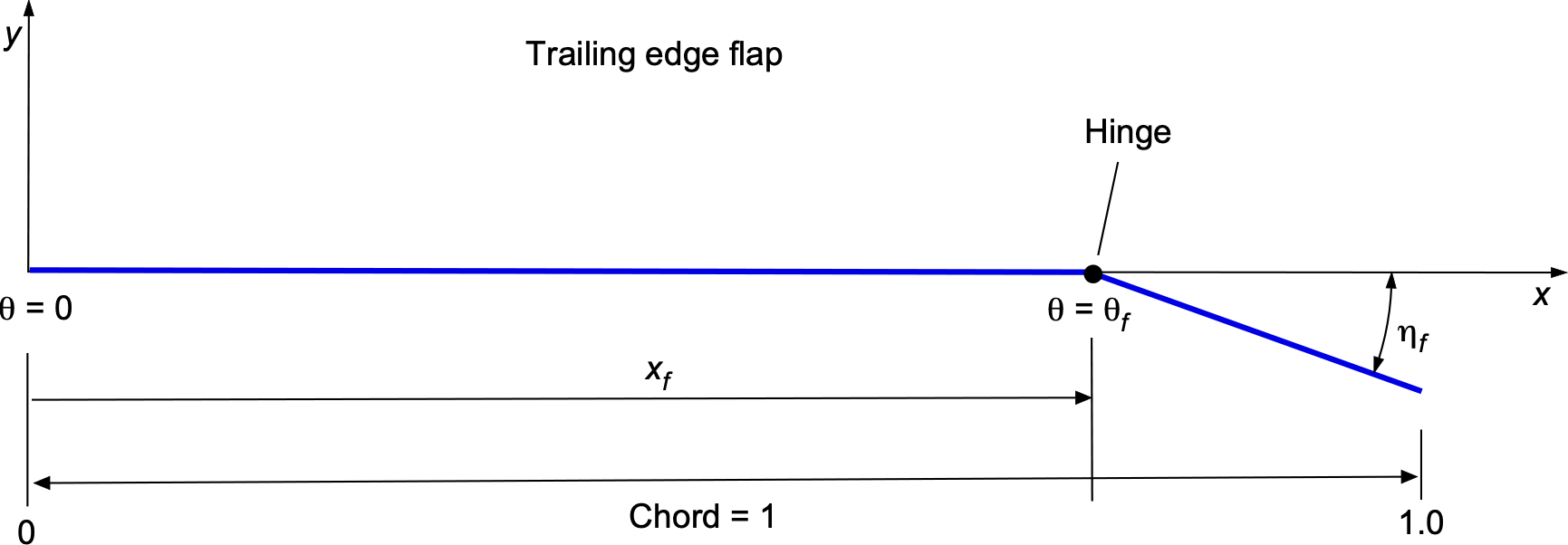
Thin airfoil with a trailing edge flap.

The slope of the camberline, which describes the shape of the airfoil’s mean camber, is

(56)   \begin{equation*} \frac{dy}{dx} = \begin{cases} 0 & \text{for } 0 \leq x \leq x_f \\[9pt] \eta_f & \text{for } x_f \leq x \leq 1 \end{cases} \end{equation*}

The camberline is flat, i.e., \dfrac{dy}{dx} = 0, from the leading edge to the flap hinge point, x_f, and then it has a constant slope of \eta_f from the hinge point to the trailing edge. Notice that \theta_f = \cos^{-1}(1 - 2x_f) is the angular location of the flap hinge, determined by the hinge location x_f.

The Fourier coefficients for the camberline slope are found by integrating over the range [\theta_f, \pi], giving

(57)   \begin{equation*} A_0 = \frac{1}{\pi} (\pi - \theta_f) \eta_f \quad \text{and} \quad A_n = \frac{2}{\pi} \left( \frac{\sin n \theta_f}{n} \right) \eta_f \end{equation*}

and so for A_1 and A_2, then

(58)   \begin{equation*} A_1 = \frac{2}{\pi} \sin \theta_f \, \eta_f \quad \text{and} \quad A_2 = \frac{1}{\pi} \sin 2\theta_f \, \eta_f \end{equation*}

The lift coefficient is given by

(59)   \begin{equation*} C_l = 2 \eta_f \left( 1 - \frac{\theta_f}{\pi} + \frac{\sin\theta_f}{\pi} \right) \end{equation*}

The moment coefficient about the leading edge is given by

(60)   \begin{equation*} C_{m_{\rm LE}} = -\eta_f \left( 1 - \frac{\theta_f}{\pi} + \frac{2\sin\theta_f}{\pi} - \frac{\sin 2\theta_f}{2\pi} \right) \end{equation*}

and the moment coefficient about the quarter-chord is given by

(61)   \begin{equation*} C_{m_{1/4}} = C_{m,\text{LE}} + \frac{1}{4} C_l \end{equation*}

Substituting the expressions for C_{m,\text{LE}} and C_L, gives

(62)   \begin{equation*} C_{m_{1/4}} = \eta_f \left( \frac{\theta_f}{2\pi} - \frac{3\sin\theta_f}{2\pi} + \frac{\sin 2\theta_f}{2\pi} - \frac{1}{2} \right) \end{equation*}

This flap configuration is widely used in aircraft control surfaces (e.g., elevators, ailerons, flaps) and can be optimized for specific flight conditions or performance goals. Notice that lift increases from the contributions from A_0 and A_1, capturing the effect of flap deflection \eta and hinge location \theta_f. The leading-edge moment reflects the influence of flap deflection. Smaller values of \theta_f (hinge near trailing edge) increase flap effectiveness, while larger values of \theta_f (hinge closer to the leading edge) reduce it.

Modeling Drag

In potential flow, which assumes an inviscid, irrotational, and steady fluid, drag is theoretically absent, which, as previously discussed, is known as d’Alembert’s paradox. This outcome occurs because the pressure distribution around a body in such a flow is chordwise symmetric, resulting in no net force opposing motion. Potential flow does not account for effects like viscosity or flow separation, which contribute to drag. Consequently, while potential flow theory is insightful for understanding flow patterns and lift, it cannot predict drag. Therefore, the drag on an airfoil is zero according to the thin airfoil.

Viscous effects must be considered to address this limitation. The problem has two aspects: 1. The effects of viscosity on the pressure drag and 2. The production of shear (skin friction) drag. At a minimum, boundary layer theory must be used to model the effects of viscosity near the surface to obtain the skin friction drag and the contribution of pressure drag, i.e., because of boundary layer displacement effects.

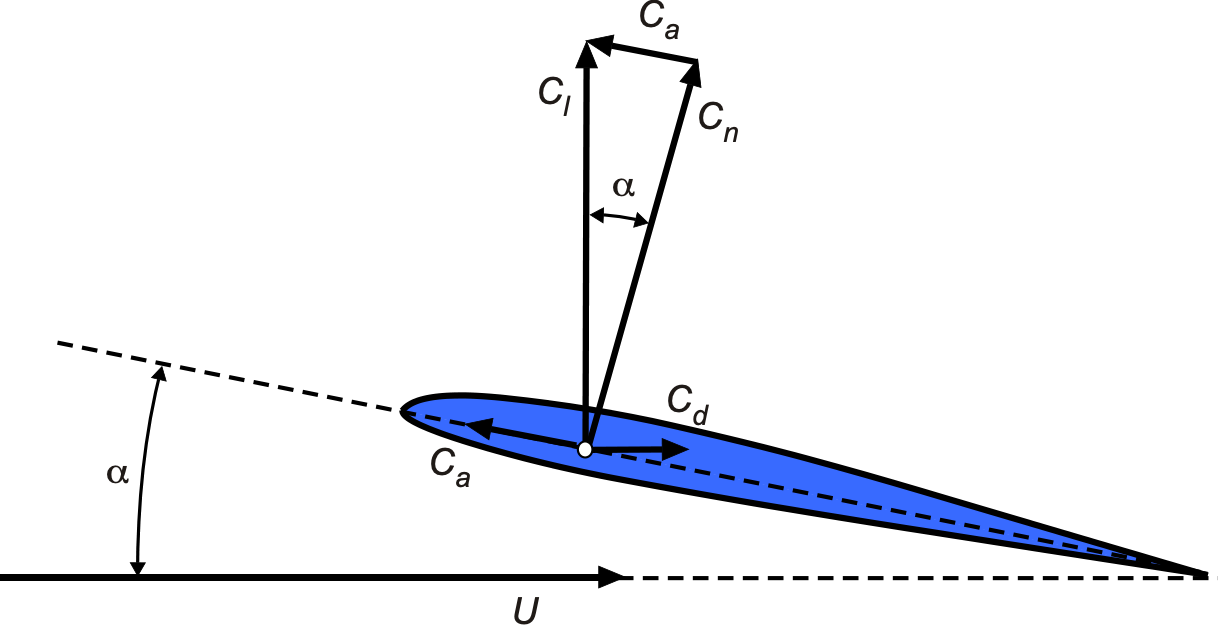
For the pressure drag, consider the following approach by resolving the components of the normal force and axial chord force (or leading edge suction force) through the angle of attack, i.e., in the coefficient form, then

(63)   \begin{equation*} C_{d} = C_n \sin \alpha - C_a \cos \alpha \end{equation*}

where C_n is the normal force coefficient, and C_a is the leading edge suction force, as shown in the figure below. Notice that the normal force acts normal or perpendicular to the airfoil’s chord, and the leading edge suction force acts parallel to the chord.

The chord force or “leading edge suction force” acts parallel to the chordline of the airfoil.

The general expression to determine the leading edge suction force is

(64)   \begin{equation*} C_a = \frac{\pi}{8} \lim_{\bar{x} \rightarrow 0} \left( \Delta C_p^2 \, \bar{x} \right) \end{equation*}

which is a limiting process because of the singular nature of the pressure at the sharp leading edge.[8] A formal theoretical derivation of this result involves the integration of the pressure singularity around the leading edge, which gives a finite suction force proportional to the square of the leading edge singularity. In essence, the term \Delta C_p^2 arises in calculating the suction force because the suction force depends on the localized energy density contribution near the leading edge. Dynamic pressure is proportional to the square of the velocity, i.e., q \, \propto \, \dfrac{\text{velocity}^2}{2}. From Bernoulli’s equation, velocity differences in the flow correspond to pressure differences, i.e., \Delta C_p \, \propto \, \text{velocity difference}. Therefore, \Delta C_p^2 is proportional to the energy contribution per unit area (pressure difference squared).

The thin airfoil theory gives the chordwise pressure distribution as

(65)   \begin{equation*} \Delta C_p (\bar{x} ) = 4 \alpha \sqrt{ \dfrac{1 - \bar{x}}{\bar{x} }} \end{equation*}

so the leading edge suction force is

(66)   \begin{equation*} C_a = \frac{\pi}{8} \lim_{\bar{x} \rightarrow 0} \left( 4 \alpha \sqrt{ \dfrac{1 - \bar{x}}{\bar{x} }} \right)^2 \, \bar{x} \end{equation*}

which gives

(67)   \begin{equation*} C_a = \frac{\pi}{8} \lim_{\bar{x} \rightarrow 0} \bigg( 16 \alpha^2 \left( 1 -\bar{x} \right) \bigg) = 2 \pi \alpha^2 \end{equation*}

The pressure drag can now be obtained by force resolution using

(68)   \begin{equation*} C_{d} = C_n \sin \alpha - C_a \cos \alpha \end{equation*}

where to a small angle approximation C_n = 2 \pi \alpha, \cos \alpha = 1, and \sin \alpha = \alpha. Therefore,

(69)   \begin{equation*} C_{d} = C_n \, \alpha - C_a = (2\pi \alpha) \alpha - 2\pi \alpha^2 = 2\pi \alpha^2- 2\pi \alpha^2 = 0 \end{equation*}

which, as expected, is zero for a potential flow.

However, in a real flow, it can be expected that the effects of finite airfoil shape and viscosity will limit the leading edge suction pressure to a value less than the theoretically achievable value, as shown in the figure below. This effect can be represented using a leading edge suction efficiency or recovery factor, \eta_a, such that \eta_a < 1. In this case, then

(70)   \begin{equation*} C_{d} = C_n \sin \alpha - \eta_a \, C_a \cos \alpha\approx 2\pi \alpha^2 - \eta_a \, 2\pi \alpha^2= 2\pi \left( 1 - \eta_a \right)\alpha^2, \quad \text{which is greater than 0} \end{equation*}

Therefore, the pressure drag is non-zero in an actual flow, as expected, and increases proportionally with \alpha^2, i.e., quadratically. Notice that if \eta_a = 1.0, then C_d = 0, thereby recovering the potential flow solution.

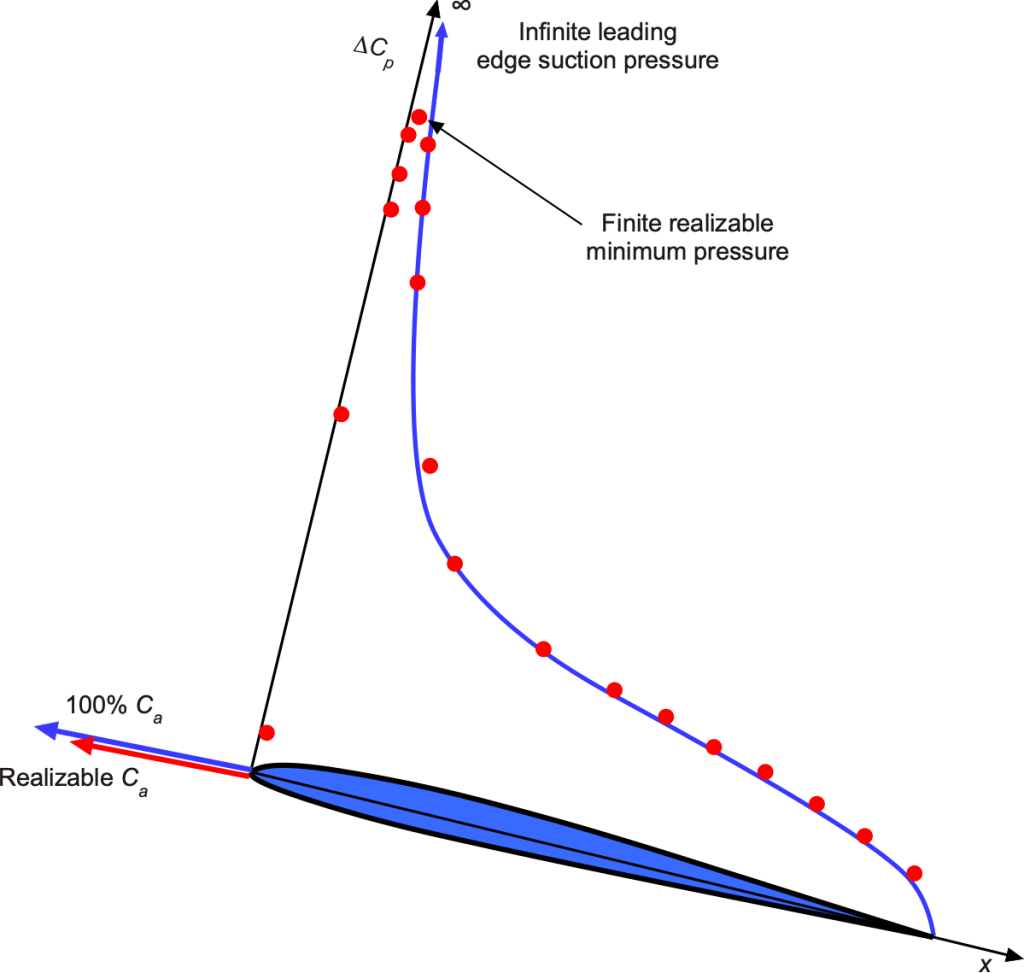
The effects of viscosity and finite thickness will limit the leading edge suction to a value somewhat less than the theoretical value.

However, the limitation of this approach is that the value of \eta_a is not known a priori and must be assumed or estimated. Experience with drag modeling on airfoils suggests that \eta_a \approx 0.95 for airfoils with low to moderate values of the thickness-to-chord ratio, i.e., t/c < 0.12, and lower for airfoils with greater thickness and/or a larger nose radius.

In general, for a cambered airfoil then C_n \approx 2\pi (\alpha - \alpha_0), so that the drag is

(71)   \begin{equation*} C_{d} = 2\pi \left( \alpha - \alpha_0 \right) \sin \left( \alpha - \alpha_0 \right) - \eta_a \, C_a \cos \alpha \approx 2\pi \left( \alpha - \alpha_0 \right)^2 - \eta_a \, 2 \pi \alpha^2 \end{equation*}

and collecting the other terms gives

(72)   \begin{equation*} C_{d} = 2\pi \alpha_0^2 - 4 \pi \alpha_0 \, \alpha + \left( 1 - \eta_a \right) \alpha^2 \end{equation*}

which is of the form

(73)   \begin{equation*} C_d = C_{d_{1}} + C_{d_{2}} \alpha + C_{d_{3}} \alpha^2 \end{equation*}

Equation 73 is a good approximation to the pressure drag behavior with the angle of attack of most airfoils in the low-speed flow regime, which is referred to as the “Modified thin airfoil theory” shown in the figure below.

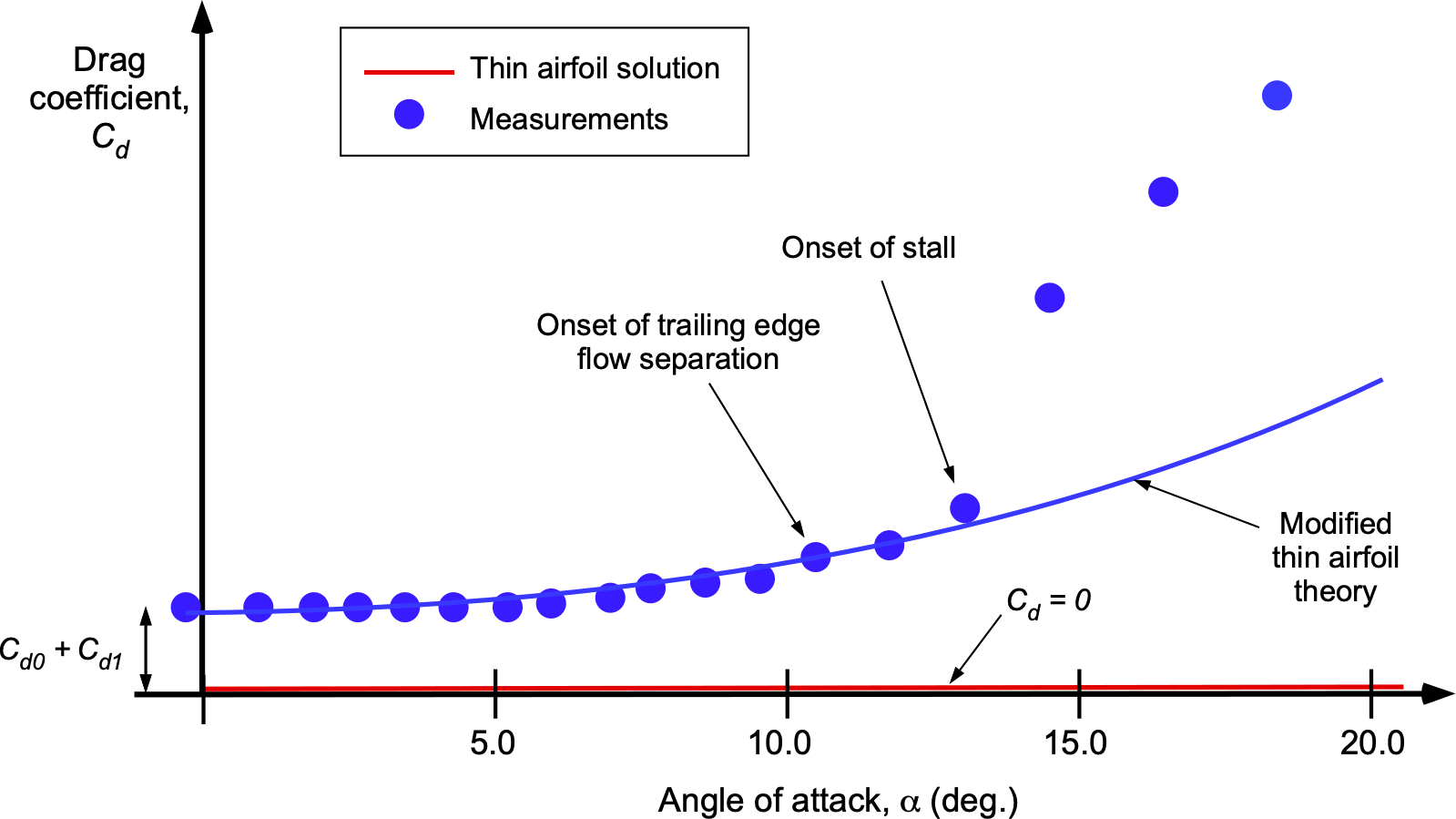
While the thin airfoil theory gives zero pressure drag, a modification based on practically realizable leading edge suction can be used to successfully modify the theory.

The effect of viscous shear drag (boundary layer drag) can be approximated by adding another (constant) term, C_{d_{0}}, i.e.,

(74)   \begin{equation*} C_d = \left( C_{d_{0}} + C_{d_{1}} \right) + C_{d_{2}} \alpha + C_{d_{3}} \alpha^2 \end{equation*}

Equation 74 is valid only in the regimes where the flow is fully attached. This result also applies more to higher chord Reynolds numbers where the boundary layer is thinner and conforms closely to the airfoil surface. Notice that C_{d_{1}} = 0 for a symmetric airfoil because \alpha_0 = 0, so that

(75)   \begin{equation*} C_d = C_{d_{0}} + C_{d_{3}} \alpha^2 = C_{d_{0}} + \left( 1 - \eta_a \right) \alpha^2 \end{equation*}

It will be apparent that a drag model such as Eq. 74 is not predictive but postdictive, i.e., after-the-fact modeling. More advanced models, such as XFOIL, could be used to find the coefficients, including C_{d_{0}}. These coefficients can also be found by fitting Eq. 74 from wind tunnel data. However, in terms of an initial predictive capability, it will be sufficient to assume that \eta_a =0.95 for airfoils with low to moderate values of the thickness-to-chord ratio, i.e., t/c < 0.12.

Inverse Airfoil Design

Inverse airfoil design involves determining the shape of an airfoil based on a desired aerodynamic goal, such as a pressure distribution, C_p(x), the idea being outlined in the figure below. Constraints will also be imposed, both aerodynamic and geometric. For example, an aerodynamic constraint may limit the pitching moment about some axis. A geometric constraint may be the minimum or maximum camber or net thickness. The process is performed iteratively, and careful attention must be given to the convergence process.

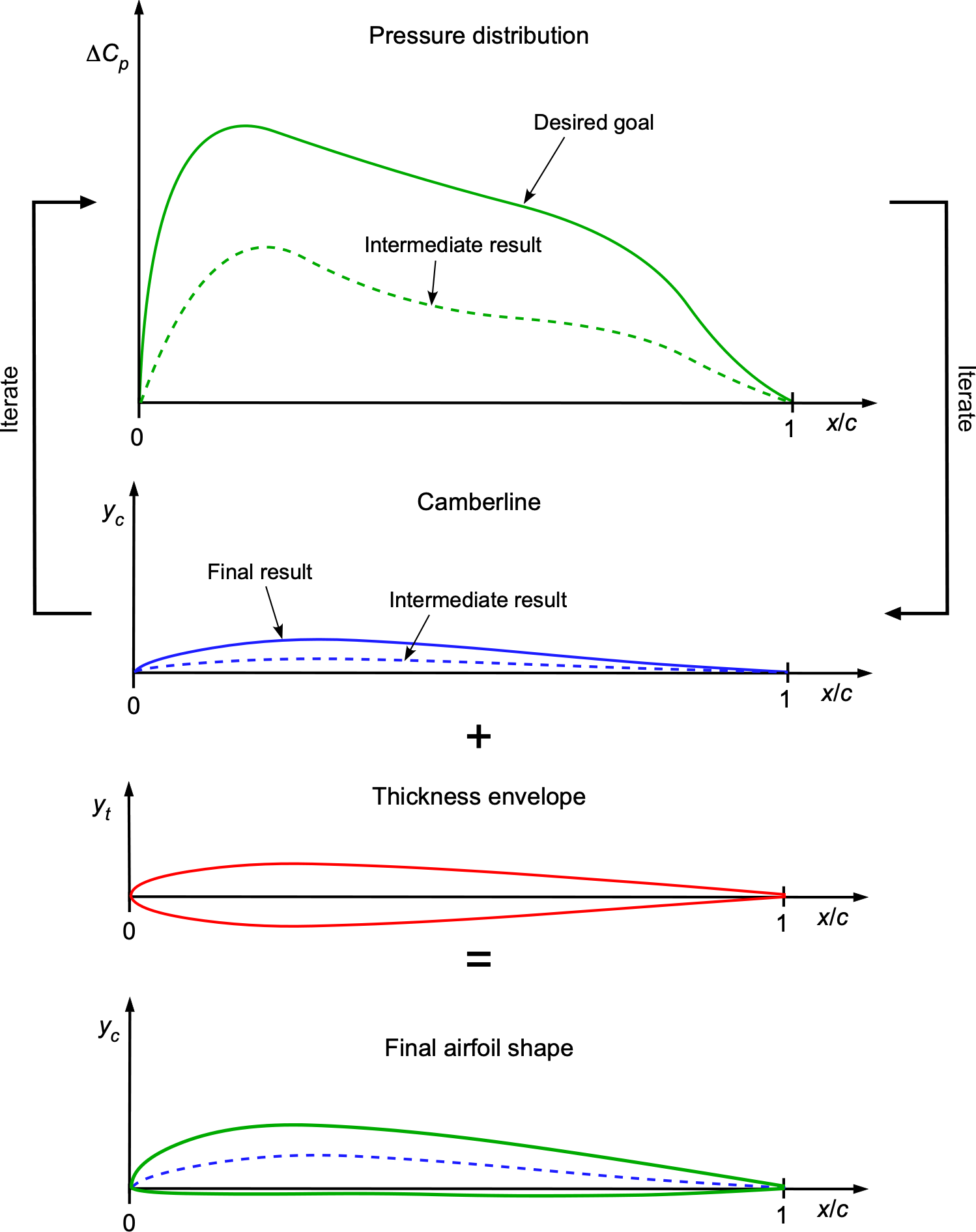
Starting from the fundamental equation of thin airfoil theory, i.e.,

(76)   \begin{equation*} \frac{1}{2 \pi \, U} \int_0^c \frac{\gamma (x)}{x - x_1} \, dx = \alpha -\frac{dy}{dx} \end{equation*}

which can be written in terms of the pressure coefficient as

(77)   \begin{equation*} \frac{1}{2 \pi} \int_0^c \frac{\Delta C_p (x) }{x - x_1} \, dx = \alpha - \frac{dy}{dx} \end{equation*}

The initial slope of the camberline, \dfrac{dy_c}{dx}, is determined from the desired differental pressure coefficient distribution C_p(x) about some reference angle of attack using the equation

    \[ \frac{dy_c }{dx} = \frac{1}{2\pi} \int_0^1 \frac{C_p(x)}{x_1 - x} \, dx \]

The process can be conducted numerically for discrete values of x_1. Sometimes, a characteristic camberline may need to be specified, e.g., parabolic, cubic, two-part, etc., and the iteration performed with the camberline coefficients rather than the camberline shape per se. Notice that in the thin airfoil theory, only the differential pressure, i.e., the difference in pressure between the lower and upper surfaces, can be predicted about the desired operating angle of attack.

Once a first approximation to the slope is obtained, the camberline, y_c(x), is obtained by integration using

    \[ y_c(x) = \int_0^x \frac{dy_c}{dx} \, dx \]

which can again be done numerically for each x1 value across the chord. These latter two steps are then repeated in an attempt to obtain convergence.

The airfoil shape is then defined in terms of the upper and lower surfaces by adding the thickness envelope, i.e.,

    \[ y_u(x) = y_c(x) + \frac{y_t(x)}{2} \quad \text{and} \quad y_l(x) = y_c(x) - \frac{y_t(x)}{2} \]

where y_u(x) is the upper surface of the airfoil and y_l(x) is the lower surface. The thickness distribution, y_t(x), is typically pre-defined or derived from airfoil thickness equations, e.g., the NACA 00XY thickness functions. While a thickness envelope is usually distributed normally (perpendicular) to the camber, when the camberline is thin, there is no practical difference in the resulting airfoil shape.

Of course, this thin airfoil approach only approximates the required shape. The linearization assumptions of the theory will lead to greater uncertainties in shape prediction as the slope of the camberline increases. Still, it often provides a good initial shape for further design optimization, such as using a surface singularity (panel) method or a CFD (grid-based) method.

Summary & Closure

Thin airfoil theory provides analytical solutions for the lift coefficient, moment coefficients, and the aerodynamic center. Notably, the lift coefficient is directly proportional to the angle of attack with a slope of 2\pi per radian, which is the angle of attack and the zero-lift angle of attack. The pitching moment about the quarter-chord point is constant and independent of the angle of attack. The aerodynamic center, where the moment coefficient remains constant, is at the quarter-chord point for thin airfoils. Thin airfoil theory is widely used in preliminary airfoil design. However, its limitations must be acknowledged. It does not account for viscous effects, which are crucial for predicting drag. Nevertheless, some corrections can be applied using the concept of leading-edge suction. It is also invalid for thick airfoils or at high angles of attack where flow separation occurs. Compressibility effects are neglected, limiting its applicability to low-speed incompressible flows. Despite these constraints, thin airfoil theory remains a fundamental tool in aerodynamics, offering a blend of simplicity and utility. It is an important stepping stone for more advanced theoretical studies and bridges toward practical engineering applications of airfoil theory.

5-Question Self-Assessment Quickquiz

For Further Thought or Discussion

  • How does the camberline shape affect the lift slope and moment coefficients in thin airfoil theory?
  • What role does the camber distribution play in determining the zero-lift angle of attack for a cambered airfoil?
  • How does thin airfoil theory account for pitching moments, and why is the moment coefficient about the leading edge different for symmetric and cambered airfoils?
  • How does the thin airfoil theory lift slope of 2π per radian of the angle of attack compared with experimental data?
  • What is the significance of the Kutta condition in thin airfoil theory, and how does it ensure a smooth trailing edge flow?
  • How might the thin airfoil theory be applied to predict the aerodynamic performance of a multi-element airfoil system?
  • Why does thin airfoil theory fail for thick or highly cambered airfoils, and what modifications might improve its applicability?
  • According to Kelvin’s circulation theorem, circulation is conserved. Consider what happens to the flow at the tailing edge if an airfoil is set into impulsive motion and suddenly stops again.

Other Useful Online Resources

To understand more about the thin airfoil theory and how to apply it, then explore some of these online resources:

  1. Munk, Max M. (Max Michael), "Isoperimetrische Aufgaben Aus Der Theorie Des Fluges," Göttingen: Dieterichsche universitäts-buckdruckerei, 1919. ↵
  2. Glauert, H., "The Elements of Aerofoil and Airscrew Theory," Cambridge University Press, 1926 ↵
  3. Notice that lowercase subscripts always indicate the application to a two-dimensional wing or airfoil section. ↵
  4. Other than the effects of viscosity, this flow is equivalent to a singularity in potential flow, ↵
  5. . It cannot be ignored in an unsteady flow analysis, which opens up the field of unsteady aerodynamic theory. ↵
  6. Time is measured non-dimensionally in terms of chord lengths of airfoil travel, which must approach 10 to reach the steady state. ↵
  7. Bairnbaum, W, "Die tragende Wirbelfläche als Hilfsmittel zur Behandlung des ebenen Problems der Tragflügeltheorie," ZAMM, 1923, 3, pp 290–297. ↵
  8. Jones, R. T., "Leading-Edge Singularities in Thin-Airfoil Theory," Journal of the Aeronautical Sciences, Vol. 17, No. 5, May 1950. ↵

License

Icon for the Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License

Introduction to Aerospace Flight Vehicles Copyright © 2022–2025 by J. Gordon Leishman is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License, except where otherwise noted.

Share This Book