Helicopters & Vertical Flight

J. Gordon Leishman

doi:https://doi.org/10.15394/eaglepub.2022.1066.9

61 Helicopters & Vertical Flight

Introduction^[1]

Helicopters are a type of Vertical Take Off and Landing (VTOL) aircraft. They can take off and land from almost anywhere on the ground or at sea, hover motionless in the air, and then fly in any direction at the pilot’s whim. VTOL capability for an aircraft, sometimes referred to as a runway-independent aircraft, is a tremendously helpful aviation asset. However, there is a significant price to pay for VTOL capability in terms of engineering complexity, limited flight range, endurance, and other compromised flight capabilities, as well as much higher acquisition and operational costs.

The development of the helicopter, which is also a type of rotorcraft, lagged behind successful airplanes by almost 30 years. The success of helicopters can be attributed to the continued advancement of aeronautical technologies and scientific understanding. Over time, they have evolved into technologically advanced aircraft with many valuable capabilities that are crucial in multiple roles, including in civil and military service. The versatility and unique capabilities of helicopters have made them indispensable aircraft for rescue operations, medical evacuation, surveillance, policing, border patrol, and defense. Today, any military service would fail to function without access to the helicopter’s unique capabilities.

Modern helicopters use state-of-the-art aerospace technologies, including advanced aerodynamics, composite materials, fly-by-wire (FBW) flight controls, and health and usage monitoring systems (HUMS).

Modern helicopters utilize cutting-edge aerospace technologies to enhance performance, safety, and efficiency. Advances in aerodynamics have led to increased flight performance, while composite materials contribute to weight reduction and improved structural durability. Fly-by-wire (FBW) flight control systems enable greater precision in hovering, station-keeping, and maneuvering flight, significantly improving operational capability. Health and usage monitoring systems (HUMS) also provide real-time tracking of a helicopter’s condition and performance, allowing for proactive maintenance strategies that extend service life and improve reliability. These innovations have solidified the helicopter’s role in aviation, ensuring continued advancements in rotorcraft technology.

In parallel, VTOL jet thrust aircraft have also benefited from technological innovations to improve efficiency, maneuverability, and operational flexibility. Unlike helicopters, which rely on large rotors for lift, VTOL jets achieve vertical takeoff and landing using powerful amounts of jet thrust, which is an inefficient form of thrust generation, so they consume significantly much more fuel. As with helicopters, lightweight composite materials and modular design approaches have enhanced durability and reduced overall weight, contributing to greater fuel efficiency. These innovations have played a crucial role in expanding the operational effectiveness of VTOL jet aircraft, making them increasingly viable for military applications.

Learning Objectives

Know the history and challenges of developing a successful helicopter compared to an airplane.
Better understand the factors that affect the hovering, climbing, and descending flight performance of helicopters and other rotating-wing aircraft.
Understand the essential performance characteristics in forward flight and the potential aerodynamic limitations.
Appreciate how a helicopter is controlled during flight, including using blade hinges and cyclic blade pitch.
Know about other types of VTOL aircraft and their capabilities and limitations.

History

Jacob Ellehammer of Denmark was an aeronautical engineer and one of the pioneers of the helicopter, his coaxial rotor machine being photographed in flight as early as 1913, as shown below. His machine made brief hops off the ground and short hovering flights but could not fly forward or do anything useful. In France, Paul Cornu and Louis Breguet also made notable attempts to build and fly helicopter concepts between 1906 and 1909, but they were failures. The helicopter concepts built by Oehmichen, DeBothezat, and D’Ascanio during the 1920s were somewhat more successful, but their capabilities were marginal. Many other attempts were made to develop and fly helicopters in the following decade, but only with incremental success.

A black and white photograph showing a dual blade concept helicopter in silhouette. — One of the first helicopters, designed by Jacob Ellehammer in Denmark in 1913, made only short hops off the ground.

The first successful rotorcraft concept was not a helicopter but an autogiro with an unpowered rotor called an autogiro. The name “autogiro” comes from the Greek words αὐτός (auto) and γύρος (turning in a circle or forming a disk), essentially meaning a “self-turning” or “autorotating” rotor. “Autogiro” is a proprietary name coined by Cierva, but all aircraft in this class are known as “autogiros.” Today, the FAA refers to such aircraft as gyroplanes. Juan de la Cierva’s fourth attempt to build an autogiro resulted in the C-4, as shown in the photograph below, which first flew in 1923.

A grainy black and white photograph of an aircraft lifting off with one large rotating propeller above the pilot. — Cierva’s C-4 Autogiro was the first successful rotating-wing aircraft, but it could not hover because the rotor was unpowered.

Cierva’s primary innovation was using an independent flapping hinge that attached each blade to the rotating shaft, i.e., a pin-joint. This hinge allowed the blades to move freely up and down out of the plane of rotation (i.e., to flap) to help balance the asymmetric aerodynamic forces over the rotor disk when the machine flew forward. While the autogiro could take off and land at very low airspeeds, essentially a stall-proof aircraft, it could not hover, and the aircraft always required forward and/or downward motion through the air for the rotor to autorotate.

Through the mid-1930s, the autogiro proved its usefulness in various military and civil missions, albeit limited, but it was a helicopter with the ability to hover that was ultimately desired. Nevertheless, the autogiro proved an engineering platform for the helicopter’s future development. Of significance in the development of the autogiro was the development of not just flapping hinges but lead/lag hinges to allow in-plane movement of the rotor blades to alleviate Coriolis forces. Still, Cierva was not to take that critical step toward developing the helicopter.^[2] Notable engineering advancements were made by Raoul Hafner by finally integrating the successful elements of collective and cyclic blade pitch with flapping hinges on his AR-III gyroplane of 1935.

Before the outbreak of WWII, the first technically successful helicopters had started to appear, including the Gyroplane Laboratoire, the Focke-Wulf Fw-61, and the Sikorsky R-4. Germany used several types of helicopters during WWII, albeit in limited numbers. However, it was not until the late 1940s that helicopters became a significant part of the aviation spectrum for military and commercial use. To this end, many advancements in helicopter performance were made during the 1940s and 1950s, much of them in the U.S. The first helicopters included the Bell-47, as shown in the photo below, the Bristol Sycamore, the Sikorsky S-55 and S-61, and the Vertol CH-46.

Photograph of a helicopter on a grey sky background. The helicopter has mostly visible framework and a transparent bubble-style cockpit. — The U.S. military first used the Bell-47 in 1948. It was also the world’s first civil-certified helicopter built in large numbers.

Sustained development in areas of helicopter technology over the last half-century has led to many other successful military and civil helicopter designs. During the 1970s and 1980s, several notable helicopters emerged. The Sikorsky UH-60 Black Hawk is exemplary, which was to become one of the world’s most successful military helicopters and remains in production today. The Boeing AH-64 Apache, first flown in 1975 and introduced in 1986, became the U.S. Army’s primary attack helicopter with its advanced avionics and Hellfire missiles. The Bell AH-1 Cobra and its upgraded Super-Cobra variants continued serving as effective attack helicopters, particularly with the U.S. Marine Corps.

The British Westland Lynx, first flown in 1971, became a highly versatile helicopter used in both land and naval roles, and it was also notable for setting a world speed record for a helicopter in 1986, which still stands today. The Eurocopter AS332 Super Puma, introduced in 1980, became a widely used transport helicopter for military and civilian operations. The Mil Mi-24 Hind, introduced by the Soviet Union in the 1970s, became a heavily armed and armored gunship capable of transporting troops. These helicopters played crucial roles in global military operations. Today, helicopters are increasingly made of advanced composite materials and incorporate many current aerospace technologies to improve their performance, reliability, and safety. For example, the EC-145, as shown in the photograph below, is built primarily of composite materials, which give the airframe strength and lightness. Indeed, modern helicopters have matured into sophisticated aircraft with extraordinary performance capabilities and have a unique role within the aviation spectrum provided by no other aircraft.

A modern helicopter, in this case an EC-145, is built primarily of composite materials, which give the airframe strength and lightness.

One issue with helicopters is that they are inherently low-speed aircraft, with maximum airspeeds of only about 150 knots (173 mph, 278 kph), as shown in the figure below. This speed limitation is partly caused by the rotor system’s conflicting aerodynamic characteristics. Unlike an airplane, the helicopter’s rotor begins to experience both compressibility and stall effects simultaneously. Remember that an airplane will only experience stall at low airspeeds and compressibility effects at high airspeeds, so in this regard, the helicopter is very unique.

While the attainable speed of helicopters has improved over the decades, they have reached a plateau because of physical limitations.

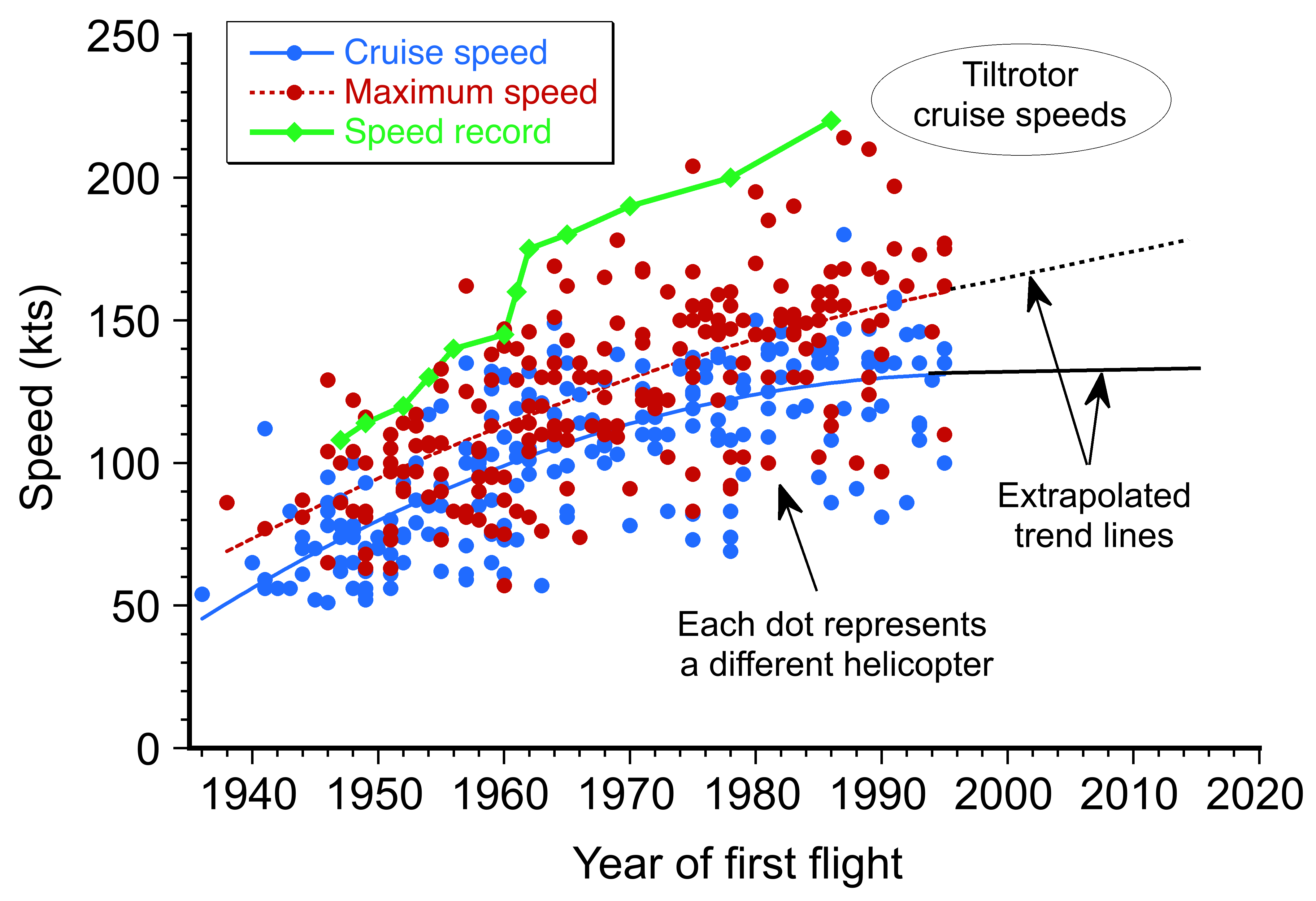
To overcome such inherent limitations, some helicopters have been fitted with auxiliary fixed wings or a separate propulsion system, which are known as compound helicopters. These systems can allow them to fly faster, but usually at the expense of more power and fuel, and carrying less payload. The Sikorsky S-97, as shown below, includes variable-speed rigid coaxial main rotors and a variable-pitch pusher propeller, making the S-97 a compound helicopter. The close spacing of the rotor hubs reduces drag in forward flight. The propeller relieves the rotor of propulsion demands, allowing the helicopter to achieve higher airspeeds.

The Sikorsky S-97 is a compound helicopter with variable-speed rigid coaxial main rotors and a pusher propeller.

Hybrid rotorcraft such as the notorious Bell-Boeing V-22 Osprey and the long-awaited Leonardo/AgustaWestland AW609 (formerly the Bell/Agusta BA609) attempt to combine the vertical takeoff and hover capabilities of helicopters with the increased speed and efficiency offered by airplanes. However, tiltrotors are not as good as helicopters for the things a helicopter does well (e.g., to hover or operate at low airspeeds) and also not as good as airplanes for the things airplanes do well (e.g., flying faster over longer distances when carrying a significant payload). Nevertheless, the V-22 Osprey tiltrotor has proven helpful for particular military missions. Whether the tiltrotor can succeed in the civil market remains to be seen, partly because its role is unclear, and, by any standard, it will have much higher acquisition and operational costs than an airplane or a helicopter.

A tiltrotor attempts to combine the benefits of a helicopter with those of an airplane, although it cannot match the unique capabilities of either.

Vertical Takeoff and Landing (VTOL) airplanes, other than helicopters, have a long history as engineers sought aircraft capable of operating without long runways. Early experimental designs in the 1950s and 1960s, such as the British Hawker Siddeley P.1127, which evolved into the Harrier Jump Jet, successfully demonstrated vectored-thrust technology, allowing jets to hover and transition to forward flight. Around the same time, the U.S. and Germany experimented with tail-sitting VTOL aircraft like the Convair XFY Pogo and Dornier Do 31, though these designs proved impractical. Modern VTOL designs like the F-35B Lightning II, which employs a lift fan and a swiveling engine nozzle, represent the state-of-the-art in VTOL capability.

Drones or unoccupied aerial vehicles (UAV), more generally known as unoccupied aerial systems (UAS), have become part of the rotating-wing aircraft spectrum. These aircraft use rotors and/or propellers explicitly designed for VTOL capability. UAVs are preferred for D³ or “Dull, Dirty, & Dangerous” missions. UAVs originated for military applications, but their use has recently expanded almost exponentially for numerous civil applications. The quad-rotor or “quadcopter” design has become a prevalent configuration for smaller UAVs. Most UAVs carry cameras, although other sensor packages may be used, too. They can be used for surveillance, aerial photography, surveys, disaster relief, etc.

Helicopter Configurations

Early helicopter concepts before 1930 were of the coaxial or side-by-side (lateral) rotor configuration; the various rotor configurations are shown in the figure below. The most straightforward idea of using a single main rotor with a smaller, sideward-thrusting tail rotor to compensate for torque reaction was not used until much later in the development of the helicopter. Nevertheless, the single main rotor/tail rotor configuration has since become the most common, comprising over 95% of all helicopters currently flying. The tandem rotor design is attractive for helicopters that need to carry more payload, with both rotors providing beneficial lift despite the greater mechanical complexity involved in gearing and controlling the two rotors.

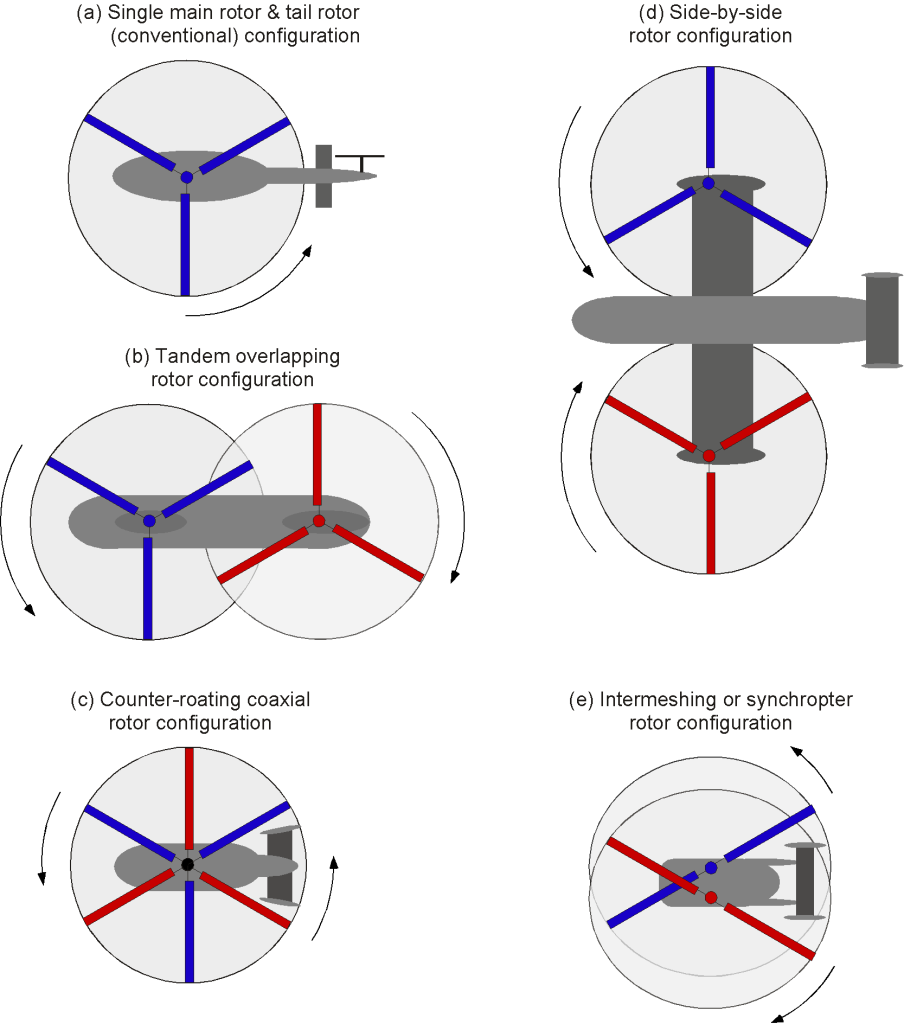
Contra-rotating “coaxial” rotors with one rotor above the other on a concentric shaft automatically balance torque reaction on the airframe, a helicopter configuration made famous laterally by the Kamov company. Another advantage is the compact footprint of a coaxial concept despite the greater mechanical complexity of the two rotors. Side-by-side rotors, especially if the shafts were inclined inwards, gave the early machines somewhat better lateral stability, but the design is uncommon today. One notable example was the enormous Mil MV-12. Again, this type of design has a greater level of mechanical complexity. The intermeshing design has outward-tilted, contra-rotating shafts with intersecting rotor disk planes, such as on the Kaman K-Max. Like the coaxial, the advantage of the intermeshing design is a smaller overall footprint, which is helpful for flight operations out of confined locations.

Basis of Helicopter Flight

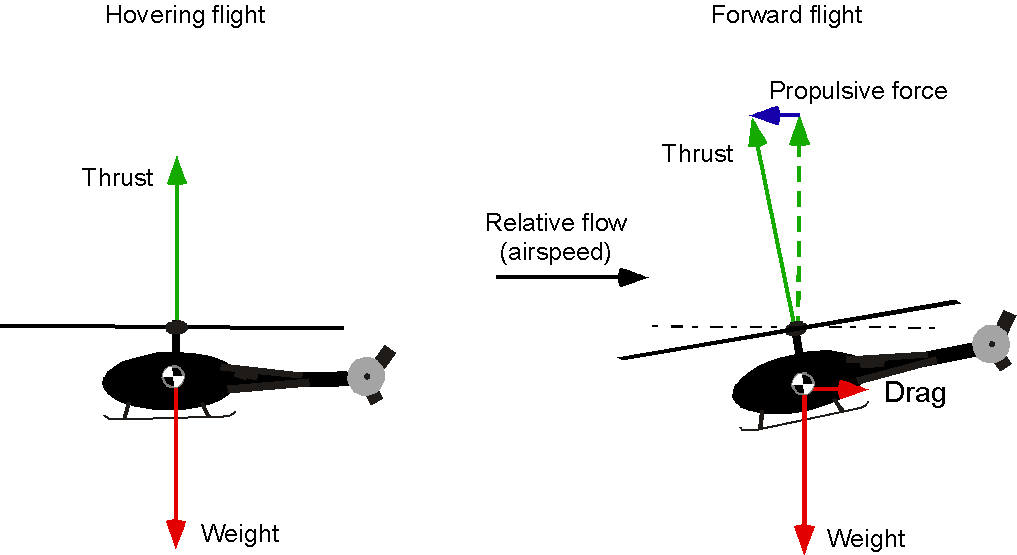
An airplane has separate lift, propulsion, and control systems, while a helicopter’s rotor system must perform all three functions. The rotor blades provide lift to overcome the helicopter’s weight, while the engine provides the power to spin the rotor and generate lift. The helicopter’s control surfaces, such as the swashplate, pedals, and collective, allow for control over the helicopter’s direction and altitude. This makes a helicopter’s design and operation more complex than an airplane’s, as the rotor system must be able to perform multiple tasks simultaneously. Nevertheless, the helicopter’s unique ability to hover and fly vertically makes it ideal for many missions where a fixed-wing aircraft is impractical.

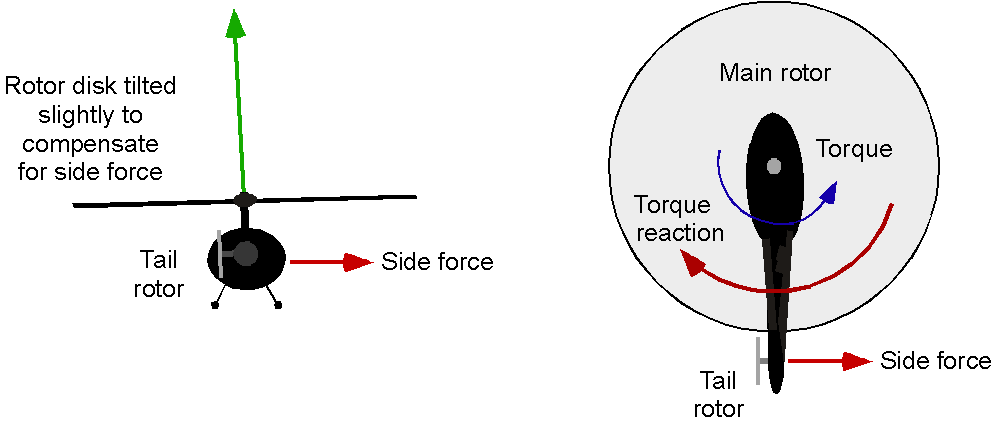
As shown in the figure below, the rotor generates a vertical lifting force (called thrust) in opposition to the helicopter’s weight, which is obtained from the collective lift forces on the spinning rotor blades. The generation of a horizontal propulsive force for forward flight is obtained by tilting the rotor disk plane forward to give a rotor thrust component to overcome the helicopter’s drag, which the pilot accomplishes by actuating the flight controls. The means of controlling a helicopter during its flight are discussed later.

The rotor provides lift to overcome weight but also a propulsive force to overcome the drag in forward flight.

The rotor also generates forces and moments to help control the helicopter’s attitude and position in three-dimensional space. This behavior is obtained by tilting the orientation rotor disk left and right as well as fore and aft, as shown in the figure below. Tilting the disk requires that the blade lift be modulated to cause flapping about the hinges, which results in a moment being applied through the hub and rotor shaft to the fuselage. So, the fuselage quickly aligns with the tilted rotor plane.

Tilting the rotor disk also gives flight control. The resulting forces and moments on the fuselage cause it to align quickly with the rotor plane.

An anti-torque and yaw control system is necessary for a single-rotor helicopter, which is typically achieved with a tail rotor that generates sideward thrust. The pilot can modulate the tail rotor thrust for yaw control. In other rotor configurations, such as coaxial, tandem, and side-by-side, a tail rotor is unnecessary as the net torque reaction is already balanced. However, a slight residual torque reaction can be balanced through the differential tilts of the two rotor disks, i.e., creating a couple.

An anti-torque system is needed to balance the torque reaction on the airframe from the main rotor.

Rotor Flow Environment

The lifting capability of a lifting surface is related to its local angle of attack and flow velocity, specifically the dynamic pressure. In the case of a fixed (non-rotating) wing, the free-stream velocity and the lift are relatively uniform along its span. However, in the case of a rotor, the flow velocity varies linearly along the span because of the rotation. The consequence is that the aerodynamic loads over the rotor disk are much more biased toward the blade tip, as shown in the figure below.

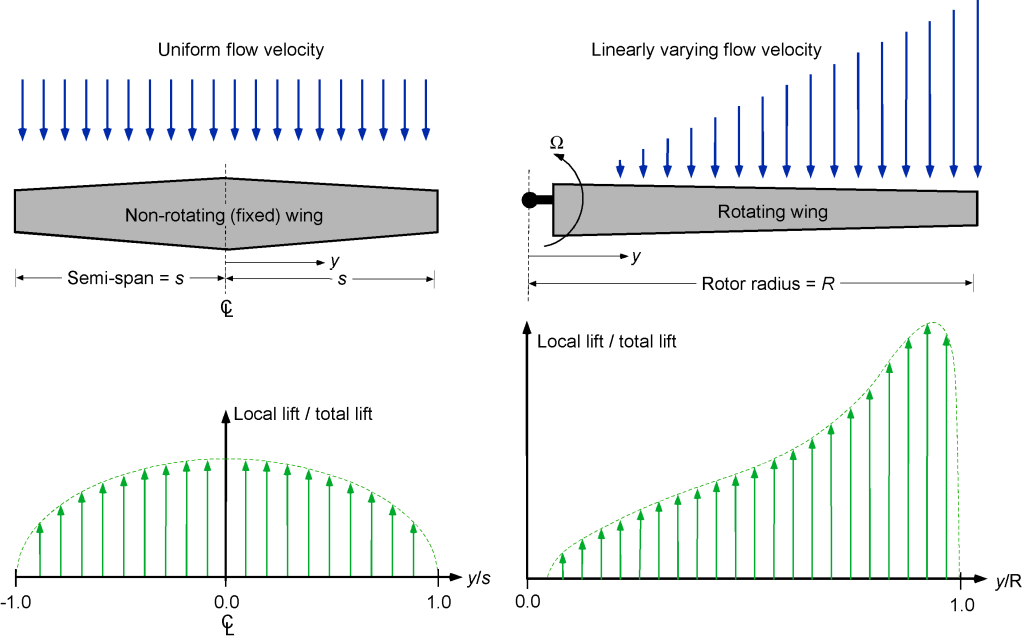
The difference between a non-rotating wing and a rotating wing is that the former needs to move forward to create lift, whereas a rotor will create lift without any forward speed.

A fixed wing must constantly be moving forward to create lift. However, with a rotating wing or rotor blade, which for now can be assumed to be in hovering (non-translating forward) flight, the lift can be generated without any “free-stream” flow or forward motion. There will be no flow velocity at the rotational axis, but the flow velocity will increase linearly along the span of the blade. The velocity will reach a maximum at the blade tip, $V_{\rm tip} = \Omega \, R$ , where $R$ is the rotor radius and $\Omega$ is the rotor’s angular velocity. The consequence of the preceding is that a wing will have a reasonably uniform lift distribution, but a helicopter rotor will have a lift distribution that is much more biased toward the blade tips.

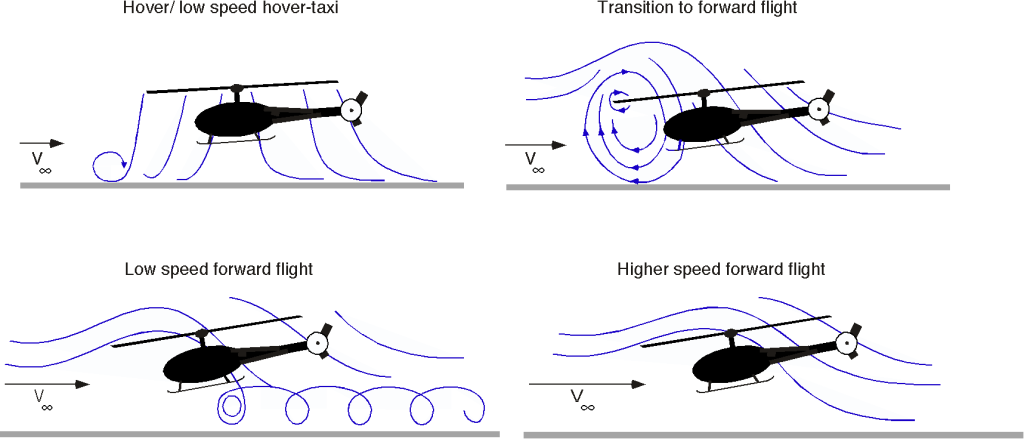
Asymmetry of Aerodynamics

When a rotor flies forward such that there is a component of a “free stream,” the flow over the rotor blades will no longer be axisymmetric about the center of the rotor, as shown in the schematic below. To explain the effects on the aerodynamics, the blade position can be defined in terms of an azimuth angle, $\psi$ , which is defined as zero when the blade is pointing downstream, as shown in the figure below. Now a component of the free stream, $V_{\infty}$ , adds to or subtracts from the rotational velocity at each part of the blade, i.e., for the tip section, then

(1) $\begin{equation*} V_{\rm{tip}} =\Omega R + V_{\infty} \sin \psi \end{equation*}$

and at any radial position a distance ${y}$ from the rotational axis, then

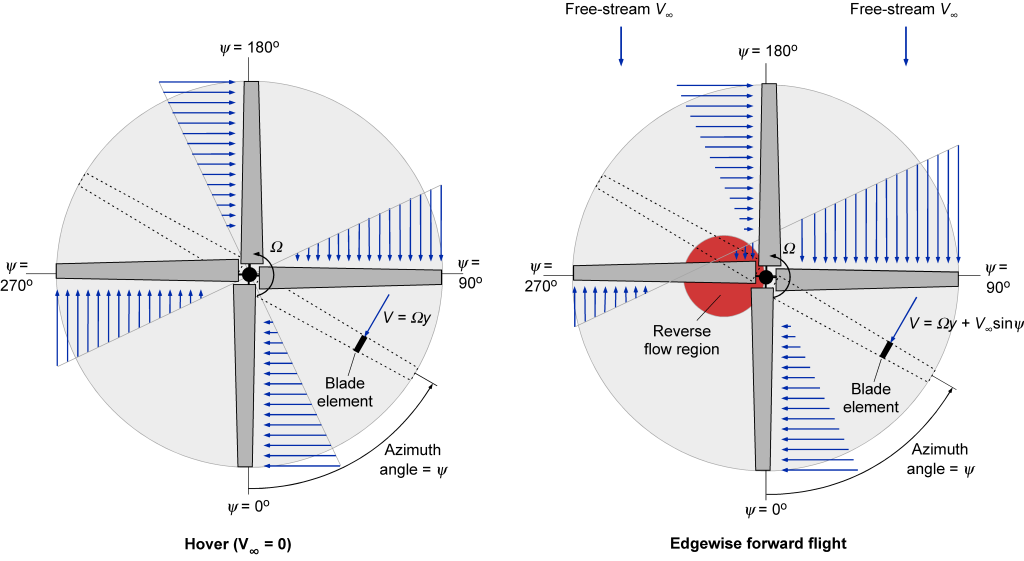
(2) $\begin{equation*} V (y, V_{\infty}) =\Omega y + V_{\infty} \sin \psi \end{equation*}$

The asymmetries in the flow velocities at a rotor in forward flight cause substantially different aerodynamic environments over the rotor disk.

The consequence of this effect is that the rotor disk’s right side (advancing side), where the blades advance into the free-stream flow, will see a higher overall flow velocity and dynamic pressure, resulting in a higher lift. On the rotor disk’s left (retreating) side, with a much lower dynamic pressure, the blades will experience less lift. This problem, often referred to as the “dissymmetry in the lift,” was initially identified by Juan de la Cierva, as explained in the schematic below. Consequently, with blades that are rigidly attached to the rotor shaft, there will be substantial aerodynamic rolling moments on the rotor (turning the rotor toward its retreating side) that will make a rotorcraft of any kind impossible to fly. This problem was resolved by incorporating flapping hinges into the rotor system.

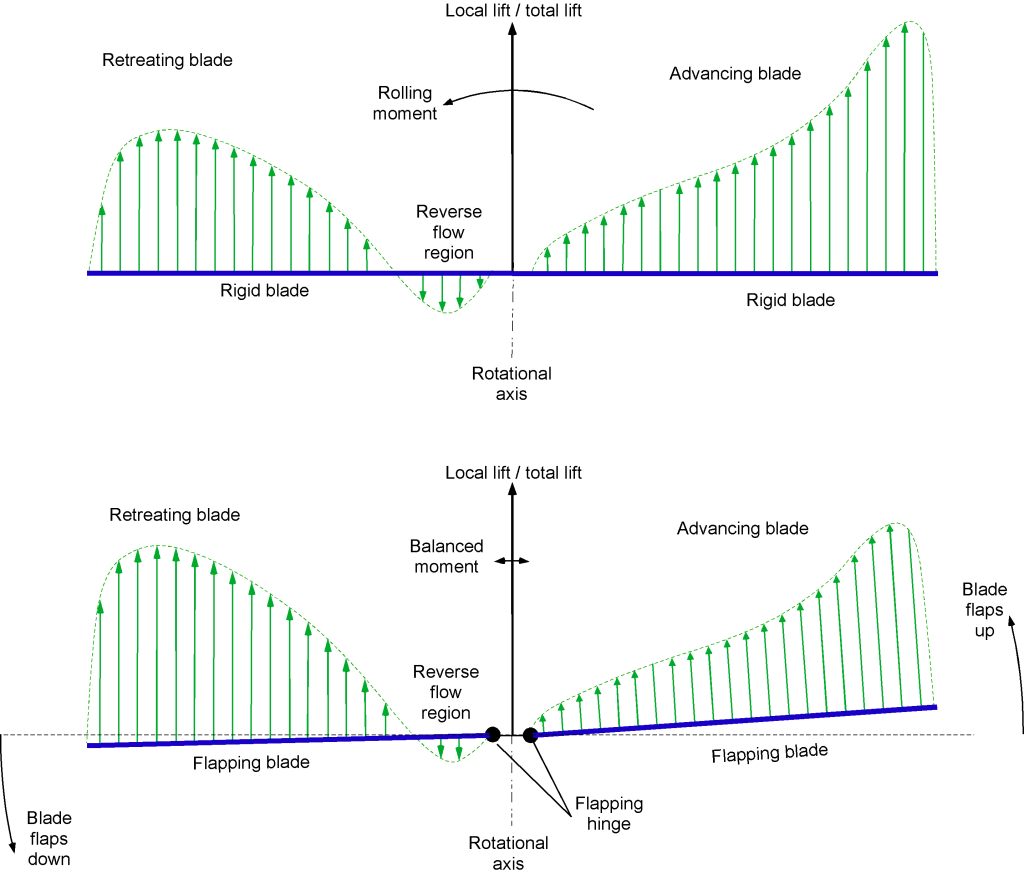
A solution to the “dissymmetry in the lift” problem is to use a flapping hinge on each blade to allow them to respond naturally to the changing flow velocities and balance the airloads

Blade Flapping

Cierva’s solution to this problem was to modulate the asymmetry in blade lift between the two sides of the rotor disk by using a pin-joint or “flap hinge” at the root of each blade, as shown in the figure above. This hinge allowed each blade to move up and down in response to the changing aerodynamic lift, as shown in the schematic below. The effects of blade flapping change the angles of attack on the blades favorably to help balance the distribution of aerodynamic loads over the rotor disk. On the advancing side of the rotor disk, where there is an excess of lift because of the higher dynamic pressure, the blade flaps up about the hinge, decreasing the effective angle of attack and reducing the lift, as shown in the schematic below. With a much lower dynamic pressure, the blade flaps down on the retreating side, increasing the lift there.

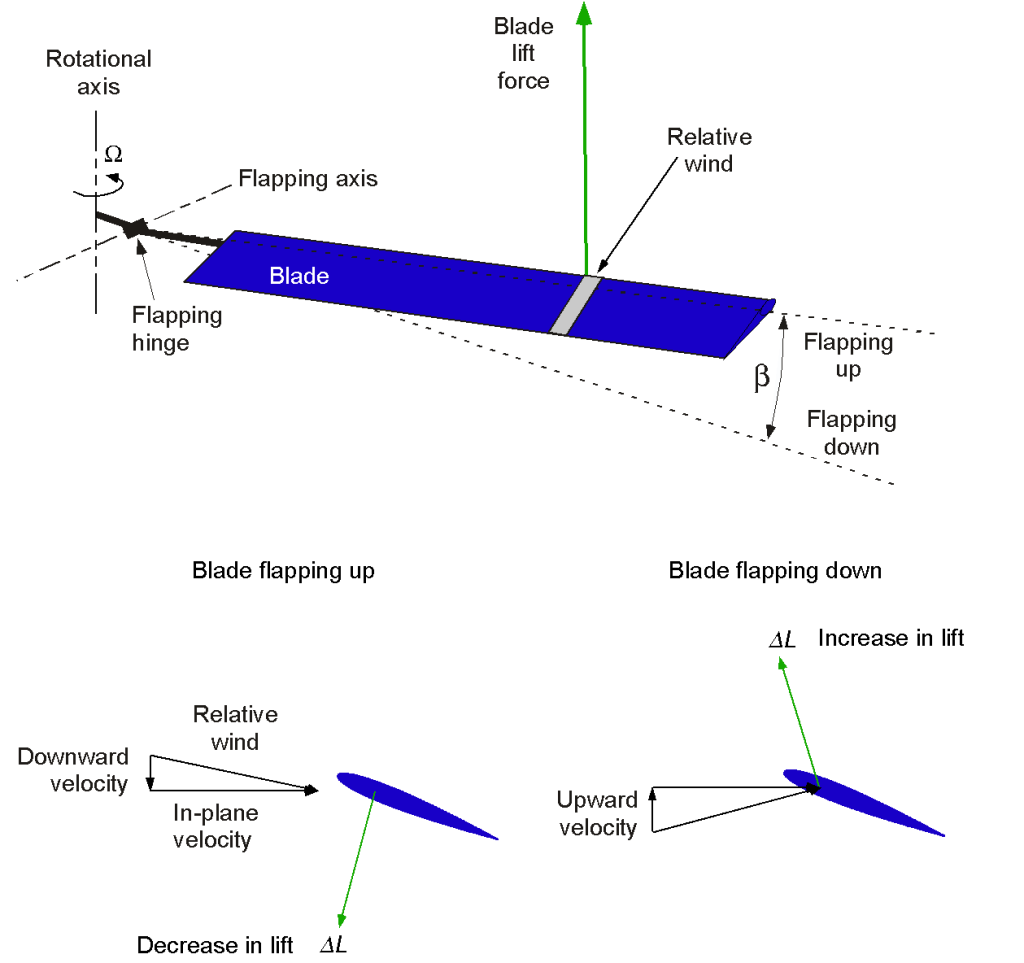
As a blade flaps up and down about the hinge, the changes in angles of attack lift act to oppose flapping, in effect dampening the flapping response.

Therefore, a flapping hinge allows the lift forces and moments created on the advancing and retreating sides to be better balanced overall and over the entire rotor disk. Indeed, Cierva refers to his invention of the flapping hinge as his “secret of success,” which allowed his C-4 autogiro to fly successfully in 1923.

Leading & Lagging

Cierva later introduced a “lead-lag” hinge to alleviate the in-plane inertia loads caused by Coriolis accelerations as the blade flaps up and down. This behavior occurs because, as the blade flaps up and down in the rotational plane, its center of gravity (or radius of gyration) shifts inward and outward relative to the rotational axis. Conservation of angular momentum means there are resulting Coriolis forces in the plane of rotation that can induce significant structural stress on the rotor system. This fundamental behavior is illustrated in the figure below, which shows how a lead-lag motion is caused by blade flapping.

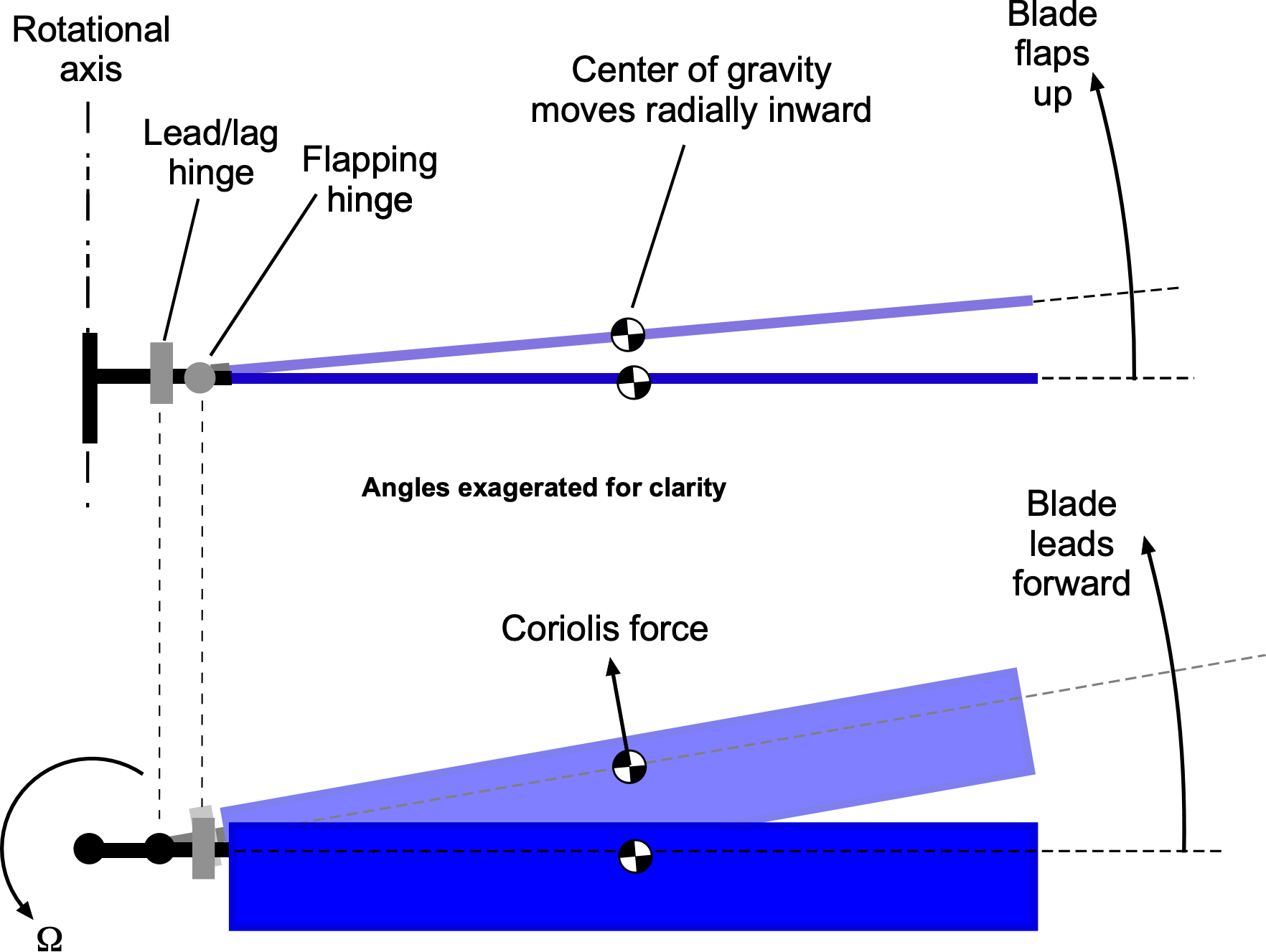
Blade flapping introduces in-plane Coriolis effects that cause in-plane lead-lag motion.

These issues came to a head with the Cierva model C.8, which suffered a catastrophic rotor failure during a test flight in 1927. One of its blades detached mid-flight because of excessive in-plane stresses acting on the rotor hub, a direct result of Coriolis-induced loads that were not fully understood then. To compensate for these forces, the lead-lag hinge allows the blade to move slightly forward and backward in the plane of rotation, dissipating the stresses that the hub structure would otherwise have to absorb. Without this hinge, excessive in-plane bending moments would be transferred to the rotor hub, potentially leading to high structural stresses that can cause fatigue and structural damage over time. Cierva patented the concept, which is shown in the figure below, which turned out to be an essential step in developing the fully articulated rotor system.

Juan de la Cierva patented a system in the 1930s that incorporated lead-lag hinges with flapping hinges.

Blade Pitch

By further modulating the blade pitch angle or “feathering” angle, the lift on the blades can be changed so that the blades will flap up or flap down at the appropriate location around the rotor azimuth, thereby causing the rotor disk plane as a whole to tilt left and right or fore and aft. Tilting the rotor disk gives a basis for controlling the orientation of the rotor thrust vector and the forces and moments acting on the helicopter as a whole. This is called a fully articulated rotor system and proved key to the success of the plane as a viable form of aircraft.

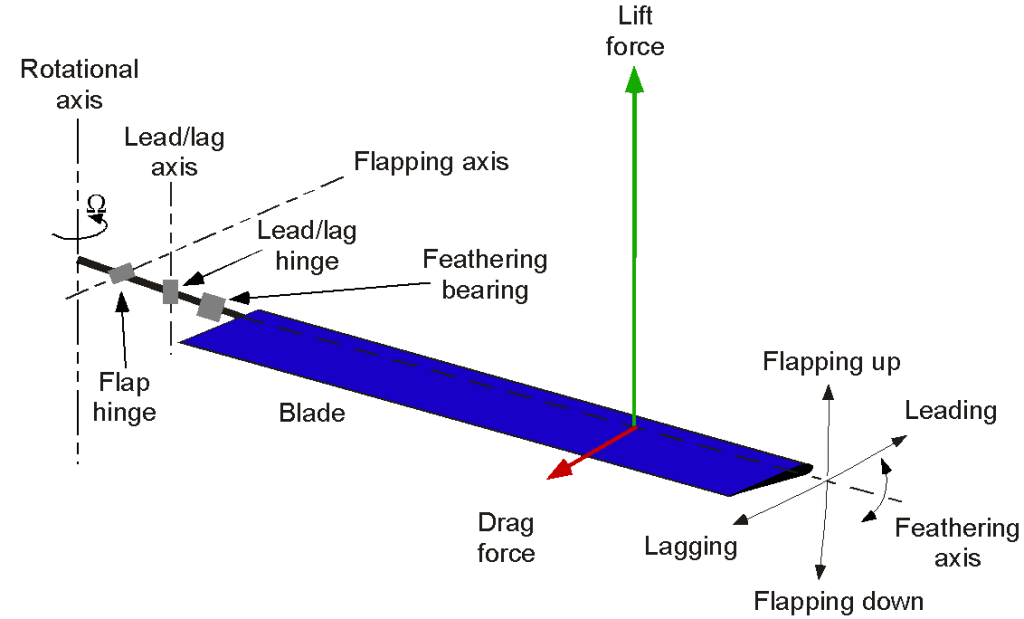
Depending on design requirements, a rotor system’s hinges and feathering bearings can be arranged in different sequences. A fully articulated rotor system typically includes:

Flapping hinges – Allow blades to move up and down, compensating for the dissymmetry of lift.
Lead-lag hinges – Enable in-plane motion to absorb Coriolis forces.
Feathering bearings – Permit cyclic and collective pitch changes, which can be used to control the flapping of the rotor system.

One example of a fully articulated rotor is shown in the photograph below, illustrating how these components are integrated into the rotor hub. In this particular arrangement, [describe notable features of the rotor in the image, e.g., the placement of lead-lag hinges, the orientation of feathering bearings, or any unique design aspects].

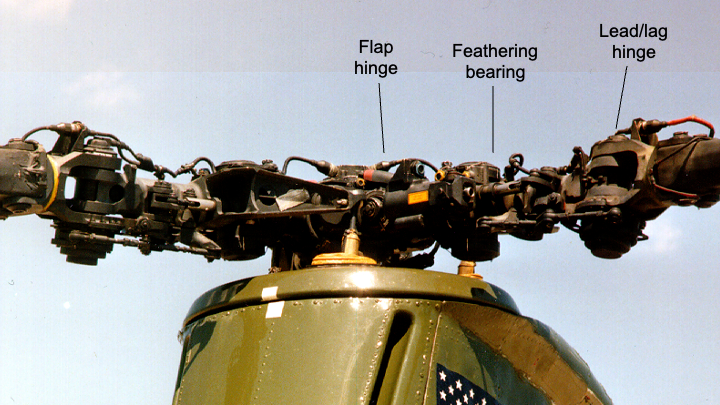
A fully articulated rotor system using flap hinges, feathering bearings, and lead/lag hinges. The combined mechanisms with pitch links, pitch horns, etc., produce a rather complicated-looking rotor hub.

Rotor Wake & Blade Tip Vortices

One consequence of lift generation is that “tip vortices” form and trail from the tip of each rotating blade, just as they would trail from the tips of a wing. The figure below shows an example of the physical nature of the vortical wake generated by a helicopter rotor in hovering flight compared to that of an airplane. In each case, the tip vortices are rendered visible by the natural condensation of water vapor in the air, which leaves wispy white clouds in the flow behind the tips.

The vortical wake generated by a helicopter rotor compared to an airplane’s wake. The tip vortices are rendered visible by the natural condensation of water vapor in the air, forming long, thin white clouds.

For an airplane, the vortices trail downstream and are left behind in the wake, never to influence the wing again, at least directly. However, for a rotor, the vortices are convected downward below the rotor and form a series of interlocking, almost helical, trajectories. Therefore, the rotor blades can encounter their self-generated vortices as well as the vortices generated by other blades, which, overall, creates a much more complex flow environment at the rotor.

For this latter reason, predicting the strengths and locations of the tip vortices plays an essential role in estimating blade airloads and rotor performance. The significant non-uniformity of the angles of attack that the blade sections experience as they sweep around the rotor disk during edgewise forward motion is one complication with the helicopter rotor that makes its aerodynamic analysis difficult.

Hovering Flight Analysis

Unlike airplanes, helicopters can hover, which is a flight condition where they are specifically designed to be operationally efficient. In hovering flight, the primary purpose of the rotor is to provide a vertical lifting force in opposition to the helicopter’s weight. The thrust generation requires torque (and power) to be applied through the rotor shaft. In hover or axial flight, the flow is a nominally axisymmetric streamtube that passes through the rotor disk. This flow regime is the easiest to analyze in the first instance, and, in principle, it should be the easiest to predict using mathematical models.

Although it must be remembered that the actual physical flow through the rotor will generate a complicated vortical wake structure, as previously discussed, the primary performance of the rotor can be analyzed by a more straightforward approach known as the momentum theory. This approach allows predictions of the relationships between rotor thrust and the power required to produce that thrust. Also, it exposes some other parameters that can be used to determine rotor performance and efficiency, including disk loading and blade loading.

Time-Averaged Flow Field

Hover is a unique flight condition where the rotor has zero forward (edgewise) speed and zero vertical speed (no climb or descent). A set of velocity measurements in a diametric plane near a hovering rotor and its wake is shown in the figure below. The flow velocity smoothly increases as it is entrained into and through the rotor disk plane. There is no jump in velocity across the rotor disk, although because a thrust is produced on the rotor, there must be a jump in time-averaged pressure over the disk.

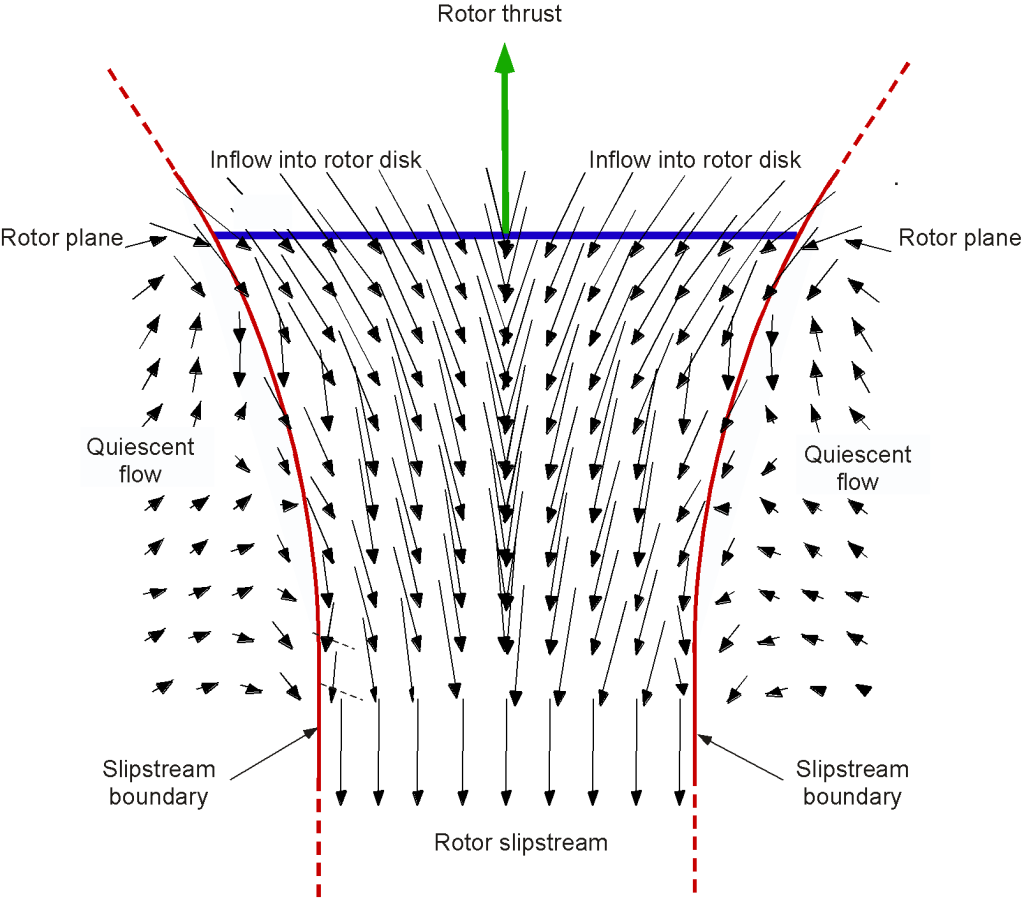
Measurements of the velocity field in a diametric plane near and below a two-bladed rotor operating in hover.

These measurements reveal a clear wake boundary or slipstream, with the flow velocity outside this boundary being relatively calm or quiescent. Inside the wake boundary, the flow velocities are substantial and may be distributed non-uniformly across the streamtube and in the slipstream. Notice also the contraction in the diameter of the streamtube and wake boundary below the rotor, which corresponds to an increase in the slipstream velocity.

Flow Model

With the physical picture of the hovering rotor flow apparent, it is possible to develop a mathematical model. Consider the application of the three basic conservation laws (conservation of mass, momentum, and energy) to the rotor and its flow field. The conservation laws will be applied in a steady, incompressible, inviscid, axisymmetric, one-dimensional integral formulation to a control volume surrounding the rotor and its wake.

This simplified approach permits the most basic analysis of the rotor performance (e.g., to determine the thrust produced and power required) but without considering the details of the flow environment or what is happening locally at each blade section. This approach, called the momentum theory, was first developed by William Rankine in 1865 to analyze marine propellers and formally generalized by Hermann Glauert in 1935 for application to helicopter rotors and autogiros. The assumptions are:

One-dimensional, steady, incompressible, inviscid flow.
The rotor is an infinitesimally thin disk that acts as a pressure discontinuity within the moving fluid.
The disk offers no resistance to fluid passing through it.
The pressure and velocity are uniform over the disk.
The flow is at ambient static pressure far upstream and downstream of the rotor.

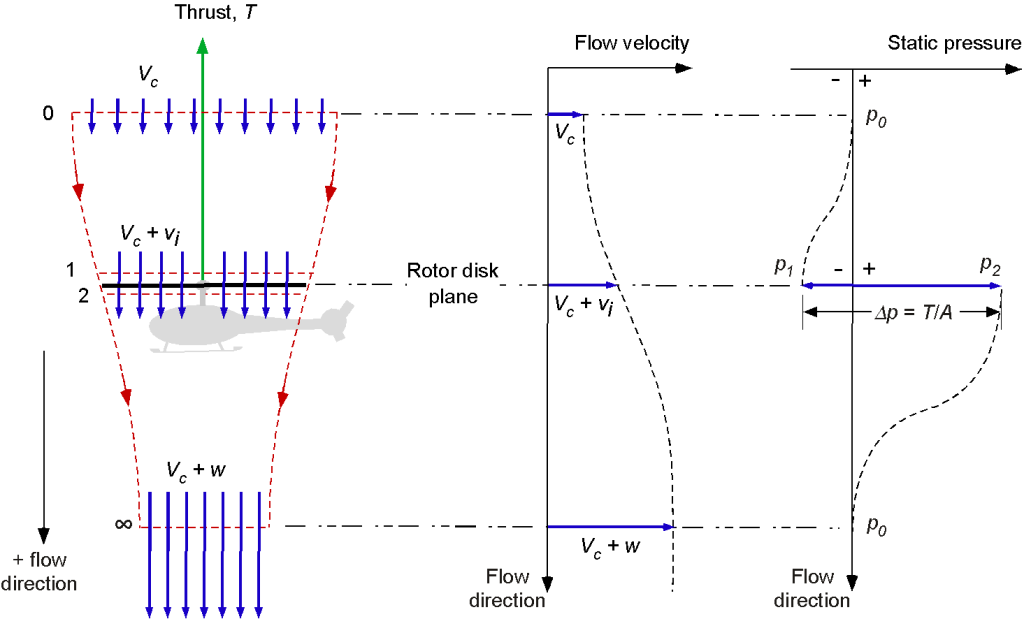
Consider the figure shown below. Let cross-section 0 denote the plane far upstream of the rotor, where the air is still or quiescent. $A$ indicates the rotor disk area. Cross-sections 1 and 2 are the planes just above and below the rotor disk, respectively. The slipstream “far” wake is downstream of the rotor at $\infty$ . The flow through the rotor increases smoothly and continuously, although there must be a jump in static pressure over the disk to create thrust.

A one-dimensional axisymmetric flow model is used to analyze the performance of a hovering rotor.

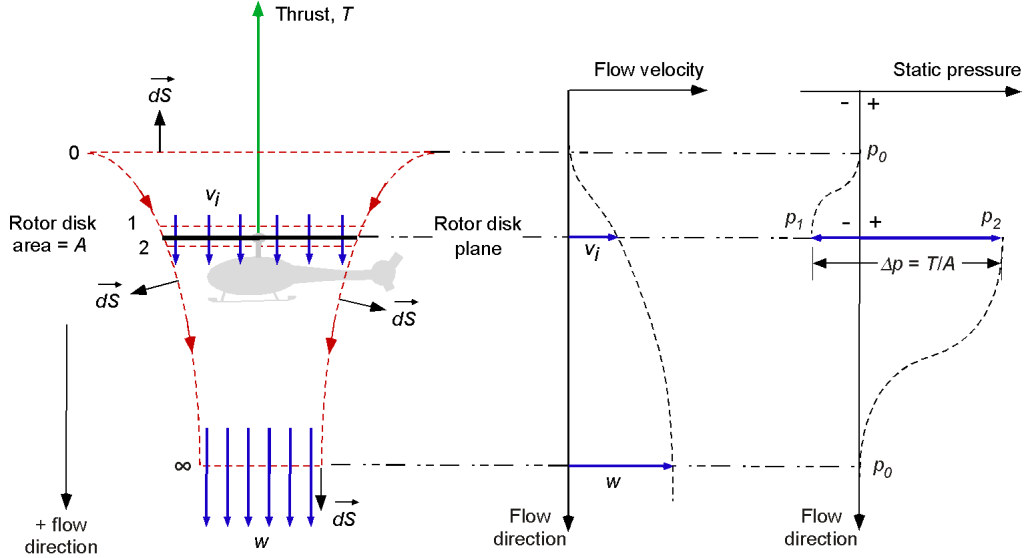
A fundamental assumption in the momentum theory is that the rotor can be idealized as an infinitesimally thin actuator disk supporting a pressure difference; this concept is equivalent to an infinite number of blades of zero thickness. This actuator disk supports the thrust generated by rotating blades about the shaft and their action on the air. The work done by the rotor on the air leads to a gain in kinetic energy of the flow in the rotor’s slipstream, an unavoidable energy loss, and a byproduct of thrust generation called the induced power. By Newton’s third law, the force on the flow means an equal and opposite force is produced on the rotor, i.e., the rotor thrust, $T$ .

Application of the Conservation Principles

At the plane of the rotor, assume that the velocity there, which is called the induced velocity, is $v_i$ . In the far slipstream, the velocity will be increased over that at the plane of the rotor, and this velocity is denoted by ${w}$ . The mass flow rate, $\overbigdot{m}$ , must be constant within the boundaries of the rotor wake, i.e., inside the control volume. The only cross-section of the wake boundary that is uniquely defined is at the rotor disk, so based on the assumed flow characteristics, then

(3) $\begin{equation*} \overbigdot{m} = \varrho \, A_\infty \, w = \varrho \, A_2 \, v_i = \varrho \, A \, v_i \end{equation*}$

The principle of conservation of momentum gives the relationship between the rotor thrust, $T$ , and the net time rate-of-change of momentum of the air out of the control volume (Newton’s second law). The rotor thrust is equal and opposite to the force on the air. For an unconstrained flow, the net pressure force on the air inside the control volume is zero, so the effects of external pressure can be neglected. Therefore, the rotor thrust can be written as

(4) $\begin{equation*} T = \overbigdot{m} w \end{equation*}$

From the principle of energy conservation, the work done on the rotor is equal to the gain in energy of the air per unit time. The work done per unit time, or the power consumed by the rotor, is $Tv_i$ , so that

(5) $\begin{equation*} T v_i = \dfrac{1}{2} \overbigdot{m} w^2 \end{equation*}$

From Eqs. 4 and 5 then it is apparent

(6) $\begin{equation*} v_i = \dfrac{1}{2} w \quad \mbox{or~that} \quad w = 2 v_i \end{equation*}$

This latter result, therefore, gives a simple relationship between the induced velocity in the rotor plane, $v_i$ , and the velocity ${w}$ in the slipstream. Notice also that, based on ideal flow assumptions, the slipstream comprises an area that is precisely half of the rotor disk area.

Induced Velocity

The rotor thrust is related to the induced velocity at the rotor disk using

(7) $\begin{equation*} T = \overbigdot{m} w = \overbigdot{m} (2 v_i) = 2 (\varrho \, A \, v_i) v_i = 2 \varrho \, A v_i^2 \end{equation*}$

Rearranging Eq. 7 to solve for the induced velocity gives

(8) $\begin{equation*} v_i = \sqrt{ \frac{T}{2 \varrho \, A} } = \sqrt{ \left( \frac{T}{A}\right) \dfrac{1}{2\varrho} } \equiv v_h \end{equation*}$

Notice that $v_h (\equiv v_i)$ is used for the induced velocity in hover because it becomes a reference when the axial climb, descent, and forward flight conditions are considered. It is significant here that the ratio ${T/A}$ appears in Eq. ??, known as the disk loading, and it is a critical parameter in rotor analysis.

Power Required to Hover

The power required to hover, which is the time rate of work done by the rotor on the air, is given by

(9) $\begin{equation*} P = T \, v_i \equiv T \, v_h = T \sqrt{\frac{T}{2\varrho \, A}} = \frac{T^{3/2}}{\sqrt{2\varrho \, A} } \end{equation*}$

This power value, called ideal power, is entirely induced in nature because the contribution of viscous effects has yet to be considered. In other words, this power value is the absolute lowest and, hence, the “ideal” amount required to generate a given rotor thrust.

Because $w = 2 v_i$ , it can also be written that

(10) $\begin{equation*} P = T v_h = 2 \overbigdot{m} \, v_h^2 = 2 (\varrho \, A \, v_h) v_h^2 = 2 \varrho \, A \, v_h^3 \end{equation*}$

This latter equation shows that the power required to hover will increase with the cube of the induced velocity $v_h$ at the disk. Therefore, to make a rotor hover generating a given amount of thrust with the minimum power required, the induced velocity $v_h$ at the disk must be as low as possible. If $v_h$ is too low, then the rotor will generate no thrust because, for a given mass flow rate, then

(11) $\begin{equation*} { T = 2 \overbigdot{m} \, v_h } \end{equation*}$

Therefore, to minimize the power required to produce thrust, the goal is that $v_h$ must be as low as possible, but the mass flow rate $\overbigdot{m}$ through the disk must be as large as possible. This goal, consequently, requires a large rotor disk area to entrain the needed mass flow; large-diameter rotors are a fundamental design feature of all helicopters.

Pressure Variations

The pressure variation through the rotor flow field in the hover state can be found from the application of Bernoulli’s equation above and below the rotor disk. However, remember that there is a pressure jump across the disk because of energy added by the rotor, so Bernoulli’s equation cannot be applied across the disk.

Referring to the previous figure and applying Bernoulli’s equation up to the rotor disk between stations 0 and 1 produces

(12) $\begin{equation*} p_0 = p_{\infty} = p_1 + \dfrac{1}{2} \varrho \, v_i^2 \end{equation*}$

and below the disk, between stations 2 and $\infty$ , then

(13) $\begin{equation*} p_2 + \dfrac{1}{2} \varrho \, v_i^2 = p_\infty + \dfrac{1}{2} \varrho \, w^2 \end{equation*}$

Because the jump in pressure $\Delta p$ is assumed to be uniform across the disk, this pressure jump must be equal to the disk loading, ${T/A}$ , that is

(14) $\begin{equation*} \Delta p = p_2 - p_1 = \frac{T}{A} \end{equation*}$

Therefore, it can be written that

(15) $\begin{equation*} \frac{T}{A} = p_2 - p_1 = \left( p_\infty +\dfrac{1}{2} \varrho \, w^2 - \dfrac{1}{2} \varrho \, v_i^2 \right) - \left( p_\infty - \dfrac{1}{2} \varrho \, v_i^2 \right) = \dfrac{1}{2} \varrho \, w^2 \end{equation*}$

From this, it can be seen that the rotor disk loading (which is a pressure) is equal to the dynamic pressure in the slipstream.

The pressure just above and below the disk can also be obtained in terms of disk loading. Just above the disk, the use of Bernoulli’s equation gives

(16) $\begin{equation*} p_1 = p_\infty - \dfrac{1}{2} \varrho \, v_i^2 = p_\infty - \dfrac{1}{2} \varrho \left( \frac{w}{2} \right) ^2 = p_\infty - \dfrac{1}{4} \left( \frac{T}{A} \right) \end{equation*}$

and just below the disk, then

(17) $\begin{equation*} p_2 = p_0 + \dfrac{1}{2} \varrho \, w^2 - \dfrac{1}{2} \varrho \left(\frac{w}{2}\right)^2 = p_0 + \frac{3}{4} \left( \frac{T}{A} \right) \end{equation*}$

Therefore, the conclusion to be drawn is that the static pressure is reduced by $\dfrac{1}{4}(T/A)$ above the rotor disk and increased by $\dfrac{3}{4}(T/A)$ below the disk, i.e., the net pressure jump is $\dfrac{T}{A}$ .

Check Your Understanding #1 – Distribution of thrust over the rotor disk

The simple momentum theory assumes that the jump in pressure across the actuator disk of a hovering rotor is constant and uniform. By considering an elemental annulus of the rotor disk, prove that this result must be consistent with a distribution of lift (or thrust force grading) across the rotor disk that varies linearly from a value of zero at the center (rotational axis) of the rotor to a maximum value around the edges of the rotor disk.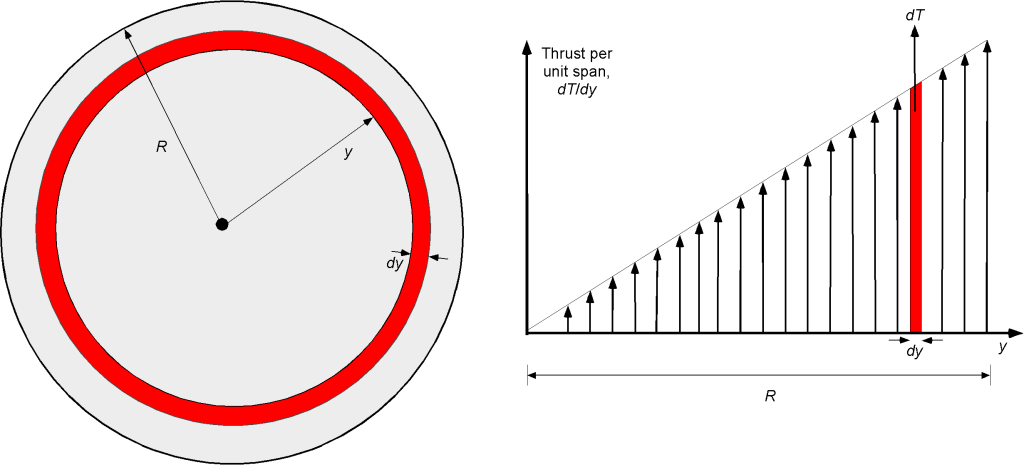

Show solution/hide solution.

The area of the elemental annulus is

$dA = 2\pi y dy$

The rotor disk loading (a pressure) is

$\frac{T}{A} = \Delta p = \mbox{constant}$

where $\Delta p$ is the pressure jump over the disk. Therefore, the thrust $dT$ on the elemental annulus is

$dT = \left( \Delta p \right)\, dA = \left( \Delta p \right) 2\pi y dy = 2\pi (\Delta p) \, y dy$

Finally, the thrust per unit span or thrust distribution is

$\frac{dT}{dy} = 2\pi (\Delta p) \, y$

which is a linear distribution over the radial dimension of the rotor disk from the rotational axis to the disk’s edge.

Disk Loading & Power Loading

A parameter frequently used in helicopter analysis that appears in the preceding equations is the disk loading, ${T/A}$ , denoted by $DL$ . Because for a single-rotor helicopter in a hover, the rotor thrust, $T$ , is equal to the helicopter’s weight, $W$ , the disk loading is sometimes written as $W/A$ or $W/\pi R^2$ . To compute the disk loading for multi-rotor helicopters such as tandems and coaxials or tiltrotors, a first assumption is that each rotor carries an equal proportion of the vehicle’s weight.

Disk loading is measured in pounds per square foot (lb ft $^{-2}$ ) in USC or Newtons per square meter (N m $^{-2}$ ) in SI. In the SI system, the disk loading may also be quoted in kilograms per square meter (kg m $^{-2}$ ). However, be aware that the direct use of the kilogram (kg) as a surrogate for a unit of force is strictly incorrect.

The power loading is defined as $T/P$ , denoted by $PL$ . Power loading is measured in pounds per horsepower (lb hp $^{-1}$ ) in USC or Newtons per kilowatt (N kW $^{-1}$ ) or kilograms per kilowatt (kg kW $^{-1}$ ) in the SI system. Remember that the induced (ideal) power required to hover is given by $P = T v_h$ . The ideal power loading is inversely proportional to the induced velocity at the disk, i.e.,

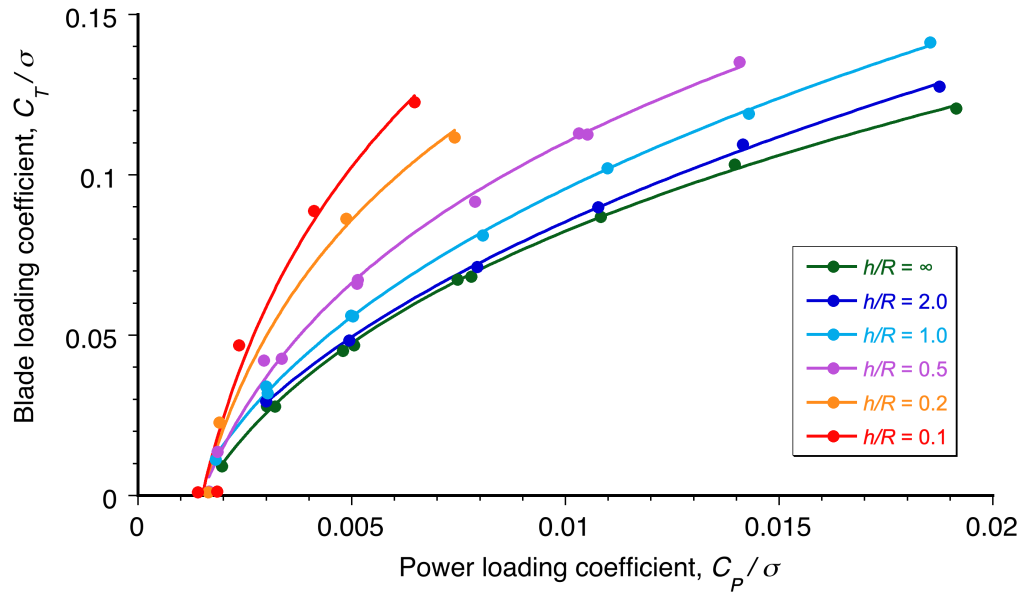
(18) $\begin{equation*} v_h = \sqrt{ \frac{T}{2\varrho A}} = \sqrt{ \frac{DL}{2 \varrho}} = \frac{P}{T} = PL^{-1} \end{equation*}$

According to the results in the figure below, the power loading, $PL = T/P$ , decreases quickly with increasing disk loading, $DL (= T/A)$ ; notice the logarithmic scales. Therefore, vertical lift aircraft with low effective disk loading will have relatively low power requirements per unit of thrust produced, i.e., they will have a high power loading. This outcome means they will tend to be more efficient, i.e., the rotor will require less power and consume less fuel to generate a given amount of thrust.

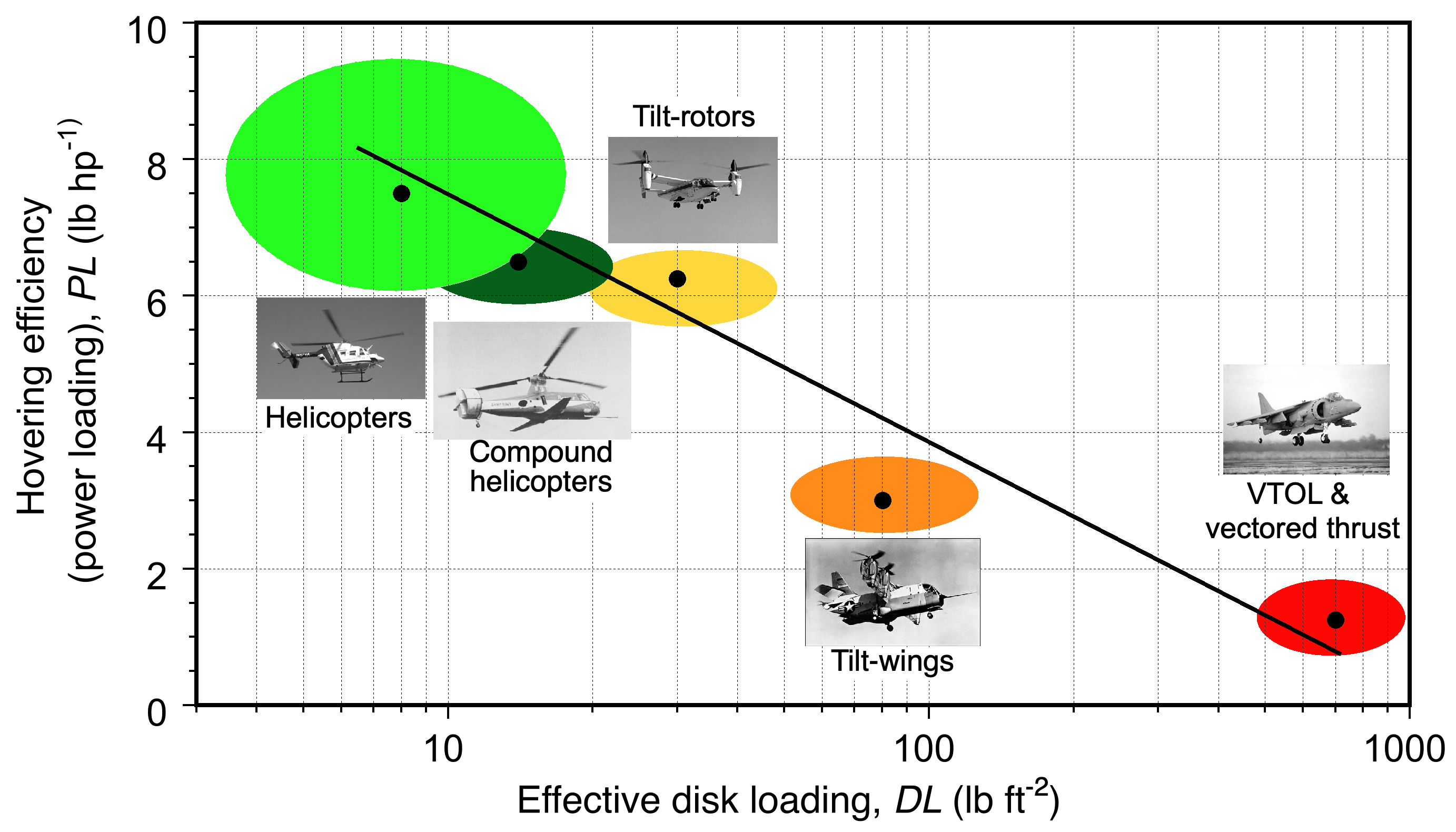
Hovering efficiency versus disk loading for a range of vertical lift aircraft. Traditional helicopters have the best efficiency compared to other VTOL aircraft.

Helicopters operate with low disk loadings in the region of 5 to 10 lb ft $^{-2}$ or 24 to 48 kg m $^{-2}$ , so they can provide a large amount of lift for relatively low power with power loadings of up to about 5 kg kW $^{-1}$ (50 N kW $^{-1}$ or 10 lb hp $^{-1}$ ). The above figure shows that the helicopter is a very efficient aircraft in hovering flight compared to other VTOL aircraft. Tiltrotors, because of their compromised design, have higher rotor disk loadings, so they are less efficient than a helicopter of the same in-flight weight. Jet thrust concepts have very high effective disk loadings because of their high jet velocities and low exit areas.

Non-Dimensional Hovering Analysis

As for airfoils and wings, non-dimensional parameters are used in rotor analysis to generalize aerodynamic performance. The non-dimensional value of the inflow, $\lambda_i$ , called the induced inflow ratio, is written as

(19) $\begin{equation*} \lambda_i = \frac{v_i}{\Omega R} \end{equation*}$

and in the hover case

(20) $\begin{equation*} \lambda_h = \frac{v_h}{\Omega R} \end{equation*}$

Recall that the angular or rotational speed of the rotor is denoted by $\Omega$ , and $R$ is the rotor radius; the product is the tip speed, i.e., $V_{\rm{tip}} = \Omega R$ .

For helicopter rotors, it is the convention to non-dimensionalize all velocities by the blade tip speed in hovering flight $V_{\rm{tip}}$ , and the reference area is the rotor disk area, $A$ . The rotor thrust coefficient is defined as

(21) $\begin{equation*} C_T = \frac{T}{\varrho \, A \, V_{\rm tip}^2} = \frac{T}{\varrho \, A \, \Omega^2 \, R^2} \end{equation*}$

Now it can be seen that the hover value of the inflow ratio, $\lambda_h$ , is related to the thrust coefficient by

(22) $\begin{equation*} \lambda_h = \frac{v_i}{\Omega R} = \dfrac{1}{\Omega R} \sqrt{\frac{T}{2 \varrho \, A}} = \sqrt{ \frac{T}{2 \varrho \, A (\Omega R)^2}} = \sqrt{\frac{C_T}{2}} \end{equation*}$

The corresponding rotor power coefficient is defined as

(23) $\begin{equation*} C_P = \frac{P}{\varrho \, A \, V_{\Omega R}^3} = \frac{P}{\varrho \, A \, \Omega^3 R^3} \end{equation*}$

Therefore, based on the momentum theory, the power coefficient for the hovering rotor becomes

(24) $\begin{equation*} C_P = \frac{T v_i}{\varrho \, A (\Omega R)^3} = \left( \frac{T}{\varrho \, A (\Omega R)^2} \right) \left( \frac{v_i}{\Omega R} \right) = C_T \lambda_i = \frac{C_T^{3/2}}{\sqrt{2}} \end{equation*}$

Again, this result is calculated based on uniform inflow over the rotor disk and no viscous losses, which is called the ideal power.

The corresponding rotor shaft torque coefficient is defined as

(25) $\begin{equation*} C_Q = \frac{Q}{\varrho A V_{\Omega R}^2 R} = \frac{Q}{\varrho A \Omega^2 R^3} \end{equation*}$

Notice that because power $P$ is related to torque $Q$ by $P=\Omega Q$ , then numerically, $C_P$ has the same value as $C_Q$ , although it would be incorrect to write that $C_P = C_Q$ .

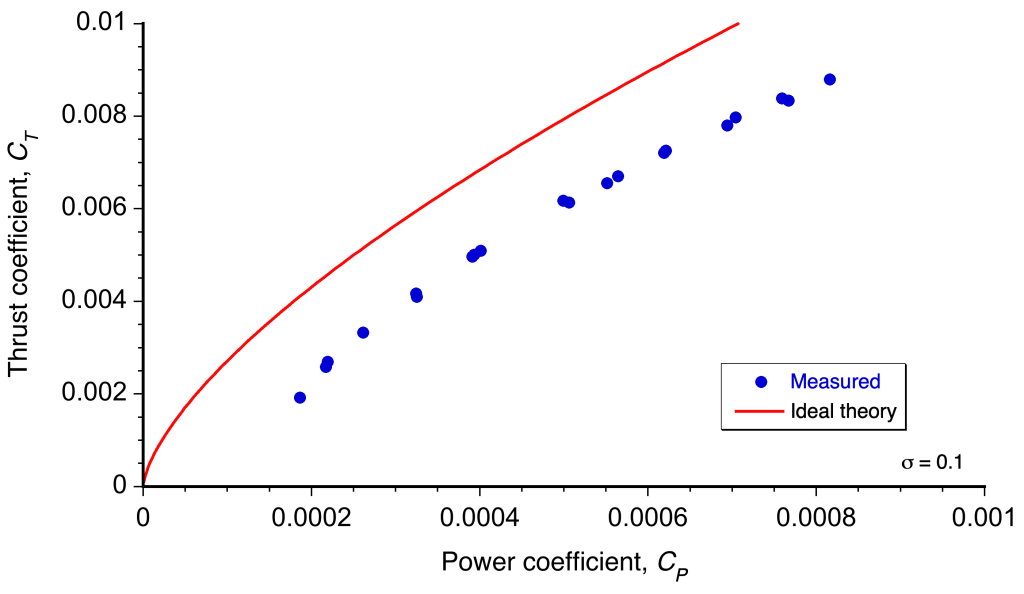
Measured Rotor Performance

In terms of coefficients, the ideal power to hover according to the simple momentum theory can be written as

(26) $\begin{equation*} C_P = \frac{C_T^{3/2}}{\sqrt{2}} \end{equation*}$

The figure below compares Eq. 26 with thrust and power coefficient measurements made for a hovering rotor. The form of presentation is called a power polar and is analogous to the drag polar used for airplane wings. The momentum theory underpredicts the required power, but the predicted trend that $C_P \propto C_T^{3/2}$ is correct. These differences between the momentum theory and experiments occur because viscous effects (i.e., non-ideal effects) have not been included in the basic theory. However, this deficiency can be rectified using empirical corrections to the theory.

Power polar for a hovering rotor. The simple momentum theory, absent of effects originating in viscosity, substantially underpredicts the power required.

Non-Ideal Effects

Non-ideal but physical effects that are not included in the basic momentum theory include things such as non-uniform inflow, tip losses, wake swirl, less than ideal wake contraction, finite number of blades, and so on. One of the most significant contributors to non-ideal effects is “tip loss,” which reflects that a lifting surface cannot create a finite lift at its tips, so the lift on the blade decreases rapidly as the tip is approached. Generally, non-ideal effects can be split into lifting (induced) and non-lifting contributions.

Induced Effects

In the ideal rotor theory, then $\kappa = 1$ . For an actual rotor, $\kappa$ can be derived from rotor measurements or flight tests. For preliminary design, most helicopter manufacturers use their own measurements and experience to estimate values of $\kappa$ . A typical average value is about 1.15. Values of $\kappa$ can also be computed directly using more advanced blade element methods, where the effects of the actual flight condition can be more accurately represented. This issue is significant for high-speed forward flight, where the increasing nonuniformity of the inflow from the reverse flow on the retreating blade must be accounted for.

Profile Drag Effects

Estimates of the profile power consumed by a rotor require knowledge of the drag coefficients of the airfoils that make up the rotor blades. Therefore, the drag coefficient will be a function of the Reynolds number and Mach number, varying along the blade’s span. However, a simple baseline result for the profile power can be obtained from an element-by-element summation of the sectional drag forces. i.e., the blade element method, the idea being shown in the figure below.

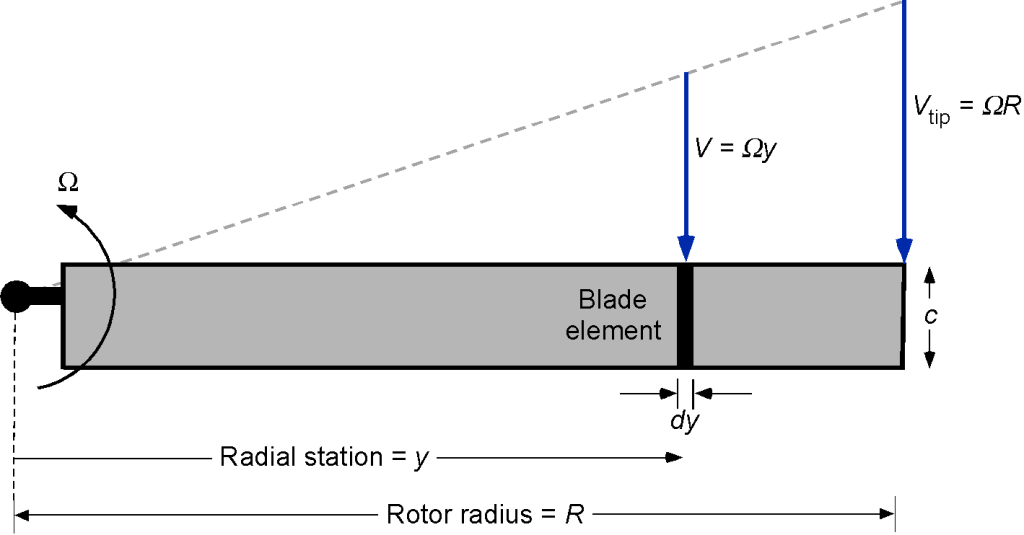
The principle of a blade element analysis of the rotor.

The power required to spin the blade in the absence of thrust (i.e., the profile power, ${P_0}$ ) can be obtained by radially integrating the sectional drag force along the length of the blade using

(27) $\begin{equation*} P_0 = \Omega N_b \int_0^R D y dy \end{equation*}$

where $N_b$ is the number of blades, and $D$ is the drag force per unit span at a section on the blade at a distance ${y}$ from the rotational axis. The drag force on each blade element can be expressed conventionally as

(28) $\begin{equation*} D = \dfrac{1}{2} \varrho \, V^2 c C_{d} = \dfrac{1}{2} \varrho (\Omega y)^2 c C_{d} \end{equation*}$

where ${c}$ is the blade chord, which is assumed constant in this case, i.e., the blade has a rectangular planform.

If the section profile drag coefficient, $C_{d}$ , is also assumed to be constant, i.e., $C_d = C_{d_{0}}$ , then the profile power integrates to

(29) $\begin{equation*} P_0 = \frac{1}{8} \varrho \, N_b \Omega^3 c C_{d_{0}} R^4 \end{equation*}$

Converting this result to a power coefficient by dividing through by $\varrho \, A (\Omega R)^3$ gives

(30) $\begin{equation*} C_{P_{0}} = \frac{1}{8} \left( \frac{N_b \, c \, R}{A} \right) C_{d_{0}} = \frac{1}{8} \left( \frac{N_b \, c \, R}{\pi R^2} \right) C_{d_{0}} = \frac{1}{8} \left( \frac{N_b \, c}{\pi R} \right) C_{d_{0}} = \frac{1}{8} \sigma \, C_{d_{0}} \end{equation*}$

The grouping

(31) $\begin{equation*} \frac{N_b \, c \, R}{A} = \frac{N_b \, c \, R}{\pi R^2 } = \frac{N_b \, c}{\pi \, R } = \sigma \end{equation*}$

is known as the rotor solidity. Typical values of $\sigma$ for a helicopter rotor range between 0.05 and 0.12.

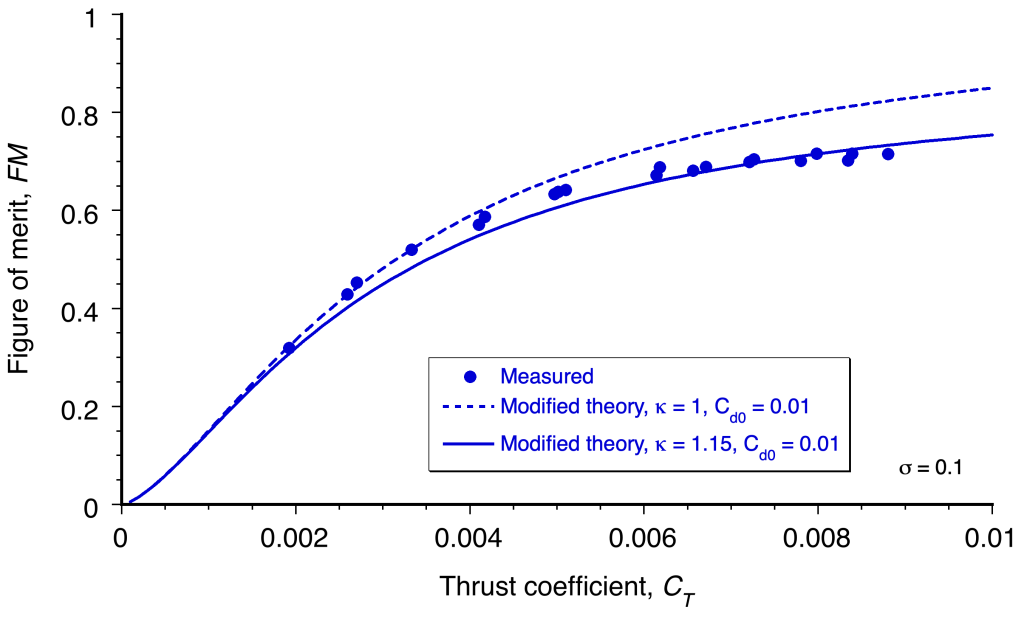
Modified Theory Versus Measurements

It is now possible to recalculate the rotor power requirements by using the modified momentum theory such that

(32) $\begin{equation*} C_P = \frac{\kappa C_T^{3/2}}{\sqrt{2}} + \frac{\sigma \, C_{d_{0}}}{8} \end{equation*}$

This result is shown in the figure below and calculated by assuming $\sigma = 0.1$ , $\kappa = 1.15$ , and $C_{d_{0}}=0.01$ .

Power polar for a hovering rotor using the modified momentum theory, which substantially improves predictions of the power required.

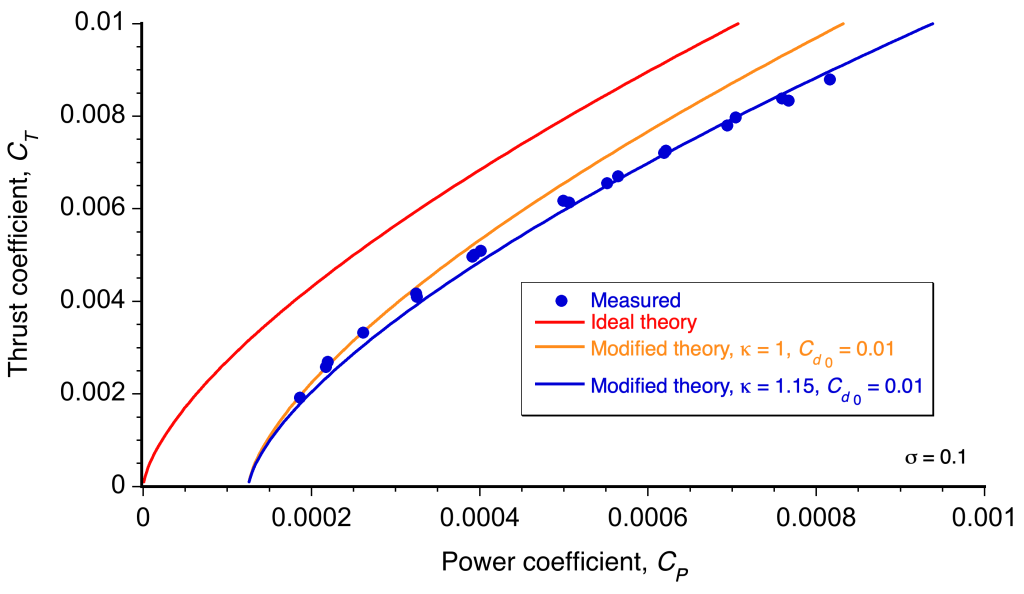
In the first case, to show the effect of adding profile power losses, it has been assumed that $\kappa=1.0$ (ideal induced losses), and in the second case, $\kappa=1.15$ (non-ideal losses). Notice the need to account for non-ideal induced losses and profile losses to give agreement with the measured data. The overall level of correlation thus obtained gives considerable confidence in the modified momentum theory approach for basic rotor performance studies, at least in hover.

Figure of Merit

Determining an efficiency factor for a helicopter rotor is difficult. Many parameters are involved, such as disk area, solidity, blade aspect ratio, airfoil characteristics, and tip speed. As discussed previously, the power loading, $PL$ , is an absolute measure of rotor efficiency because a helicopter of a given weight should be designed to hover with the minimum power requirements, i.e., the ratio $T/P$ should be made as large as possible.

However, the power loading is dimensional, so a relative non-dimensional measure of hovering thrust efficiency called the figure of merit is used. This quantity is calculated using the simple momentum theory as a reference and is defined as the ratio of the ideal power required to hover to the actual power required, i.e.,

(33) $\begin{equation*} FM = \frac{\mbox{Ideal power required to hover}}{\mbox{Actual power required to hover}} < 1 \end{equation*}$

The simple momentum gives the ideal power result in Eq. 24. Therefore, for an actual rotor, the figure of merit will always be less than unity.

Using the modified form of the momentum theory with the non-ideal approximation for the power, the figure of merit can be written as

(34) $\begin{eqnarray*} FM =\frac{\mbox{Ideal power}}{\mbox{Induced power $+$ profile power}} = \frac{P_{\rm ideal}}{\kappa P_{\rm ideal} + P_0} = \frac{ \displaystyle{ \frac{C_T^{3/2}}{\sqrt{2}}}}{\displaystyle{ \frac{\kappa C_T^{3/2}}{\sqrt{2}}} + \displaystyle{ \frac{\sigma C_{d_{0}}}{8}} } \end{eqnarray*}$

A representative plot of the measured figure of merit versus rotor thrust is shown below. It will be apparent that the $FM$ reaches a maximum and then remains constant or drops off slightly. This latter behavior is because of the higher profile drag coefficients ( $> C_{d_{0}}$ ) obtained at higher rotor thrusts. For some rotors, especially those with less efficient airfoils, the curve can exhibit a peak in $FM$ , followed by either a progressive or abrupt decrease after that. Therefore, the $FM$ behavior in the high thrust range will, to some extent, be a function of the airfoils used on the blades and their stall type. In practice, maximum $FM$ values between 0.65 and 0.75 represent a good hovering performance for a helicopter rotor.

Figure of merit predictions made with modified momentum theory compared to measured results for a hovering rotor.

The figure of merit for the best hovering efficiency can now be established, i.e., maximum power loading. The ratio of the power required to hover to the thrust produced is

(35) $\begin{equation*} \frac{P}{T} = \frac{P}{W}= \frac{(\Omega R) C_P}{C_T} \end{equation*}$

which can be written in terms of the modified momentum theory with the parameters $\kappa$ and $C_{d_{0}}$ as

(36) $\begin{equation*} \frac{P}{T} = \Omega R \frac{C_P}{C_T} = \Omega R \left( \kappa \sqrt{\frac{C_T}{2}} + \frac{C_{P_{0}}}{C_T} \right) = \frac{\Omega R}{C_T} \left( \kappa \frac{C_T^{3/2}}{\sqrt{2}} + \frac{\sigma C_{d_{0}}}{8} \right) \end{equation*}$

The operating $C_T$ to give the best power loading can be obtained by differentiating Eq. 36 with respect to $C_T$ , i.e.,

(37) $\begin{eqnarray*} \frac{d(P/T)}{d C_T} & = & -\Omega R C_T^{-2} \left( \frac{ \kappa C_T^{3/2}}{\sqrt{2}} + \frac{\sigma C_{d_{0}}}{8} \right) + \Omega R C_T^{-1} \left( \frac{3 \kappa C_T^{1/2}}{2 \sqrt{2}} \right) \nonumber \\ & = & -\frac{\Omega R}{C_T^{2} }\left( \frac{ \kappa C_T^{3/2}}{\sqrt{2}} + \frac{\sigma C_{d_{0}}}{8} \right) + \Omega R C_T^{-1/2} \left( \frac{3 \kappa}{2 \sqrt{2}} \right) \end{eqnarray*}$

which must be zero for a minimum. Therefore,

(38) $\begin{equation*} \frac{3 \kappa}{2 \sqrt{2}} = \left( \frac{\kappa}{\sqrt{2}} + \frac{\sigma C_{d_{0}} C_T^{-3/2}}{8} \right) \end{equation*}$

which, on rearrangement, gives

(39) $\begin{equation*} C_T = \left( \frac{\sqrt{2}}{4}\right)^{2/3} \left( \frac{ \sigma C_{d_{0}}}{\kappa} \right)^{2/3} = \frac{1}{2} \left( \frac{ \sigma C_{d_{0}}}{\kappa} \right)^{2/3} \end{equation*}$

Substituting the result that $C_T = \dfrac{1}{2} ( \sigma C_{d_{0}}/\kappa )^{2/3}$ into the figure of merit expression gives

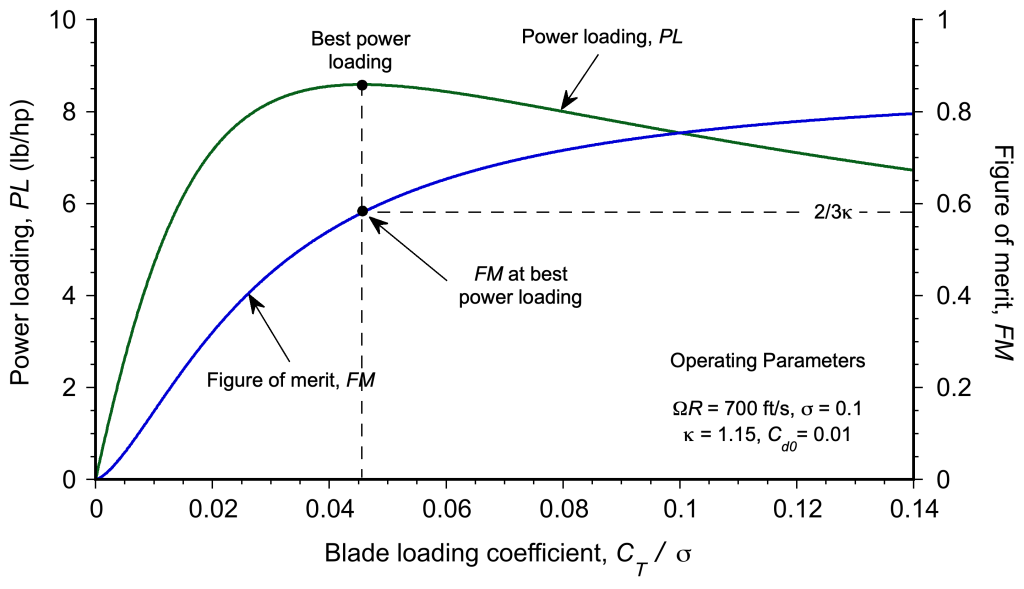
(40) $\begin{equation*} FM = \frac{ \displaystyle{ \dfrac{1}{2 \sqrt{2}} } \left( \displaystyle{ \frac{\sigma C_{d_{0}}}{\kappa}} \right) }{ \displaystyle{ \frac{\kappa}{2 \sqrt{2}} } \left( \displaystyle{ \frac{\sigma C_{d_{0}}}{\kappa}} \right) + \displaystyle{ \frac{ \sqrt{2}}{8} \sigma C_{d_{0}} }} = \frac{\kappa^{-1}}{1 + \dfrac{1}{2}} = \frac{2}{3 \kappa} \end{equation*}$

For an ideal rotor, the best power loading is obtained at a figure of merit of 2/3. Using the modified momentum theory, this condition occurs at a figure of merit of 2/3 $\kappa$ .

For design purposes, solving for the rotor radius would determine its optimum value for a given gross weight of the helicopter, the rotor tip speed, and the operational density altitude. However, in most cases, the resulting radius is too high to be practical, i.e., the rotor will be so oversized that it would be excessively heavy. Furthermore, the helicopter would not be able to fit into a hangar or otherwise be accommodated at an airfield. Therefore, the rotor must be operated at a higher disk loading than the optimum.

As shown in the figure below, the rotor’s efficiency is relatively insensitive to thrust at its most efficient operation, as the $C_T/C_P$ curve is reasonably flat above a particular thrust coefficient. Therefore, there is some latitude when selecting the rotor radius, which may be constrained by factors other than aerodynamics.

Finally, a word of caution about the figure of merit is appropriate. To be meaningful, the figure of merit must only be used as a gauge of rotor efficiency when two or more rotors are compared at the same disk loading, which can be seen if the figure of merit is written dimensionally as

(41) $\begin{equation*} FM = \frac{P_{\rm ideal}}{\kappa P_{\rm ideal} + P_0} = \frac{1}{\kappa + \displaystyle{ \frac{P_0}{P_{\rm ideal}} } } = \frac{1}{\kappa + \displaystyle{\frac{\sqrt{2\varrho}}{T} \frac{P_0}{\sqrt{DL}}}} \end{equation*}$

Therefore, it would be inappropriate to compare the figures of merit of two rotors with different disk loadings because, with all other factors being equal, the rotor with the higher disk loading will generally always give the higher figure of merit.

Solidity & Blade Loading Coefficient

It will be seen from Eq. 34 that the solidity, $\sigma$ , appears in the expression for the figure of merit, $FM$ . For a rotor with rectangular blades, the solidity represents the ratio of the lifting area of the blades to the area of the rotor, i.e.,

(42) $\begin{equation*} \sigma = \frac{\mbox{blade~area}}{\mbox{disk~area}} = \frac{A_b}{A} = \frac{N_b c R}{\pi R^2} = \frac{N_b c}{\pi R} \end{equation*}$

As previously noted, typical values of $\sigma$ range from about 0.05 to 0.15 for helicopters.

If $FM$ is plotted for rotors with different values of $\sigma$ , the behavior is typified by the figure below. While the number of blades also affects rotor performance, there are no known measurements of solidity effects independently of blade number. Results predicted using the modified momentum theory are also shown. From the measurements at zero thrust, it was deduced that $C_{d_{0}} = 0.011$ and that $\kappa$ was about 1.25.

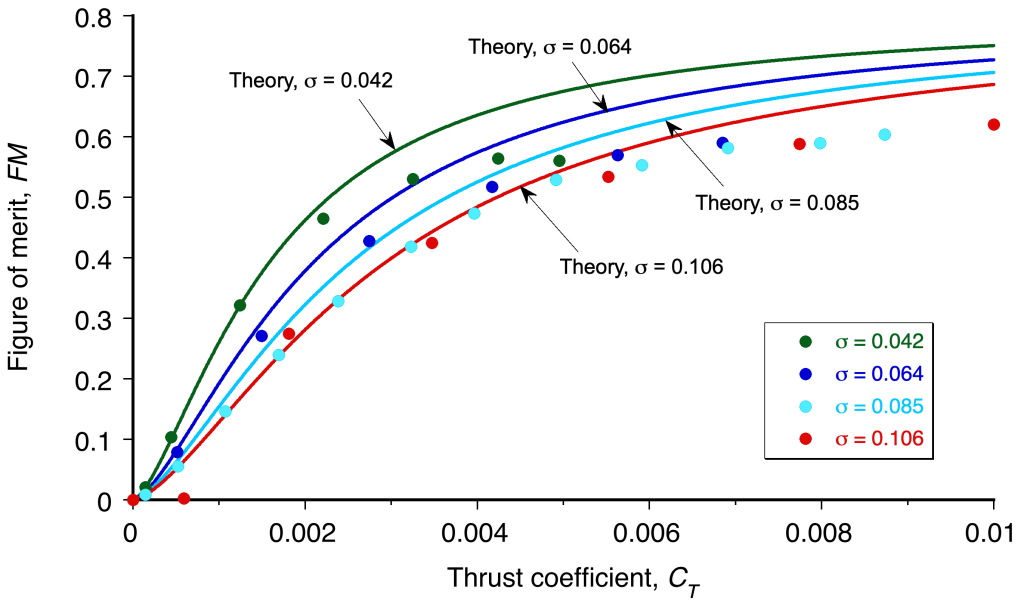
Measured and predicted values of the figure of merit versus the thrust coefficient for a hovering rotor with different values of solidity.

It will be noted that higher values of $FM$ are obtained with the lowest possible solidity at the same design $C_T$ , i.e., same aircraft gross weight or disk loading. This result is hardly unexpected from Eq. 34, all other terms such as $\kappa$ being assumed constant, meaning that the viscous drag on the rotor is minimized by reducing the net blade area. However, the minimization of $\sigma$ must be done with caution because reducing the blade area must always result in a higher angle of attack of the blade sections (and higher lift coefficients) to obtain the same values of $C_T$ .

Therefore, the lowest allowable value of $\sigma$ must ultimately be limited by the onset of blade stall. The results show this latter effect for the lowest solidity of 0.042, where a progressive departure occurs from the theoretical predictions for $C_T > 0.004$ . This behavior would occur at higher values of $C_T$ for a full-scale rotor because of the higher values of maximum lift found at the higher Reynolds numbers on the blades.

Therefore, an alternative presentation is to plot the figure of merit versus the blade-loading coefficient, $C_T/\sigma$ , as shown in the figure below. In this case, $C_T/\sigma$ can be written as

(43) $\begin{equation*} \frac{C_T}{\sigma} = \frac{T}{\varrho \, A (\Omega R^2)} \left( \frac{A}{A_{b}} \right) = \frac{T}{\varrho \, A_{b} (\Omega R)^2 } \end{equation*}$

where $A_{b}$ is the area of the blades.

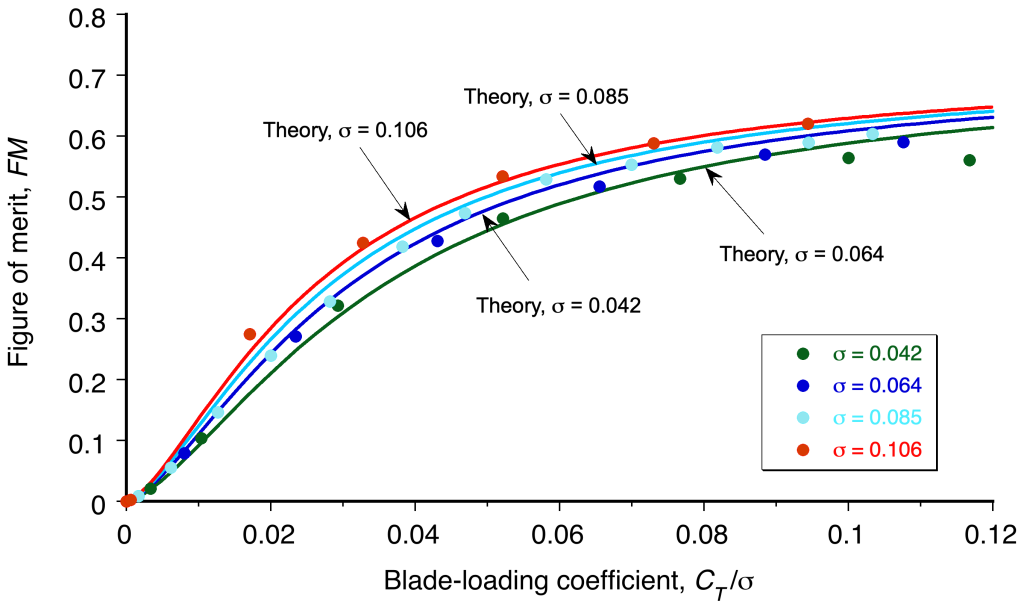
Measured and predicted figure of merit versus blade-loading coefficient for a hovering rotor with different solidities.

Notice that reducing the value of $\sigma$ results in higher values of $C_T/\sigma$ for the same value of $C_T$ . Although the rotor operates at higher values of $FM$ with an increased blade loading coefficient, the maximum value is limited by the onset of blade stall. Typically, for a contemporary helicopter rotor, the maximum realizable value of the blade loading coefficient without stall is about 0.12 to 0.14. However, the influence of Reynolds number on blade stall must also be considered, especially with subscale rotors.

The maximum attainable value of $C_T/\sigma$ will also depend on the distribution of local lift coefficients along the blade, which depends on both the blade twist and its planform shape. The local lift coefficients can be related to the blade loading using the blade element theory, so the blade twist and blade planform can be designed to delay the stall effects to higher values of $C_T/\sigma$ . A rotor that uses airfoils with higher values of the maximum lift coefficient can also be designed to have lower solidity. This approach has the benefit of a lower blade and hub weight, which significantly contribute to total helicopter weight.

Ground Effect

Just like airplanes, helicopter performance is affected by the presence of the ground or any other boundary that may alter or constrain the flow into the rotor or constrain the development of the wake, as shown in the figure below. Because the ground must be a streamline to the flow, the rotor slipstream tends to expand rapidly as it approaches the ground. This behavior alters the slipstream velocity, the induced velocity in the rotor plane, and, therefore, the rotor thrust and power. Similar effects are obtained in hover and forward flight, but the effects are most substantial in the hovering state.

The effects of the ground will distort the rotor wake and change the rotor performance. The effects are most substantial in hover and diminish as the helicopter flies forward.

A representative set of power polars for a rotor hovering near the ground is shown in the figure below. The results suggest significant effects on hovering performance for heights less than one rotor diameter. When the hovering rotor operates in ground effect, the rotor thrust is increased for a given power. Alternatively, this effect can be viewed as a reduction in power for a given thrust (weight). Remember that a straight line drawn from point (0,0) to any point on any polar curve gives the ratio of $C_T/C_P$ or the power loading, so it measures efficiency. Notice that the efficiency is the highest for the lowest rotor heights off the ground.

Power polars for rotors hovering in ground effect. The effects on rotor performance diminish quickly for rotor heights greater than one diameter above the ground.

Check Your Understanding #2 – Estimating power requirements for flight

In 1907, Paul Cornu built a primitive twin-rotor helicopter. Each rotor of his machine was approximately 19.7 ft in diameter. The machine had a net gross weight (with the pilot) of about 575 lb. Use momentum theory to verify the power requirements for flight, free of the ground and out of ground effect.

Show solution/hide solution.

Assuming each rotor lifted half of the total aircraft weight, then the momentum theory gives a result for the net minimum possible power (or ideal power) required to drive both rotors using

$P_{\rm ideal} = 2 \left( \frac{(W/2)^{3/2}}{\sqrt{2\varrho \, A}} \right)$

where the total take-off weight $W = 575$ lb and each rotor had a swept disk area, $A=304$ ft ${^{2}}$ . Assuming sea level air density, this gives the ideal shaft power (in horsepower) required to drive both rotors of Cornu’s machine as

$P_{\rm ideal} = \frac{2}{550} \left( \frac{(575/2)^{3/2}}{\sqrt{2 \times 0.002378 \times 304}} \right) = 14.7~\mbox{hp}$

Therefore, free flight would require an installed power of at least 14.7 hp, but only if the rotors were aerodynamically 100% efficient and there were no transmission losses. Realistically, with the primitive rotors used by Cornu, the aerodynamic efficiency of the rotors could be expected to be no better than 50% (a figure of merit of 0.5), giving a power requirement of about 30 hp.

Cornu also used an inefficient belt and pulley system to drive the rotors from an engine that produced only 24 hp. In his logbooks, Cornu constantly talks about the challenges of slipping belts. Therefore, considering the relative inefficiency of the rotors, the installed power required for flight would need to have been about 40 hp. Thus, the conclusion is that using an engine with a power output of only 24 hp, it is doubtful that Paul Cornu’s machine ever flew in sustained flight free of the ground.

Check Your Understanding #3 – Hovering power required

A tiltrotor has a gross weight of 45,000 lb (20,400 kg). The rotor diameter is 38 ft (11.58 m). Based on the momentum theory, estimate the power required for the aircraft to hover at sea level on a standard day out of ground effect where the density of air is 0.002378 slugs ft ${^{-3}}$ or 1.225 kg m ${^{-3}}$ . Assume that the figure of merit of the rotors is 0.75 and transmission losses amount to 5%.

Show solution/hide solution.

A tiltrotor has two rotors, each assumed to carry half of the total aircraft weight, that is, $T =$ 2,500 lb. Each rotor’s disk area is $A = \pi (38/2)^2 = 1134.12$ ft ${^{2}}$ . The induced velocity in the plane of the rotor is

$v_h = \sqrt{ \frac{T}{2 \varrho \, A} } = \sqrt{ \frac{22500}{2 \times 0.002378 \times 1134.12} } = 64.56~\mbox{ ft s$^{-1}$}$

The ideal power per rotor will be

$T v_h = 22,500 \times 64.56 = 1,452,600~\mbox{ft-lb s$^{-1}$}$

This result is converted into horsepower (hp) by dividing by 550 to give 2,641 hp per rotor. Remember that the figure of merit accounts for the aerodynamic efficiency of the rotors. Therefore, the actual power required per rotor to overcome induced and profile losses will be 2,641/0.75 = 3,521.5 hp, followed by multiplying the result by two to account for both rotors, that is, $2 \times 3,521.5 =$ 7,043 hp. Transmission losses account for another 5%, so the total power required to hover is $1.05 \times 7,043 =$ 7,395 hp.

The problem can also be worked on in SI units. In this case, $T = 10,200 \times 9.81 =$ 100,062 N. The disk area is, $A = \pi (11.58/2)^2 = 105.32$ m ${^{2}}$ . The induced velocity in the plane of the rotor is

$v_i = \sqrt{ \frac{T}{2 \varrho \, A} } = \sqrt{ \frac{100062}{2 \times 1.225 \times 105.35} } = 19.69~\mbox{ m s$^{-1}$}$

The ideal power per rotor will be

$T v_h = 100,062 \times 19.69 = 1,970.2~\mbox{kW}$

The actual power required per rotor to overcome induced and profile losses will be 1,970.2/0.75 = 2,626.9 kW, followed by multiplying the result by two to account for both rotors, 5,253.8 kW. Transmission losses mean the total power required to hover will be 5,515.7 kW.

Check Your Understanding #4 – Induced power factor & profile power

A student makes measurements of rotor performance at a fixed rotor speed for a series of blade pitch angles. The rotor has a solidity of 0.1. The values of the thrust coefficient, $C_T$ , that were measured were 6.0000E-06, 0.001049, 0.002375, 0.004075, and 0.005582, and the corresponding values of the power coefficient, $C_P$ , were 0.000196, 0.000225, 0.000281, 0.000404, and 0.000554, respectively. The student wants to estimate this rotor’s induced power factor, the zero thrust (profile) power, and the mean section drag coefficient.

Show solution/hide solution.

The simple momentum theory gives the ideal power as

$C_{P_{\rm ideal}} = \frac{C_T^{3/2}}{\sqrt{2}}$

and the modified momentum theory is

$C_{P_{\rm}} = \frac{\kappa \, C_T^{3/2}}{\sqrt{2}} + C_{P_{0}}$

The student wants to find values of $\kappa$ and $C_{P_{0}}$ so we can write

$C_{P_{\rm}} = \kappa \, C_{P_{\rm ideal}} + C_{P_{0}}$

and so to find these values we can plot $C_{P_{\rm}}$ versus $C_{P_{\rm ideal}}$ , which should be close to a straight-line.
The best straight-line fit (least-squares) gives the slope $\kappa$ , and the intercept on the ${y}$ -axis is $C_{P_{0}}$ . In this case the value of $\kappa$ is 1.206 and $C_{P_{0}}$ is 0.000192. It is then possible to estimate the average drag coefficient of the airfoils that comprise the rotor using

$C_{P_{0}} = \frac{\sigma C_{d_{0}}}{8}$

so

$C_{d_{0}} = \frac{8 C_{P_{0}}}{\sigma}$

If $\sigma = 0.1$ as stated, then $C_{d_{0}} = 0.0154$ , which seems fairly reasonable.

Axial Climbing & Descending Flight

Adequate climbing flight performance is an essential operational consideration for a helicopter, and sufficient power reserves must be available to ensure adequate climbing performance over a wide range of flight weights and operational density altitudes. Increasing altitude takes more power than losing altitude. Estimates of the power required to climb and descend can also be established from a momentum theory analysis.

Climbing Flight

The three conservation laws are applied to a control volume surrounding the climbing rotor and its flow field, as shown in the figure below. As before, consider the problem one-dimensional, and the flow properties will be assumed to vary only in the vertical direction over cross-sectional planes parallel to the disk. At each cross-section, the flow properties are distributed uniformly.

In contrast to the hover case where the climb velocity is identically zero, the relative velocity far upstream relative to the rotor will now be $V_c$ . At the plane of the rotor, the velocity will now be $V_c+v_i$ , and the slipstream velocity is now $V_c+w$ .

The mass flow rate $\overbigdot{m}$ is constant within the slipstream boundary and can be defined at the rotor, i.e.,

(44) $\begin{equation*} \overbigdot{m} = \varrho \, A_\infty (V_c + w) = \varrho \, A (V_c + v_i) . \end{equation*}$

The thrust on the rotor, in this case, will be

(45) $\begin{equation*} T = \overbigdot{m} (V_c + w) - \overbigdot{m} V_c = \overbigdot{m} w \end{equation*}$

Notice that this is the same equation for the rotor thrust as in the hover case, i.e., Eq. 4.

Because the work done by the climbing rotor is $T(V_c + v_i)$ , then

(46) $\begin{eqnarray*} T (V_c + v_i) = \frac{1}{2} \overbigdot{m} (V_c + w)^2 - \frac{1}{2} \overbigdot{m} V_c^2 = \frac{1}{2} \overbigdot{m} w (2V_c + w) \end{eqnarray*}$

From Eqs. 45 and 46 it is readily apparent that $w = 2 v_i$ .

The relationship between the rotor thrust and the induced velocity at the rotor disk in hover is

(47) $\begin{equation*} v_h \equiv v_i = \sqrt{ \frac{T}{2 \varrho \, A} } \end{equation*}$

and for the climbing rotor using Eq. 45, then

(48) $\begin{equation*} T = \overbigdot{m} w = \varrho \, A (V_c + v_i) w = 2 \varrho \, A (V_c + v_i) v_i \end{equation*}$

so that

(49) $\begin{equation*} \frac{T}{2\varrho \, A} = v_h^2 = (V_c + v_i) v_i = V_c v_i + v_i^2 \end{equation*}$

which is a quadratic equation in $v_i$ . Dividing through by $v_h^2$ to make it non-dimensional gives

(50) $\begin{equation*} \left( \frac{v_i}{v_h} \right)^2 + \frac{V_c}{v_h} \left( \frac{v_i}{v_h} \right) - 1 = 0 \end{equation*}$

which is a quadratic equation in $v_i/v_h$ . This equation has the solution

(51) $\begin{equation*} \frac{v_i}{v_h} = -\left(\frac{V_c}{2v_h} \right) \pm \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 +1 } \end{equation*}$

Although there are two possible solutions (positive and negative), $v_i/v_h$ must always be positive in the climb to avoid violating the assumed flow model. The valid solution is

(52) $\begin{equation*} { \frac{v_i}{v_h} = -\left(\frac{V_c}{2v_h} \right) + \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 +1 } } \end{equation*}$

Descending Flight

The climb flow model cannot be used in a descent (where $V_c \ll 0$ ) because now $V_c$ is directed upward so that the slipstream will be above the rotor. This will be the case whenever $|V_c|$ is more than twice the average induced velocity at the disk. For cases where the descent velocity is in the range $-2v_h \le V_c \le 0$ , the velocity at any plane through the rotor slipstream can be upward or downward. Under these circumstances, a definitive control volume surrounding the rotor and its wake cannot be established.

The assumed flow model and control volume surrounding the descending rotor are shown in the figure below. To proceed, the assumption must be made that $|V_c|> 2v_h$ so that a well-defined slipstream will always exist above the rotor and encompass the limits of the rotor disk. Far upstream (well below) the rotor, the magnitude of the velocity is the descent velocity, which is equal to $|V_c|$ . Notice that to avoid ambiguity, it will be assumed that the velocity is measured as positive when directed downward. At the plane of the rotor, the velocity is $|V_c|-v_i$ . In the far wake (above the rotor), the velocity is $|V_c|-w$ .

Flow model used for momentum theory analysis of a rotor in a vertical (axial) descent.

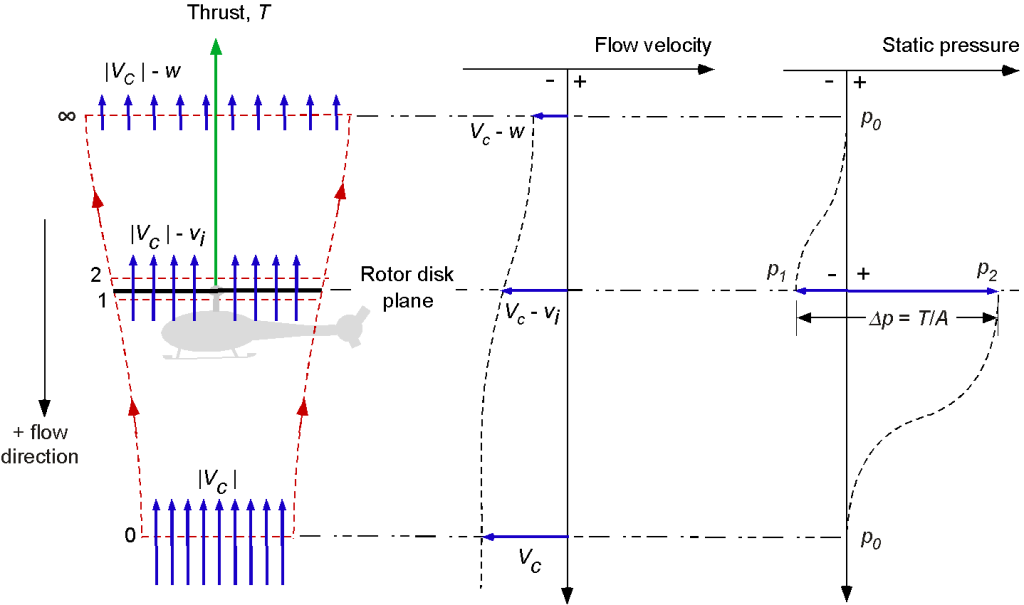
The mass flow rate, $\overbigdot{m}$ , through the rotor disk is

(53) $\begin{equation*} \overbigdot{m} = \varrho \, A_\infty (V_c + w) = \varrho \, A (V_c + v_i) \end{equation*}$

The thrust, in this case, can be expressed as

(54) $\begin{equation*} T = ( -\overbigdot{m}) (V_c + w) - (-\overbigdot{m}) V_c = -\overbigdot{m} w \end{equation*}$

Notice that $T$ is not negative because $\overbigdot{m}$ is negative using the assumed sign convention.

The work done by the rotor is

(55) $\begin{equation*} T (v_i+V_c) = \frac{1}{2} \overbigdot{m} V_c^2 - \frac{1}{2} \overbigdot{m} (V_c + w)^2 = -\frac{1}{2} \overbigdot{m} w (2V_c + w) \end{equation*}$

which is a negative quantity. Therefore, the rotor must extract power from the airstream, and this operating condition is known as the windmill state. It is usually referred to as the windmill brake state because the rotor in this condition decreases or “brakes” the velocity of the flow.

Using Eqs. 54 and 55 it is seen, again, that $w = 2 v_i$ . Note, however, that the net velocity in the slipstream is less than $|V_c|$ , so from continuity considerations, the wake boundary expands above the descending rotor disk. For the descending rotor, then

(56) $\begin{equation*} T = -\overbigdot{m} w = -\varrho \, A (V_c + v_i) w = -2 \varrho \, A (V_c + v_i) v_i \end{equation*}$

so that

(57) $\begin{equation*} \frac{T}{2\varrho \, A} = v_h^2 = -(V_c + v_i) v_i = - V_c v_i -v_i^2 \end{equation*}$

Dividing through by $v_h^2$ gives

(58) $\begin{equation*} { \left( \frac{v_i}{v_h} \right)^2 + \frac{V_c}{v_h} \left( \frac{v_i}{v_h} \right) + 1 = 0 } \end{equation*}$

which is a quadratic equation in $v_i/v_h$ . This equation has the solution

(59) $\begin{equation*} \frac{v_i}{v_h} = -\left(\frac{V_c}{2v_h} \right) \pm \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 -1 } \end{equation*}$

Again, like the climb case, two possible solutions exist for $v_i/v_h$ in descent. The only valid solution is

(60) $\begin{equation*} \frac{v_i}{v_h} = -\left(\frac{V_c}{2v_h} \right) - \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 -1 } \end{equation*}$

which is applicable for $V_c/v_h\le -2$ .

Induced Velocity Curves

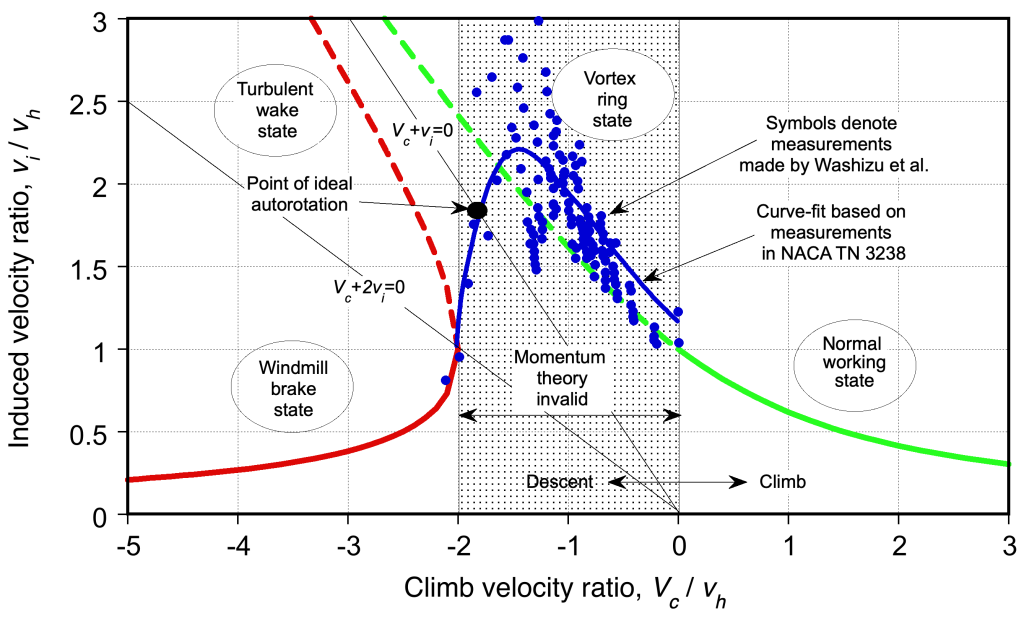
Results from the preceding analysis are shown below. It is apparent that as the climb velocity increases, the induced velocity at the rotor decreases. This condition is called the normal working state of the rotor, with hover being the lower limit. The branch of the induced velocity curve denoted by the broken line gives a solution to Eq. 52 for negative values of $V_c$ , i.e., a descent. However, as the rotor begins to descend, there can be two possible flow directions, one of which violates the assumed flow model, so this solution is physically invalid. This condition is called the Vortex Ring State (VRS) and can only be described based on measurements.

Induced velocity variation as a function of climb and descent velocity based on momentum theory (complete induced velocity curve).

Power Required Curves

Because both climb and descent change the induced velocity at the rotor, the induced power will also be affected. The power ratio can be written as

(61) $\begin{equation*} \frac{P}{P_h} = \frac{V_c + v_i}{v_h} = \frac{V_c}{v_h} + \frac{v_i}{v_h} \end{equation*}$

where the first term is the useful work to change the potential energy of the rotor (helicopter), and the second term is the work done on the air by the rotor, i.e., the irrecoverable induced losses.

Using Eq. 52 and substituting and rearranging gives the power ratio for a climb as

(62) $\begin{equation*} \frac{P}{P_h} = \frac{V_c}{2v_h} + \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 +1 } \mbox{~~which is applicable for~$\displaystyle{\frac{V_c}{v_h}} \ge 0$} \end{equation*}$

In a descent, Eq. 60 is applicable. Substituting this into Eq. 61 and rearranging gives the power ratio as

(63) $\begin{equation*} \frac{P}{P_h} = \frac{V_c}{2v_h} - \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 - 1 } \mbox{~~which is applicable for~$\displaystyle{\frac{V_c}{v_h}} \le -2$} \end{equation*}$

The figure below shows the total rotor power ratio, $P/P_h$ , plotted versus the climb ratio, $V_c/v_h$ . The power required to climb is always greater than that needed to hover. However, as the climb velocity increases, the induced power becomes a progressively smaller percentage of the total power required to climb. It is also significant to notice that in a descent, at least above a specific rate, the rotor extracts power from the air and uses less power than required to hover, i.e., the rotor now operates like a windmill. However, a helicopter rotor will never operate in this condition.

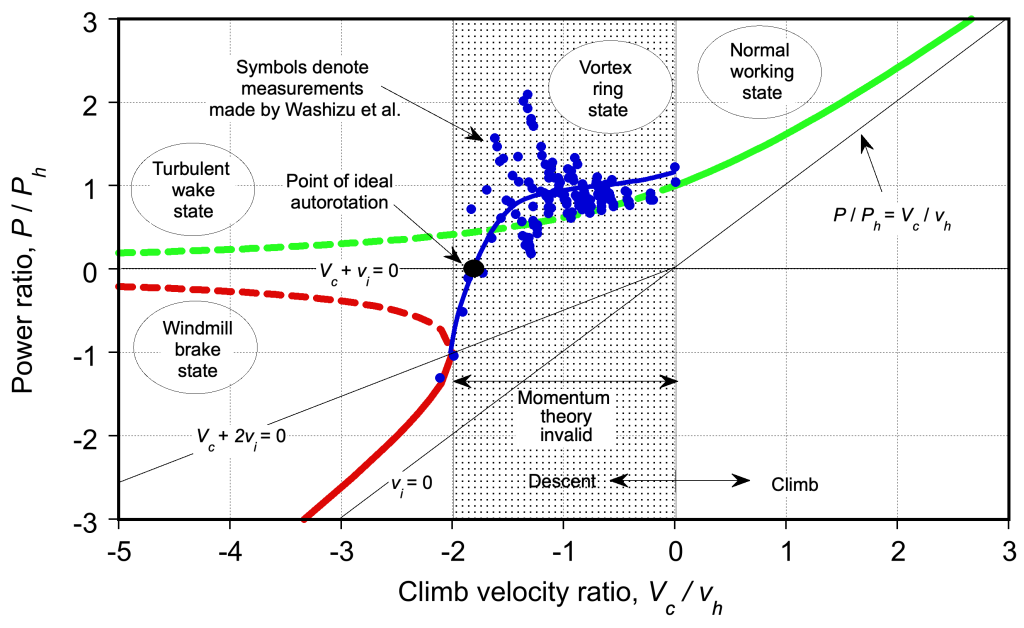
Total power required as a function of climb and descent velocity (universal power curve).

Vortex Ring State

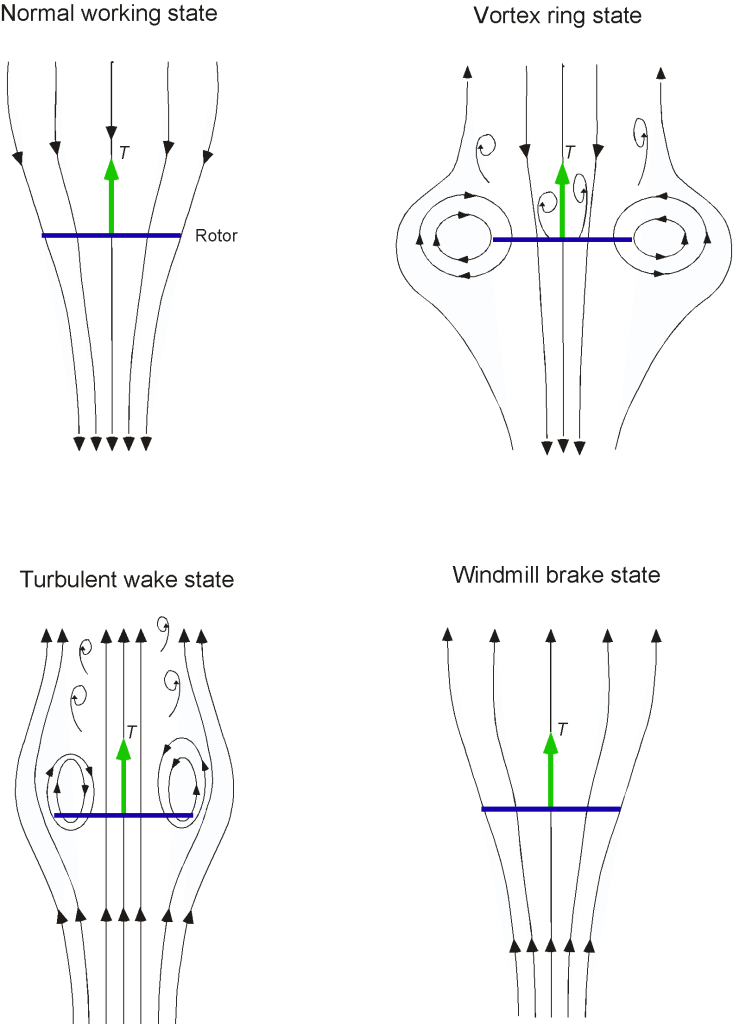
In the region $-2\le V_c/v_h \le 0$ , called the vortex ring state (VRS), the momentum theory is invalid because the flow can take on two possible directions, and a well-defined slipstream ceases to exist, as shown in the figure below. In the VRS, the rotor can experience highly unsteady flow with regions of concurrent upward and downward velocities, and the flow can periodically break away from the rotor disk. This means a control volume encompassing only the rotor disk’s physical limits cannot be defined. From a piloting perspective, VRS is not a sustainable flight condition. The unsteadiness of flow at the rotor in VRS can lead to a loss of rotor control. If the VRS occurs on the tail rotor, such as during a sideways flight or hovering in a crosswind, then directional (yaw) control may be seriously impaired.

The induced velocity curve in the VRS can still be defined empirically, albeit only approximately, based on experiments with rotors. Even then, measurements of rotor thrust and power are difficult to make. The average induced velocity is then obtained indirectly from the measured rotor power and thrust using the assumed form

(64) $\begin{equation*} P_{\rm meas} = T(V_c + \overline{v}_i) + P_0 \end{equation*}$

where ${P_0}$ is the profile power, and where $\overline{v}_i$ is recognized as only an averaged value of the induced velocity through the disk. Using the result that $P_h=T \overline{v}_h$ then

(65) $\begin{equation*} \frac{V_c + \overline{v}_i}{\overline{v}_h} = \frac{P_{\rm meas} - P_0}{P_h} = \frac{P_{\rm meas} - P_0}{T \sqrt{T/2\varrho \, A}} = \frac{(P_{\rm meas} - P_0)\sqrt{2\varrho \, A}}{W^{3/2}} \end{equation*}$

Therefore, in addition to the measured rotor power $P_{\rm meas}$ , it is necessary to know the rotor profile power to estimate the averaged induced velocity ratio. As shown previously using Eq. 30, a straightforward estimate for the profile power coefficient of a rotor with rectangular blades is $C_{P_{0}}= \sigma C_{d_{0}}/8$ . Because of the high levels of turbulence near the rotor in the VRS, the derived measurements of the average induced velocity contain a relatively large amount of scatter.

These measurements can then be used to find a “best-fit” approximation for $v_i$ at any rate of descent. One approximation is

(66) $\begin{equation*} \frac{v_i}{v_h} = \left\{\begin{array}{ll} 1 - \displaystyle{\frac{V_c}{v_h}} & \mbox{~~for~} -1.5 \le \displaystyle{\frac{V_c}{v_h}} \le 0 \\[20pt] 7 + 3 \displaystyle{\frac{V_c}{v_h}} & \mbox{~~for~} -2 \le\displaystyle{\frac{V_c}{v_h}} \le -1.5\end{array} \right. \end{equation*}$

Autorotation in Vertical Flight

The principle of autorotation can be seen in nature in the flight of sycamore or maple seeds, which spin rapidly as they slowly descend and are often carried on the wind for a considerable distance. An autorotation is a maneuver that can be used to recover the helicopter to the ground in the event of an engine failure, transmission problems, or loss of the tail rotor. It requires that the pilot let the helicopter descend at a sufficiently high but controlled rate, where the energy to drive the rotor can be obtained by giving up potential energy (altitude) for kinetic energy taken from the relative upward flow through the rotor, thereby averting a ballistic fall.

Notice that from the power curve shown previously, there is a value of $V_c/v_h$ for which zero net power is required for the rotor, i.e., $P = T(V_c + v_i) = 0$ or $P/P_h = 0$ . This condition is called ideal autorotation for vertical flight. It is a self-sustained operating state where the energy to drive the rotor comes from potential energy converted to kinetic energy from the relative descent velocity (upward relative to the rotor). Based on assuming the validity of Eq. 66, it will be apparent that the power curve crosses the ideal autorotation line $V_c + v_i = 0$ at

(67) $\begin{equation*} \frac{V_c}{v_h} = -\left( \frac{7 \kappa}{1 + 3 \kappa} \right) \end{equation*}$

which gives $V_c/v_h=-1.75$ for an ideal rotor ( $\kappa=1$ ). In practice, a real (actual) autorotation in axial flight occurs at a slightly higher rate than this because, in addition to induced losses at the rotor, there is also a proportion of profile losses to overcome. In a real autorotation, then

(68) $\begin{equation*} P = \kappa T \left( V_c + v_i \right) + P_0 = 0 \end{equation*}$

Therefore, in a stable autorotation, an energy balance must exist where the decrease in potential energy of the rotor $T V_c$ balances the sum of the induced $(\kappa Tv_i)$ and profile $(P_0)$ losses of the rotor. Using Eq. 68, this condition is achieved in vertical descent when

(69) $\begin{equation*} \frac{V_c + v_i}{v_h} = -\frac{P_0}{\kappa T v_h} = - \frac{P_0 \sqrt{2 \varrho \, A}}{\kappa T^{3/2}} = - \frac{P_0 \sqrt{2 \varrho \, A}}{\kappa W^{3/2}} \end{equation*}$

which depends primarily on the disk loading. Also, using the definition of figure of merit (and assuming the induced and profile losses do not vary substantially from the hover values), then

(70) $\begin{equation*} \frac{P_0 \sqrt{2 \varrho \, A}}{T^{3/2}} = \left( \frac{1}{FM} - \kappa \right) \end{equation*}$

Using Eq. 66 for the induced velocity with Eq. 70 gives the real autorotation condition

(71) $\begin{equation*} \frac{V_c}{v_h} = -\frac{FM^{-1} - \kappa}{1 + 3 \kappa} - \frac{7 \kappa}{1 + 3 \kappa} \end{equation*}$

The first term on the right-hand side of Eq. 71 will vary in magnitude from $-0.04$ to $-0.09$ , depending on the rotor efficiency. Compared to the second term, the extra rate of descent required to overcome profile losses is relatively small. Therefore, based on the preceding, it is apparent that a real vertical autorotation will occur for values of $V_c/v_h$ between $-1.85$ and $-1.9$ .

It is found that autorotations must be performed at relatively high rates of descent with a helicopter. Using the result that $v_h \approx 14.49 \sqrt{T/A}$ , where ${T/A}$ is in lb/ft ${^{2}}$ , gives $V_c \approx -26.81 \sqrt{T/A}$ ft s $^{-1}$ for autorotation at sea level conditions, which for a representative disk loading of 10 lb ft $^{-2}$ leads to a vertical rate of descent of about 5,000 ft min $^{-1}$ . However, as will be discussed later in this chapter, with some forward speed, the power required at the rotor is considerably lower than in the hover case.

Check Your Understanding #5 – Climb power required

A helicopter weighing 6,000 lb is required to hover and climb vertically at 600 ft/min. The main rotor’s radius is 20 ft, and its figure of merit is 0.75. Determine the power required. Assume sea level conditions. Discuss the factors that will determine a helicopter’s maximum vertical climb rate.

Show solution/hide solution.

The power required in an axial climb can be estimated from the momentum theory result that

$\frac{P}{P_h} = \frac{V_c}{2v_h} + \sqrt{ \left( \frac{V_c}{2 v_h}\right)^2 +1 } \approx \frac{V_c}{2v_h} + 1 \mbox{~~for low to moderate climb rates.} \label{powerclimb3}$

In hover, the induced velocity, $v_h$ , is

$v_h = \sqrt{ \frac{T}{2 \varrho \, A} } = \sqrt{ \frac{ 6,000}{2 \times 0.00238 \times \pi \times (20.0)^2 }} = 31.67~\mbox{ft/s}$

where at sea level conditions $\varrho = 0.00238$ slugs/ft ${^3}$ . The power required to hover will be

$P_h = (1/0.75) \frac{T^{3/2}}{\sqrt{ 2 \varrho \, A}} = \frac{ 1.333 \times (6,000)^{3/2}}{\sqrt{2 \times 0.00238 \times \pi \times (20.0)^2 }} \times \frac{1}{550} = 460.66~\mbox{hp}$

The power required to climb at 600 ft/min (10 ft/s) will be

$P \approx P_h \left( \frac{V_c}{2 v_h} + 1 \right) = 460.66 \left( \frac{10.0}{2 \times 31.67} + 1 \right) = 533.49~\mbox{hp}$

The maximum rate of climb of the helicopter will be determined by the excess power available over and above that is required to hover at the same weight and density altitude. The vertical drag produced on the airframe may also be a factor in the climb condition, albeit at high climb rates.

Hovering Coaxial Rotor

The recent interest in coaxial rotor systems has been driven by advancements in electric propulsion, urban air mobility (UAM), and military high-speed VTOL development. Coaxial rotors give a helicopter a smaller footprint, making them ideal for eVTOL air taxis and uncrewed aerial vehicles (UAVs). Still, this advantage comes at a price, including significantly lower aerodynamic efficiency for a given net thrust, i.e., higher power requirements. The main reason is that the lower rotor operates in the wake of the upper rotor, thereby incurring higher aerodynamic losses.

There are not one but four primary cases of interest for a coaxial rotor system: 1. The two rotors corotate in the same plane (or very nearly so in practice) and are operated at the same individual values of thrust; 2. The two rotors corotate in the same plane at different thrusts but are operated at a balanced (equal and opposite) torque; 3. The rotors are operated at the same individual thrust values, but the lower rotor operates in the fully developed slipstream, i.e., in the fully contracted wake of the upper rotor; 4. The rotors are operated at balanced torque, with the lower rotor operating in the fully contracted wake of the upper rotor. Solutions for each one of these operating cases can be derived using the momentum theory.

Corotation in the Same Plane

If the two rotors of a coaxial corotate in the same plane, they can be operated at the same individual thrust values or a balanced torque, the latter being more practical. The momentum theory can analyze both conditions.

Equal Thrusts

Assume first that the distance between the rotor planes is infinitesimally small, as shown in the figure below, such that they share the same induced velocity $v_e$ and that each rotor of the system provides an equal fraction of the total system thrust (i.e., $W=2T$ ) where $T_u = T_l = T = W/2$ . The effective induced velocity of the rotor system will be

(72) $\begin{equation*} v_u = v_l = \sqrt{ \frac{T}{\varrho A} } = \sqrt{ \frac{W}{2\varrho A} } = v_e \end{equation*}$

where $A$ is the disk area of any one rotor. Therefore, the induced power of the system, $P_{i}$ , is

(73) $\begin{equation*} (P_i)_{\rm{\rm coax}} = 2 \left( T v_e \right) = 2T \sqrt{ \frac{2T}{2\varrho A} } = W \sqrt{\frac{W}{2 \varrho A}} = \frac{W^{3/2}}{\sqrt{2 \varrho A}} \end{equation*}$

Flow model for a coaxial rotor in hover where the co-rotating rotors are closely spaced.

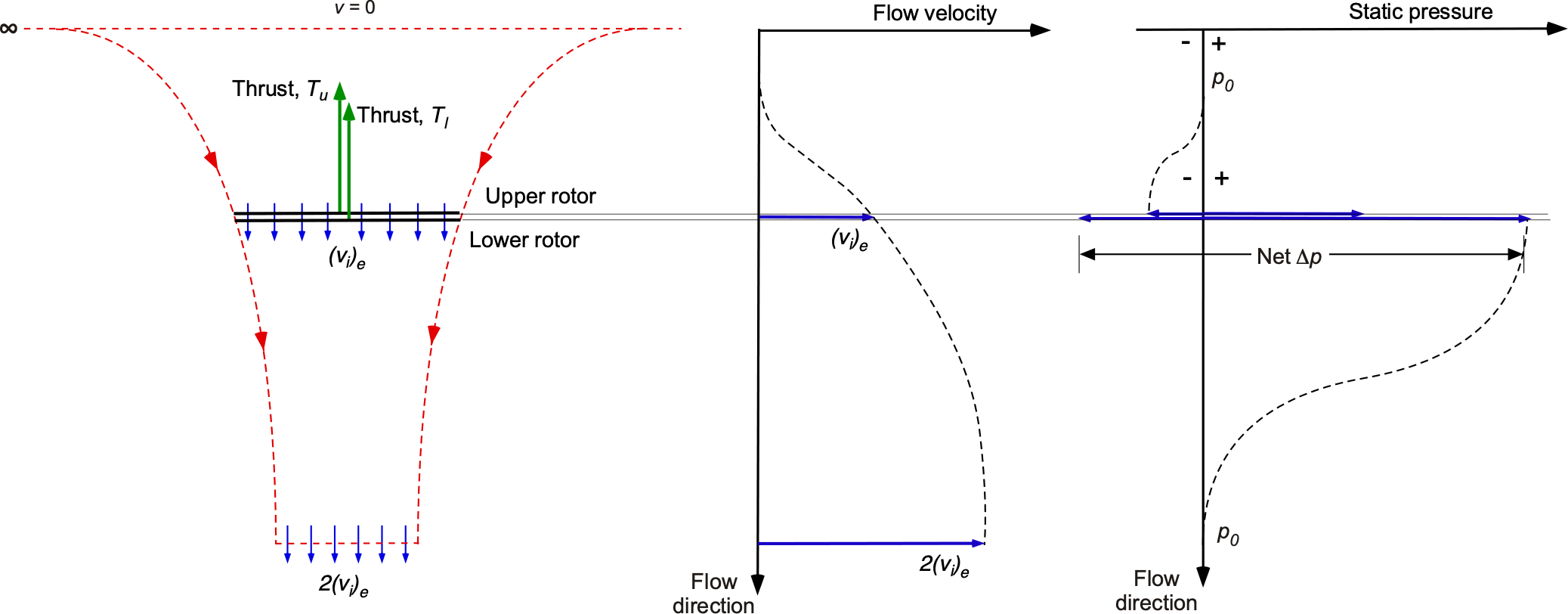
One way to compare coaxial rotor performance levels is to consider each rotor operating separately as entirely free, isolated rotors. This is equivalent to comparing the coaxial rotor with two single isolated rotors operated at the same disk loading as either rotor of the coaxial. The main point is to compare rotor performance levels based on the same disk loading to avoid adversely biasing the results.

The induced power for either isolated rotor will be $Tv_u$ (the upper rotor is equivalent to the isolated rotor because its performance is assumed to be unaffected by the lower rotor), and so for the two separate rotors, the total induced power is

(74) $\begin{equation*} (P_i)_{\rm isolated} = 2 \left( \frac{T^{3/2}}{\sqrt{2 \varrho A}} \right) = \frac{2 T^{3/2}}{\sqrt{2 \varrho A}} = \frac{W^{3/2}}{\sqrt{4 \varrho A}} \end{equation*}$

The interference-induced power factor $\kappa_{\rm int}$ for the coaxial rotor system can then be defined as the ratio of the results in Eqs. 73 and 74, giving

(75) $\begin{equation*} \kappa_{\rm int} = \frac{(P_i)_{\rm coax}}{(P_i)_{\rm isolated}} = \left( \frac{W^{3/2}}{\sqrt{2 \varrho A}}\right)\left( \frac{\sqrt{4 \varrho A}}{W^{3/2}} \right) = \sqrt{2} = 1.4142 \end{equation*}$

In this case, there is a 41% increase in the induced power required relative to the power required to operate the same two rotors of the system separately in complete isolation. This is a significant loss in performance. The net ideal induced power of the coaxial rotor system can, therefore, be written simply as

(76) $\begin{equation*} (P_i)_{\rm{\rm coax}} = 2 \kappa_{\rm int} \left( \frac{T^{3/2}}{\sqrt{2\varrho A}} \right) = \kappa_{\rm int}\frac{W^{3/2}}{\sqrt{4\varrho A}} \end{equation*}$

Notice that two single rotors each of area $A$ and each carrying a thrust $T$ is equivalent to one single rotor of thrust $2T$ and of twice the disk area, i.e., one single rotor with its radius increased by a factor $1/\sqrt{2}$ and with the same disk loading ${T/A}$ . Of course, in practice, such an equivalent single rotor must have the same net solidity as the coaxial rotor for any equivalence to be considered valid. Furthermore, notice that the wake area contraction for the coaxial rotor under these assumptions is $1/2$ , the same as the contraction for a single rotor.

Balanced Torques

In practice, the two rotors of the coaxial rotor system are operated at whatever individual thrust levels are necessary to give zero net torque, i.e., they must be operated at equal and opposite torque levels. However, if the two rotors are sufficiently close that they co-rotate in substantially the same plane at the same thrust, they must also require the same torque. This is because both rotors share the same value of induced velocity (i.e., Eq. 72). It is, therefore, easily shown that for the upper rotor, the induced power required is

(77) $\begin{equation*} P_u = T_u v_e \equiv T v_e = T \sqrt{ \frac{2T}{2\varrho A} } = T \sqrt{ \frac{T}{\varrho A} } \end{equation*}$

and for the lower rotor, the induced power required is

(78) $\begin{equation*} P_l = T_l v_e \equiv T v_e = T \sqrt{ \frac{2T}{2\varrho A} } = T \sqrt{ \frac{T}{\varrho A} } \end{equation*}$

so that $P_u = P_l = P_i$ . This means that for this second case of a coaxial rotor system with the rotors in the same plane operated at the same torque, $\kappa_{\rm int} = 1.4142$ , as found previously. Furthermore, it should be noted that where the rotors operate in the same plane, a torque balance can only be achieved if $T_u = T_l$ ; other variations of thrust sharing will spoil the torque balance.

Lower Rotor in the Slipstream of the Upper Rotor

The foregoing momentum analysis of the coaxial rotor problem is overly pessimistic compared to measurements on coaxial rotors. One often cited reason for the overprediction of induced power is related to the actual (finite) spacing between the two rotor planes. Generally, in practical coaxial designs, the rotors are spaced sufficiently far apart to prevent inter-rotor blade collisions from blade flapping so that the lower rotor typically always operates in the fully contracted wake of the upper rotor. This is justified by Taylor’s flow visualization results, as shown in the photograph below, where the wake contracts quickly below the upper rotor and can be considered fully contracted when the lower rotor ingests it.

Flow visualization of a coaxial rotor where the lower rotor operates in the slipstream of the upper rotor.

Proceeding by assuming that there is no non-ideal wake contraction and that the lower rotor does not affect the inflow, performance, or wake contraction of the upper rotor,^[3] then the inner one-half of the disk area of the lower rotor must operate in the slipstream velocity induced by the upper rotor. This situation is a more complicated physical problem to model, in general, because it involves wake-blade interactions and local viscous effects at the edges of the wake boundary. However, the solution can be approached following the same principles and assumptions made with the classic momentum theory.

Equal Thrusts

The flow model for this alternative situation is shown in the figure below. Assume first that the two rotors operate at the same thrust values, i.e., $T_u = T_l = T$ . The induced velocity at the upper rotor is

(79) $\begin{equation*} v_u = \sqrt{ \frac{T}{2 \varrho A} } \end{equation*}$

The fully contracted wake produced by the upper rotor has an area of $A/2$ with slipstream velocity $2v_u$ . This represents the ideal case, although, in practice, the wake contraction may not be as significant as this. Nevertheless, the ideal case represents the smallest fraction of the disk area on the lower rotor that would be affected by the upper rotor, so it must represent the minimum induced loss condition. Therefore, at the plane of the lower rotor, there is a velocity of $2v_u + v_l$ over the inner one-half of the disk area. Over the outer one-half of the disk area, the induced velocity is $v_l$ . Assume that the velocity in the fully developed slipstream of the lower rotor (plane 3) is uniform with velocity $w_l$ .

Flow model for a coaxial rotor in hover where the lower rotor operates in the fully developed slipstream of the upper rotor.

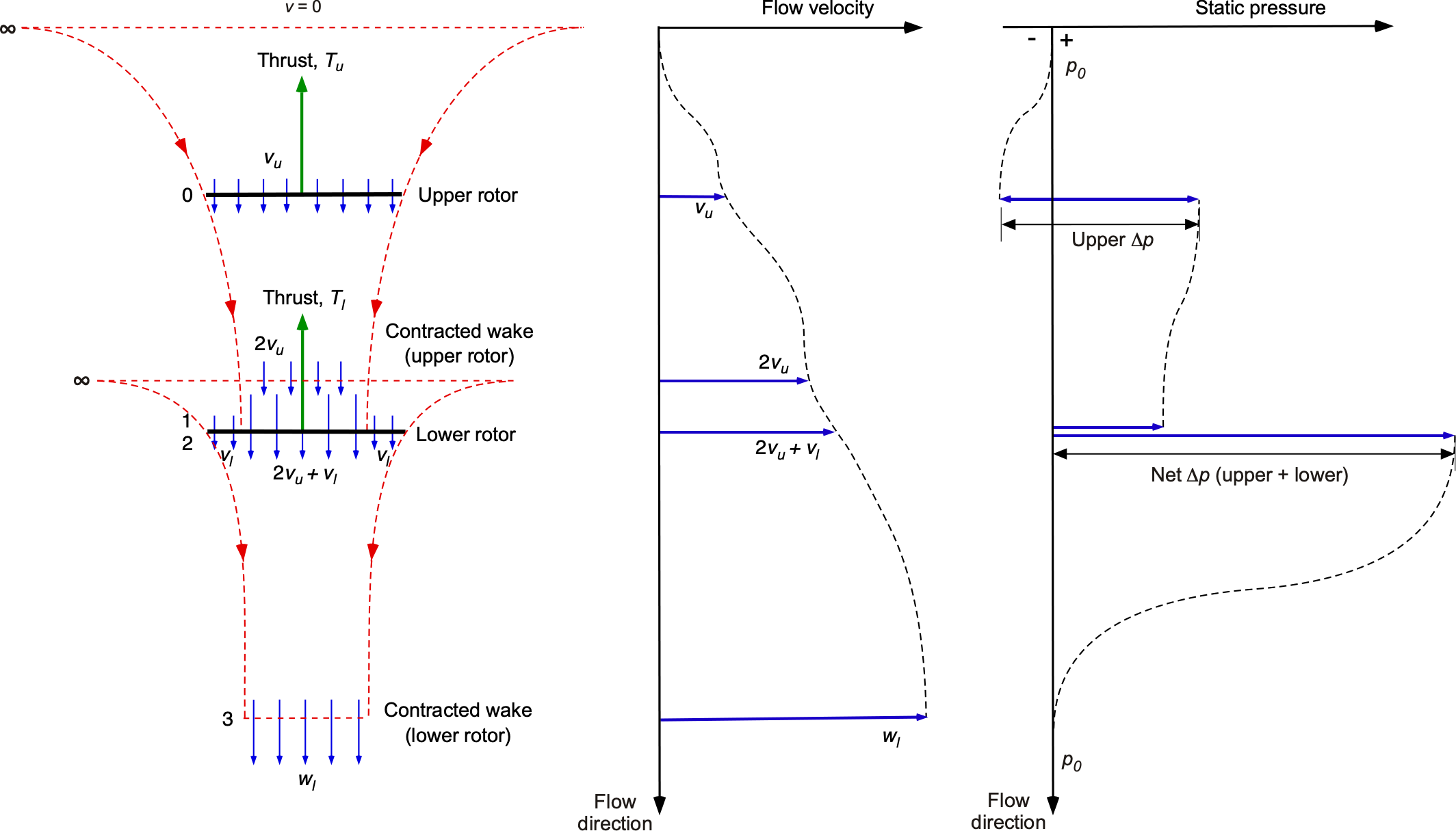
The mass flow rate of air through the upper rotor is $\varrho A v_u$ , so the momentum flux exiting in the slipstream of the upper rotor is $(\varrho A v_u) 2 v_u = 2 \varrho A v_u^2$ . This is the momentum flux of the air entering the lower rotor. The mass flow rates over the inner and outer parts of the lower rotor are $\varrho (A/2)(2v_u + v_l)$ and $\varrho (A/2) v_l$ , respectively. Therefore, the total mass flow rate through the lower rotor, $\overbigdot{m}$ , is

(80) $\begin{equation*} \overbigdot{m} = \varrho \frac{A}{2} \left( 2 v_u + v_l \right) + \varrho \left( \frac{A}{2} \right) v_l = \varrho A \left( v_u + v_l \right) \end{equation*}$

The momentum flux out of plane 3 is ${\overbigdot{m} w_l}$ assuming uniform velocity, so the thrust on the lower rotor is

(81) $\begin{equation*} T_l = \varrho A \left( v_u + v_l \right) w_l - 2 \varrho A v_u^2 \end{equation*}$

The work per unit time done on the air by the lower rotor is

(82) $\begin{equation*} P_l = T_l ( v_u + v_l) \end{equation*}$

This is equal to the gain in kinetic energy of the air in the slipstream. Therefore, expanding out gives

(83) $\begin{eqnarray*} T_l ( v_u + v_l) & = & \frac{1}{2} \varrho A \left( v_u + v_l \right) w_l^2 - \frac{1}{2} \varrho \left( \frac{A}{2} \right) ( 2 v_u) (2 v_u)^2 \nonumber \\ & = & \frac{1}{2} \varrho A \left( v_u + v_l \right) w_l^2 - 2 \varrho A v_u^3 \end{eqnarray*}$

Assuming equal thrusts on the upper and lower rotors (i.e., $T_l = T_u = T$ ), then $T = 2 \varrho A v_u^2$ . From Eq. 81

(84) $\begin{equation*} T_l = T = \frac{1}{2} \varrho A (v_u + v_l) w_l \end{equation*}$

and from Eq. 83 then

(85) $\begin{equation*} { T ( 2 v_u + v_l) = \frac{1}{2} \varrho A (v_u + v_l ) w_l^2 } \end{equation*}$

Using Eqs.~84 and 85 gives $w_l = 2 v_u + v_l$ and substituting this into Eq. 84 and remembering that $T = 2\varrho A v_u^2$ gives

(86) $\begin{equation*} 4 \varrho A v_u^2 = \varrho A \left( v_u + v_l \right) w_l = \varrho A \left( v_u + v_l \right) \left( 2 v_u + v_l \right) \end{equation*}$

Rearranging this latter equation as a quadratic in terms of $v_l$ and solving gives

(87) $\begin{equation*} v_l = \left( \frac{-3 + \sqrt{17}}{2} \right) v_u = 0.5616 v_u \end{equation*}$

The interference-induced power factor for this case can now be evaluated. The power for the upper rotor is $P_u = T v_u$ , and for the lower rotor, $P_l = T ( v_u + v_l) = 1.5616 T v_u$ . Therefore, the sum of the powers of both rotors is $2.5616 T v_u$ . This is compared to $2 T v_u$ when the rotors operate in isolation.^[4] This means that the interference-induced power factor, $\kappa_{\rm int}$ , is given by

(88) $\begin{equation*} \kappa_{\rm int} = \frac{(P_i)_{\rm coax}}{(2 P_i)_{\rm isolated} } = \frac{2.5616 T v_u}{2 T v_u} = 1.2808 \end{equation*}$

This now represents a 28.1% increase in the induced losses compared to a 41% increase when the two rotor disk planes have no vertical separation, i.e., in this case, $\kappa_{\rm int}$ = 1.2808. Such a result is much closer to the values that can be indirectly deduced from most experiments; see, for example, the coaxial rotor measurements of Harrington (hover) and Dingeldein (forward flight).

In this case, the wake area contraction differs for the single rotor and the coaxial rotors that corotate in the same plane. The wake area can be found from the continuity of the flows between the lower rotor and the far downstream wake, i.e.,

(89) $\begin{equation*} \frac{A_3}{A} = \frac{v_u + v_l}{2v_u + v_l} = \frac{1.5616}{2.5616} = 0.6096 \end{equation*}$

which means that the far downstream wake will not contract quite as much as for a single rotor.

Balanced Torques

A comparison of performance based on equally balanced torques between the upper and lower rotors is a much more realistic operational assumption for a coaxial rotor. In this case, two solutions are of interest, and they differ in how they evaluate the interference-induced power factor. From the results of Eq. 81 and Eq. 83) with a torque balance such that $P_u=P_l$ (i.e., equal rotor rotational and tip speeds), then

(90) $\begin{equation*} T_u v_u = T_l \left( v_u + v_l \right) \end{equation*}$

Multiplying Eq. 81 by $v_u(v_u + v_l)$ and rearranging gives

(91) $\begin{equation*} P_l \left( 2 v_u + v_l \right) = \varrho A \left( v_u + v_l \right)^2 v_u w_l \end{equation*}$

Now, $P_u = 2\varrho A v_u^3 = P_l$ at the torque balanced condition, and using Eqs.~82 and 83 leads to

(92) $\begin{equation*} P_l = \frac{1}{4} \varrho A \left( v_u + v_l \right) w_l^2 \end{equation*}$

Substituting Eq. 92 into Eq. 91 and rearranging gives

(93) $\begin{equation*} w_l = 4 v_u \left( \frac{ v_u + v_l }{ 2 v_u + v_l } \right) \end{equation*}$

Again, $P_u = 2\varrho A v_u^3 = P_l$ and substituting Eq. 93 in Eq. 82 gives

(94) $\begin{equation*} P_l = 2 \varrho A v_u^3 = 8 \varrho A (v_u + v_l ) v_u^2 \frac{ (v_u + v_l )^2}{(2v_u + v_l)^2} - 2\varrho A v_u^3 \end{equation*}$

which, on rearrangement, leads to

(95) $\begin{equation*} 2 v_l^3 + 5 v_u v_l^2 + 2 v_u^2 v_l - 2 v_u^2 = 0 \end{equation*}$

Solving this cubic numerically leads to $v_l = 0.4375 v_u$ . Again, notice that the area contraction of the far downstream wake is different from the prior cases, in this case being

(96) $\begin{equation*} \frac{A_3}{A} = \frac{v_u + v_l}{2v_u + v_l} = \frac{1.4375}{2.4375} = 0.5897 \end{equation*}$

In this case, the interference-induced power factor can now be evaluated using the performance of the two isolated rotors (or equivalent single rotors at the same disk loading) when they are operated independently at equal thrust, as done previously. For a torque balance, then

(97) $\begin{equation*} T_u v_u = T_l ( v_u + v_l ) \end{equation*}$

The thrust-sharing ratio is then

(98) $\begin{equation*} \frac{T_u}{T_l} = \frac{v_u + v_l}{v_u} = 1 + \frac{v_l}{v_u} = 1.4375 \end{equation*}$

As before, the interference-induced power factor is written as

(99) $\begin{equation*} \kappa_{\rm int} = \frac{(P_i)_{\rm coax}}{(2 P_i)_{\rm isolated} } = \frac{P_u + P_l}{2 T v_u} \end{equation*}$

where the basis of comparison is with two isolated rotors operating at equal thrusts $T = W/2$

Now $P_u = P_l$ at the torque balance, and also $W = 2 T = T_u + T_l$ and $T_u/T_l$ = 1.4375, so that

(100) $\begin{equation*} \kappa_{\rm int} = \frac{2 P_u}{(T_u + T_l) v_u} = 2 \sqrt{2} \left( \frac{T_u}{T_l} \right)^{3/2} \left(1 + \frac{T_u}{T_l} \right)^{-3/2} = 1.2810 \end{equation*}$

An alternative basis of comparison with the coaxial rotor system is to use the performance of the two rotors (or equivalent single rotor) when they are operated independently but at the same thrust-sharing ratio as found in the torque-balanced case. The interference-induced power factor in this situation is

(101) $\begin{equation*} \kappa_{\rm int} = \frac{(P_i)_{\rm coax}}{(2 P_i)_{\rm isolated} } = \frac{P_u + P_l}{T_u v_u + T_l v_l} \end{equation*}$

Again, $P_u = P_l$ at the torque balance, so in this case

(102) $\begin{eqnarray*} \kappa_{\rm int} & = & \frac{2 P_u}{\displaystyle{\frac{T_u^{3/2}}{\sqrt{2 \varrho A}} + \frac{T_l^{3/2}}{\sqrt{2 \varrho A}}}} = \frac{2 T_u^{3/2}}{T_u^{3/2} + T_l^{3/2}} \nonumber \\[10pt] & = & \frac{2 \displaystyle{\left( \frac{T_u}{T_l}\right)^{3/2}}}{\displaystyle{\left(\frac{T_u}{T_l}\right)^{3/2}} + 1 } = 1.2657 \end{eqnarray*}$

This means that for the assumptions made in this (final) case, the interference-induced power factor is 1.2657, which is just slightly smaller than the value obtained when the basis of comparison is against two isolated rotors operating at equal thrusts or an equivalent single rotor of equal disk loading.

Remember that these foregoing results present the absolute minimum induced losses for each coaxial configuration under the stated assumptions and set the baseline for comparison with any real coaxial rotor system regarding operating efficiency.

Validity of the Coaxial Theory

Measurements, in this case, of a hovering coaxial rotor can establish the validity of the rotor momentum theory. The importance of the hovering condition cannot be underestimated, as a rotor with poor hovering performance will likely underperform in forward flight and other parts of the flight envelope.

There are very few measurements of coaxial rotor performance, but Harrington made performance measurements on two sets of nominally full-scale corotating coaxial rotors operating in hovering conditions. Both rotors had a diameter of 25 ft (7.62 m) and untwisted blades. Rotor 1 had two blades per rotor that were tapered in planform (approximately a 3:1 taper ratio) and corresponding thickness, with a thrust-weighted solidity $\sigma$ , of 0.027 (i.e., 2 $\sigma =$ 0.054 when operated as a coaxial). Rotor 2 had two blades per rotor that were tapered only in thickness-to-chord ratio with a solidity of 0.076 (i.e., 2 $\sigma =$ 0.152 as a coaxial). Rotor 1 had an inter-rotor plane spacing of 0.186 $R$ , and Rotor 2 had a rotor spacing of 0.16 $R$ . Both sets of rotors were operated at a torque balance.

In summary, the theoretical power coefficient for the single-rotor system can be calculated using

(103) $\begin{equation*} C_P = \frac{ \kappa \ C_T^{3/2}}{\sqrt{2}} + \bigg( \frac{ \sigma \ C_{d_{0}} }{8} \bigg) \end{equation*}$

The corresponding power coefficient for the coaxial rotor system can be calculated using

(104) $\begin{equation*} C_P = \frac{ \kappa_{\rm int} \; \kappa (C_{T_{u}} + C_{T_{l}})^{3/2}}{2} + \bigg( \frac{\sigma C_{d_{0}} }{4} \bigg) = \frac{ \kappa_{\rm int} \; \kappa C_W^{3/2}}{2} + \bigg( \frac{\sigma C_{d_{0}} }{4} \bigg) \end{equation*}$

where $\kappa_{\rm int}$ represents the rotor-on-rotor induced power interference.

The two figures below show the results for the Harrington Rotor 1 and Rotor 2. It can be seen from the results that average values of $\kappa$ = 1.1 for the Harrington 1 rotor and $\kappa$ = 1.2 for the Harrington 2 rotor with $\kappa_{\rm int}$ = 1.280 in each case (Eqs. 103 and 104) give reasonably good overall agreement with the measurements of the power polars. Because the upper rotor generally operates at a higher thrust than the lower rotor to achieve a torque balance, the assumption that $C_{T_{u}}/C_{T_{l}}$ = 1.2 gives a slightly better agreement with measurements than an assumption of balance thrusts.

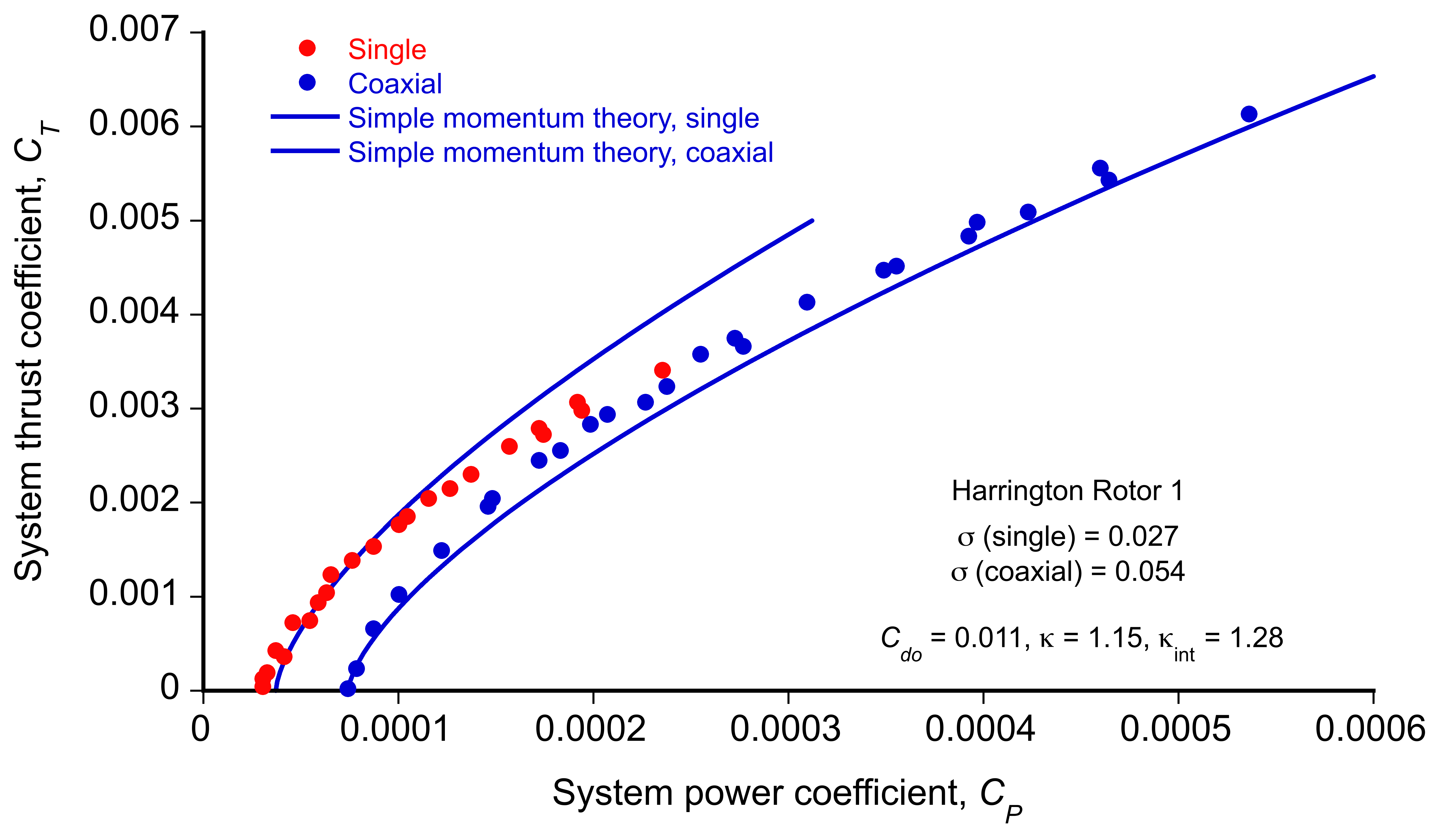
Power polar for a single and coaxial rotor using the momentum theory compared to the Harrington 1 rotor system measurements.

Notice that the inferred value of $\kappa_{\rm int}$ of 1.28 is just slightly larger than the minimum theoretical value of the interference-induced power factor with the lower rotor operating in the fully developed slipstream of the upper rotor, i.e., $\kappa_{\rm int}$ = 1.2657. Overall, these results confirm that the coaxial rotor behaves essentially as two isolated single rotors operating with the same disk loading as either of the upper or lower coaxial rotors, and with an induced interference effect between the rotors accounted for by an average induced power interference factor $\kappa_{\rm int}$ .

While it will be seen from the results that the agreement between predictions and experiment^[5] are not uniformly good over all the operating conditions of the two sets of rotors. However, the momentum theory still gives a rational basis for analysis and a good benchmark for predicting coaxial rotor performance over some operational thrust ranges. Part of the underprediction in power stems from the assumption that the lower rotor does not affect the upper rotor, which will slightly increase its power requirements. Values of both $\kappa$ and $\kappa_{\rm int}$ are functions of the rotor thrust, and the power required for the upper rotor is dependent on the lower rotor and helps resolve the remaining differences seen here using the momentum theory. The under-prediction of power for the coaxial most likely arises from viscous interactions between the upper and lower rotors.’ Nevertheless, the intrinsic value of the momentum theory is evident in that it still brings out an understanding of the primary performance characteristics of both the single and coaxial rotor systems.

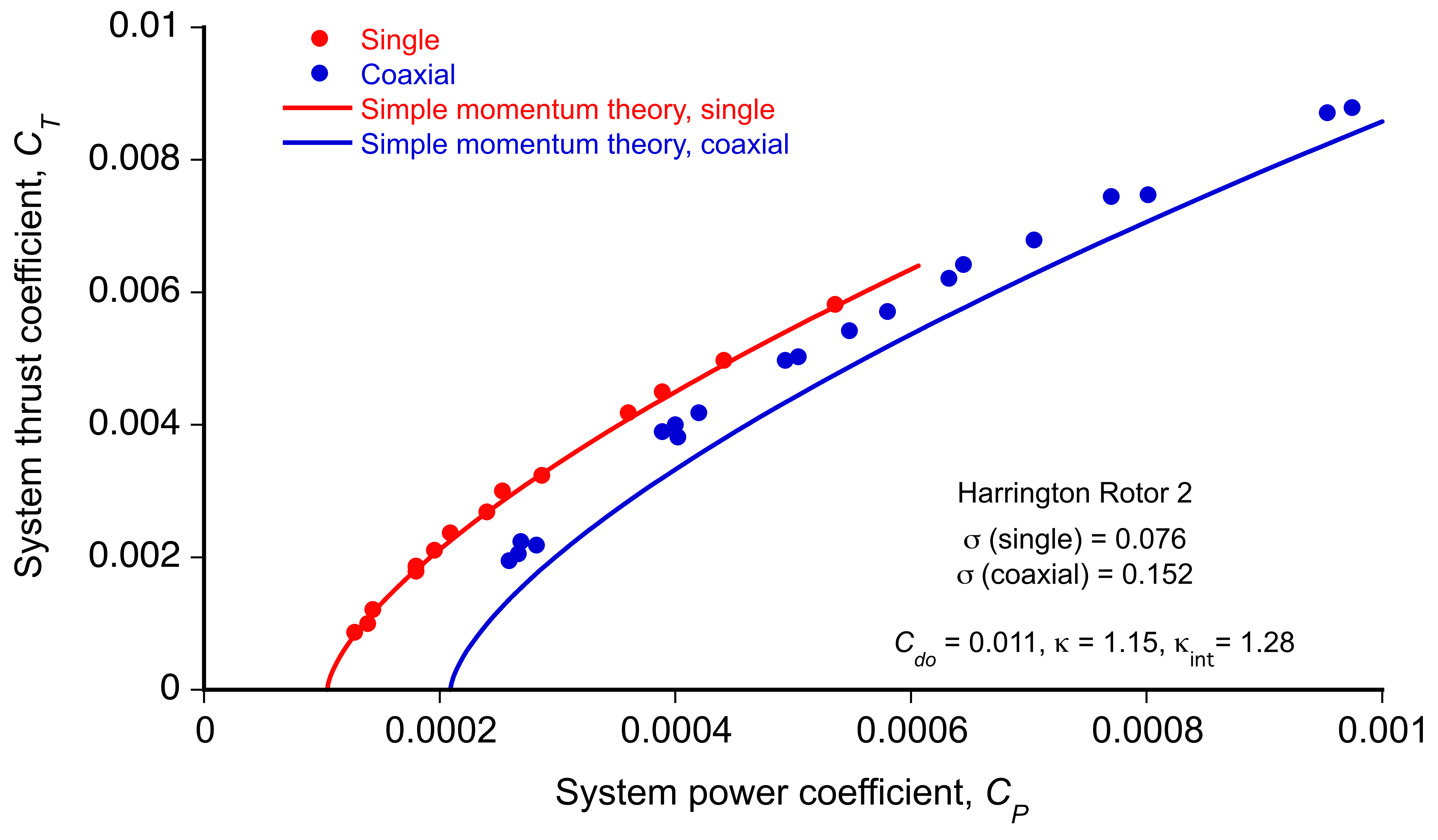
Power polar for a single and coaxial rotor using the momentum theory compared to the Harrington 2 rotor system measurements.

In each case, the two single rotors produce considerably better overall performance (better than 20%) than the coaxial rotor at all net thrust conditions, i.e., when carrying the same net weight. This result is an unambiguous confirmation of the rotor-on-rotor losses associated with a coaxial rotor system, regardless of how a figure of merit might be defined. Rotor 2 (with the higher solidity) is less efficient overall, both as a single and a coaxial.

However, remember that in application, a single rotor system will also incur additional losses from a tail rotor or other anti-torque system, typically about 10% of the net power. When this effect is considered, as a hovering system, the coaxial rotor can be of nearly comparable net aerodynamic efficiency to the single rotor and tail rotor combination, but it is unlikely ever to be better. The coaxial rotor’s clear advantage is that it will always have a smaller diameter relative to a single rotor to generate the same thrust but at the expense of 20% to 40% more power.

Notice that the momentum theory also always requires the a priori specification of $\kappa$ , $\kappa_{\rm int}$ , and $C_{d_{0}}$ , which must be assumed or derived empirically by baselining predictions against some known rotor performance. In this regard, the modified momentum theory only has a postdictive modeling capability.

Forward Flight Analysis

Under forward flight conditions, the rotor moves through the air with an edgewise velocity component parallel to the rotor disk’s plane. Under these conditions, the axisymmetry of the flow through the rotor is lost. Now, the flow field in which the rotor operates is considerably more complex than that of a fixed-wing, giving rise to several aerodynamic problems that ultimately limit the rotor’s performance.

Flow Environment

The overall aerodynamic complexity of the helicopter in forward flight can be appreciated from the schematic below. One issue is that blade tips on the advancing side of the rotor disk can start to penetrate into supercritical and transonic flow regimes, with the associated formation of compressibility zones and, ultimately, strong shock waves. In addition, the occurrence of wave drag and the possibilities of shock-induced flow separation must be considered, as the onset of either or both phenomena requires much more power to drive the rotor. The periodic formation of shock waves is also a source of obtrusive noise.

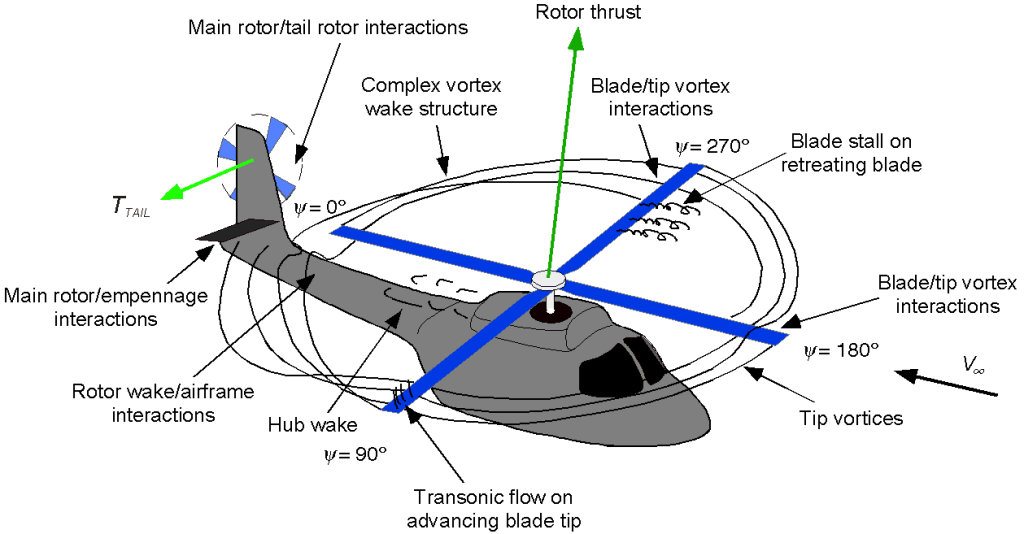
When compressibility effects manifest, the increased power demands on the rotor system will eventually limit forward flight speeds. Although compressibility effects on contemporary rotors can be somewhat relieved by using swept tip blades and thin “transonic” airfoils, the problems of increased power requirements and noise are only delayed to moderately higher forward flight speeds and are not eliminated.

Momentum Theory Analysis in Forward Flight

Despite the inherently more complicated nature of the rotor flow in forward flight, the simple momentum theory can be extended to forward flight based on certain assumptions. Because helicopter rotors are required to produce both a lifting force (to overcome the helicopter’s weight) and a propulsive force (to propel the helicopter forward), the rotor disk must be tilted forward at an angle of attack relative to the oncoming flow. The following treatment of rotor performance in forward flight was first derived by Glauert, where the analysis is performed with respect to an axis aligned with the rotor disk, as shown in the figure below.

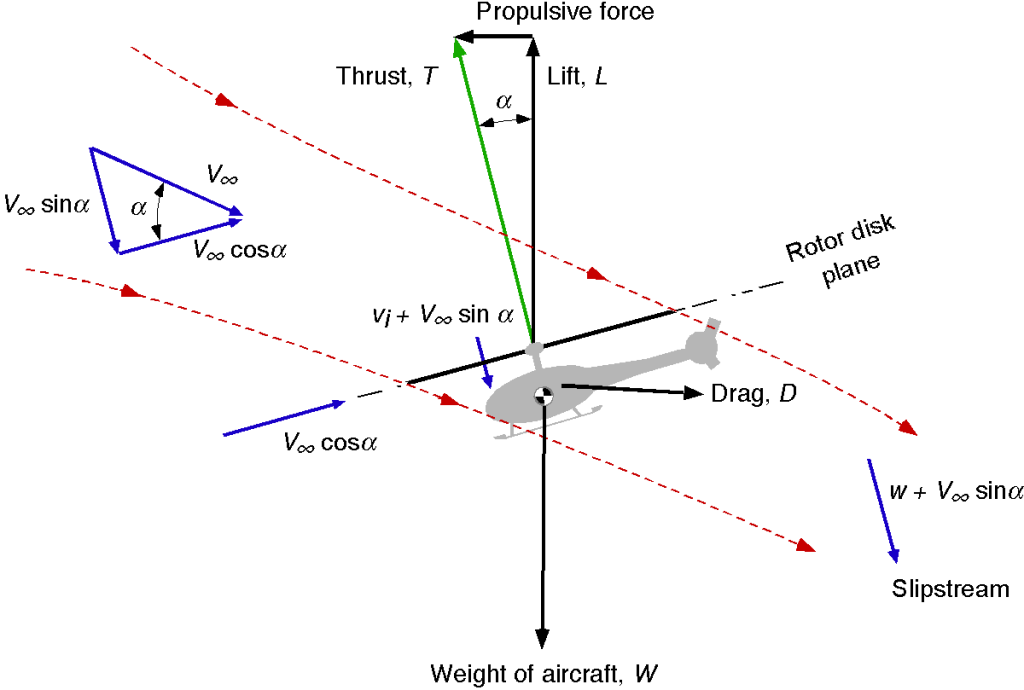
Glauert’s flow model for the momentum analysis of a rotor in forward flight.

Mass Flow Rate

In this case, Glauert defines the mass flow rate, $\overbigdot{m}$ , through the actuator disk as

(105) $\begin{equation*} \overbigdot{m} = \varrho \, A \, U \end{equation*}$

where $U$ is the resultant velocity at the disk as given by

(106) $\begin{equation*} U = \sqrt{(V_{\infty}\cos\alpha)^2 + (V_{\infty}\sin\alpha + v_i)^2} = \sqrt{ V_{\infty}^2 + 2V_{\infty}v_i \sin\alpha + v_i^2 } \end{equation*}$

Thrust

The application of the conservation of momentum in a direction normal to the disk gives

(107) $\begin{equation*} T = \overbigdot{m} ( w + V_{\infty}\sin\alpha) - \overbigdot{m} V_{\infty}\sin\alpha = \overbigdot{m} w \end{equation*}$

Power Required

By the application of conservation of energy, then

(108) $\begin{equation*} P = ( v_i + V_{\infty}\sin\alpha ) = \frac{1}{2} \overbigdot{m} (V_{\infty}\sin\alpha + w)^2 - \frac{1}{2} \overbigdot{m} V_{\infty}^2 \sin^2\alpha \end{equation*}$

which gives

(109) $\begin{equation*} P = \frac{1}{2} \overbigdot{m} \left( 2V_{\infty}w\sin\alpha + w^2 \right) \end{equation*}$

Using Eqs. 107 and 109 then

(110) $\begin{equation*} 2wv_i + 2V_{\infty}w\sin\alpha = 2V_{\infty}w\sin\alpha + w^2 \end{equation*}$

giving $w = 2v_i$ , the same relationship as the axial flight cases. Therefore,

(111) $\begin{equation*} { T = 2 \overbigdot{m} v_i = 2 \varrho \, A \, U \, v_i = 2 \varrho \, A \, v_i \sqrt{V_{\infty}^2 + 2V_{\infty}v_i \sin\alpha + v_i^2} } \end{equation*}$

Notice that for hovering flight, $V_{\infty}=0$ , so that Eq. 111 reduces to the result for hover (Eq. 7), i.e.,

(112) $\begin{equation*} T = 2\varrho \, A \, v_i^2 = 2\varrho \, A \, v_h^2 \end{equation*}$

As forward flight speed increases such that $V_{\infty} \gg v_i$ , then Eq. 111 reduces to

(113) $\begin{equation*} T = 2 \varrho \, A \, v_i \, V_{\infty} \end{equation*}$

which is called Glauert’s “high-speed” approximation.

The rotor thrust is given by

(114) $\begin{equation*} T = 2 \overbigdot{m} v_i = 2(\varrho \, A \, U) v_i \end{equation*}$

which can be written as

(115) $\begin{equation*} T = 2 \varrho \, A \, v_i \sqrt{(V_{\infty}\cos\alpha)^2 + (V_{\infty}\sin\alpha + v_i)^2} \end{equation*}$

Recall from Eq. 112 that for hovering flight $v_h^2 = T/2\varrho A$ , so the induced velocity $v_i$ in forward flight becomes

(116) $\begin{equation*} v_i = \frac{v_h^2}{\sqrt{(V_{\infty}\cos\alpha)^2 + (V_{\infty}\sin\alpha + v_i)^2}} \end{equation*}$

The idea of a tip speed ratio or advance ratio, $\mu$ , can now be introduced. By using the velocity parallel to the plane of the rotor, then it is defined as

(117) $\begin{equation*} \mu = V_{\infty} \cos\alpha/\Omega \end{equation*}$

In most cases, the value of ${\alpha}$ is sufficiently small so that

(118) $\begin{equation*} \mu = V_{\infty} /\Omega \end{equation*}$

Inflow

The inflow ratio is $\lambda=(V_{\infty} \sin\alpha + v_i)/\Omega R$ so

(119) $\begin{equation*} \lambda = \frac{V_{\infty} \sin\alpha}{\Omega R} + \frac{v_i}{\Omega R} = \mu \tan\alpha + \lambda_i \end{equation*}$

Also, Eq. 116 becomes

(120) $\begin{equation*} \lambda_i = \frac{\lambda_h^2}{\sqrt{\mu^2 + \lambda^2}} \end{equation*}$

But, it is also known from the hover case that $\lambda_h = \sqrt{C_T/2}$ , therefore,

(121) $\begin{equation*} \lambda_i = \frac{C_T}{2\sqrt{\mu^2+\lambda^2}} \end{equation*}$

Finally, the solution for the inflow ratio, $\lambda$ , is

(122) $\begin{equation*} \lambda = \mu\tan\alpha + \frac{C_T}{2 \sqrt{\mu^2+\lambda^2}} \end{equation*}$

This is a form of implicit equation in that the unknown value, $\lambda$ , appears on both sides.

While analytic solutions to Eq. 122 can be found under certain assumptions, using a numerical method to solve for $\lambda$ is preferable. The simplest approach is a fixed-point iteration. The algorithm consists of a loop to iteratively compute new estimates of $\lambda$ until a termination criterion has been met. To this end, Eq. 122 can be written as the iteration equation

(123) $\begin{equation*} \lambda_{n+1} = \mu \tan \alpha + \frac{C_T}{2 \sqrt{\mu^2+\lambda_n^2}} \end{equation*}$

where $n$ is the iteration number. The starting value for $\lambda_0$ is usually the hover value (i.e., $\lambda_0 = \lambda_h=\sqrt{C_T/2}$ ). The error estimator is

(124) $\begin{equation*} \epsilon = \bigg\| \frac{\lambda_{n+1} - \lambda_n}{\lambda_{n+1}} \bigg\| \end{equation*}$

and the convergence criteria is when $\epsilon < 0.0005$ . One normally finds that between 10 and 15 iterations are required for convergence.

Results for the inflow ratio $\lambda/\lambda_h$ computed are shown in the figure below for several different values of ${\alpha}$ (both positive and negative) and over a range of values of $\mu/\lambda_h$ typical of a helicopter, i.e.,

(125) $\begin{equation*} \lambda = \lambda_i + \mu \tan \alpha \end{equation*}$

or

(126) $\begin{equation*} \frac{\lambda}{\lambda_h} = \frac{\lambda_i}{\lambda_h} + \frac{\mu}{\lambda_h} \tan \alpha \end{equation*}$

The induced part of the total inflow $\lambda_i$ decreases with increasing advance ratio, but the total inflow increases and becomes dominated by the $\mu \tan \alpha$ term at higher advance ratios.

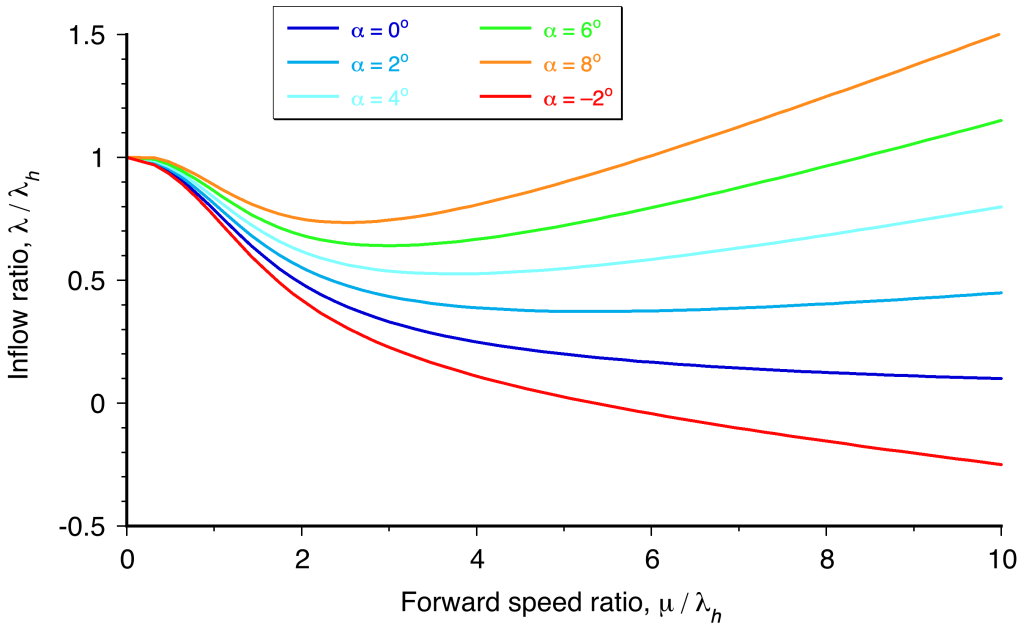
Power Requirements in Forward Flight

The rotor power in forward flight is given by

(127) $\begin{equation*} P = T (V_{\infty}\sin\alpha + v_i) = T V_{\infty}\sin\alpha + T v_i \end{equation*}$

The first term on the right-hand side of the above equation is the power required to propel the rotor forward and climb. The second term is the induced power. As for the axial flight case, the rotor power in forward flight can be referenced to the hovering result, and so

(128) $\begin{equation*} \frac{P}{P_h} = \frac{P}{T v_h} = \frac{T (V_{\infty}\sin\alpha + v_i)}{T v_h} = \frac{ V_{\infty}\sin\alpha + v_i}{v_h} = \frac{\lambda}{\lambda_h} \end{equation*}$

Recall that

(129) $\begin{equation*} \lambda = \mu\tan\alpha + \frac{C_T}{2 \sqrt{\mu^2+\lambda^2}} = \mu\tan\alpha + \frac{\lambda_h^2}{\sqrt{\mu^2+\lambda^2}} \end{equation*}$

Therefore,

(130) $\begin{equation*} \frac{P}{P_h} = \frac{\lambda}{\lambda_h} = \frac{\mu}{\lambda_h} \tan\alpha + \frac{\lambda_h}{\sqrt{\mu^2+\lambda^2}} \end{equation*}$

The first term on the right-hand side of the above equation is the extra power to meet propulsion and climb requirements, whereas the second is the induced power.

The inflow and power required for flight depend on the disk angle of attack, which must always be tilted forward slightly for propulsion, which in turn requires a knowledge of the helicopter’s drag, $D$ . Assuming straight-and-level flight, the disk angle of attack, ${\alpha}$ , can be calculated from a simple force equilibrium, as shown in the figure below.

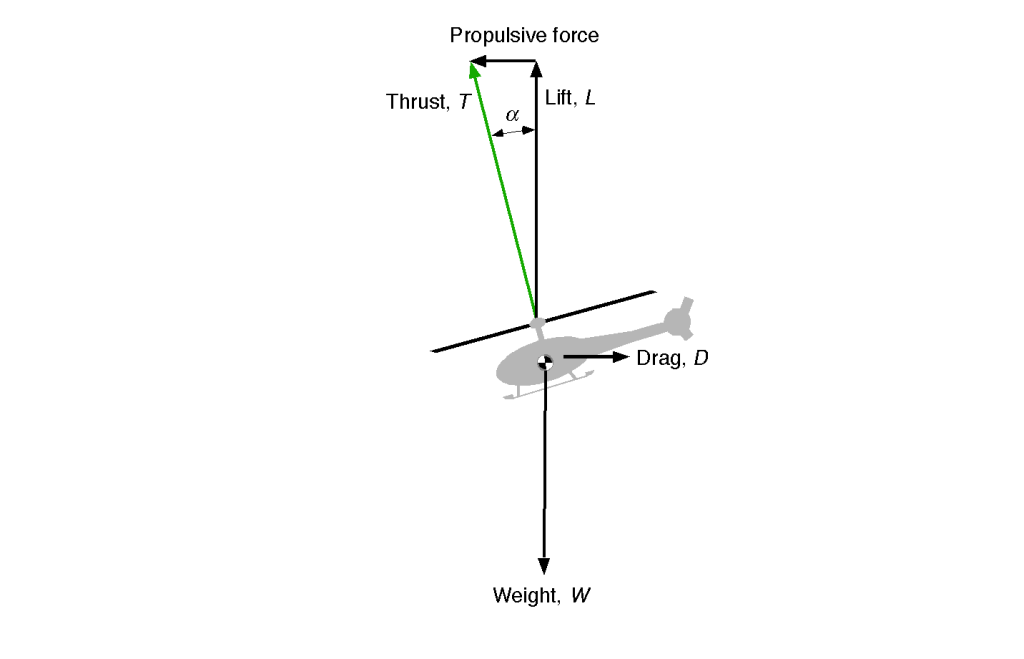
A simple force equilibrium “trim” analysis of a helicopter in forward flight.

For vertical equilibrium $T\cos\alpha = W$ , and for horizontal equilibrium $T\sin\alpha=D\cos \alpha \approx D$ . Therefore, the disk angle of attack can be found from

(131) $\begin{equation*} \tan \alpha = \frac{D}{W} = \frac{D}{L} \approx \frac{D}{T} \end{equation*}$

Therefore, the power equation in straight-and-level flight can be written as

(132) $\begin{equation*} \frac{P}{P_h} = \frac{\mu}{\lambda_h} \left( \frac{D}{T} \right) + \frac{\lambda_h}{\sqrt{\mu^2+\lambda^2}} \end{equation*}$

However, determining the drag $D$ on the helicopter requires knowledge of both the drag on the rotor and the drag on the airframe, the latter of which is called parasitic drag. The rotor drag must be estimated using the blade element theory.

Forward Flight Performance

For a helicopter in level forward flight, the total power required at the rotor, $P$ , can be expressed by

(133) $\begin{equation*} P = P_i + P_0 + P_p \end{equation*}$

where ${P_i}$ is the induced power, ${P_0}$ is the profile power required to overcome viscous losses at the rotor, and ${P_p}$ is the parasitic power required to overcome the drag of the helicopter. Each contributing part can now be analyzed, and the effects can be combined.

Induced Power

The induced power of the rotor, ${P_i}$ , is given by

(134) $\begin{equation*} P_i = \kappa T v_i \end{equation*}$

or in coefficient form

(135) $\begin{equation*} C_{P_{i}}= \kappa C_T \lambda_i \end{equation*}$

where $\kappa$ is the now-familiar empirical correction for many non-ideal effects. The value of $\kappa$ cannot necessarily be assumed independent of the advance ratio. Still, using a mean value between 1.15 and 1.25 is usually sufficiently accurate for preliminary predictions of power requirements.

Substituting the value of $\lambda_i$ in forward flight gives

(136) $\begin{equation*} C_{P_{i}} = \frac{ \kappa C_T^2}{2 \sqrt{\lambda^2 + \mu^2 }} \approx \frac{\kappa C_T^2}{2 \mu} \mbox{ for larger $\mu$} \end{equation*}$

Notice that if the forward velocity is sufficiently high, say $\mu > 0.1$ , then the induced velocity can be approximated by Glauert’s “high-speed” asymptotic result, i.e.,

(137) $\begin{equation*} C_{P_{i}} \approx \frac{\kappa C_T^2}{2 \mu} \end{equation*}$

Profile Power

The profile power coefficient can be approximated as

(138) $\begin{equation*} C_{P_{0}} = \frac{\sigma C_{d_{0}}}{8} ( 1 + K \mu^2) \end{equation*}$

which comes from the blade element theory, where the numerical value of $K$ varies depending on the various assumptions and/or approximations used in the blade element integration.

In practice, a value of $K =\! 4.65$ is often used for helicopter performance predictions for $\mu < 0.5$ . At higher advance ratios, experimental evidence suggests that profile power grows more quickly than given by Eq. 138 as a result of radial and reverse flow and compressibility effects on the rotor.

Parasitic Power

The parasitic power, ${P_p}$ , is a power loss from the drag on the airframe, rotor hub, etc. This drag source can be significant because helicopter airframes are much less aerodynamic than their fixed-wing counterparts, often with regions of large-scale flow separation.

The parasitic power contribution can be written as

(139) $\begin{equation*} { P_p = \left( \frac{1}{2} \varrho \, V_{\infty}^2 S_{\rm{ref}} \, C_{D_{f}} \right) V_{\infty} } \end{equation*}$

where $S_{\rm{ref}}$ is some reference area and $C_{D_{f}}$ is the drag coefficient based on this reference area. In nondimensional form, this becomes

(140) $\begin{equation*} C_{P_{p}} = \frac{1}{2} \left( \frac{S_{\rm{ref}}}{A} \right) \mu^3C_{D_{f}} = \frac{1}{2} \left( \frac{f}{A} \right) \mu^3 \end{equation*}$

where $A$ is the rotor disk area, and ${\scriptstyle{f}}$ ( $=C_{D_{f}} S_{\rm ref}$ ) is known as the equivalent wetted area or equivalent flat-plate area. This area parameter accounts for the drag of the hub, fuselage, landing gear, and so on in aggregate.

The concept of the equivalent wetted area comes from noting that while the drag coefficient can be written in the conventional way as

(141) $\begin{equation*} C_{D_{f}} = \frac{D_f}{\frac{1}{2} \varrho \, V_{\infty}^2 S_{\rm{ref}}} \end{equation*}$

where $S_{\rm{ref}}$ is a reference area, the definition of $S_{\rm{ref}}$ may not be unique. Thus, an equivalent wetted area is used, which is defined as

(142) $\begin{equation*} f = \frac{D_f}{\frac{1}{2} \varrho \, V_{\infty}^2 } \end{equation*}$

Such an approach avoids any confusion that could arise by defining different reference areas.

It is found that values of ${\scriptstyle{f}}$ range from about 10 ft ${^{2}}$ (0.93 m ${^{2}}$ ) on smaller helicopters to as much as 50 ft ${^{2}}$ (4.65 m ${^{2}}$ ) on large utility helicopter designs.

Tail Rotor Power

The power required by the tail rotor typically varies between 3% and 5% of the main rotor power in routine flight and up to 20% at the extremes of the flight envelope. It is calculated similarly to the main rotor power, with the required thrust equal to the value necessary to balance the main rotor torque reaction on the fuselage. Using vertical tail surfaces to produce a side force in forward flight can help reduce the power fraction required for the tail rotor, albeit at the expense of some increase in parasitic and induced drag.

The interference between the main rotor, the tail rotor, and the vertical fin should be addressed even in an initial analysis. These effects may be accounted for by an increase in the induced power factor, $\kappa$ , to take into account the generally higher nonuniform inflow at the tail rotor location. Although the tail rotor power consumption is relatively low, interference effects may increase the power required by up to 20%, depending on the tail rotor and fin configuration. Because of the relatively low amount of power consumed by the tail rotor, the power required for first performance estimates can be expressed as a fraction of the total main rotor power, with a reasonable estimate of 5%.

Total Power

In light of the preceding, the total power coefficient for the helicopter in forward flight can be written in the form

(143) $\begin{equation*} C_P \equiv C_Q = \frac{\kappa C_T^2}{2 \sqrt{\lambda^2 + \mu^2}} + \frac{\sigma C_{d_{0}}}{8} ( 1 + K \mu^2 ) + \frac{1}{2} \left( \frac{f}{A} \right) \mu^3 + C_{P_{\hbox{\scriptsize\it TR}}} \end{equation*}$

The tail rotor power must always be added to obtain a proper estimate of total helicopter power requirements. For larger values of $\mu$ , then $\lambda \ll \mu$ , so that Glauert’s formula allows Eq. 157 to be simplified to

(144) $\begin{equation*} C_P \equiv C_Q = \frac{\kappa C_T^2}{2 \mu} + \frac{\sigma C_{d_{0}}}{8} ( 1 + K \mu^2 ) + \frac{1}{2} \left( \frac{f}{A} \right) \mu^3 + C_{P_{\hbox{\scriptsize\it TR}}} \end{equation*}$

Comparison with In-Flight Measurements

The figure below shows the representative results of net power required for an exemplary helicopter in straight-and-level flight. A gross weight of 16,000 lb (7,256 kg) and an operating altitude of 5,200 ft (1,585 m) have been assumed. The rotor disk angle of attack was calculated at each airspeed to satisfy the horizontal force equilibrium, which, although not a complete trim calculation, provides reasonably acceptable results. The predicted components of the total rotor power are also shown, including that of the tail rotor. The helicopter’s equivalent flat-plate area, ${\scriptstyle{f}}$ , is 23.0 ft ${^{2}}$ (2.137 m ${^{2}}$ ). For both the main and tail rotors, it is assumed that $\kappa = 1.15$ and $C_{d_{0}} = 0.008$ . The distance between the main and tail rotor shafts, $x_{\hbox{\scriptsize\it TR}}$ , is 32.5 ft (9.9 m).

Predicted power curve for a helicopter versus flight test measurements. The breakdown of the constituent parts of the power is also shown.

Notice that the induced and propulsive part of the power initially decreases with increasing airspeed but increases again as the disk is progressively tilted forward to meet more significant propulsion requirements. It is insufficient to assume induced losses are only a result of lift generation, so induced losses decrease rapidly with airspeed to a point and then increase again from losses associated with propulsive forces.

The power required for forward flight increases quickly at higher airspeeds because the parasitic losses are proportional to $\mu^3$ . The power growth rate is even higher when reverse flow and compressibility losses on the rotor are considered. However, the airframe drag significantly contributes to the total power required in high-speed flight. In design practice, much can be done to expand the flight envelope by striving for a more streamlined airframe.

Reverse Flow Region

In forward flight, a region of the rotor disk is on the retreating side called the “Reverse Flow Region.” In this region, the flow over the rotor blade section comes from tail to nose rather than nose to tail, as shown in the figure below. Because the flow in this region is “reversed” with the sharp trailing edge pointed into the airflow, the lift is negative, and the contribution of the profile drag on the blade section to the overall rotor torque and power required will be somewhat higher. The effects can usually be neglected at lower airspeeds.

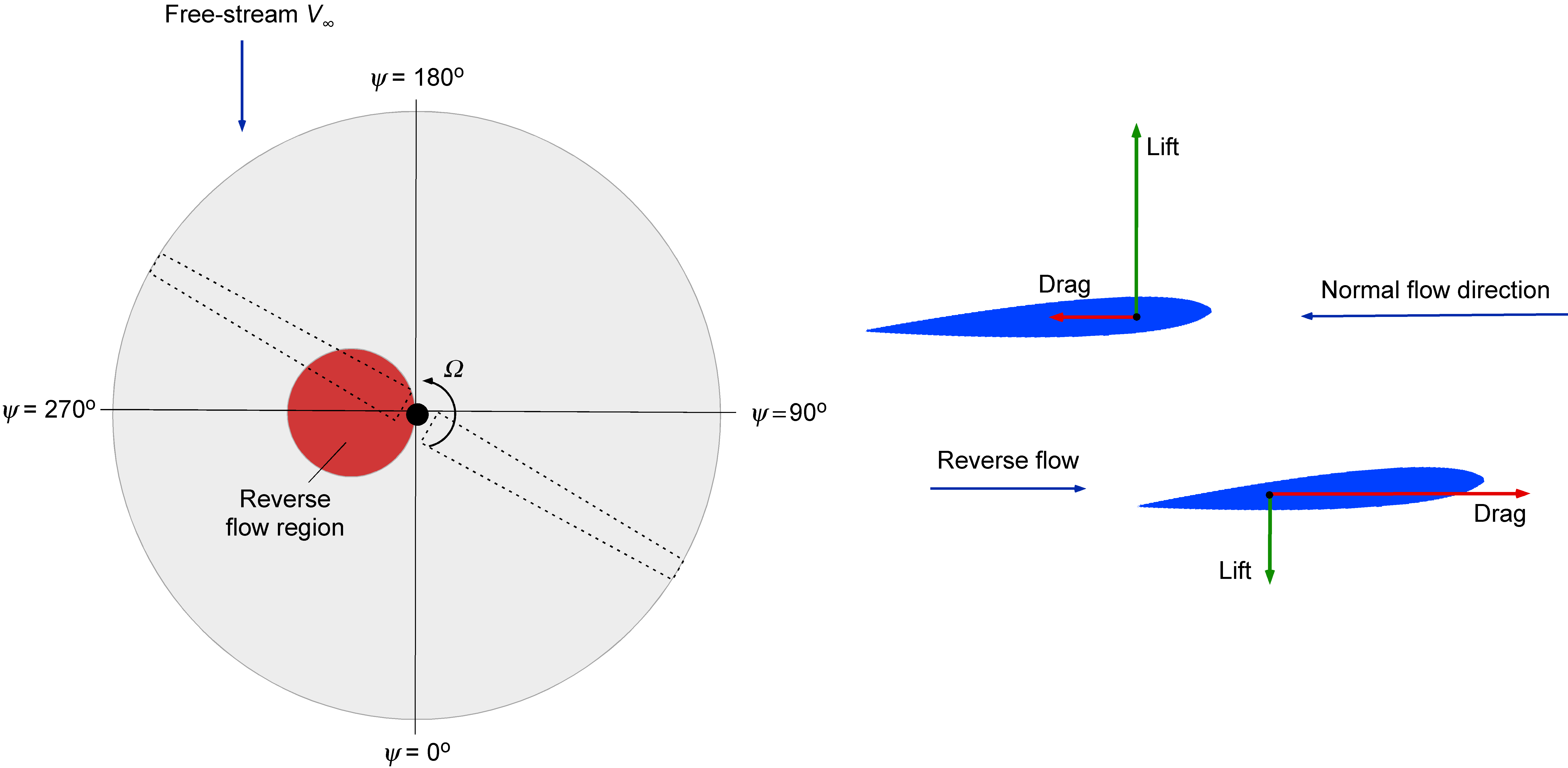
The geometry of this reverse flow region can be calculated using Eq. 2 with $V \le 0$ , i.e.,

(145) $\begin{equation*} V (y, V_{\infty}) =\Omega y + V_{\infty} \sin \psi \le 0 \end{equation*}$

This means that

(146) $\begin{equation*} \Omega y \le V_{\infty} \sin \psi \end{equation*}$

and for the boundary of the reverse flow region, then

(147) $\begin{equation*} y =\frac{V_{\infty}}{\Omega} \sin \psi \quad \mbox{or} \quad r = \mu \sin \psi \end{equation*}$

with $r = y/R$ , which is the equation of a circle in polar coordinates with a center located at $(r, \psi) = (\mu/2, 3 \pi/2)$ .

Effects of Weight

Representative results showing the effect of the helicopter’s weight on the power required are shown below for the exemplar helicopter at mean sea-level (MSL) conditions. Notice that higher flight weights require more power. In this case, the power available at MSL is 2,800 hp, and for a turboshaft engine, this stays relatively constant with airspeed.

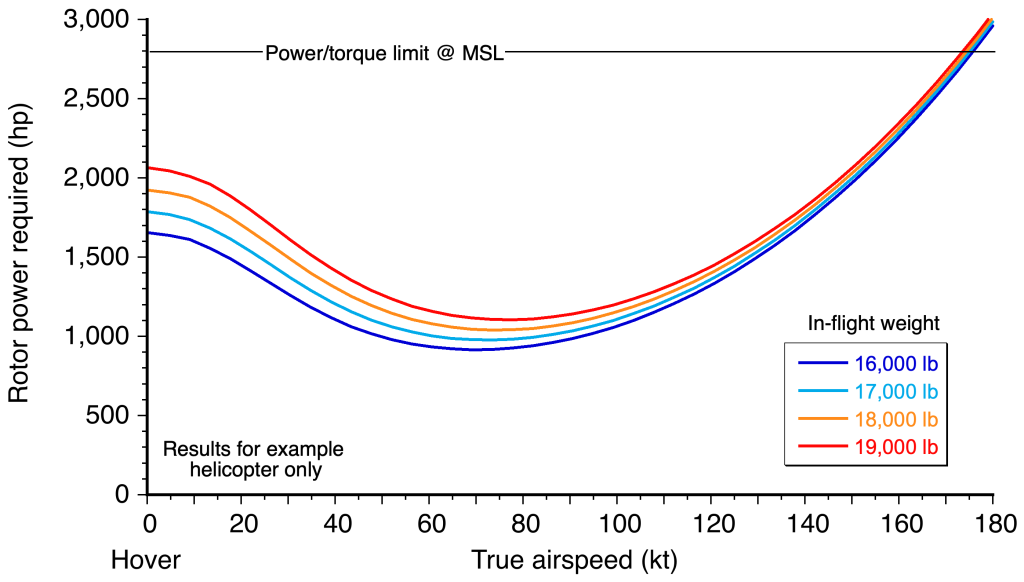
Predictions of power required in forward flight at different flight weights.

The airspeed obtained at the intersection of the power required curve with the power or torque available gives the maximum flight speed. Most helicopters powered by turboshaft engines may be torque-limited, which is a gearbox and transmission strength limitation rather than being limited by the shaft power from the engine. However, the maximum airspeed may also be determined by the onset of rotor stall and compressibility effects before reaching this point.

Effects of Density Altitude

The effect of altitude on performance is a crucial operational consideration for any airplane. As shown in the figure below, increasing density altitude increases the power required in hover and at lower airspeeds. Lower air density results in a lower power requirement because of the reduction of parasitic drag at higher airspeeds and higher altitudes.

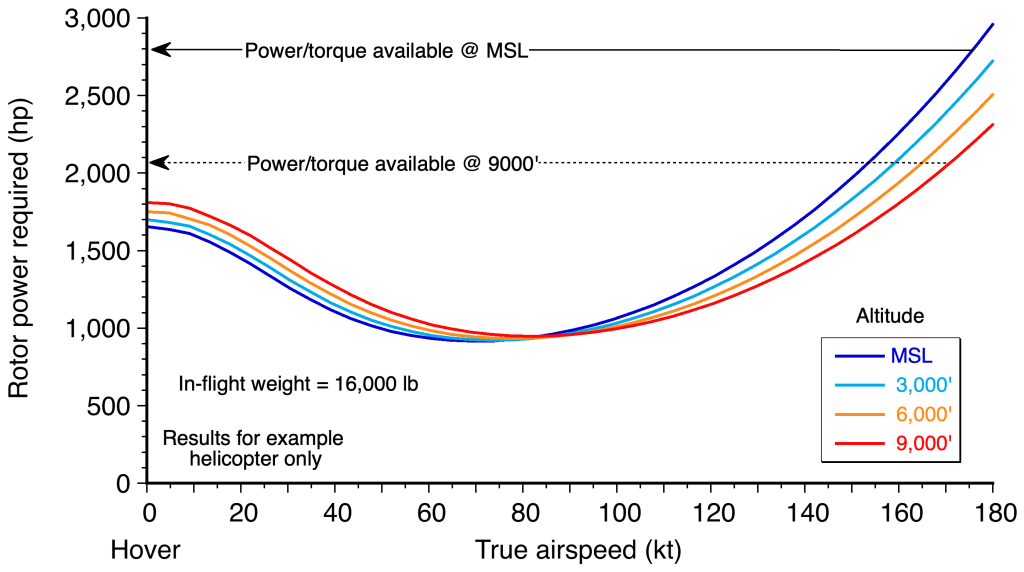
Predictions of power required in forward flight at different density altitudes.

However, a higher density altitude will also affect the engine power available. At 9,000 ft, the power available is about 25% less than that available at sea-level conditions, resulting in a significant decrease in the excess power available at any airspeed relative to that at sea-level conditions. Again, remember that helicopters powered by turboshaft engines may be torque-limited, which is a gearbox and transmission strength limitation rather than being limited by the shaft power from the engine.

Climb Performance

The general power equation can be used to estimate the climb velocity, $V_c$ , that is possible at any given airspeed based on the excess power available, i.e.,

(148) $\begin{equation*} W \, V_c = P_{\rm avail} - \left( P_0 + P_p + \displaystyle{ \frac{\kappa T^2}{2 \varrho \, A \, V_{\infty}}} \right) \end{equation*}$

It is realistic to assume that for low rates of climb or descent, the rotor-induced power, ${P_i}$ , the profile power, ${P_0}$ , and the airframe drag, $D$ , remain nominally constant so

(149) $\begin{equation*} { V_c = \frac{P_{\rm avail} - P_{\rm level}}{T} = \frac{\Delta P}{T} = \frac{\Delta P}{W} } \end{equation*}$

Notice that $P_{\rm{level}}$ is simply the net power required to maintain level flight conditions at the same forward speed. If the installed power available is $P_{\rm avail}$ (which may vary with flight condition), then it will be seen that the power available to climb varies with forward flight speed. The climb velocity can then be obtained from

(150) $\begin{equation*} V_c = \frac{P_{\rm{avail}} -(P_i + P_0 + P_p )}{T} = \frac{\Delta P}{W} \end{equation*}$

where $\Delta P$ is the excess power available at that combination of airspeed and altitude.

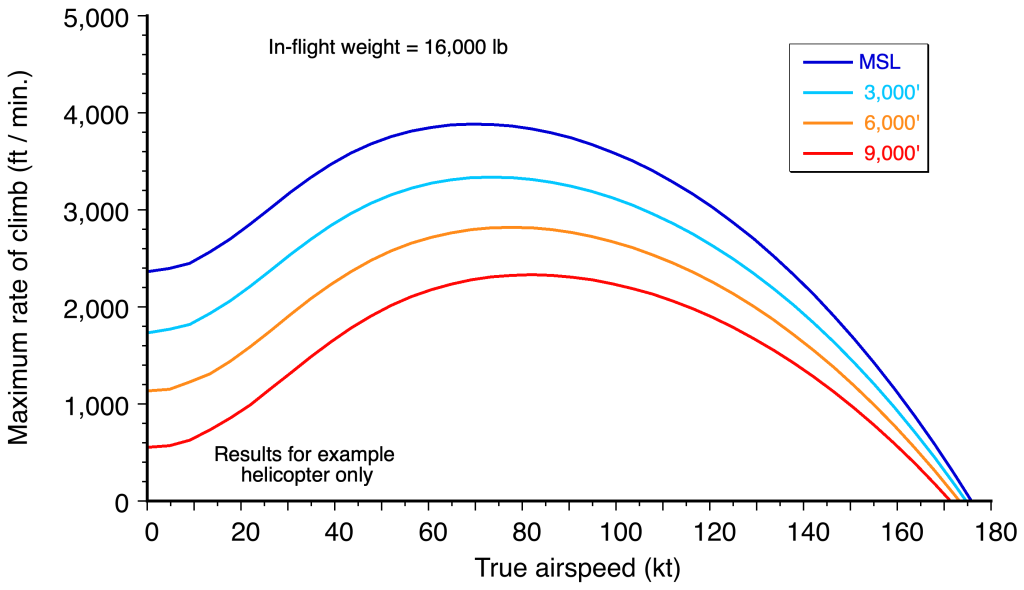
Example of the rate of climb versus airspeed for the exemplar helicopter.

Calculations of the maximum rate of climb as a function of flight speed and density altitude are shown in the figure below for the exemplary helicopter. Notice that climb performance is substantially affected by density altitude. These curves will mimic the excess power available because the climb (or descent) velocity is determined simply by the excess (or decrease) in power required, $\Delta P$ , relative to steady-level flight conditions. Notice that the rate of climb improves markedly when translating from hover into forward flight. Pilots often call the tendency of the helicopter to climb when accelerating from hover by the name “translational lift.” However, the term is a misnomer because the helicopter climbs by the excess power available and not the extra rotor lift.

Check Your Understanding #6 – Power required in forward flight

A helicopter is operating in level forward flight at a true airspeed of 210 ft/s under the following conditions: shaft power supplied = 655 hp, $W$ = 6,000 lb, $\varrho$ = 0.00200 slugs/ft ${^{3}}$ . The rotor parameters are $R$ = 19 ft, $\sigma$ = 0.08, $\Omega R$ = 700 ft/s, $\kappa = 1.15$ , and $C_{d_{0}}$ = 0.01. Neglect the effects of reverse flow, stall, and compressibility. (i) How much power is required to overcome induced losses? (ii) How much power is required to overcome profile losses? (iii) What is the equivalent flat-plate area, ${\scriptstyle{f}}$ ? (iv) If the installed power is 800 hp, estimate the maximum rate of climb possible at this airspeed.

Show solution/hide solution.

(i) The induced power of the rotor, ${P_i}$ , can be approximated by

$P_i = \kappa T v_i$

If the forward velocity is above $\mu>0.1$ ( $\mu = 210.0/700.0 = 0.3$ in this case), then Glauert’s “high-speed” formula can approximate the induced velocity. Therefore, the induced power required does not need a solution to the inflow equation and can be determined from

$P_i = \kappa T \left(\frac{T}{2 \varrho \, A \, V_{\infty}} \right) = \frac{\kappa W^2}{2 \varrho \, A \, V_{\infty}}$

Substituting the appropriate parameters gives the induced power at this operating condition as

$P_i = \frac{ 1.15 \times (6,000)^2}{2 \times 0.002 \times \pi \times (19)^2 \times 210.0} \times \frac{1}{550} = 79.0 \mbox{hp}$

(ii) The profile power coefficient can be estimated from

$C_{P_{0}} = \frac{\sigma C_{d_{0}}}{8} ( 1 + K \mu^2)$

where $K$ can be assumed to be 4.7; different values may be assumed for $K$ . Therefore, at this operating condition, the profile power is

$\begin{eqnarray*} P_0 & = & \frac{1}{8} \varrho \, A (\Omega R)^3 \sigma C_{d_{0}} \left( 1 + 4.7 \mu^2 \right) \nonumber \\ & = & \left( \frac{1}{8} \right) \times 0.002 \times \pi \times 19^2 \times 700^3 \times 0.08 \times 0.01 \times \nonumber \\ & & \hspace*{30mm}\left( 1 + 4.7 \times 0.3^2 \right) \times \frac{1}{550} \nonumber \\ & = & 201.3~\mbox{hp} \end{eqnarray*}$

(iii) The total power in forward flight is the sum of induced, profile, and parasitic power, i.e.,

$P = P_i + P_0 + P_p$

Knowing the induced, parasitic, and total shaft power supplied means the parasitic power will be ${P_p}$ = 655 – 79.0 – 201.3 = 374.7 hp. The parasitic power coefficient can be written as

$C_{p_{p}} = \frac{1}{2} \left( \frac{f}{A} \right) \mu^3$

where ${\scriptstyle{f}}$ is the equivalent parasitic drag area. In dimensional units, the parasitic power is

$P_p = \frac{1}{2} \varrho \, f \, V_{\infty}^3 = 0.5 \times 0.002 \, f \, 210^3 = 374.7 \times 550$

Solving for ${\scriptstyle{f}}$ gives

$f = \frac{2 \times 374.7 \times 550}{0.002 \times 210^3} = 22.25~\mbox{ft${^{2}}$}$

(iv) The maximum rate-of-climb, $V_c$ , is determined by the excess power available over and above that required from a straight-and-level flight at the same airspeed. In this case

$V_c = \frac{\Delta P}{W} = \frac{ (800 - 655 ) \times 550}{6,000} = 13.26~\mbox{ft/s}$

Conventionally, in aviation, this result would be expressed in terms of feet-per-minute, which gives $V_c = 13.26 \times 60 =$ 798 ft/min.

Fuel Flows

For many aircraft performance problems, such as the calculation of range and endurance, a knowledge of the engine’s fuel burn (s) is required. From the power required curves for the helicopter, the fuel consumption can be estimated for any given type of engine, e.g., piston engine or turboshaft engine. Engine performance characteristics are usually expressed in terms of brake-specific fuel consumption or BSFC (in units of lb hp $^{-1}$ hr $^{-1}$ or kg kW $^{-1}$ hr $^{-1}$ ) versus shaft power (in units of hp or bhp or kW). These curves are a function of atmospheric conditions, so a series of curves is required for different altitudes and operating temperatures. Fuel flow curves must be derived versus indicated airspeed and gross weight.

For a normally-aspirated (non-supercharged) piston (reciprocating) engine, the power curves vary almost linearly with density ratio, $\sigma$ . One common approximation is

(151) $\begin{equation*} P_{\rm alt} = P_{\rm MSL} \left( 1.133 \sigma - 0.133 \right) \end{equation*}$

where $P_{\rm alt}$ is the power available at altitude and $P_{\rm MSL}$ is the power available at mean sea level conditions. The value of the density ratio $\sigma$ can be found from the ISA model depending on the pressure altitude and outside air temperature.

For a turboshaft engine, a good approximation is

(152) $\begin{equation*} P_{\rm alt} = P_{\rm MSL} \left( \frac{\delta}{\theta} \right) \end{equation*}$

where $\delta$ is the pressure ratio at that altitude and $\theta$ is the corresponding absolute temperature ratio, which can be found from the ISA model.

Because helicopters will operate with the engines operating close to their rated power for much of the flight, a first-level approximation is to assume that the BSFC remains independent of power output. In this regard, the fuel flow rate $\overbigdot{W_f}$ is given by

(153) $\begin{equation*} \overbigdot{W_f} = \frac{W_f}{dt} = {\rm BSFC} \, P_{\rm alt} \end{equation*}$

where $P_{\rm alt}$ is the total power required for the helicopter at altitude. Generally, fuel flow curves versus airspeed (at a given density altitude) are fairly flat. Therefore, for most helicopters, the fuel flow curves will mimic the shapes of the power-required curves. In this regard, a representative fuel flow curve for a helicopter is shown in the figure below. Notice that the best flight endurance will occur at the airspeed for the lowest fuel burn rate. The best range will occur at the airspeed for the lowest fuel burn per unit distance or airspeed, obtained at the tangent point, as shown.

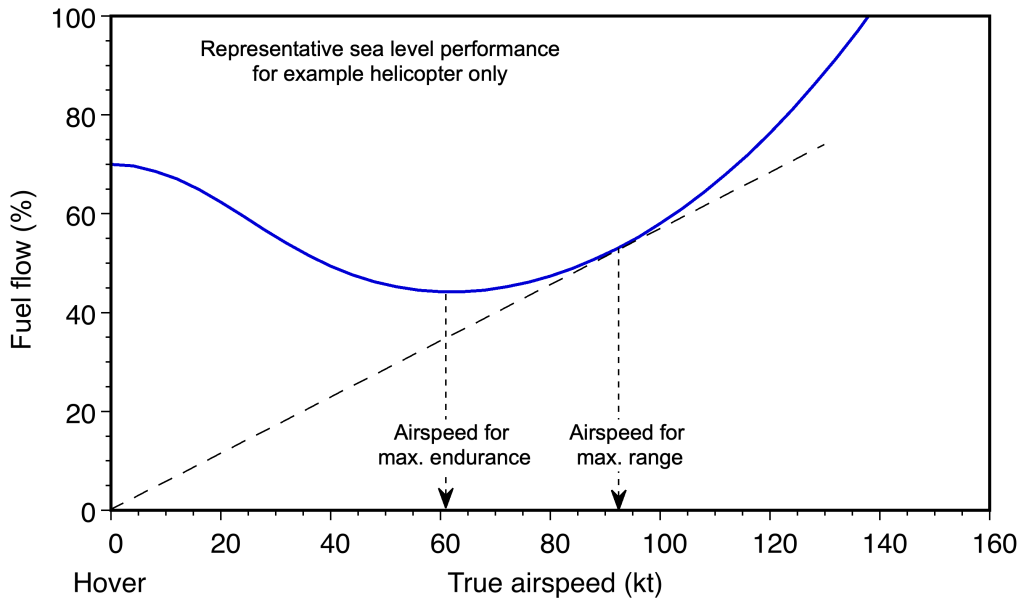
Range & Endurance

The fuel flow curves provide the information to determine range/payload and endurance/payload charts. These charts provide critical information on the effects of aircraft range and endurance, especially when trading off payload for fuel, and will be used by pilots for flight planning. Helicopters have a relatively low useful load capability compared to airplanes, so the payload trade with fuel is usually severe. Specific mission profiles for any aircraft must be defined when calculating flight endurance and range, although most missions will involve a flight from point A to point B. Engine characteristics must be considered to determine the maximum endurance and range, i.e., its BSFC characteristics.

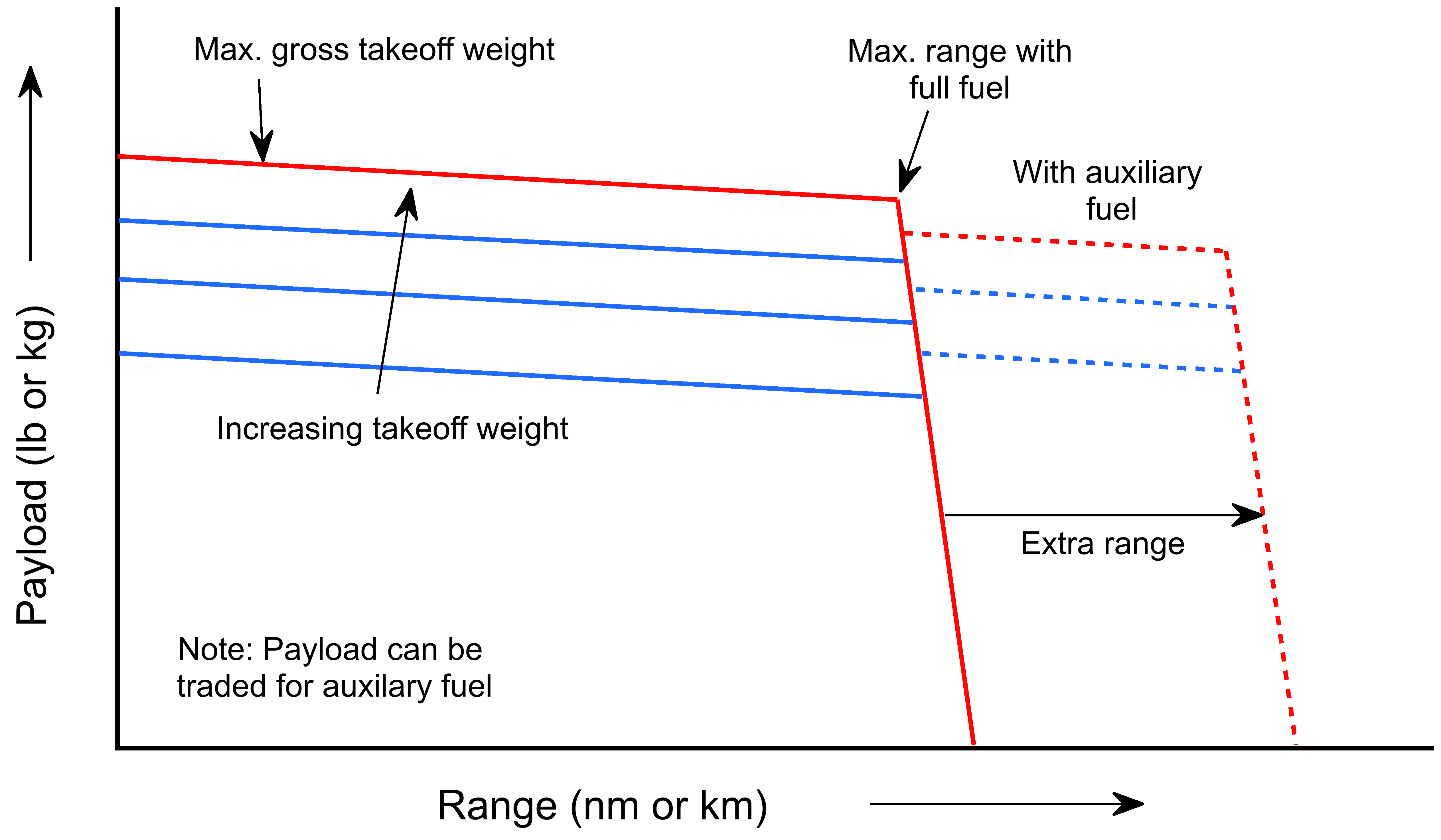
A representative payload-range chart for a helicopter. The range can be extended with auxiliary fuel tanks but with a reduced payload.

The fuel burn rate, $W_F$ , with respect to distance, $R$ , will be

(154) $\begin{equation*} \frac{d W_F}{d R} = \frac{P \times (\rm BSFC)}{V_{\infty}} \end{equation*}$

where BSFC is the specific fuel consumption of the engine(s). The power required, $P$ , varies with gross weight and density altitude, and as already shown, the BSFC itself depends on power and density altitude. Because the weight decreases as fuel is burned, Eq. 154 must be integrated numerically to find the range. Fuel burned during takeoff, climb, and descent is factored into the calculation, along with a mandated fuel reserve in minutes of flying time. Because the fuel weight on a helicopter is normally a small fraction of the total gross weight, the fuel burn rate can be evaluated fairly accurately at the point in the cruise where the aircraft weight is equal to the initial gross weight (gross takeoff weight $W_{\rm GTOW}$ ) is less half the initial fuel weight, where $W_F$ is the initial fuel weight. In this case, the range, $R$ , of the helicopter is given by

(155) $\begin{equation*} R = W_F \left[ \frac{V_{\infty}}{P \times \rm {BSFC}} \right]_{W=W'} \end{equation*}$

less an allowance for the other contingency factors described previously.

By a similar process, the estimated endurance, $E$ , will be given by

(156) $\begin{equation*} E = W_F \left[ \frac{1}{P \times {\rm BSFC} } \right]_{W=W'} \end{equation*}$

Generally, estimating endurance by dividing the usable fuel onboard by the average fuel flow rate is sufficiently accurate.

Coaxial Rotor in Forward Flight

As previously explained, counter-rotating coaxial rotors incur an additional induced power when the lower rotor operates in the wake of the upper rotor. This unavoidable loss occurs irrespective of whether the two rotors operate at the same thrusts or torques. In this case, the total power equation in forward flight can be written as

(157) $\begin{equation*} C_P = \frac{\kappa_{\rm int} \, \kappa C_T^2}{2 \sqrt{\lambda^2 + \mu^2}} + \frac{\sigma C_{d_{0}}}{8} ( 1 + K \mu^2 ) + \frac{1}{2} \left( \frac{f}{A} \right) \mu^3 \end{equation*}$

where $\kappa_{\rm int}$ is the induced power factor that accounts for rotor-on-rotor interference. The inflow for two closely spaced coaxial rotors, which share the same inflow, can be calculated at the total thrust of the system, as in the manner performance of the single rotor using the inflow equation. In this case, using $\kappa_{\rm int}$ = 1.28 would be appropriate.

Measurements of coaxial rotor performance in forward flight are rare. However, the Harrington 1 coaxial rotor, as described previously, was tested in a NACA wind tunnel by Dingledein. The results in the figure below show a comparison of the momentum theory with the measured levels of rotor performance. Notice that the theory generally slightly overpredicts the power requirements for the single rotor and underpredicts the power required for the coaxial rotor system. Such performance trends have also been shown using other predictive methods.

It would appear that any differences in the measured and predicted power values with the coaxial rotor are at least partly associated with uncertainties in the way the rotor was trimmed (using collective and cyclic pitch). These uncertainties are combined with some uncertainty in determining the equivalent parasitic drag area, which determined the rotor shaft angle in the wind tunnel and, thus, the equivalent parasitic component of power required to overcome drag. To this end, notable uncertainties are apparent in the measurements.

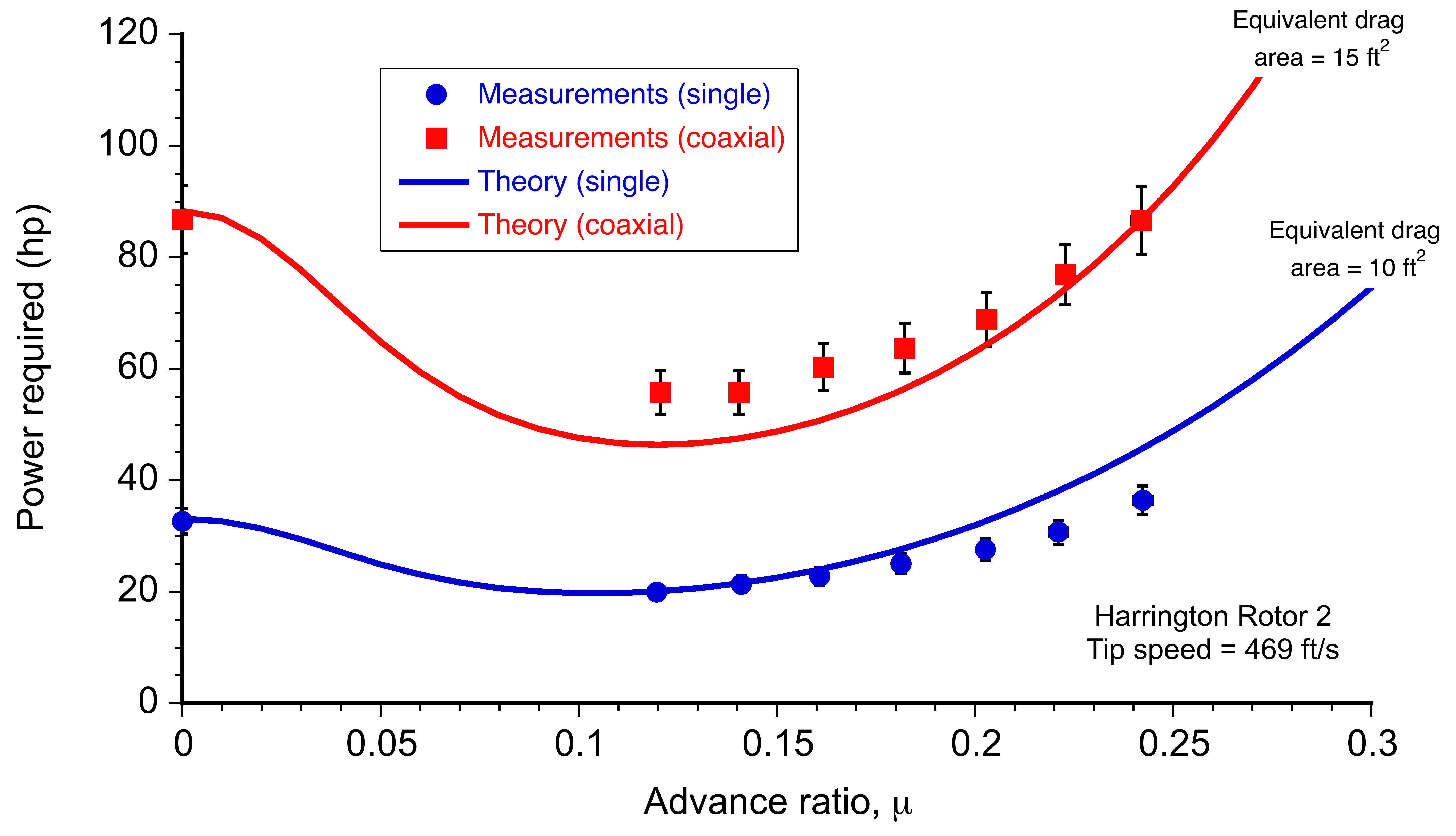
Measurements and predictions of coaxial rotor performance in forward flight.

While it is clear that the agreement between the theory and the measurements for a coaxial is not perfect, it would seem that the momentum theory can give reasonable predictions of coaxial rotor performance, at least preliminary design in both hover and forward flight. It should be remembered, however, that there will always be some uncertainties in the predicted performance of any rotorcraft that uses a coaxial rotor system, especially when actual flight test data are unavailable for validation. As flight test results are obtained, it will be possible to retroactively refine the predictions of rotor loads and aircraft performance (including range, endurance, etc.) by including empirical refinements to the various aerodynamic theories (e.g., providing better estimates of the profile drag coefficients, equivalent parasitic drag area, etc.). In particular, the accurate determination of the parasitic drag of the aircraft will be critical for estimating performance.

Controlling the Helicopter

One of the greatest mysteries to most people about the helicopter is how it is controlled during flight. In the case of an airplane, it is much more intuitive because control is obtained by using easily identified aerodynamic surfaces, such as ailerons, elevators, and rudder, which are deflected to give the appropriate forces and moments on the airplane during its flight. In the case of a helicopter, control is obtained by using forces and moments produced by the rotor, which are obtained by tilting the orientation of the rotor disk. The question now is how this is done.

Main Rotor

First, it can be shown that the dynamic natural frequency of a rotor blade in its flapping motion (up and down) about the flapping hinge is equal to the rotational frequency, $\Omega$ . While this result is strictly valid for a blade with a hinge at the rotational axis, it is close enough for any practical rotor and does not change the physics of the following discussion.

The critical issue is understanding that if any first-order dynamic system is excited by a forcing function at its natural frequency, it will respond at the natural frequency but with a 90 $^{\circ}$ phase lag between the forcing and the response. For example, in the case of a rotor blade, if its lift (the forcing) is increased, then the blade response (the flapping angle) does not change instantaneously but will increase over time and only reach its maximum flapping displacement 90 $^{\circ}$ later. This 90 $^{\circ}$ lag between changes in blade pitch (lift) and the flapping response (displacement) is the key to understanding how to control the helicopter.

Some mechanism is required to change the blade pitch angles to change the blade lift. Most often, this is done using a swashplate, an example used on an actual helicopter being shown in the photograph below. A swashplate consists of two plates, the upper one rotating with the rotor and the lower one fixed and connected to the pilot’s controls. The upper swashplate is connected to the blades using pitch links, so the movement of the swashplate (up and down and tilting) then translates into displacements of the pitch links, producing changes in the blade pitch angles. A set of bearings between the two disks allows the upper disk to rotate with the rotor while the lower is non-rotating. Both disks can be slid up and down the shaft in response to collective pitch inputs, and the swashplate can also be tilted to an arbitrary orientation in response to cyclic pitch inputs, both inputs originating from the pilot’s controls.

Photograph of a swashplate, which is used to impress a one-per-revolution change in blade pitch (and hence blade lift) to cause the blades to flap about the hinges and tilt the rotor disk’s orientation and corresponding thrust vector.

The inputs produced by the swashplate consist of the average or collective pitch $\theta_0$ and the lateral cyclic, $\theta_{1c}$ , and the longitudinal cyclic, $\theta_{1s}$ , i.e.,

(158) $\begin{equation*} \theta(\psi) = \theta_0 + \theta_{1c} \cos \psi + \theta_{1s} \sin \psi \end{equation*}$

The collective pitch, $\theta_0$ , controls the average pitch and the total rotor thrust. The cyclic pitch controls the orientation or tilt of the rotor disk and the direction of the rotor thrust vector. The blade pitch motion itself is induced through a pitch or feathering bearing. A pitch horn is attached to the blade outboard of the pitch bearing. A pitch link is connected to the pitch horn and the upper (rotating) part of the swashplate so that as the upper plate rotates, the vertical displacement of the pitch link produces blade pitch motion.

The novel part of the helicopter swashplate is its ability to tilt to an arbitrary orientation, which requires a gimbal or spherical bearing between the swashplate and the rotor shaft. This mechanism allows a first harmonic blade-pitch input with any phase angle. The upshot is that the rotor disk plane can be tilted to any orientation in space, the basic idea being shown in the schematic below.

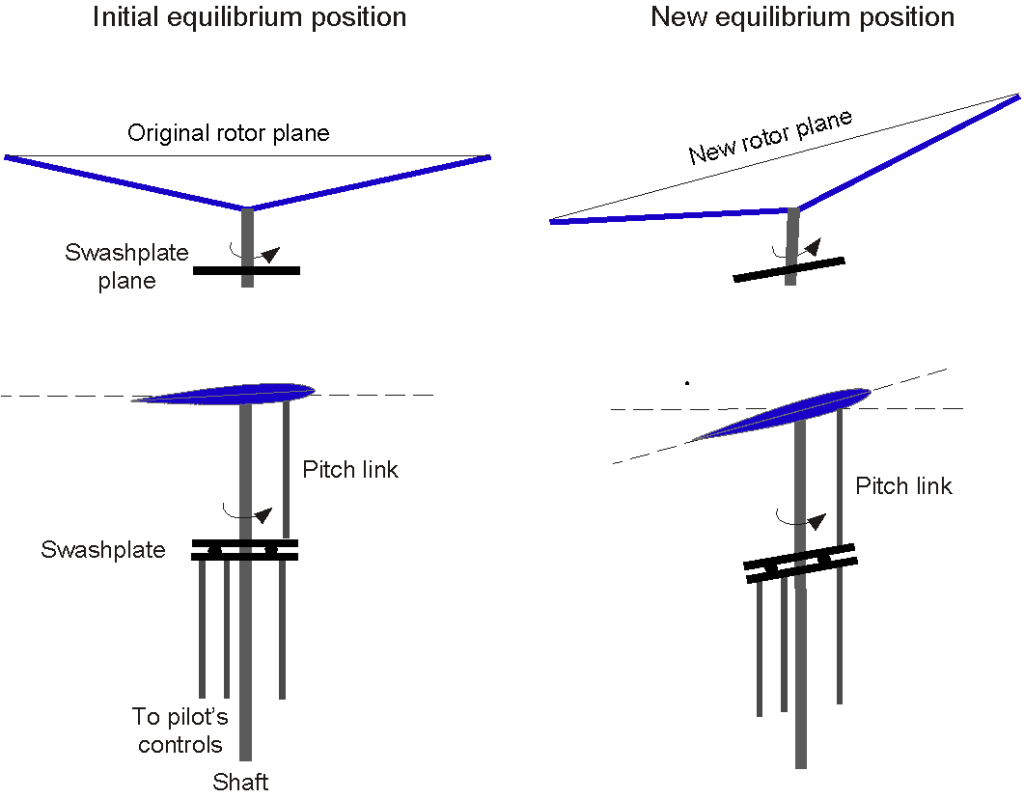
The swashplate allows a first harmonic blade-pitch input with any phase angle so the rotor disk plane can be tilted to any orientation.

The two figures below explain the relationship between blade pitch (and lift) and blade flapping displacements. Imposing pitch changes other than at once per revolution for flight control is unnecessary. Again, the blade pitch (or feathering) motion imposed by the swashplate is described by

(159) $\begin{equation*} \theta(\psi) = \theta_0 + \theta_{1c} \cos \psi + \theta_{1s} \sin \psi \end{equation*}$

remembering that the imposed (controlled) value of $\theta_0$ modulates the value of the rotor thrust.

The first harmonic blade of the blade flapping response is given by

(160) $\begin{equation*} \beta(\psi) = \beta_0 + \beta_{1c} \cos \psi + \beta_{1s} \sin \psi \end{equation*}$

The mean value, $\beta_0$ , is called the coning angle, which results from a balance of the moment of the blade lift and the centrifugal force about the flapping hinge.

For $\theta_{1c}$ and $\theta_{1s}$ inputs then the blade flapping response will be

(161) $\begin{eqnarray*} \beta ( \psi ) & = & \beta_0 + \theta_{1c} \sin \psi - \theta_{1s} \cos \psi \\ & = & \beta_0 + \theta_{1c} \cos \bigg( \psi - \frac{\pi}{2} \bigg) + \theta_{1s} \sin \left(\psi - \frac{\pi}{2} \right) \end{eqnarray*}$

Notice that because of the dynamic behavior of the blade, the flapping response lags the blade pitch (aerodynamic) inputs by $\pi/2$ or 90 $^{\circ}$ .

Therefore, if the swashplate produces a once-per-revolution aerodynamic forcing, it will cause the rotor blades to flap at once-per-revolution, and the rotor disk plane will then precess to a new orientation in space, thereby tilting the orientation of the thrust vector. The system is fundamentally straightforward; an aerodynamic forcing is applied at (or close) to the natural frequency of the flapping blade, and the blades then respond so that one unit of cyclic pitch input results in (almost) one unit of flapping response. This behavior is strictly for a rotor with a flapping hinge at the rotational axis, but even with a hinge offset, the underlying physics and rotor response are essentially similar.

The figure below illustrates more clearly what happens with a $\theta_{1s}$ blade pitch input (longitudinal cyclic) from the swashplate, which means that the blade lift will be phased around the rotor disk such that it will reach a maximum at $\psi = 90^{\circ}$ and a minimum at $\psi = 270^{\circ}$ . Because of the 90 $^{\circ}$ phase lag between the generation of lift and the blade flapping response, in this case, the blades will flap up and reach a maximum displacement at $\psi = 180^{\circ}$ and a minimum displacement at $\psi = 0^{\circ}$ , the net effect, being that the rotor disk plane will tilt backward.

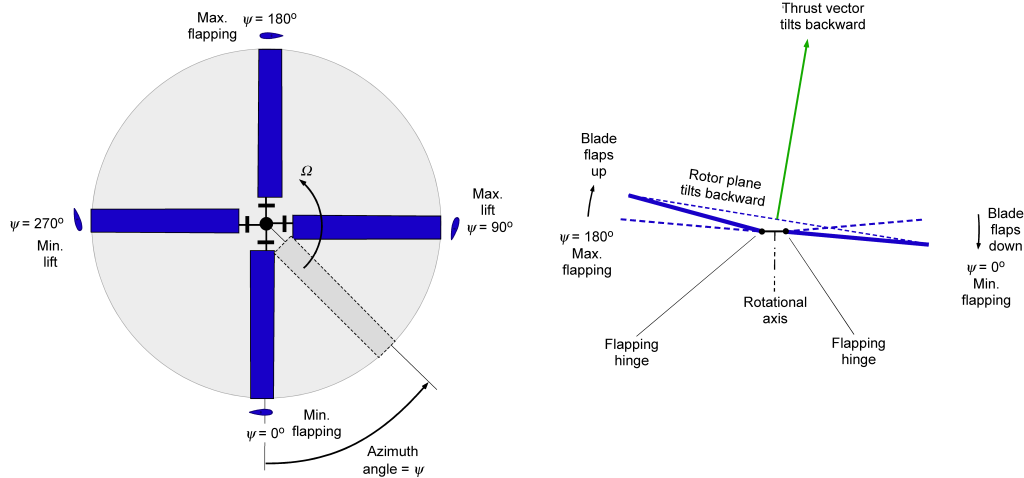
Positive longitudinal cyclic pitch produces a longitudinal flapping displacement, tilting the rotor disk backward.

The second figure, as shown below, illustrates what happens with a $\theta_{1c}$ blade pitch input (lateral cyclic) from the swashplate, which means that the blade lift will be phased around the rotor disk such that it will reach a maximum at $\psi = 0^{\circ}$ and a minimum at $\psi = 180^{\circ}$ . Again, because of the 90 $^{\circ}$ phase lag between the generation of lift and the blade flapping response, the blades, in this case, will flap up and reach a maximum displacement at $\psi = 90^{\circ}$ and a minimum displacement at $\psi = 270^{\circ}$ , the net effect is that the rotor disk plane will tilt to the left (to port) when viewed from behind. The mystery of how to control the helicopter is now solved!

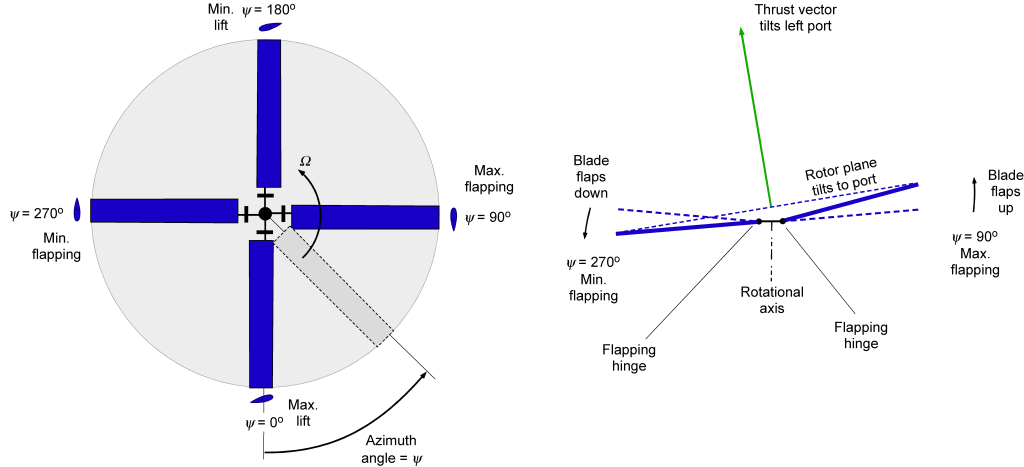
Positive lateral cyclic pitch produces a lateral flapping displacement, tilting the rotor disk to port.

Tail Rotors

The primary purpose of the tail rotor is to provide a sideward force on the airframe in a direction of sufficient magnitude to counter the main rotor torque reaction. If the distance from the main rotor shaft to the tail rotor shaft is $x_{\hbox{\scriptsize\it TR}}$ , the tail rotor thrust required will be

(162) $\begin{equation*} T_{\hbox{\scriptsize\it TR}} = \frac{(P_i + P_0 + P_p)}{\Omega~x_{\hbox{\scriptsize\it TR}}} \end{equation*}$

where $\Omega$ is the angular velocity of the main rotor. This latter equation assumes no interference or off-loading of the tail rotor by the fin in hover or forward flight.

The second purpose of the tail rotor is to provide directional (yaw) control by modulating its thrust. The pilot’s feet control the thrust by pushing on a set of floor-mounted pedals, similar to the rudder pedals on an airplane. For example, if the rotor turns in the conventional direction (counterclockwise when viewed from above), pushing on the left pedal increases the tail rotor thrust (positive to starboard), and the helicopter will yaw nose-left about its center of gravity.

As for the main rotor, the power required to drive the tail rotor depends on the disk loading. A larger diameter may be preferable for low-induced power requirements, but several factors outweigh this choice. First, a larger diameter usually means a heavier design, which is undesirable because of its adverse effects on the helicopter’s center of gravity location. Second, to meet certification requirements, the tail rotor disk loading and induced velocities are usually desirable to be high enough for sideward flight without the tail rotor entering the vortex ring state (VRS). These constraints dictate using a relatively small tail rotor with a high disk loading.

Types of Tail Rotors

Tail rotors may be either of the pusher or tractor variety, as shown in the figure below, and can be located on the left or right-hand side of the vertical fin. All designs suffer from interference effects between the rotor and the fin, more or less, these effects being a function of the tail rotor size or disk area, fin area, and spacing of the tail rotor plane from the fin. Canted fins attempt to minimize tail rotor/fin aerodynamic interference. Canted tail rotors can produce an element of vertical thrust and pitching moment on the fuselage, widening the helicopter’s allowable center of gravity envelope.

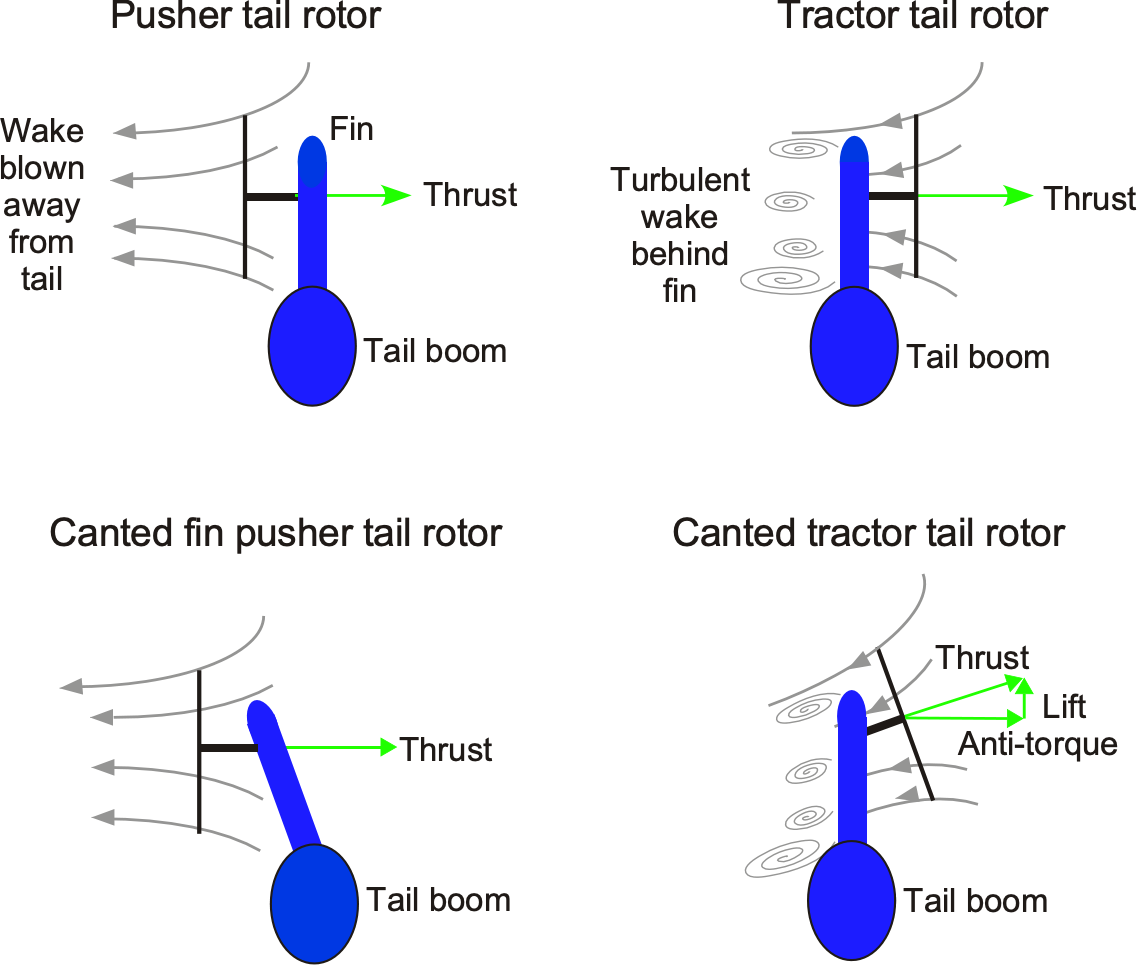
In contrast, the tractor-tail rotor design has a vertical fin inside the high-energy region from the tail rotor wake. While this “blockage” effect tends to increase the tail rotor thrust, a significant force is also applied to the vertical tail that is in the opposite direction to the anti-torque thrust requirement. It is found, however, that the net effect is a decrease in thrust compared to what would be obtained if the rotor were operating in isolation. The interference effects become more significant in both cases with larger fins and smaller rotors. As a result, most modern helicopters use a pusher tail rotor design because this configuration tends to have a higher overall anti-torque-producing efficiency.

Other Effects

A secondary effect of the anti-torque side force is the tendency for the helicopter to drift sideways. This effect is corrected by the main rotor, which is tilted slightly to the left (using cyclic pitch inputs) so that a component of the main rotor thrust produces an equal and opposite side force. This behavior is why it will be noticed that a helicopter will tend to hover with one wheel (or skid) lower than the other. On larger helicopters, the main rotor shaft is physically tilted slightly (as part of the design, thereby introducing a pretilt) so that the pilot does not require as much cyclic pitch input to counter the tail rotor side force. The tail rotor thrust and the main rotor side force component act together, producing a couple and a rolling moment about the center of gravity (c.g.). To reduce this moment, the tail rotor is located vertically up on the tail structure so that the line of action of its thrust vector is close to the helicopter’s center of gravity.

The tail rotor must also provide the specified yaw acceleration in the maximum specified crosswind conditions, considering possible losses in efficiency because of aerodynamic interference effects between the tail rotor and the vertical fin. Furthermore, when the main rotor thrust or power is increased, for example, to climb, the reaction torque on the fuselage is increased. This effect means the tail rotor thrust must also increase to balance this torque reaction. Therefore, when the pilot raises the collective pitch to climb, foot pressure must be applied to the appropriate pedal to keep the nose pointed straight in the desired direction of flight.

Design Features

Tail rotors typically have two or four blades, with no particular aerodynamic advantage of one number over the other. Only collective pitch is required because there is no need to control the orientation of the tail rotor disk plane. Tail rotor blades may have some built-in twist to help minimize induced power requirements. Although some blade designs may use cambered airfoil sections, the tail rotor blades on many helicopters use symmetric airfoils because of their excellent overall performance and low-pitching moments. Generally, tail rotors are designed to operate at tip speeds comparable to the main rotor’s. Lower tip speeds are desirable to minimize noise. However, tail rotors operating at lower tip speeds require higher solidity to prevent blade stall for a given thrust. A lower tip speed also increases the torque requirement. Both of these factors will increase the weight of the drive system.

Common amongst all tail rotors is the lack of any cyclic pitch; only collective pitch is used because control of the tail rotor disk orientation is not required. Nevertheless, the tail rotor must be provided with flapping hinges so that the blades can respond to the changing aerodynamic environment. Lead/lag hinges are not usually used to save weight and reduce mechanical complexity. Instead, pitch/flap coupling is built into the tail rotor design, which can be discerned from the photograph below. In this case, the coupling is introduced by locating the pitch horn 45 $^{\circ}$ in front of the blade feathering axis. This approach provides a means of allowing the blades to pitch cyclically in such a way as to minimize blade flapping produced by the changing aerodynamic loads in forward flight.

Pitch/flap coupling can be introduced into the tail rotor design so that blade flapping changes the blade pitch and minimizes the flapping.

Autorotation

All aircraft must land safely in the event of a complete power failure; this is a certification requirement for civil aircraft and an acceptance criterion for military aircraft. Helicopters are no exception. Loss of power in a helicopter may occur because of a mechanical problem with the main rotor or gearbox, tail rotor failure, other related tail rotor drive issues, or even fuel starvation (yes, it does happen). Autorotation is a high-performance maneuver used to land a helicopter in the event of power failure, i.e., the helicopter establishes a flight condition equivalent to gliding in an airplane. Unfortunately, a helicopter is not a good glider, partly because of its relatively low lift-to-drag ratio. However, a well-trained helicopter pilot should be able to conduct a safe autorotational landing after losing power.

Autorotation is a rapidly descending flight condition during which the upward flow through the rotor produces in-plane aerodynamic forces that create a net torque to drive the rotor, i.e., the rotor self-rotates because it absorbs power from the relative airstream rather than requiring power delivered at the shaft from the engine, as shown in the figure below. Underlying this behavior is the principle of conservation of energy. Because of the upward flow through the rotor, the lift vectors at some points over the blades are tilted forward rather than backward, driving the rotor, the upshot being a zero-torque descending flight condition.

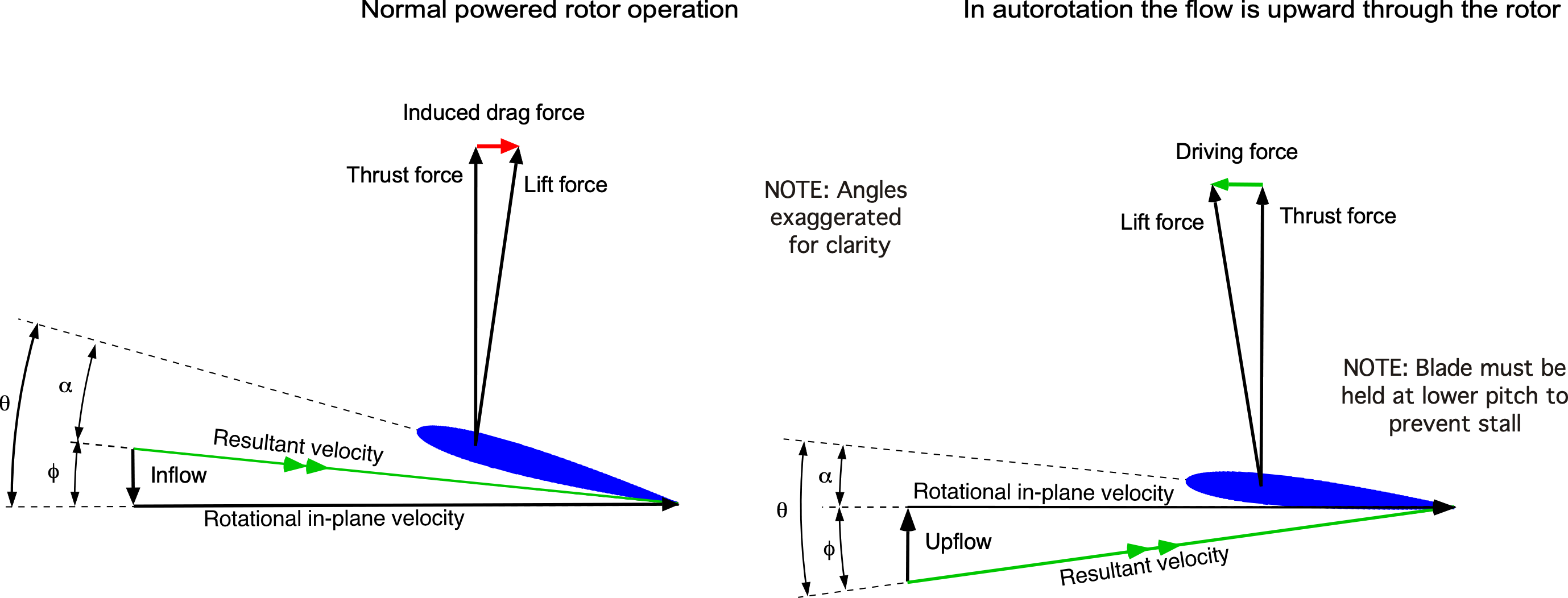
In an autorotation, the upward flow velocity through the rotor disk causes some parts to provide a driving force, creating a torque that maintains the rotor’s speed.

Energy Analysis

Under established, steady autorotative conditions at a constant airspeed, there is an energy balance where the decrease in aircraft potential energy per unit time equals the power required to sustain the rotor speed to produce thrust and control. In other words, the pilot gives up altitude at a controlled rate in return for any available energy to continue turning the rotor and keep it producing thrust.

In general, the instantaneous energy state of the helicopter, $E$ , can be written as the sum of its potential energy (altitude), $E_h$ , translational kinetic energy (airspeed), $E_V$ , and stored rotor energy (rotor rotational speed), $E_R$ , i.e.,

(163) $\begin{equation*} E = E_h + E_V + E_R = W \, h + \frac{1}{2} \left( \frac{W}{g} \right) V_{\infty}^2 + \frac{1}{2} I_R \, \Omega^2 \end{equation*}$

where $W$ is the weight of the helicopter, ${h}$ is the height above ground level (AGL), $V_{\infty}$ is the true airspeed, $I_R$ is the rotor’s polar moment of inertia, and $\Omega$ is the rotor’s angular velocity.

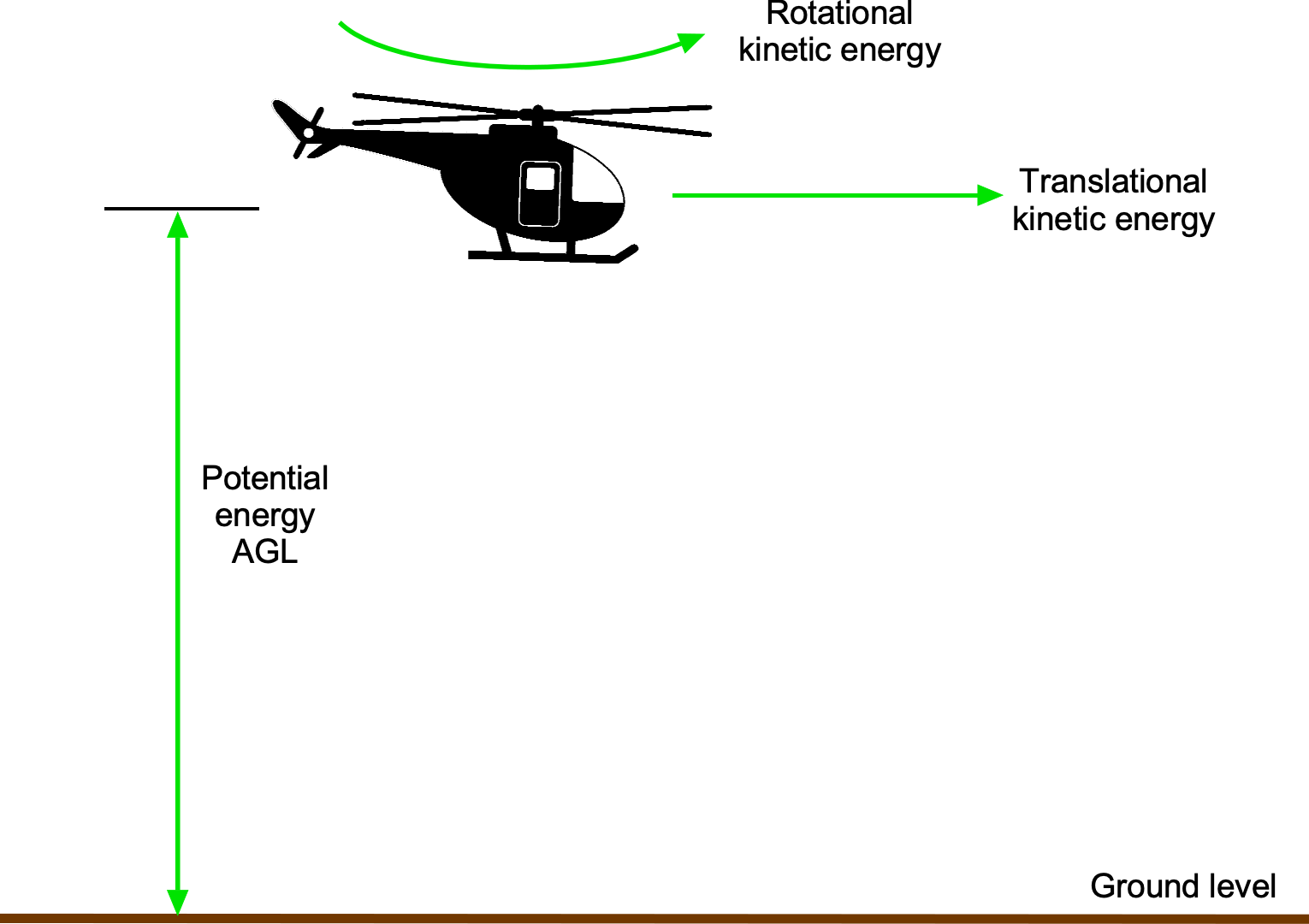
A helicopter’s energy state comprises potential energy (altitude), kinetic energy (airspeed), and stored rotational energy.

Differentiating Eq. 163 with respect to time gives

(164) $\begin{equation*} \frac{d E}{d t} = W \left( \frac{d h}{dt}\right) + \left( \frac{W}{g} \right) V_{\infty} \left( \frac{d V_{\infty}}{dt} \right) + I_R \, \Omega \left( \frac{d \Omega}{dt} \right) \end{equation*}$

The time rate of change of energy is power, so Eq. 164 represents a power available equation from a given energy state that could be used to drive the rotor in the event of engine power loss, i.e., to make up for the engine power loss. Notice that $dh/dt$ is a rate of altitude change relative to the ground with respect to time, i.e., a rate of descent.

In an autorotation, this initial energy must be given up at a controlled rate to produce the power to drive the rotor; this means that the sign of $dE/dt$ is negative. If the initial energy state is $E_0$ , then at any subsequent time $t$ , the energy state will be

(165) $\begin{equation*} E = E_0 - \int_{0}^{t} \left( \frac{dE}{dt} \right) dt \end{equation*}$

where

(166) $\begin{equation*} \frac{d E}{d t} = W V_c + \left( \frac{W}{g} \right) V_{\infty} \left( \frac{d V_{\infty}}{dt} \right) + I_R \Omega \left( \frac{d \Omega}{dt} \right) \end{equation*}$

The initial energy state can be expressed as

(167) $\begin{equation*} E_0 = W \, h_0 + \frac{1}{2} \left( \frac{W}{g} \right) V_{0}^2 + \frac{1}{2} I_R \, \Omega_{\rm \mbox{\tiny NR}}^{~2} \end{equation*}$

where $h_0$ is the initial height AGL, $V_0$ is the initial airspeed, and ${\Omega_{\rm \mbox{\tiny NR}}}$ is the normal rotor speed. Equation 166 can also be written as

(168) $\begin{equation*} -\frac{d E}{d t} = W V_d - \left( \frac{W}{g} \right) V_{\infty} \left( \frac{d V_{\infty}}{dt} \right) - I_R \, \Omega \left( \frac{d \Omega}{dt} \right) \end{equation*}$

where $V_d$ is the rate of descent of the helicopter. Notice that in a decent, $V_c$ is negative, i.e., $V_d = -V_c$ .

All three energy elements in Eq. 168 could, in principle, be used to drive the rotor and sustain autorotational flight after the complete loss of power to the rotor:

Give up altitude, i.e., potential energy.
Decrease airspeed, i.e., give up translational kinetic energy.
Decrease rotor speed, i.e., give up stored rotor energy.

It would be undesirable, at least in the first instance, to use rotor energy, i.e., rotor speed, because of the need for the rotor to maintain its thrust and allow for control. Indeed, the ability to use the stored rotor energy will be limited by the lowest allowable rotor speed, $\Omega_{\rm min}$ , such that

(169) $\begin{equation*} E_R = \frac{1}{2} I_R \bigg( \Omega_{\rm \mbox{\tiny NR}} - \Omega_{\rm min} \bigg)^2 \end{equation*}$

where ${\Omega_{\rm \mbox{\tiny NR}}}$ is the normal rotor speed. Usually ${\Omega_{\rm min}}$ is about 80% of ${\Omega_{\rm \mbox{\tiny NR}}}$ , but it depends on the helicopter.

However, as will become increasingly apparent in the following discussion, the ability to perform a successful autorotation to the ground ultimately requires the pilot to use all three available energy sources so that the helicopter’s net energy state at the end of the autorotation is approximately zero. This goal becomes an energy management problem of the flight trajectory, which, in turn, requires the pilot’s proper use of the flight controls.

Autorotative Rate of Descent

Assume a constant airspeed and rotor speed, then for a given weight, $W$ , then

(170) $\begin{equation*} -\frac{d E}{d t} = W \, V_d \end{equation*}$

This result shows that the pilot must descend at a sufficiently high rate to continue supplying power to drive the rotor, i.e., give up altitude or potential energy at just the correct rate from the initial energy state after power loss. Potential energy (altitude) is by far the most significant energy source, so giving up altitude is a necessary condition for an autorotation to occur.

Vertical Autorotation

In the zero-airspeed condition, i.e., a pure vertical autorotation, it has been previously shown that the equilibrium condition is achieved when

(171) $\begin{equation*} P_{\rm req}= 0 = W (V_c + v_i) + P_0 \end{equation*}$

which, as previously shown, occurs at the condition

(172) $\begin{equation*} \frac{V_c}{v_h} \approx -1.85 \end{equation*}$

when including the profile power contribution, ${P_0}$ . Therefore,

(173) $\begin{equation*} V_d = 1.85 v_h = 1.85 \sqrt{ \frac{T}{2 \varrho \, A}} = 1.85 \sqrt{ \frac{W}{2 \varrho \, A} } = 1.85 \sqrt{\frac{W}{A}} \left( \frac{1}{2 \varrho}\right) \end{equation*}$

It is assumed that thrust equals weight, i.e., $T = W$ . Notice that the rate of the autorotative descent increases with the square root of disk loading, $W/A$ , i.e., increasing the helicopter’s weight, and with increasing density altitude, i.e., lower air density. Indeed, it is found that the helicopter’s autorotational rate of descent is relatively high and much higher than what could be sustained to the ground, an issue discussed later.

Autorotation in Forward Flight

In forward flight, the power required for flight can be approximated as

(174) $\begin{equation*} P_{\rm req} \approx W \left(V_c + v_i \right) + P_0 + P_p + \mbox{Power for anti-torque} \end{equation*}$

where the induced velocity, $v_i$ , is given by Glauert’s approximation, i.e.,

(175) $\begin{equation*} v_i = \frac{W^2}{2 \varrho \, A \, V_{\infty}} \end{equation*}$

which is valid for higher airspeed values, $V_{\infty}$ , and is almost independent of the rate of climb or descent, $V_c$ . During autorotative forward flight, the rotor does not operate in the VRS or turbulent wake state, so the induced velocity is predictable according to momentum theory. For a given helicopter, variations of profile power, ${P_0}$ , and parasitic power, ${P_p}$ , depend mainly on airspeed and can also be assumed independent of the rate of climb or descent. In an established autorotative descent, then

(176) $\begin{equation*} P_{\rm req} = 0 = P_{\rm req_{\rm level}} - W \, V_d \end{equation*}$

where $P_{\rm req_{\rm level}}$ is the power required for level flight at the same weight, density, altitude, and airspeed. Therefore, in the autorotative state in forward flight at a constant airspeed, the power to drive the rotor comes by giving up altitude at a controlled rate, i.e.,

(177) $\begin{equation*} -\frac{d E}{d t} = W \, V_d \end{equation*}$

as shown in the figure below.

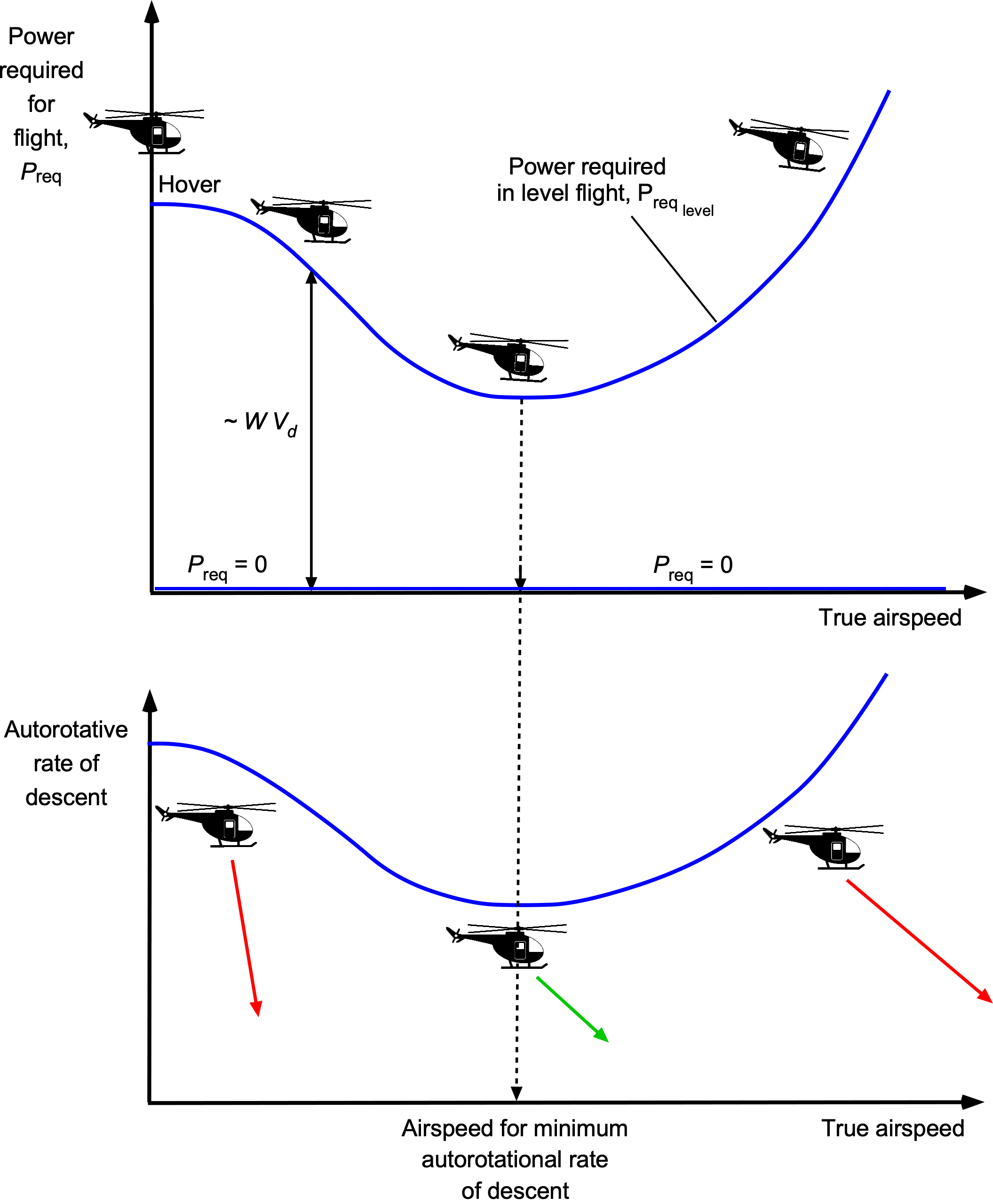
A successful autorotation means giving up altitude to drive the rotor,

It will now be apparent that the autorotative rate of descent depends on the airspeed at which the helicopter is flying. The highest rates are achieved at low and higher airspeeds, which should be avoided; a minimum rate of descent is obtained at an intermediate airspeed. However, the descent rates for an autorotating helicopter are still high at any airspeed, and they cannot be sustained as the helicopter approaches the ground.

Assume that some excess translational kinetic energy is also available at a constant autorotative rate of descent. In this case, then

(178) $\begin{equation*} -\frac{d E}{d t} = W \, V_d - \left( \frac{W}{g} \right) \left( \frac{d V_{\infty}}{dt} \right) V_{\infty} \end{equation*}$

Notice that $-d V_{\infty}/dt$ is a deceleration, which must be relatively quick to extract much energy. However, there are two conditions where airspeed can (or should) be used to control the flight path during the autorotational maneuver. The first is by pitching to obtain the lowest decent rate in the autorotation. The second is near the ground, where any remaining kinetic energy can be used to help arrest the rate of descent and reduce airspeed.

Finally, the potential effects of rotor speed on the autorotational characteristics must be considered. The rotor speed must be maintained throughout the maneuver because the rotor must maintain its thrust, produce sufficient levels of control, and avoid excessive blade flapping (from the reduction in centrifugal forces). It is also essential to be aware that if the rotor speed decreases too much, it may be impossible for the pilot to have sufficient control over the energy management in the landing phase.

The safe rotor speed range in autorotation for most helicopters is usually between 80% and 120% of the normal rotor speed, ${\Omega_{\rm \mbox{\tiny NR}}}$ , which the piloted controls using collective pitch. If the rotor speed becomes too low, the rotor will begin to stall, and excessive blade flapping may occur because of reduced centrifugal effects on the blades. There is also a risk that excessive flapping may cause the blades to contact the airframe. Conversely, structural overloads become a concern if the rotor speed becomes too high.

Summary of the Autorotational Maneuver

It is now possible to combine the elements of energy management to describe the ideal autorotational maneuver, as summarized in the figure below. A successful autorotational maneuver to the ground requires a high level of piloting skill, especially for larger and heavier helicopters.

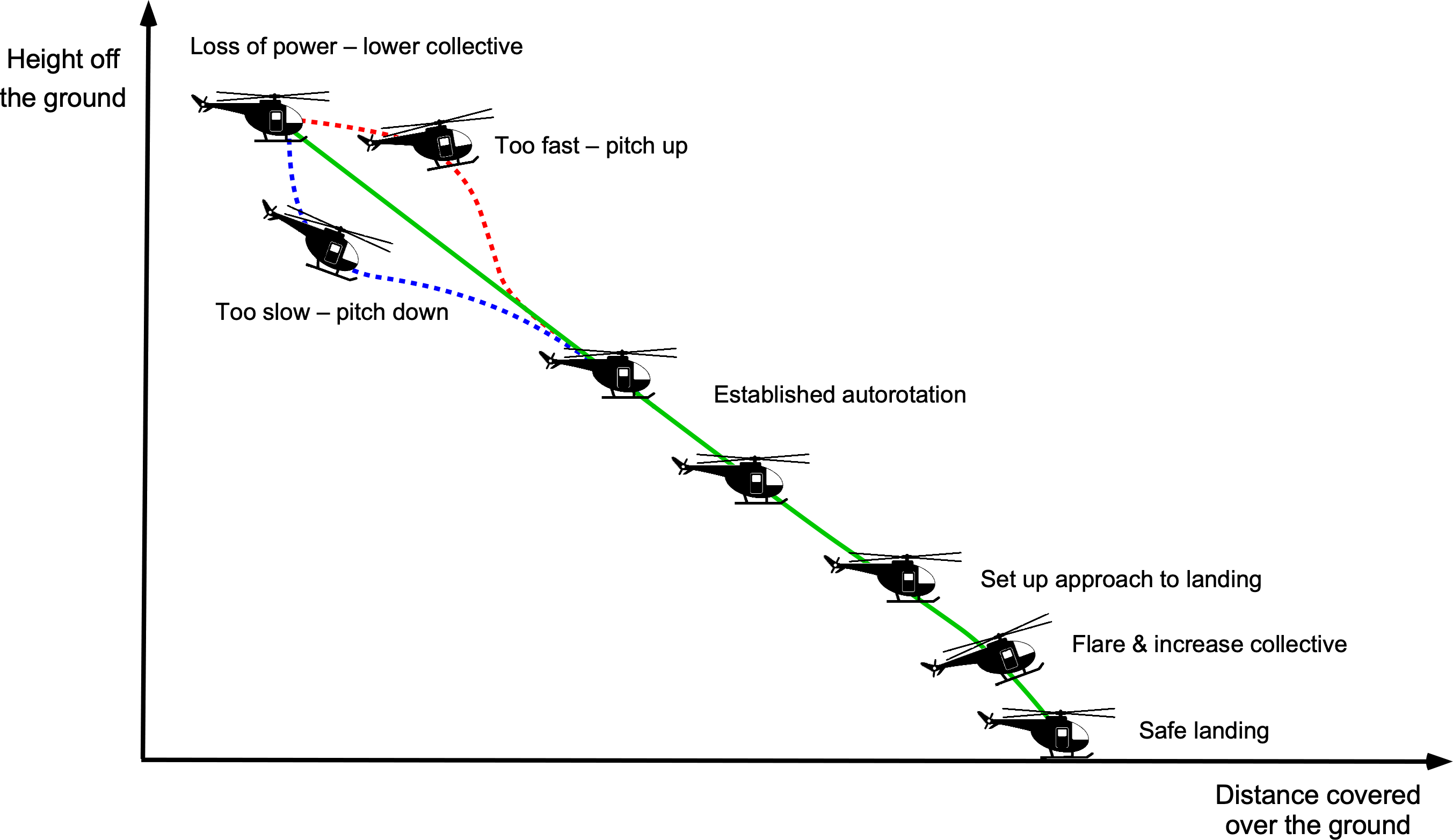
A successful autorotational landing requires careful energy management and the pilot’s skill.

Generally, a helicopter rotor’s polar moment of inertia is such that rotor speed can decay quickly, and some decrease in rotor speed can be anticipated on the entry into an autorotation unless a sufficiently low collective pitch is used to achieve steady-state autorotation. However, there may be aerodynamic and structural limits such as rotor stall, collective and cyclic pitch limits, and allowable blade flapping. The pilot must also be aware that if the collective is set too low, it can cause a rotor overspeed when established in an autorotation. In any case, the pilot’s first reaction to a complete loss of power requires the immediate reduction of the collective pitch to reduce drag on the blades and maintain the rotor speed.

In the established autorotation, the judicious control of the rate of descent and flight path will allow the pilot some time to determine a suitable landing location. Any excess airspeed can be used to slow the autorotative rate of descent closer to the lowest value. Bleeding off excess airspeed is performed by pulling back on the cyclic for a few seconds, also lowering the descent rate. If the airspeed is too low, forward cyclic can be used to reach this airspeed at the expense of some potential energy, i.e., some additional altitude loss. Most helicopters usually have the best autorotative rate of descent airspeed between 60 and 80 kts.

Near the ground, when the potential energy is essentially depleted, the stored rotor energy and any remaining kinetic energy can be used to arrest and cushion the final landing. This is done by the pilot pulling up on the collective, which will cause rotor speed to decay. Pitching up to a nose-high attitude will extract any remaining translational kinetic energy to help maintain rotor speed while ensuring the tail does not strike the ground. This type of maneuver before the final landing is common to all autorotations. The overall objective for the pilot is to cushion the rate of descent such that the helicopter touches down with a rate of descent less than about 10ft s $^{-1}$ ( ${\approx}$ 3~ms $^{-1}$ ) with minimal forward speed.

Suppose the pilot manages the controls and flight trajectory such that all initial potential, kinetic, and rotational energy is extracted at the end of the autorotational maneuver. In that case, the outcome is that the helicopter ends up on the ground with zero airspeed and a lower-than-normal rotor speed. Some residual rotor speed is desirable to maintain directional control (through the tail rotor inputs) and minimize blade flapping as the helicopter settles onto the ground.

Rotor Design for Autorotational Capability

A primary aspect of the rotor design that must be considered to assess the autorotational behavior of the helicopter is its polar moment of inertia and stored rotational kinetic energy. There are two design points to consider: 1. The potential decay in rotor speed after the initial loss of power. 2. The stored kinetic energy of the rotor system at the end of the autorotational flight maneuver can be used to arrest the rate of descent and allow the helicopter to land safely. Both conditions involve the polar moment of inertia of the rotor.

The equation describing the decay of the rotor’s rotational speed is

(179) $\begin{equation*} I_R \frac{d\Omega}{dt}= -Q \left( \frac{\Omega}{\Omega_{\rm \mbox{\tiny NR}}} \right)^{~2} \end{equation*}$

where $Q$ is the torque to the rotors shaft, and ${\Omega_{\rm \mbox{\tiny NR}}}$ is the initial or normal rotor speed. Integrating Eq. 179 using separation of variables gives

(180) $\begin{equation*} \int_{\Omega_{\rm \mbox{\tiny NR}}}^{\Omega} \, \frac{d \Omega}{\Omega^2} = -\frac{Q_{0}}{I_R \, \Omega_{\rm \mbox{\tiny NR}}^{~2}} \int_{0}^{t} dt \end{equation*}$

where the subscript 0 refers to time $t = 0$ . After rearrangement, then

(181) $\begin{equation*} \frac{\Omega}{\Omega_{\rm \mbox{\tiny NR}}} = \frac{1}{1 + \displaystyle{\frac{Q_{0} \, t}{I_R \, \Omega_{\rm \mbox{\tiny NR}}}}} = \frac{1}{1 + \displaystyle{\frac{t}{\tau}} } \end{equation*}$

where the time constant, $\tau$ , is

(182) $\begin{equation*} \tau = \frac{I_R \, \Omega_{\rm \mbox{\tiny NR}}}{Q_{0}} = \frac{I_R \, \Omega_{\rm \mbox{\tiny NR}}^{~2}}{Q_{0} \, \Omega_{\rm \mbox{\tiny NR}}} = \frac{2 \, K\!E_{\rm \mbox{\tiny NR}}}{P_{0}} \end{equation*}$

This outcome means that rotors with higher levels of stored kinetic energy and lower power requirements (i.e., lowest disk loading) will have the largest values of $\tau$ , so the slowest rate of rotational speed decay after the loss of power. For a given rotor speed, the rotor inertia controls the kinetic energy, which must have some minimum value for the helicopter to meet autorotational safety requirements, which is a design problem.

Initial Energy State (Height-Velocity Diagram)

The acceptable flight conditions, and hence the initial energy state, $E_0$ , that will allow recovery of the helicopter and safe entry into autorotation, are summarized for the pilot as height-velocity or H-V curves. The initial energy state can be expressed as

(183) $\begin{equation*} E_0 = W \, h_0 + \frac{1}{2} \left( \frac{W}{g} \right) V_{0}^2 + \frac{1}{2} I_R \, \Omega_{\rm \mbox{\tiny NR}}^2 \end{equation*}$

where $h_0$ is the initial height, $V_0$ is the initial airspeed, and ${\Omega_{\rm \mbox{\tiny NR}}}$ is the normal rotor speed. The potential and kinetic energy are high enough for the pilot to land safely using an autorotation for flight anywhere outside the AVOID region. Inside the avoid region, the initial energy state is too low to perform a successful autorotation to the ground. While flight in the AVOID region would not otherwise be prohibited, its boundaries dictate the conditions where sustained flight operations should be avoided. Some margin for pilot reaction time, which may be several seconds, must also be allowed to determine these boundaries, increasing the H-V diagram’s vertical and horizontal extent.

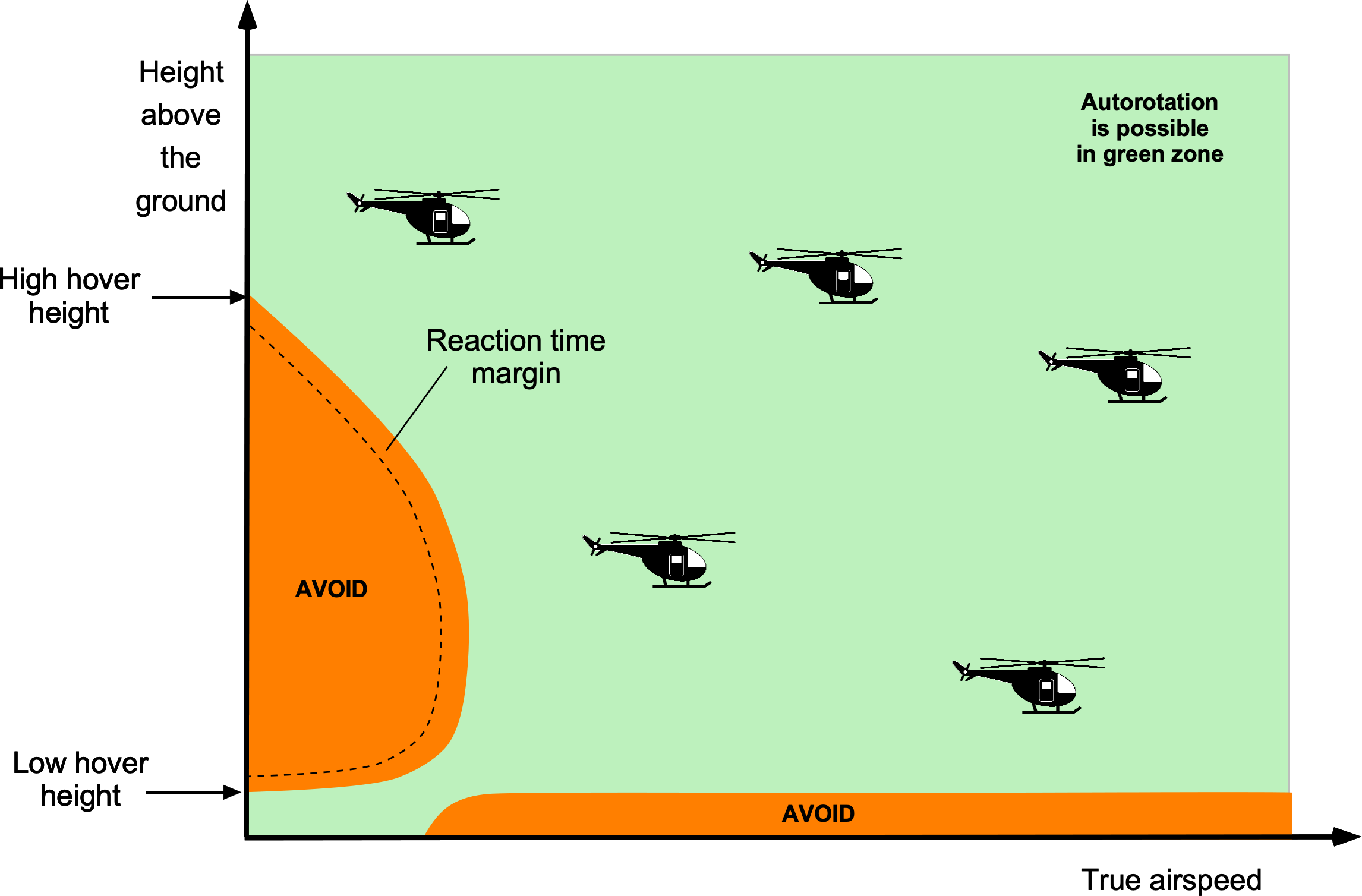
A height-velocity (H-V)diagram establishes the altitude above the ground and airspeed where a successful autorotation to the ground would be impossible.

The size and shape of the H-V curve depend on many factors, including the general characteristics of the helicopter, its gross weight, and operational density altitude. The disk loading $W/A$ is the primary parameter influencing the autorotative rate of descent and the extent of the AVOID region. Note that the H-V diagram defines two AVOID regions. The second AVOID region at lower altitudes and higher airspeeds determines the minimum altitude below which translational kinetic energy cannot be converted into potential energy using a zoom-climb before entering the autorotation. The most critical AVOID region is, however, at low airspeeds.

Reducing the size of the AVOID region is desirable from an operational point of view but generally requires more work from an engineering perspective. Helicopters with low disk loading will tend to have smaller AVOID regions; hence, the need for safe autorotative characteristics of the helicopter must be considered in the basic sizing and design of the rotor system. In this regard, the higher the polar moment of inertia, the smaller the AVOID region. The downside is that giving a rotor a lower disk loading and higher polar moment of inertia means a larger and heavier rotor, which will reduce the useful load of the helicopter.

A survey of helicopter accidents conducted by Harris et al. found that out of 8,436 accidents, 2,408 occurred because of the loss of engine power. About half of these 2,408 accidents resulted from fuel exhaustion. 935 accidents resulted in substantial damage to the helicopters, and 445 helicopters were destroyed. Besides the tragic loss of lives, such statistics are certainly not acceptable from an engineering standpoint and clearly emphasize the need for better helicopter designs with adequate single-engine inoperative performance and safe autorotational landing capability.

Autorotative Index

As will now be apparent, the autorotative performance of a helicopter depends on several factors. These include the rotor disk loading and the stored kinetic energy in the rotor system. Part of the issue is the subjective “difficulty rating” assessments by test pilots. Therefore, an autorotative index is often used to help design the rotor. Although various indices have been used, the autorotation index is a rotational kinetic energy factor. One autorotative index, $AI$ , is given by

(184) $\begin{equation*} AI = \frac{I_R \Omega^2}{2 W DL} \end{equation*}$

where $W$ would be the maximum gross weight and $DL$ , is the corresponding disk loading.

Autorotative indices for several helicopters at standard sea-level conditions based on published information for each helicopter are shown in the figure below. These indices are helpful in rotor sizing or examining the effects of autorotative characteristics with increasing gross weight or density altitude. The absolute values of the index are of no significance. Still, the relative values provide a means of comparing the autorotative performance of new designs against others with already acceptable autorotative characteristics. An index of about 20 is generally adequate for single-engine helicopters. In contrast, a multi-engine helicopter can have an index as low as 10 and still have safe flight characteristics in case of a single-engine failure.

An autorotative index (AI) is a measure of potentially successful autorotational capability. Tiltrotors cannot autorotate to a landing like helicopters.

Limitations of the Helicopter

Helicopters are versatile aircraft with unique capabilities, but like all aircraft, they have intrinsic limitations. Indeed, as summarized in the figure below, the helicopter suffers from a fascinating concatenation of aerodynamic and aeromechanical problems that limit its overall capabilities, especially as it flies faster. Airplanes are limited by the onset of wing stall at low airspeeds and compressibility at high airspeeds. The performance of a helicopter can be limited by both or either at the same airspeed! Remember that for a helicopter, however, “fast” means only about 150 kts (278 kph). As previously discussed, helicopters are less aerodynamically efficient than fixed-wing aircraft, so their flight range and payload are limited. The creation of obtrusive noise is also an issue for helicopters in both civil and military use.

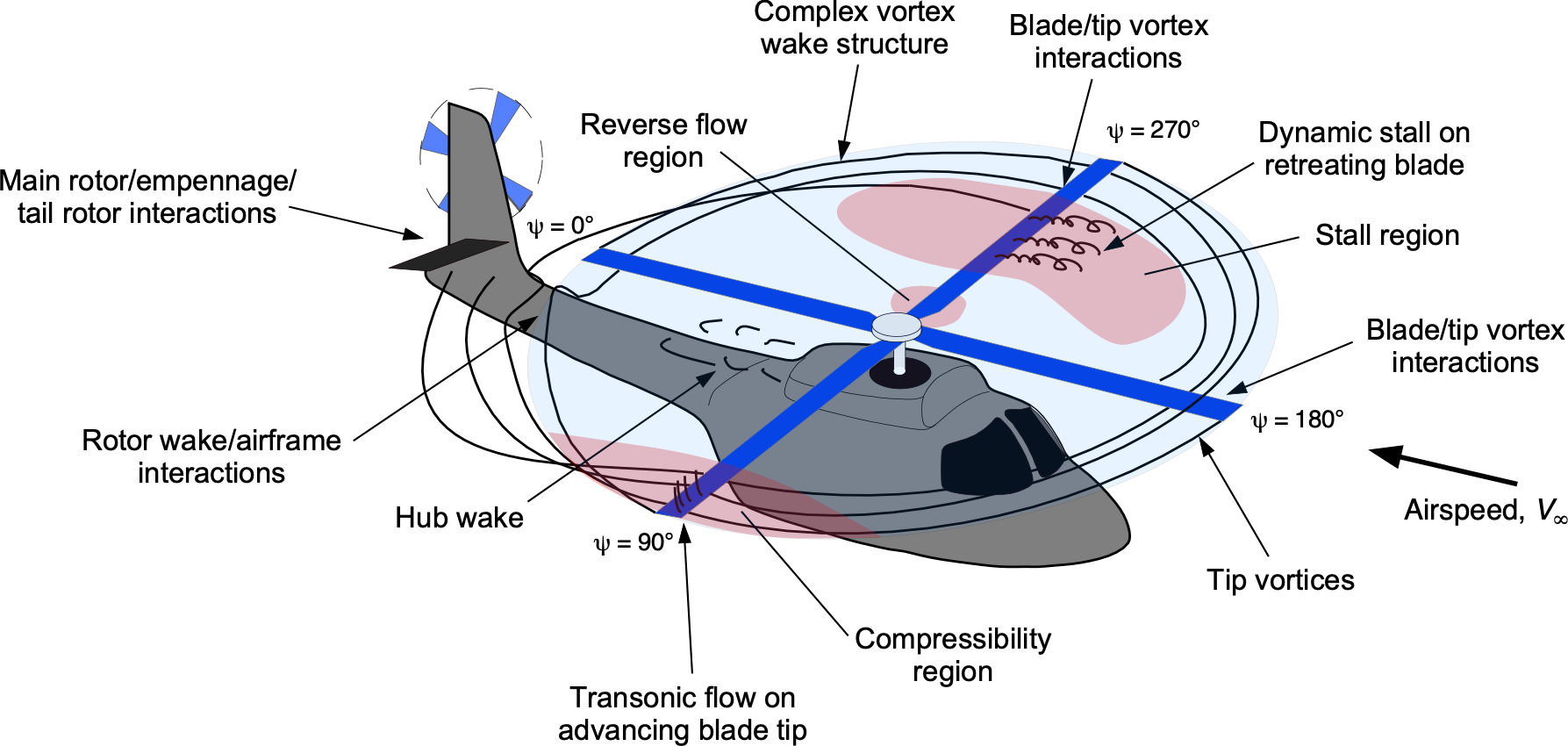
A plethora of aerodynamic problems begin to plague the helicopter’s capabilities as it flies faster.

Advancing Blade Compressibility

The most apparent aerodynamic effect limiting the forward flight performance of a helicopter is that the blade tips on the advancing side of the rotor disk suffer from compressibility effects at higher airspeeds because of the onset of supercritical and transonic flow. The upshot is the formation of shock waves and the associated wave drag, possibly even shock-induced flow separation. The periodic formation of shock waves can also be a source of impulsive noise. The increased power demands placed on the rotor system from compressibility losses can eventually limit the forward flight speed of the helicopter if it encounters its power available or mechanical shaft torque limits.

The onset of compressibility effects on the advancing blade is relatively gradual with increasing rotor advance ratio after reaching a critical threshold. Consider the Mach number at the blade tip at $\psi$ = 90^o, which is given by

(185) $\begin{equation*} M_{\rm tip} = \frac{\Omega \, R \left( 1 + \mu \right)}{a_{\infty}} \end{equation*}$

Drag from compressibility effects will begin to manifest when $M_{\rm tip} =M_{\rm dd}$ , where $M_{\rm dd}$ is the drag divergence Mach number of the airfoil section in the tip region.

The figure below shows that the blade tip can be swept back to relieve these effects, analogous to that used on an airplane wing for high-speed flight. The component of the Mach number normal to the blade’s leading edge over the tip region is what matters, which will be reduced by $\cos \Lambda$ . Therefore, the advance ratio (and hence airspeed) where compressibility effects manifest can be increased (approximately) by a factor of $1/\cos \Lambda$ before compressibility effects are re-encountered.

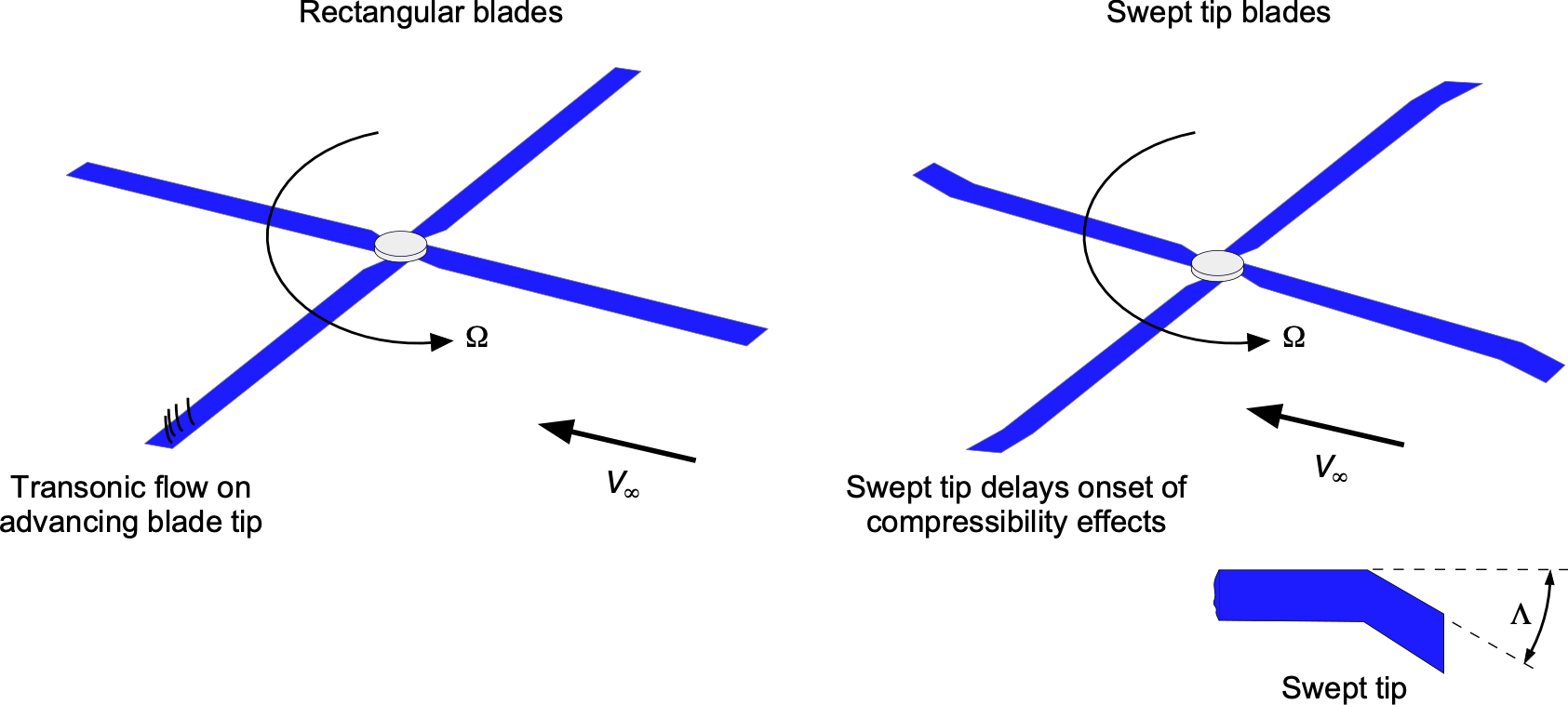
Sweeping the blade tip can limit the build-up of compressibility effects to a higher airspeed.

Retreating Blade Stall

On the retreating side of the rotor disk, the local velocities at the blades are relatively low, and the blades must operate at higher angles of attack to maintain lift and propulsion. If these angles of attack become too large, the blades will stall, losing lift and increasing drag and producing torsional pitching moments that can increase vibratory loads. At higher forward speeds, the onset of reverse flow on the retreating blade contributes to a loss of lift and higher drag. Both stall and reverse flow effects result in a loss of overall lifting and propulsive capability from the rotor and, like compressibility effects, begin to form an aerodynamic limit that impedes any further increases in forward flight speed or the ability of the rotor to carry more weight at a given flight speed.

Because of the inherently unsteady nature of the flow environment on the rotor blades in forward flight, combined with the pitch rates from cyclic pitch inputs and torsional blade dynamics, when blade stall occurs, it is called a dynamic stall. This phenomenon has received much research interest because of the unsteady aerodynamic effects produced, which include the shedding of a leading-edge vortex, as shown in the simulation below. The onset of dynamic stall can produce very high structural loads and vibration and can limit the airspeed, load-carrying capability, as well as maneuvering performance of the helicopter.

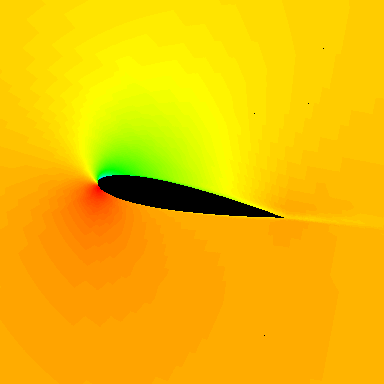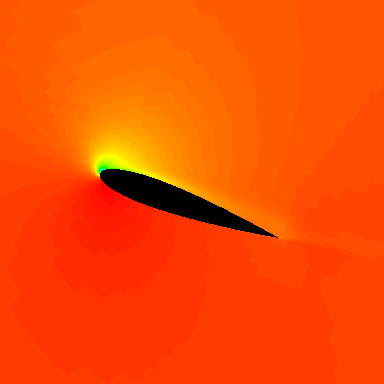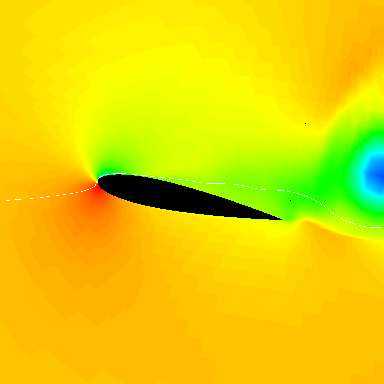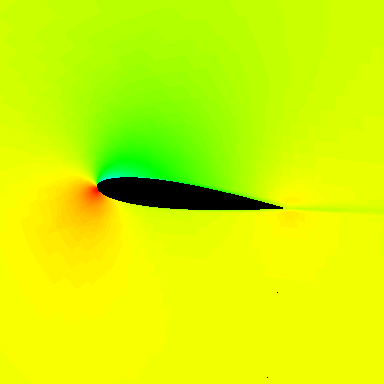
A CFD animation of dynamic stall on an oscillating airfoil. (With permission of Dr. George Barakos.)

Blade/Vortex Interactions

The low rotor disk loading and generally low average inflow velocities through a helicopter rotor cause the blade tip vortices to remain close enough to the rotor to produce a phenomenon known as blade/vortex interaction (BVI). The BVI phenomenon produces sharp changes in lift on the blades and can be a source of exceptionally high vibratory loads from the rotor. BVI is also accompanied by significant impulsive noise, a recognizable “wop-wop-wop” signature of an approaching helicopter. The tail rotor (if one is used) is also a significant source of aerodynamic noise, often appearing over a wide range of audible frequencies.

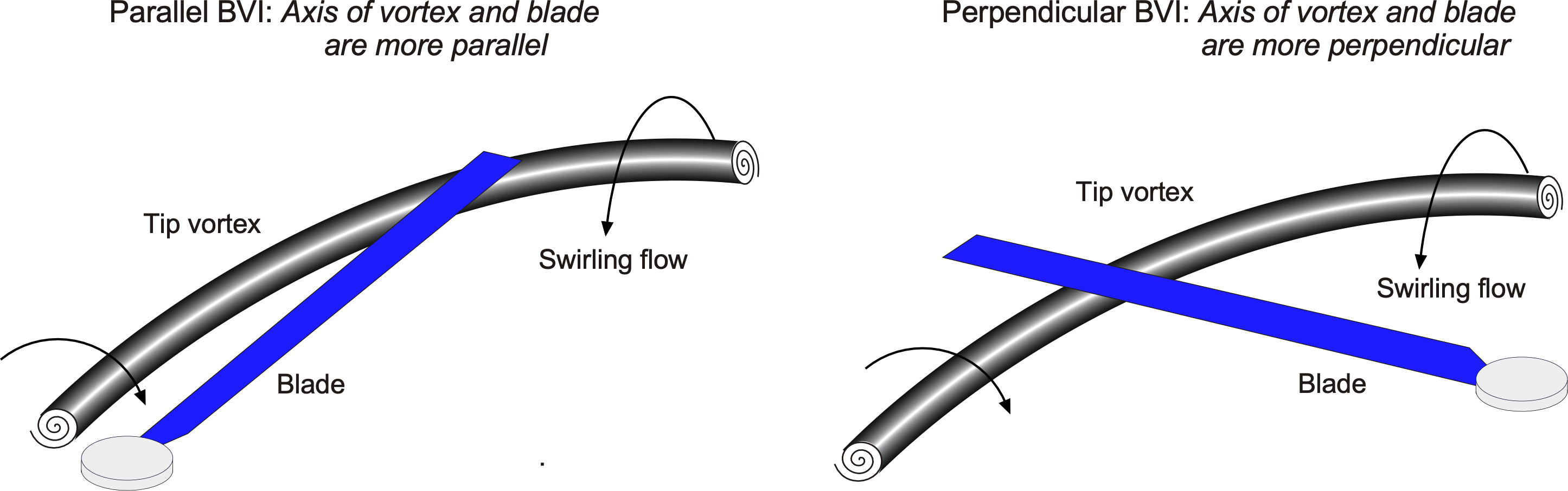
While BVI has many variations, the perpendicular and parallel interaction represent two extremes.

The reduction of rotor noise, as well as engine and transmission noise in the cabin, has been a more recent goal in helicopter design. Still, it is essential from both civil (environmental and community acceptance issues) and military (detectability) perspectives. While many mathematical models exist to predict helicopter noise levels, they are deeply inveterated in empiricism. Increasingly stringent noise-reduction requirements imposed by certification authorities to develop environmentally friendly aircraft will force helicopter manufacturers to look for more efficacious noise-reduction solutions. In most cases, understanding the root of the noise problems on helicopters lies firmly in understanding and predicting their unsteady aerodynamics.

Airframe Aerodynamics

The fuselage of a conventional helicopter is roughly shaped like a prolate spheroid, with a cylindrical mast or pylon to support the central rotor hub and controls and a long quasi-cylindrical tail boom supporting the tail rotor and empennage, as shown in the figure below. The typically high parasitic drag of these shapes significantly limits the helicopter’s cruise speed, increases its fuel consumption, and reduces its range. In cruise conditions, the primary drag on the helicopter’s airframe is pressure drag; this differs from an airplane’s fuselage, where the dominant drag source in cruise flight is mostly skin friction drag (boundary layer shear stress drag). Most parasitic drag on the helicopter is from the rotor pylon and hub, the fuselage, engine inlets, tail rotor, landing gear (skids or wheels), and component flow interference effects. As for other aircraft types, streamlining can effectively reduce airframe drag, as the figure below suggests.

Streamlining a helicopter can significantly reduce parasitic drag, although there may be other design trades to consider.

A significant contributor is the fuselage, the after-body drag, which is caused by flow separation in the region where the main fuselage tapers to the tail boom. Flow separation forms two energetic trailing vortices on some fuselage shapes, with high aft taper ratios and large upsweep angles, further adding to induced drag. These vortices are not steady, and the subsequent unsteady wake flow can interact with the empennage and tail rotor, increasing vibration levels and producing tail shake.

The exposed rotor pylon, hub, and blade attachments significantly contribute to airframe drag and are another source of unsteady flow or buffeting. As expected, the rotor hub significantly impacts drag and the helicopter’s forward flight performance. However, minimizing the rotor mast height and enclosing the swashplate and pitch linkages inside a fairing help reduce this parasitic drag.

Future Helicopters?

The schematic below shows one hypothetical future or ultimate helicopter concept incorporating a melange of technological ideas. This does not mean that future helicopters will look like this, and almost certainly not because such an aircraft will be prohibitively expensive to develop, but simply that they will incorporate one or more of the concepts synthesized in this schematic. However, it’s important to realize that developing and adopting new technologies in the helicopter industry is a gradual, if not incremental, process. Therefore, future helicopter design changes will be driven not only by technological advancements but also by regulatory considerations, reliability, environmental concerns, costs, and other requirements.

Future helicopter designs will continue incorporating state-of-the-art materials and design techniques to enhance aerodynamics and reduce structural weight, improving fuel efficiency and overall performance. Helicopters might adopt more modular designs, allowing for easier customization and upgrades. This could make it cost-effective to have helicopters with mission adaptive profiles, i.e., by swapping out modules or components for different missions. Future helicopters, at least the smaller ones, will incorporate hybrid or fully electric propulsion systems to reduce emissions and noise levels, making helicopters more sustainable and less disruptive. However, in the end, the ultimate helicopter may be a relatively simple but extremely well-designed machine with as few of these “gadgets” as possible.

Indeed, the future seems clear. On the one hand, the traditional helicopter industry, much like aerospace as a whole, tends to favor reliability and incremental improvements over innovation. This is somewhat understandable because safety regulations, high R&D costs, and the long lifecycle of aircraft contribute to this conservatism. But it seems that the extant industry is destined for stagnation. On the other hand, startup companies have the flexibility to explore new technologies, such as electric propulsion, autonomous flight, or novel airframe designs, without being weighed down by the need to conform to legacy systems and corporate bureaucracy. New companies are already pushing the envelope with eVTOL (electric vertical takeoff and landing) aircraft, which could disrupt traditional rotorcraft operations.

VTOL Jet-Thrust Airplanes

VTOL (Vertical Take-Off and Landing) jet airplanes are specialized aircraft designed to take off vertically, hover for a short time, perform a mission, and then return and land vertically. Like helicopters, these runway-independent airplanes achieve vertical lift by generating a thrust equal to or greater than their weight. However, this capability is achieved using pure jet thrust, which is ultimately an inefficient way of producing vertical lift, i.e., the effective disk loading is extremely high, about two orders of magnitude higher than for a helicopter. Once airborne, such an airplane cannot hover for long because it will use too much fuel to leave enough for the primary mission. Therefore, when it is safe, it must quickly transition and gain airspeed where conventional aerodynamic lift on its wing then replaces the lift generated by pure vertical jet thrust.

Many such V/STOL aircraft concepts have been designed over the decades. As shown below, the so-called V/STOL Wheel of Misfortune catalogs the most prominent attempts to combine a helicopter’s good vertical takeoff and landing capabilities with an airplane’s high-speed forward flight capability. The Wheel of Misfortune also reminds engineers that designing a VTOL aircraft is challenging and that they should not “reinvent the wheel” because lessons learned is a powerful tool to help in engineering design.

A wheel diagram of different types of aircraft. — The V/STOL Wheel was initially developed by McDonnell Aircraft. Michael Hirschberg has updated it over the years. (Click on the image to see a bigger version.)

Only three of the numerous VTOL or V/STOL airplanes on the wheel have succeeded and made it to production. The best-known is the Harrier Jump Jet, which became the McDonnell-Douglas AV-8B Harrier. In recent years, a version of the Lockheed Martin F-35 Lightning Joint Strike Fighter has been designed specifically for V/STOL flight (the F-35B), which uses a powerful counter-rotating lift fan in the fuselage and a swiveling jet nozzle for vertical lift, inspired by the Russian Yak-141. The turbofan engine provides thrust for forward flight, but some of the power can also be diverted to a shaft to drive a dedicated lift fan for vertical takeoff and hovering, as shown in the figure below. Like the Harrier, the F-35B has two small nozzles in the wing to give roll control.

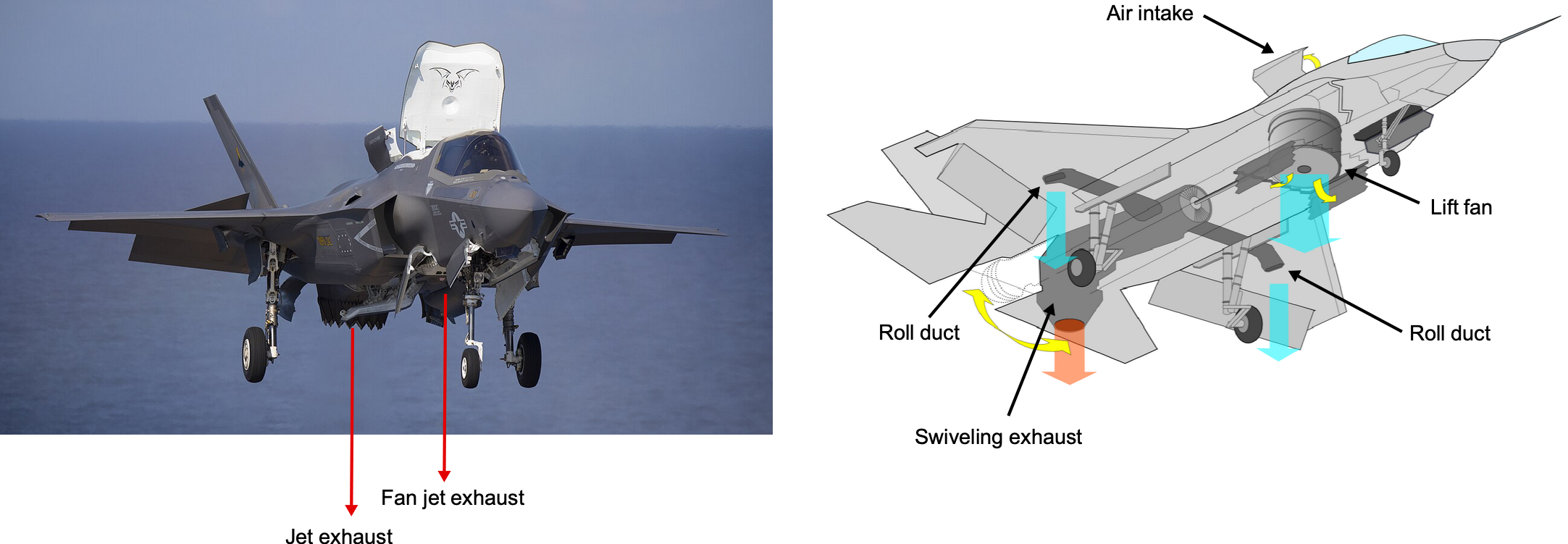
The F-35B has a V/STOL capability, obtained by combining a lift fan with a swiveling jet exhaust. Although this configuration requires a second lift mechanism, the lift fan is disconnected in forward flight.

Jet Thrust

VTOL airplanes generate vertical thrust to take off, but hovering on jet thrust requires enormous amounts of power and fuel. The thrust for VTOL operation is governed by Newton’s second and third laws, i.e., from conservation of momentum, the jet thrust, $T$ , is given by

(186) $\begin{equation*} T = \overbigdot{m} \, v_e + \left( p_e - p_{\infty} \right) A_e \end{equation*}$

where $\overbigdot{m}$ is the mass flow rate of exhaust gases, $v_{e}$ is the “jet” or “exit” velocity of the exhaust gases, $p_{\text{e}}$ is the exhaust pressure, $p_{\infty}$ is the ambient pressure at the inlet to the engine, and $A_{e}$ is the net or equivalent nozzle exit area. In most VTOL operations, the exit nozzles for vertical thrust are designed of ideal expansion so that $P_e \approx p_{\infty}$ , simplifying the thrust equation to

(187) $\begin{equation*} T = \overbigdot{m} \, v_{\text{e}} \end{equation*}$

To sustain hover, the net vertical thrust must balance the aircraft’s weight, i.e., $T = W$ . Therefore, a key metric for VTOL aircraft is the thrust-to-weight ratio (TWR), i.e.,

(188) $\begin{equation*} \text{TWR} = \frac{T}{W} \end{equation*}$

For VTOL, a $\text{TWR} > 1$ is necessary to achieve takeoff and to initiate the flight into a vertical climb. Successful VTOL airplanes must have a TWR of at least 1.1, so just a modest excess thrust over and above that required to hover. The thrust requirements in forward flight are much less than for hovering flight, so the excess thrust allows for impressive flight performance.

Aerodynamic Efficiency and Transition to Forward Flight

Hovering with pure jet thrust has high power requirements and is fuel-intensive. Therefore, VTOL jets transition quickly to aerodynamic lift, where the wings sustain flight more efficiently. The lift is given by the usual formula, i.e.,

(189) $\begin{equation*} L = \frac{1}{2} \varrho_{\infty} \, V_{\infty}^2 \, C_{L_{\rm max}} \, S = W \end{equation*}$

where $L$ is the lift force, $\varrho_{\infty}$ is the air density, $V_{\infty}$ is the airspeed, $C_{L_{\rm trans}}$ is the maximum transition lift coefficient for the wing to start flying, $S$ is the wing area, and $W$ is the weight of the airplane. The transition speed at which aerodynamic lift replaces thrust-based lift will be

(190) $\begin{equation*} V_{\text{trans}} = \sqrt{\frac{2 W}{\varrho \, C_{L_{\rm trans}} \, S}} \end{equation*}$

This speed is crucial because the aircraft must accelerate to this airspeed to achieve normal wing-borne flight. In the Harrier, for example, the transition process is seamless. The pilot gradually swivels back the nozzles, and the airplane accelerates quickly to the needed airspeed to achieve full wing-borne flight.

Harrier Thrust Vectoring System

The Harrier Jump Jet utilizes a unique thrust-vectoring system known as the “four-poster” configuration. This configuration consists of four small rotating nozzles positioned in pairs beneath the wings, as shown in the photographs below. The nozzles can be directed straight downward for hovering, fully aft for forward flight, or at intermediate angles to facilitate short takeoff, landing, and in-flight maneuverability.

The Harrier emerged as the only truly successful V/STOL out of dozens of designs.

A feature of the Harrier is that all four nozzles, along with two small roll-control jets located in the wingtips, are powered by a single turbofan engine. This integrated propulsion system eliminates the need for separate lift and cruise engines. However, this design also imposes constraints on the aircraft’s layout because the engine must be positioned close to the center of gravity of the aircraft to maintain trim and ensure effective control. The Harrier’s thrust-vectoring system gives tremendous versatility in combat, allowing operations from short runways, aircraft carriers, and even rough terrain. However, it comes at the cost of high pilot workload, high fuel consumption in hover, and strict payload and munitions weight limitations in VTOL mode.

Check Your Understanding #7 – Calculating the power and fuel consumption for the Harrier

Estimate the thrust, power, and fuel consumption for the Harrier AV-8B when it is hovering. Also, calculate the transition airspeed to wing-borne flight. The following parameters are readily available for the Harrier (AV-8B) airplane: Mass $M = 9,400$ kg, engine type Rolls-Royce Pegasus 11-61 with an exhaust velocity $v_e = 550$ m/s, wing area $S = 18.68$ m², wing transition lift coefficient $C_{L_{\rm trans}} = 1.4$ , and propulsive efficiency $\eta = 0.5$ .

Show solution/hide solution.

Based on the weight of the airplane, the thrust required for hovering flight is

$T_{\text{req}} = W = M \, g = 9,400 \times 9.81 = 92,214 \text{ N} = 92.2 \text{ kN}$

To sustain hover, the engine must provide 92.2 kN of thrust, which means the net mass flow rate (all four nozzles) must be

$\overbigdot{m} = \frac{T_{\text{req}}}{v_{e}}= \frac{92,214}{550} = 167.66 \text{ kg/s}$

Therefore, the Pegasus engine must expel the exhaust at a net mass flow rate of 167.66 kg/s with an exhaust velocity of 550 m/s. The power required for hovering flight is then

$P = \frac{T_{\text{req}} \, v_e}{\eta} = \frac{92,214 \times 550}{0.5} = 101.44 \times 10^6 \text{ W} = 101.44 \text{ MW}$

Notice that in hovering mode, the thrust-producing efficiency is relatively low because of the inherent characteristics of a turbofan engine; this efficiency improves markedly with airspeed, however. Therefore, hovering requires a massive \textbf{101.44 MW} of power, which explains why the fuel consumption is extremely high in VTOL mode. The thrust-specific fuel consumption (TSFC) of the Rolls-Royce Pegasus engine in hover mode is approximately

$\text{TSFC} \approx 1.35 \, \text{kg kN$^{-1}$ hr$^{-1}$}$

So, the fuel mass flow rate is

$\overbigdot{m}_f = \text{TSFC} \, T_{\text{req}} = 1.35 \times 92.2 = 7,468.2 \, \text{kg/h}$

The AV-8B has an internal fuel capacity of approximately 3,402 kg (7,500 lb), so hovering time is very limited! With up to three external drop tanks, its total fuel capacity increases to 6,122 kg (13,500 lb), so allowing VTOL operations with a combat radius to around 300–400 nautical miles.

The transition speed from hovering to fully wing-borne flight will be

$V_{\text{trans}} = \sqrt{\frac{2 W }{\varrho \, C_{L_{\rm trans}} S}} = \sqrt{\frac{2 \times 9,400 \times 9.81)}{1.225 \times 1.4 \times 18.68}} = 75.9 \text{ m/s} = 273 \text{ km/h} = 147.4 \text{kts}$

Therefore, the Harrier must reach 147.4 kts before fully transitioning so the airplane can fly with full aerodynamic lift on the wing.

Drones & eVTOL

The recent emergence of electrically powered VTOL or eVTOL concepts for drones and Urban Aerial Mobility (UAM), has kindled interest in advancing vertical flight technologies. Such eVTOL aircraft are envisaged to be part of a safe and efficient aviation “air taxi” UAM infrastructure, rapidly transporting passengers and cargo over short distances at lower altitudes within urban and suburban areas. The eVTOL community has many innovators, but it remains to be seen whether these less orthodox aircraft can work technically with the speed, range, and endurance needed, as well as be safely integrated within the national airspace system.

Early quadcopters and small drones, often called unoccupied aerial vehicles or UAVs, demonstrated the feasibility of distributed electric propulsion. These were soon scaled up into piloted and autonomous eVTOL air taxi concepts. Companies like Joby Aviation, Archer, and Vertical Aerospace are now developing aircraft for UAM, although it is still a niche market. eVTOL aircraft rely on multiple rotors or tilt-rotor mechanisms to generate vertical lift, and then transition to forward flight. Unlike helicopters, which use a single large rotor, most UAVs and eVTOLs distribute thrust across several smaller rotors, each driven by its own electric motor, increasing redundancy for safety. Some designs employ fixed wings for greater efficiency in cruise flight, the rotors shifting from vertical lift to horizontal propulsion. As shown in the photograph below, the hybrid quad-rotor or “quadcopter” with fixed wings for lift generation in forward flight has become a prevalent configuration for UAVs, such as drones and many eVTOL concepts.

A type of eVTOL drone, which is playing an increasingly important role in the aviation spectrum.

Performance

The biggest challenge in eVTOL development is energy storage for flight, as illustrated in the table below. Unlike airplanes powered by jet fuel or gasoline, which have high energy density (i.e., energy per unit mass), lithium-ion batteries give eVTOL aircraft much more limited range and endurance. A typical eVTOL requires high-capacity batteries with fast discharge rates and efficient power management to extend flight time.

Power Source

Energy Density (Wh/kg)

Jet Fuel

12,000

Lithium-Ion Battery

250–300

Solid-State Battery

400–600 (future)

An eVTOL functions like a multi-rotor helicopter in hover, with each rotor contributing to the total thrust. The aircraft must generate enough thrust to balance its weight, i.e.,

(191) $\begin{equation*} T_{\text{tot}} = W \end{equation*}$

For an eVTOL with $N_r$ independent rotors, it can be assumed that the thrust is evenly distributed between the rotors, i.e.,

(192) $\begin{equation*} T_{r} = \frac{W}{N_r} \end{equation*}$

where $W$ is the total weight of the vehicle. The power is then calculated using

(193) $\begin{equation*} P_h = \left( \frac{N_r}{FM} \right) \frac{T_{r}^{3/2}}{\sqrt{2 \varrho \, A_r}} \end{equation*}$

where $A_r$ is the disk area of each rotor.

Analysis

The challenges of electric flight can be demonstrated by estimating the energy requirements for a proposed 4-rotor eVTOL configuration. The aircraft is a hybrid quad-rotor, which uses the four rotors for takeoff and two of the rotors for propulsion in wing-borne flight.^[6] The manufacturer claims the following characteristics of the eVTOL:

Empty airframe mass (with motors and wiring) = 600 kg
Estimated gross takeoff mass, $M$ = 1,200 kg
Number of rotors, $N_r$ = 4
Rotor radius, $R$ = 1.3 m
Total mission duration: 45 minutes ( $t_h$ = 10 min hover + $t_c$ = 35 min cruise.)
Two passengers (150 kg each) = 300 kg

The total lifting thrust required in hover is

(194) $\begin{equation*} T_{\text{tot}} = W = Mg = 1,200 \times 9.81 = 11,772 \text{ N} \end{equation*}$

Thrust per rotor (evenly distributed across four rotors) is

(195) $\begin{equation*} T_{r} = \frac{T_{\text{tot}}}{N_r} = \frac{11,772}{4} = 2,943 \text{ N} \end{equation*}$

Using momentum theory, the power required per rotor to sustain hover is

(196) $\begin{equation*} P_{r} = \left( \frac{1}{FM} \right) \frac{T_{r}^{3/2}}{\sqrt{2 \varrho \, A_r}} \end{equation*}$

where the rotor disk area is

(197) $\begin{equation*} A_r = \pi R^2 = \pi (1.3)^2 = 5.31 \text{ m}^2 \end{equation*}$

The figure of merit, $FM$ , is assumed to be 0.6, which is a realistic rotor efficiency for smaller-scale rotors operating at lower tip Reynolds numbers.

Substituting the numerical values gives

(198) $\begin{equation*} P_{r} = \left( \frac{1}{0.6} \right) \frac{(2,943)^{3/2}}{\sqrt{2 \times 1.225 \times 5.31}} = \frac{42}{0.6} = 70 \text{ kW} \end{equation*}$

Therefore, the total power required to is

(199) $\begin{equation*} P_h = N_r \, P_r = 4 \times 70.0 = 280.0 \text{ kW} \end{equation*}$

The total energy consumption for flight can be estimated based on energy use and anticipated flight time in hover and cruise, i.e.,

(200) $\begin{equation*} E = \left(P_h \times \frac{t_h}{60}\right) + \left(P_c \times \frac{t_c}{60}\right) = \left(280 \times \frac{10}{60}\right) + \left(50 \times \frac{35}{60}\right) = 75.83 \text{ kWh} \end{equation*}$

where $P_c$ is the estimated power in cruising flight, which is typically about a fifth of the hover power when in wing-borne flight. A more detailed analysis of $P_c$ would include an estimate from induced and prasitic drag components.

For a battery with an energy density of 250 Wh/kg ( $0.25$ kWh/kg), then

(201) $\begin{equation*} M_{\text{bat}} = \frac{E}{\text{Battery energy density}} = \frac{75.83}{0.25} = 303.3 \text{ kg} \end{equation*}$

Therefore, at least a 300 kg battery pack will be required for this 45-minute eVTOL mission. With an empty weight of 600 kg, two passengers of 150 kg each, and a 300 kg battery pack, the manufacturer’s estimated maximum gross takeoff weight of 1,200 kg seems reasonable.

However, additional battery energy requirements will arise because of inefficiencies and the need for energy reserves for contingencies. Battery inefficiencies include energy lost to heat and internal resistance, reducing overall system efficiency by 70% to 80%. Additionally, energy reserves for safety must be considered, such as adverse weather, rerouting, or emergencies. Therefore, the expected cruise time of 35 minutes is unlikely to be achieved, a more realistic total flight time for these parameters being about 20 minutes. Not much can be accomplished in terms of useful transportation goals in this short time.

To improve flight time and efficiency, next-generation eVTOLs will need higher energy-density batteries, such as solid-state or lithium-sulfur technologies, as well as hybrid-electric power systems, including hydrogen fuel cells. Innovations in solid-state batteries and rapid charging systems are crucial for making eVTOLs viable for commercial operations. Vehicle design optimization may reduce aerodynamic drag and improve efficiency. Regenerative energy recovery systems may help capture energy during descent, increasing overall system efficiency. Therefore, it would seem that eVTOLs are not yet poised to transform urban transportation. It seems clear that many challenges remain in improving energy density, infrastructure, and airspace integration. However, rapid progress in automation, fast-charging battery technology, and materials science may bring the dream of practical electric air taxis closer to reality.

Summary & Closure

The factors influencing the characteristics and performance of helicopters have been extensively reviewed. The simple momentum theory has been applied to evaluate rotor performance in various flight conditions, including hover, climb, descent, and forward flight. A key parameter in rotor efficiency is disk loading, where lower values contribute to improved hovering performance by reducing power requirements. Additional design and comparison metrics, such as power loading and figure of merit, were introduced to quantify rotor efficiency. The essential momentum theory was refined to account for non-ideal effects, improving agreement with experimental measurements.

Momentum theory was also extended to coaxial rotors and to forward flight, where numerical solutions are required to determine the inflow through the rotor disk. The fundamental performance characteristics of helicopters in forward flight were analyzed, including factors that impose limitations on speed and efficiency. In contrast to helicopters, VTOL jets generate vertical thrust to achieve lift-off, relying entirely on engine jet thrust. Unlike helicopters, which achieve efficient lift through large rotors operating at low disk loading, VTOL jets operate with high power loading, leading to greater fuel consumption in hover. Consequently, jet-powered VTOL aircraft, such as the Harrier and F-35B, must transition forward quickly to have enough fuel to perform a useful mission profile. Ultimately, both rotorcraft and jet-powered VTOL aircraft are constrained by the fundamental trade-off between hover efficiency and forward-flight performance, necessitating innovative design choices to optimize operational effectiveness.

5-Question Self-Assessment Quickquiz

For Further Thought or Discussion

The history of airplanes shows that growth (in terms of the number of prototypes being developed) was rapid and monotonic. However, while the evolution of rotorcraft (helicopters) started earlier, it progressed more in a series of discrete events called “fits and starts.” Discuss this point further, assuming it is associated with technological developments.
Briefly overview the leading technical problems encountered when building and flying a helicopter before 1900. In your discussion, consider overall levels of aeronautical knowledge, stability and control requirements, the availability of suitable engines, construction materials, and anything else you feel is relevant.
Engines are often viewed as one of the most critical enabling technologies for powered flight, especially for helicopters. However, having a readily available supply of suitably formulated and inexpensive fuel for a piston engine (i.e., gasoline) was the critical enabling technology. Discuss this viewpoint.
Most modern helicopters have a single main rotor and tail rotor (conventional) configurations. For the same overall aircraft gross weight, what might be the relative advantages of using a tandem rotor helicopter over a conventional helicopter? Also, compare the potential relative merits of a coaxial rotor and a side-by-side rotor configuration over a tandem rotor design.
Discuss the physical and other features distinguishing a helicopter from an autogiro. Discuss also the reasons why the autogiro was quickly eclipsed by the success of the helicopter when it appeared in the mid to late 1930s. Are there prospects for the autogiro or gyroplane in the future?
Although tiltrotor concepts date back more than sixty years, it is only recently that civilian tiltrotor aircraft have completed much of the flight testing toward civil certification, i.e., the AW-609. Discuss the technical, economic, and other reasons for this long gestation period. Consider also the prospects that you may fly on a tilt-rotor operated by an airline within the next decade.
Use conservation of mass and momentum, but use Bernoulli’s equation instead of the general energy equation to prove that the induced velocity ${w}$ in a climbing rotor’s fully contracted wake (slipstream) is twice the induced velocity in the rotor plane.

Other Useful Online Resources

For additional resources on helicopters and other rotorcraft, follow up on some of these online resources:

A great Shell Oil film on the History of the Helicopter.
Early film. How it Works: Sikorsky H-19 Helicopter.
History Of Helicopters – Military Helicopter Invention Documentary.
A great movie on the birth of Bell Helicopters.
A video showing the details of the swashplate.
Video on the top 7 U.S. military helicopters of all time!
Your first helicopter lesson in a Robinson R-44!
The massive Sikorsky CH-53K helicopter in action!
Bell 206 JetRanger helicopter review + how to fly a helicopter.
MD 520N NOTAR helicopter review and flight test.

The author is indebted to his teachers, Professor Alfred Gessow, who was an American pioneer in the field of helicopter aerodynamics and co-author of the book "Aerodynamics of the Helicopter," and F. John Perry, Chief Aerodynamicist for Westland Helicopters. ↵
Juan de la Cierva tragically died in an airplane accident on December 9, 1936. He was a passenger on a KLM Douglas DC-2 that crashed shortly after takeoff from Croydon Airport, London. ↵
A reasonable assumption in practice, but this particular assumption is still needed to formalize the minimum loss condition. ↵
Recall that in the ideal case, the performance of the upper rotor is assumed to be unaffected by the lower rotor. ↵
The power measurements are only accurate to about 7%. ↵
To reduce energy consumption, many eVTOLs incorporate tilt-rotor and various forms of lift+cruise designs. ↵

License

Icon for the Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License

Introduction to Aerospace Flight Vehicles Copyright © 2022–2025 by J. Gordon Leishman is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License, except where otherwise noted.

Digital Object Identifier (DOI)

https://doi.org/https://doi.org/10.15394/eaglepub.2022.1066.9

Introduction[1]

History

Helicopter Configurations

Basis of Helicopter Flight

Rotor Flow Environment

Asymmetry of Aerodynamics

Blade Flapping

Leading & Lagging

Blade Pitch

Rotor Wake & Blade Tip Vortices

Hovering Flight Analysis

Time-Averaged Flow Field

Flow Model

Application of the Conservation Principles

Induced Velocity

Power Required to Hover

Pressure Variations

Disk Loading & Power Loading

Non-Dimensional Hovering Analysis

Measured Rotor Performance

Non-Ideal Effects

Induced Effects

Profile Drag Effects

Modified Theory Versus Measurements

Figure of Merit

Solidity & Blade Loading Coefficient

Ground Effect

Axial Climbing & Descending Flight

Climbing Flight

Descending Flight

Induced Velocity Curves

Power Required Curves

Vortex Ring State

Autorotation in Vertical Flight

Hovering Coaxial Rotor

Corotation in the Same Plane

Equal Thrusts

Balanced Torques

Lower Rotor in the Slipstream of the Upper Rotor

Equal Thrusts

Balanced Torques

Validity of the Coaxial Theory

Forward Flight Analysis

Flow Environment

Momentum Theory Analysis in Forward Flight

Mass Flow Rate

Thrust

Power Required

Inflow

Power Requirements in Forward Flight

Forward Flight Performance

Induced Power

Profile Power

Parasitic Power

Tail Rotor Power

Total Power

Comparison with In-Flight Measurements

Reverse Flow Region

Effects of Weight

Effects of Density Altitude

Climb Performance

Fuel Flows

Range & Endurance

Coaxial Rotor in Forward Flight

Controlling the Helicopter

Main Rotor

Tail Rotors

Types of Tail Rotors

Other Effects

Design Features

Autorotation

Energy Analysis

Autorotative Rate of Descent

Vertical Autorotation

Autorotation in Forward Flight

Summary of the Autorotational Maneuver

Rotor Design for Autorotational Capability

Initial Energy State (Height-Velocity Diagram)

Autorotative Index

Limitations of the Helicopter

Introduction^[1]