43 Aircraft Propellers
Introduction
The modern propeller stands as a testament to the achievements of aeronautical engineering. Since the earliest days of flight, propellers have been the focus of continuous refinements and technological advancements, further shaping the evolution of aircraft designs. Today, companies such as Hartzell, Sensenich, and McCauley produce highly efficient, advanced propellers made of composite materials, aerodynamically optimized airfoil sections, and sophisticated blade geometries with swept leading edges to minimize compressibility effects. These modern designs, exemplified by the photograph below, reflect more than a century of progress in aerodynamics, materials science, and manufacturing techniques, closely paralleling and often driving broader innovations in aviation.

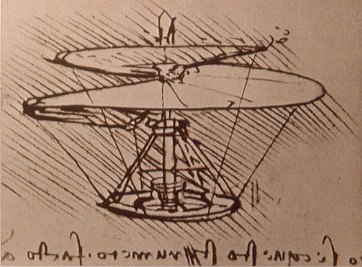
The earliest known use of propellers or rotors dates back to around 500 BC in ancient China, where children played with bamboo dragonflies. These were simple wooden toys consisting of a stick and blades that, when spun rapidly between the hands, would lift into the air. Leonardo da Vinci, in the 1480s, sketched the “aerial screw,” as shown in the figure below. This concept was an aerodynamic device derived from an Archimedes water screw, which some consider a precursor to the modern helicopter rotor. Although never built at its intended human-carrying scale,[1] Da Vinci’s design laid down the concept of using rotating wings to create an aerodynamic force parallel to their axis of rotation, a force called thrust.
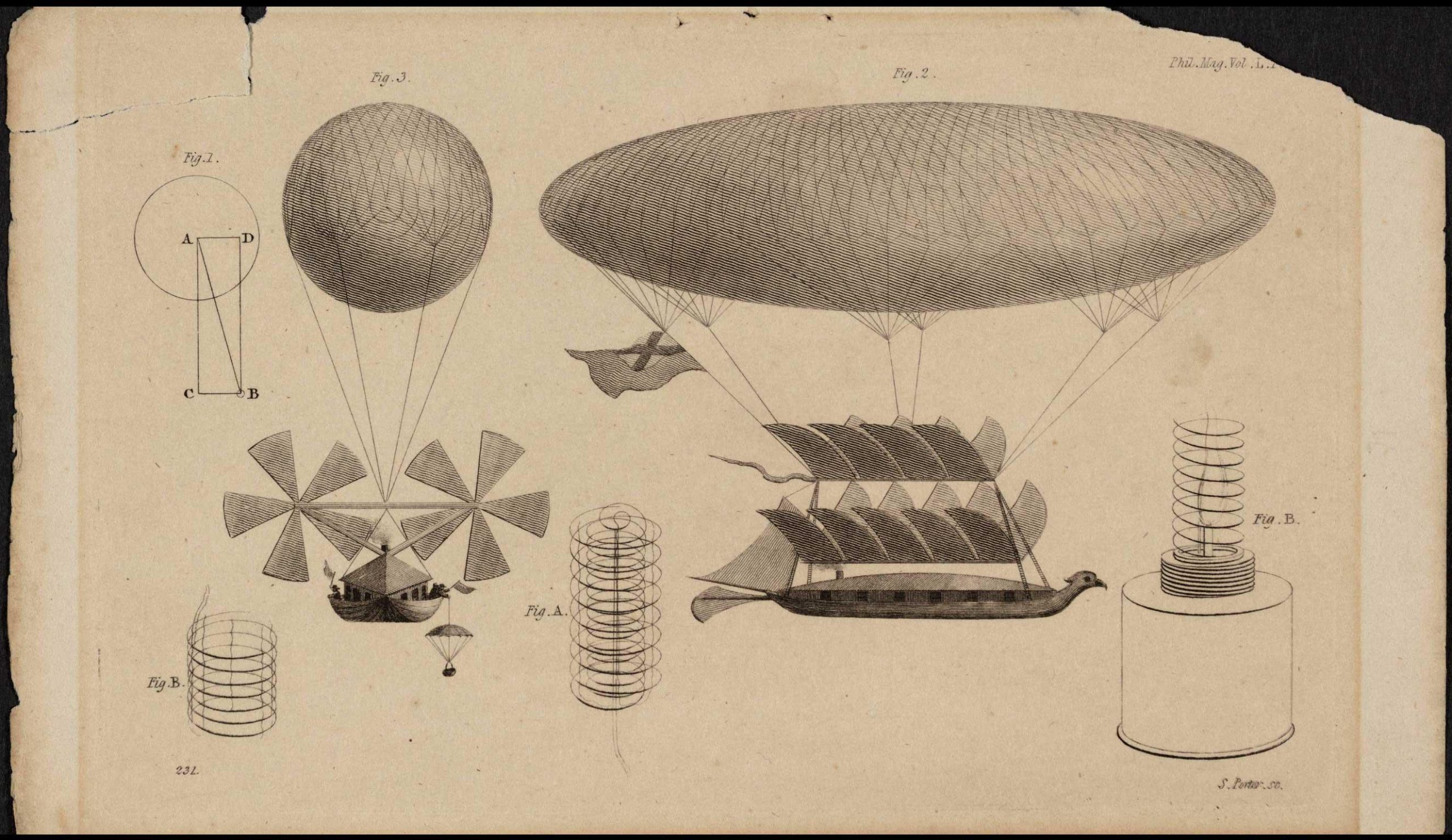
In the 19th century, George Cayley made significant contributions to understanding the principles of flight, experimenting with gliders and recognizing the need for a powerplant and propulsion mechanism if sustained flight was ever to be achieved. At least one of his airplane concepts and an airship, the latter shown in the illustration below, indicated that the means of propulsion was using “airscrews” or propellers. The power source was not specified, although the only option at the time would have been a steam engine.[2] The use of “screw” propellers for propulsion was nothing new, having been used in marine applications since the 1830s. Still, the propeller blade designs suitable for good efficiency in the air had yet to be determined. In the 1870s, Thomas Moy built the Aerial Steamer, an early powered aircraft with two, six-bladed propellers driven by a steam engine. The blades had geometric twist, their pitch becoming finer at the blade tips. Like the Cayley concept, however, although it did not fly, it was a step forward in critical thinking about how an airplane might be propelled.
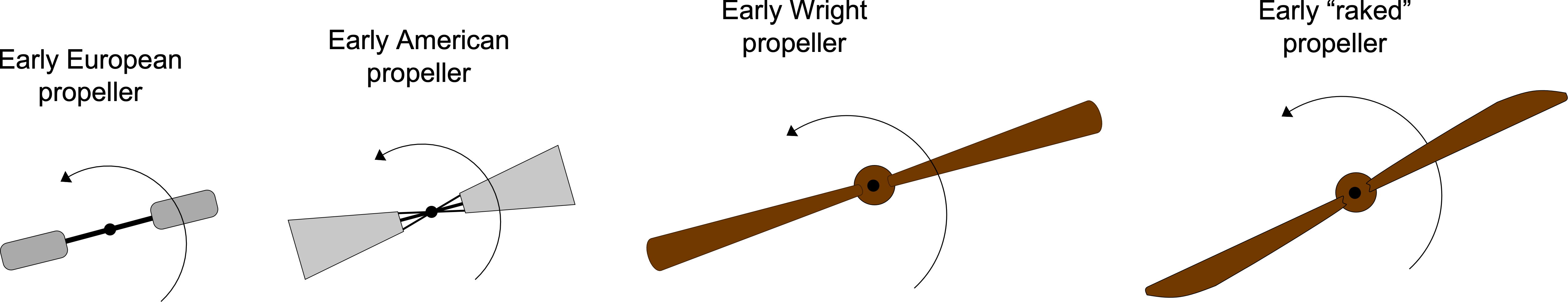
In 1903, the Wright brothers made the first successful, powered flight with their Wright Flyer, equipped with twin wooden propellers designed and built in their workshop. The Wrights discovered that there was no theory or other analysis to describe or predict propeller performance. The state of the art at the time was simply trial and error. They then set out to study the aerodynamics of propellers in a wind tunnel of their own design, demonstrating that long and thin (i.e., high aspect ratio) blades with a nose-down twist (called washout) along their span yielded the best propulsive efficiency. By 1908, they had improved their propeller designs for the Wright Flyer III, enabling flights of much longer duration and range. Their “raked” propeller had a swept leading edge and more blade area toward the tip, as shown in the figure below. This design gave further efficiency improvements, allowing more of the limited engine power to be converted into thrust. The broader propeller tips were also structurally stiffer, preventing them from buzzing and fluttering, a shortcoming of many early propeller designs.
During WWI, propeller technology advanced rapidly in response to the demand for airplanes with greater performance. Initially, fixed-pitch wooden propellers carved from laminated spruce blocks were standard, as shown in the photograph below. However, as engine power and aircraft speeds increased, the limitations of wooden propellers quickly became evident, including cracking and delamination. They were also very vulnerable to damage in combat, with a single bullet through a wooden blade often causing its catastrophic failure.

While wood was easy to work with and, with sufficient skill, propellers could be carved to almost any shape, the quest for more durable propellers led to metal blades, initially made of steel. Steel offered significantly greater strength and resistance to damage, although it introduced other challenges, such as increased weight and the need for more complex and expensive manufacturing processes. One of the earliest airplanes using steel propellers was the Handley Page O/400 bomber, which significantly improved its flight performance and reduced its vulnerability in the demanding conditions of long-range bombing missions under enemy fire.
With the formation of dedicated research institutions, such as the NACA and the Royal Aircraft Establishment (RAE), the aerodynamic principles governing propeller performance began to be systematically quantified. From the 1920s to the 1950s, NACA conducted wind tunnel tests and collated detailed data on propeller performance. Engineers experimented with various blade planforms, including tapered and hyperbolic shapes, as well as specific tip shapes and airfoil sections that were better suited for propellers. The RAE also researched propellers extensively, focusing on theoretical aerodynamic analyses, mainly by Hermann Glauert[3], supported also by wind tunnel measurements. These propeller developments laid the groundwork for more sophisticated airplane designs, contributing to the rapid evolution of aviation technology.
Materials like duralumin, an aluminum alloy invented in the mid-1920s, were used to make propellers lighter and stronger. Engineers soon began developing variable-pitch aluminum propellers, allowing pilots to adjust the blade pitch angles for optimal flight performance. This significantly improved propeller efficiency and airplane performance over a broader range of airspeeds. In the 1930s, Hamilton Standard introduced the first controllable pitch propeller. During takeoff and climbout, the pilot could use a lower blade pitch for optimal performance and then shift to a higher blade pitch for efficient cruising flight. This innovation soon led to the constant-speed propeller, which automatically adjusted the blade pitch during flight using a governor[4] to maintain a constant rotational speed and continuously maximize the propeller’s propulsive efficiency.

During WWII, propeller-driven aircraft technology reached new heights, with significant advancements in propeller design, aerodynamics, engine efficiency, structural reliability, and overall weight reduction. The introduction of propellers with more blades was another crucial development. Initially, two-blade and three-blade propellers were standard, but engineers soon realized that four-blade and five-blade designs were needed to absorb the power from increasingly powerful piston engines. Additional blades also provided smoother operation, reducing vibrations from the engine and propeller, and improving the airplane’s overall performance at higher airspeeds. As engine power levels increased even further after the advent of supercharging and turbocharging, counter-rotating tandem propellers were used to prevent the size of a single propeller from becoming prohibitively large, thereby absorbing the available power.
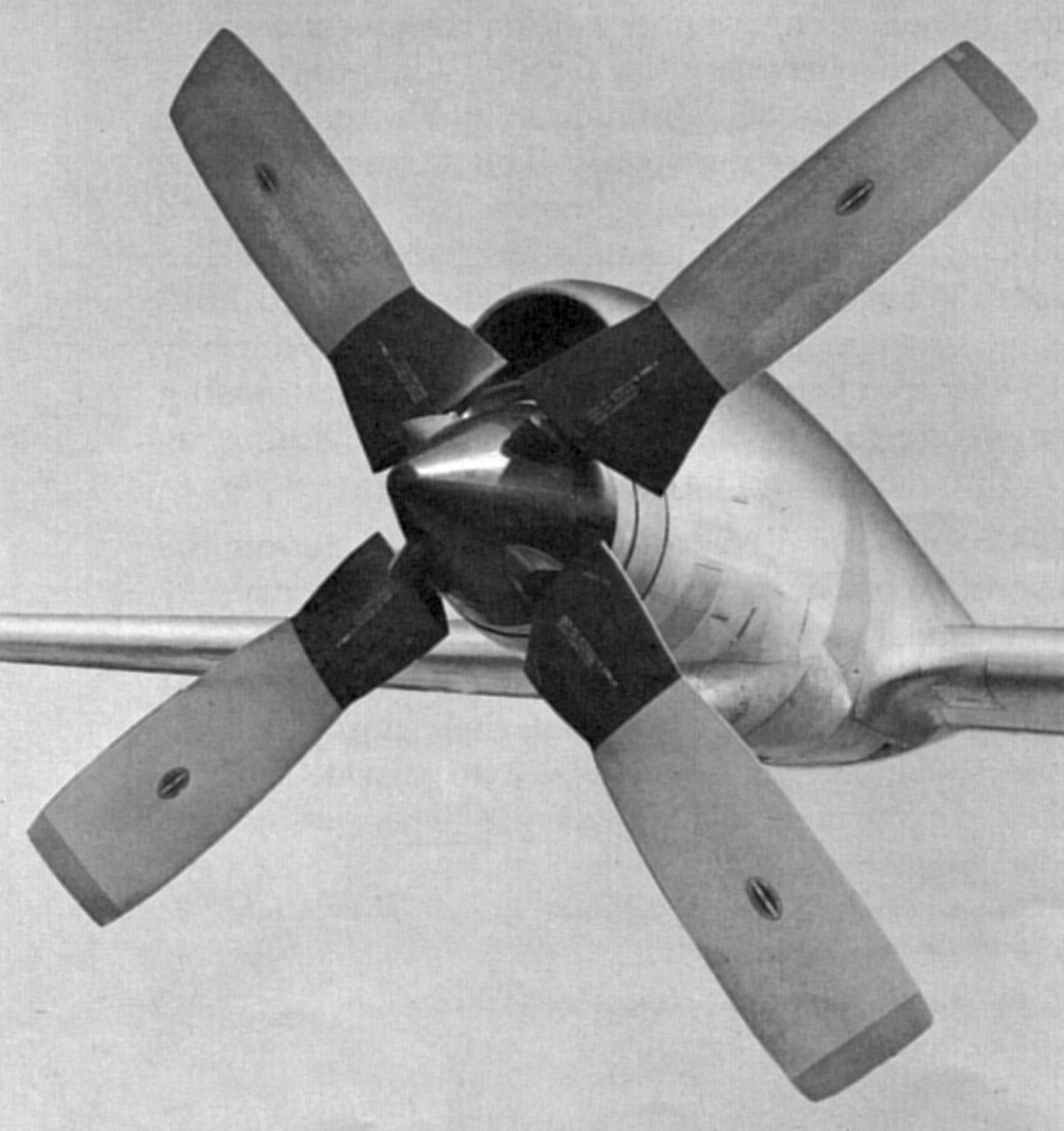
Iconic WWII aircraft, such as the British Supermarine Spitfire and the American North American P-51 Mustang, exemplified advancements in propeller and engine technology. The Spitfire, with its powerful Rolls-Royce Merlin engine and Rotol four-bladed aluminum propeller design, as shown in the photograph below, achieved exceptional performance in terms of achievable airspeeds and operational altitude. Later models of the Spitfires were equipped with a five-bladed propeller powered by a version of the Rolls-Royce Griffon engine, representing the pinnacle of piston-engine and propeller performance. The P-51 Mustang, when fitted with a four-bladed Hamilton Standard propeller, became renowned for its long flight range, speed, and effectiveness as an escort fighter.

These technological improvements with propellers were also applied to bombers and transport aircraft, such as the Boeing B-17 Flying Fortress, the Consolidated B-24 Liberator, the Avro Lancaster, and the Douglas C-47. Propellers played a crucial role in improving the speed, altitude, and range of these aircraft, ultimately contributing to the Allied victory in WWII. The increased efficiency and reliability of propeller-driven airplanes allowed for more effective long-range bombing campaigns, strategic airlifts, and supply missions. Additionally, advancements in aerodynamics and engine integration enabled these aircraft to carry heavier payloads while maintaining maneuverability and endurance in combat operations.
The transition to using gas turbine (turbojet) engines after WWII soon surpassed the use of propellers in most military and commercial aviation. Nevertheless, propeller technology continued to evolve for specific applications, including high-speed fighter airplanes. However, the most significant interest in new propeller developments was for turboprop engines, which utilized a turboshaft engine to drive a propeller, offering high propulsive efficiency at lower speeds and altitudes than pure jet engines. This advancement made turboprop “jet” airliners ideal for shorter routes where fuel efficiency and short takeoff and landing capabilities, such as at regional airports, were critical.
The Lockheed L-188 Electra, which entered service in 1958, demonstrated the enduring significance of propeller-driven airplanes; one of its four-bladed propellers is shown in the photograph below. Airplanes like the Lockheed C-130 Hercules, the modern Airbus A400M Atlas, and the C-130J Super Hercules have since leveraged advances in turboprop technology to improve their efficiency, versatility, and reliability. Today, the turboprop remains a preferred propulsion system for many airplanes and can be found on high-performance single-engine aircraft, such as the Pilatus PC-12 and the Cessna Caravan 208.
While the basic engineering principles used for aircraft propeller design have remained the same for decades, numerous detailed improvements have led to continuous gains in propulsive efficiency and operational reliability. Propeller construction methods have continued to advance, utilizing carbon and graphite composites, which provide lighter and more aerodynamically efficient blades with reduced noise. Engineers continue to utilize techniques such as computational fluid dynamics (CFD) and computer-aided design (CAD) to further optimize propeller performance, tailoring them to specific engines, aircraft, and flight conditions.
Most modern propellers feature swept leading edges and thin airfoil sections, as illustrated in the photograph below. Scimitar propellers, which have highly swept blades made primarily of composite materials, were developed in the 1980s. Like the swept wings used for high-speed aircraft, these propeller blades delay the onset of drag rise and the creation of wave drag, allowing them to remain efficient propulsors at higher flight speeds and flight Mach numbers. They also reduce noise, a significant concern for all modern aircraft because of the increasingly stringent noise standards set by the ICAO. This type of swept-blade propeller design is used in many modern turboprop airplanes, such as the ATR 72, the Bombardier Dash 8, and the Pilatus PC-12.

Ongoing developments in propeller designs suited for electrically powered airplanes are focused on improving efficiency and reducing noise, particularly for urban air mobility (UAM) and unoccupied aerial vehicles (UAVs). Engineers are exploring innovative blade geometries and lightweight materials to create quiet, compact propeller systems that meet the unique demands of drones, electric airplanes, and eVTOL concepts. The goals include optimizing flight performance using multi-rotor systems and variable-pitch propellers while leveraging improvements in battery technology and manufacturing techniques. While the tipping point in favor of electric propulsion over traditional approaches is likely decades away, technology developments are progressing rapidly.
Learning Objectives
- Have an appreciation of the historical developments of propeller technology from the days of the Wright brothers to the present.
- Understand the basic operational principles of a propeller, including the methods used to calculate its thrust, the power required, and its efficiency.
- Be familiar with momentum theory and blade element theory when calculating the performance of a propeller.
- Know how to read and interpret a propeller performance chart.
- Understand the operational advantages of variable-pitch propellers compared to fixed-pitch designs.
- Know how to select a propeller to meet a performance requirement, including for low-Reynolds-number propellers.
- Appreciate the differences between propellers and hybrid designs such as proprotors, which are used for both vertical lift and horizontal propulsion.
Propeller Fundamentals
Propellers convert rotational motion into thrust by creating aerodynamic lift forces on their blades, which act as rotating wings. As the blade moves through the air, the airfoil shapes create an aerodynamic pressure difference between the upper and lower surfaces, resulting in lift production on the blades. The resolved forward component of the lift vectors is known as the propeller’s thrust. The engine supplies a torque to the propeller shaft, which in turn overcomes the drag forces on the blades and maintains their rotation. Sedulous propeller design maximizes thrust by optimizing blade geometry, angle of attack distribution, and rotational speed, ensuring that the engine’s power is converted into propulsive force with maximum efficiency.
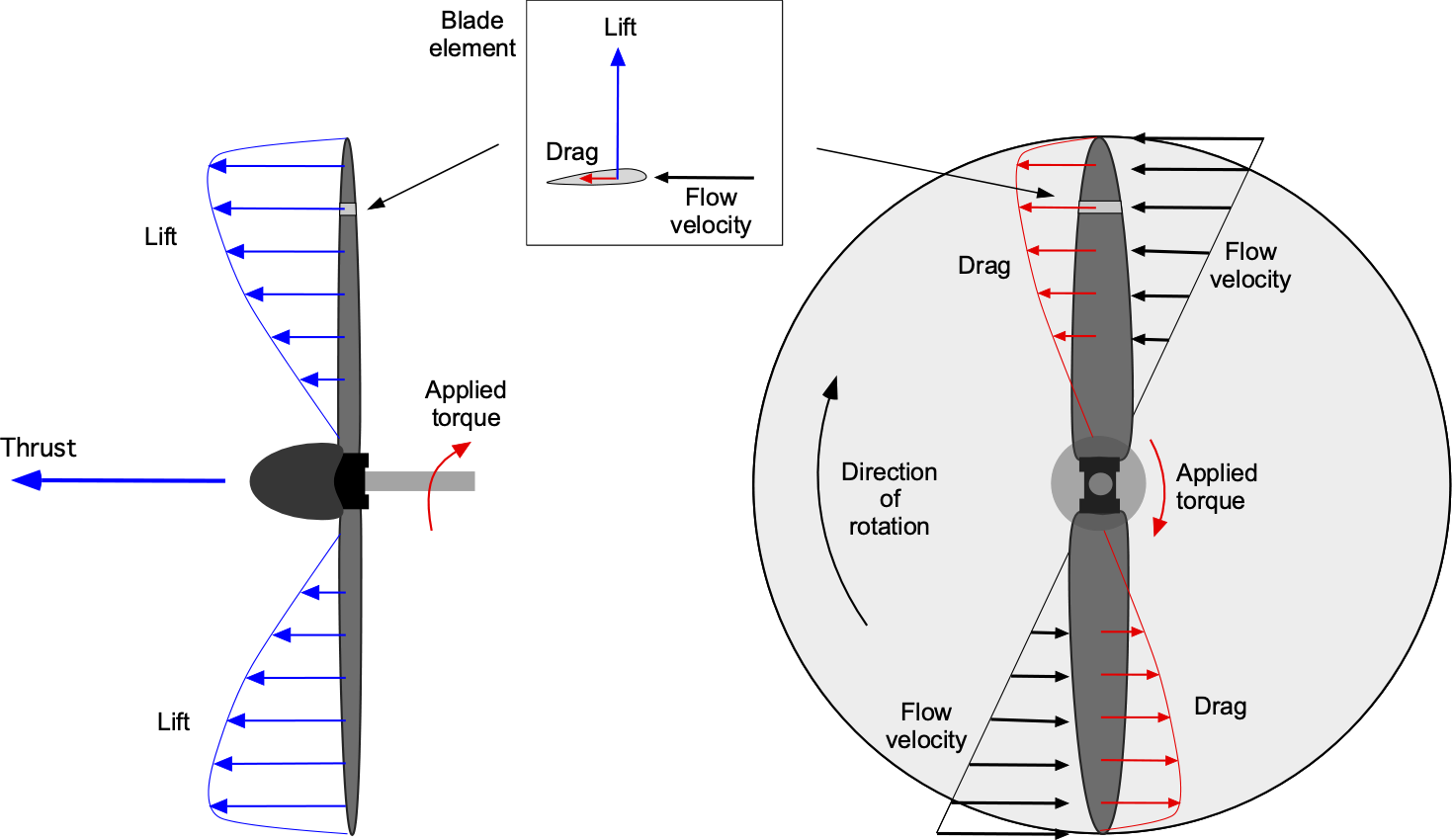
Velocity Triangle & Blade Twist
A notable design feature of propeller blades is that they are significantly twisted along their span, i.e., they have geometric washout. Each blade is composed of an airfoil section, and because of the variation of flow velocity along the blade, the twist ensures that the local angle of attack at each section remains near its most aerodynamically efficient value across the entire blade span. In practice, most sections should have angles of attack between about and
, or otherwise near their angle of attack for the peak lift-to-drag ratio, i.e.,
. The result is maximum thrust production for minimum torque and shaft power.
To understand why a propeller blade must be significantly twisted, consider the following analysis. Let be the radius of the propeller and let
be the spanwise distance from the axis of rotation to a blade element, as shown in the figure below. The corresponding nondimensional radius is
, and the root cutout, which is aerodynamically passive, is
. The angular velocity is
, and the tangential (in-plane) velocity increases linearly with
, i.e.,
. The local geometric blade pitch angle,
, is defined relative to the plane of rotation.
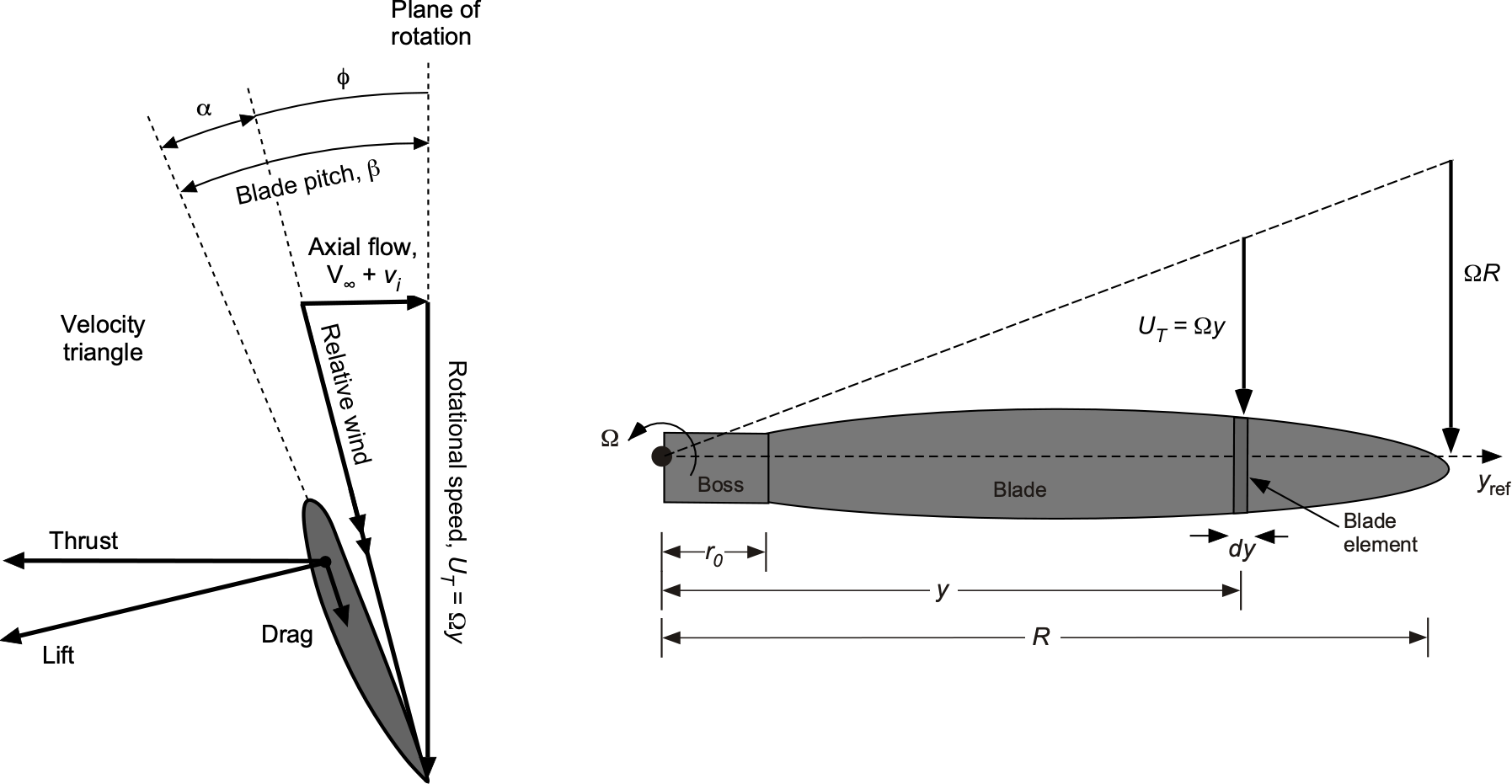
Assume that the axial velocity through the propeller disk is uniform, such that
. The total relative velocity (also known as the relative wind) at the blade section
is then
(1)
The inflow angle is
(2)
and so the sectional angle of attack is
(3)
Notice that the axial velocity is the sum of the freestream velocity (airspeed) and the induced velocity
, i.e.,
(4)
For a propeller in its normal working state during flight, , whereas for static thrust (hover)
, so that
. In either case,
is assumed to be uniform across the propeller disk, consistent with the Rankine-Froude actuator disk assumption, which is also a design goal to minimize losses.
Under the assumption of a constant aerodynamic angle of attack, i.e., , it follows that
(5)
which suggests a canonical form for the twist distribution, i.e.,
(6)
because is a constant. This expression shows that
must follow a hyperbolic variation proportional to
, i.e., in general
(7)
as shown in the figure below. The twist angle is larger near the root and decreases toward the tip, thereby ensuring a constant angle of attack along the blade span. However, because of the variations in Mach number, Reynolds number, section thickness-to-chord ratio, and camber (i.e., zero-lift angle of attack, ), the best angle of attack is not necessarily a constant. The net twist will vary for different propellers based on their intended application, but the net washout angles may be as large as 40 degrees over the blade span; of course, these twist angles are significantly larger than those used on airplane wings.
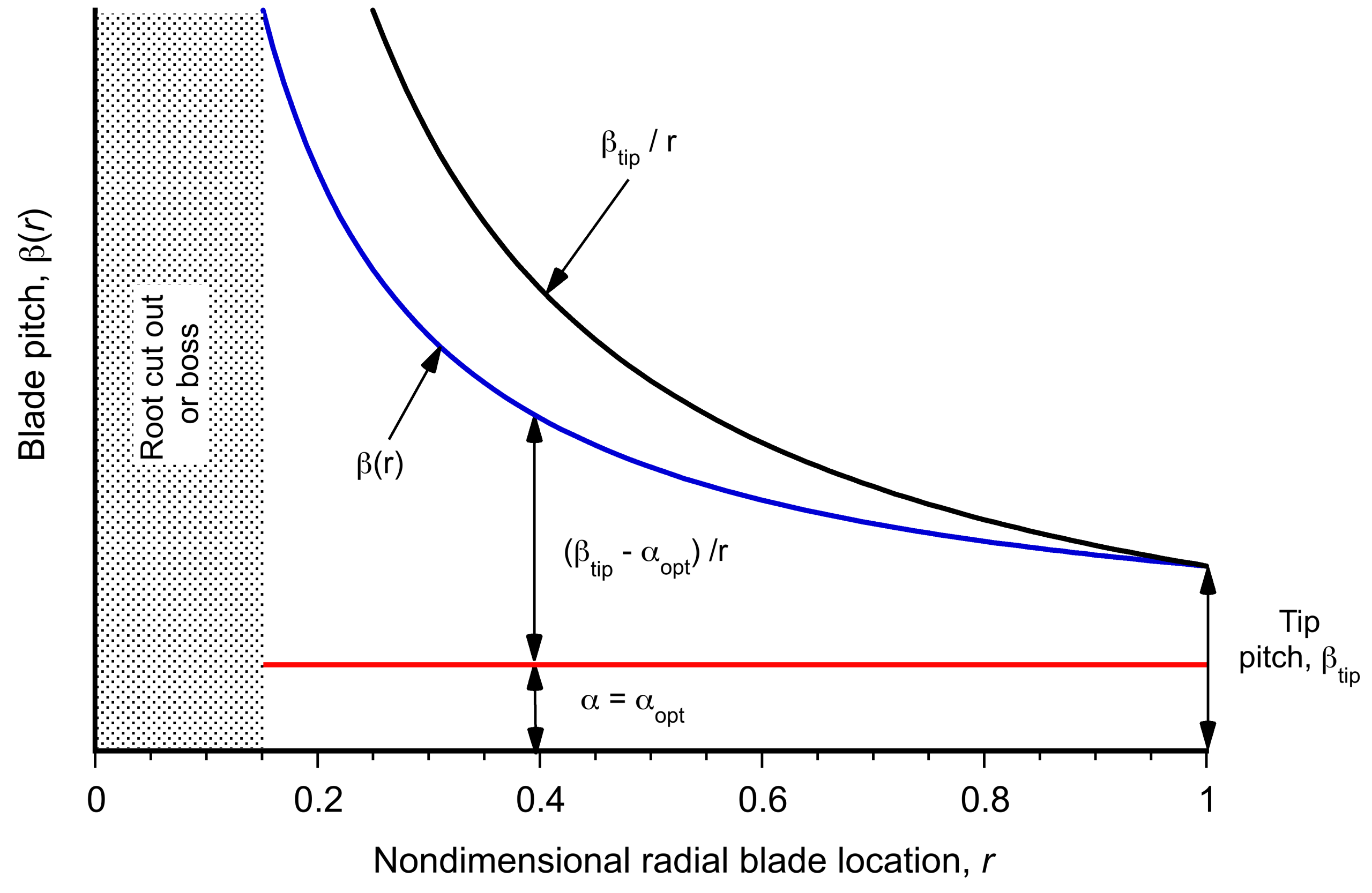
Fixed-Pitch Versus Variable Pitch
Fixed-pitch propellers have a narrow operating envelope; they are either suitable for takeoff and climb but perform poorly in cruise, or they are suitable for cruise but perform poorly in takeoff and climb. This is because at higher airspeeds, the blade section angles of attack on a propeller of fixed pitch will become progressively smaller, so the propeller produces progressively less thrust. The solution to this dilemma is to change the average blade pitch angles[5] to maintain or increase thrust production and allow the efficient operation of the propeller to be optimized as a function of the flight condition, the idea being shown in the figure below.
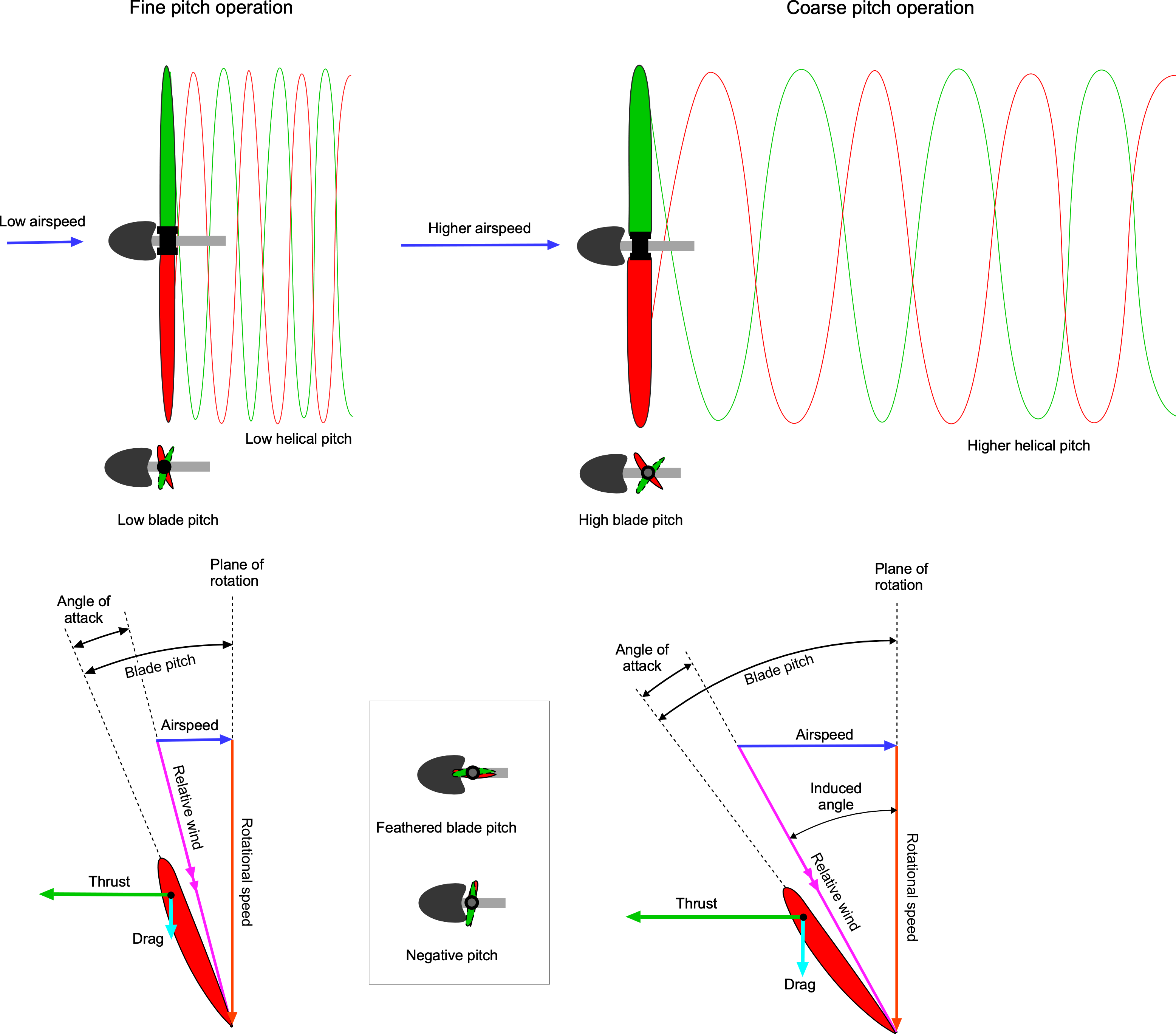
To maximize thrust and propulsive efficiency, a fine (low) blade pitch will be used for takeoff and landing, and a coarser (higher) blade pitch will be used for cruise flight. Regulating the pitch angles of the blades in this manner optimizes the propeller’s efficient operation as a function of the flight condition. Therefore, an aircraft equipped with a variable-pitch or constant-speed propeller can maintain good levels of thrust and propulsive efficiency over a broader range of airspeeds. In cruise flight, a speed governor can automatically adjust the blade pitch to maintain the constant rotational speed of the propeller, thereby offloading much of the pilot’s workload of controlling the propeller pitch.
The highest thrust from the propeller will be limited by the onset of stall, just as a regular wing is limited in its angle of attack capability by stall. However, because the local incident Mach number varies from the root to the tip of the propeller, and the stall angle of attack of an airfoil decreases with increasing Mach number, the allowable angle of attack before stall decreases from the root to the tip. To this end, thin airfoil sections with better lift-to-drag ratios and higher drag divergence Mach numbers are used. However, the onset of stall will eventually occur if the blade pitch is increased too much, accompanied by a loss of thrust, an increase in power, and a marked drop in propulsive efficiency.
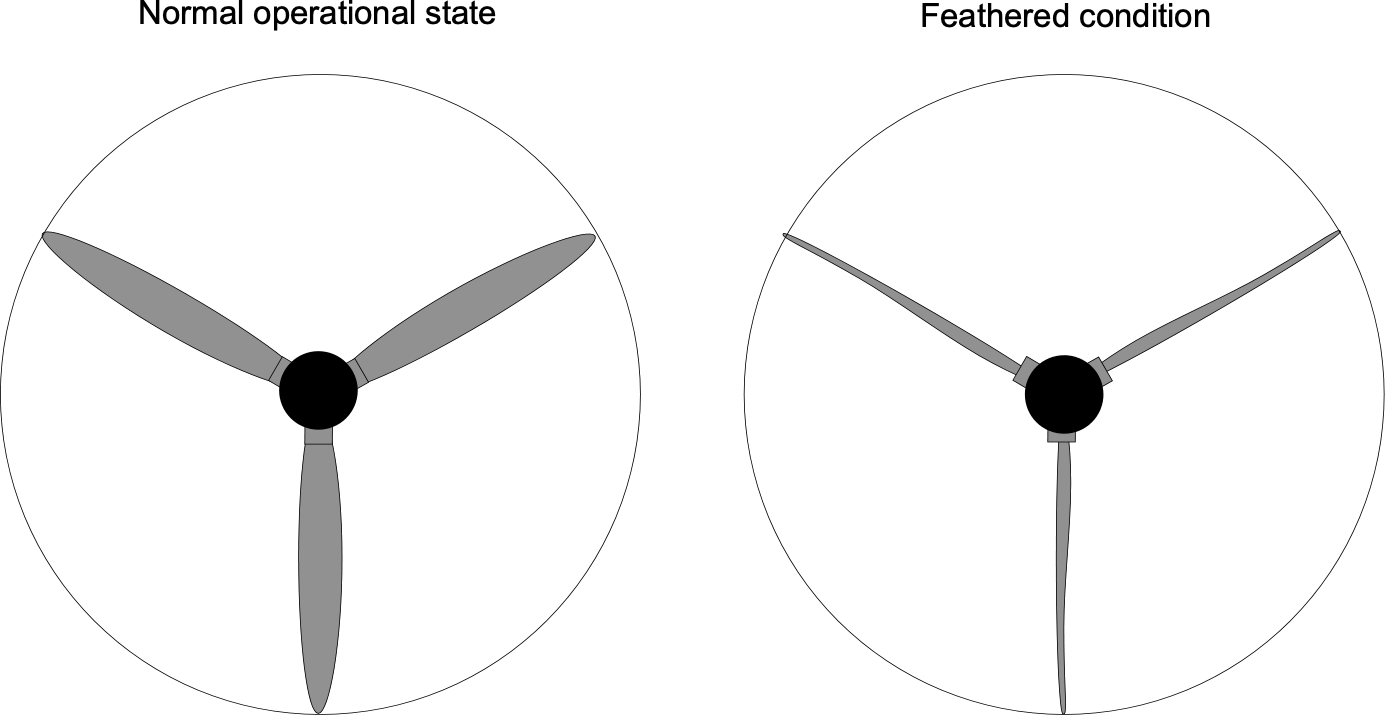
If the engine stops in flight, the propeller blades must be “feathered” into the airflow to reduce the otherwise high drag of the stationary blades. This means the leading edges of the blades will point almost directly into the airflow, as shown in the figure below. In this regard, strong springs are used on a constant-speed propeller so that the blades are automatically forced into the fully feathered position if the propeller stops rotating. The drag of an unfeathered stationary propeller is considerable, so an airplane with a fixed-pitch propeller will inevitably have a poor glide ratio. The propeller blades may sometimes be set to a negative pitch to produce negative thrust, thereby acting as an aerodynamic brake during landing. However, this latter feature is usually only included in high-performance turboprop airplanes.
Types of Propellers
Many propeller types are in use, ranging from those with just two blades to those with four or more blades, some with fixed pitch and others with variable pitch, as shown in the photographs below. In addition, some propellers may have swept blades, a design feature used to reduce compressibility drag loss at the tips when the airplane operates at higher flight speeds, similar to the design used on the wings of supersonic airplanes. As engine power increases, more blades (or a greater blade area) are required to deliver the power to the air as useful thrust. In some cases, to prevent the propeller diameter from becoming too large, counter-rotating propellers may be used to absorb the high amounts of available power from the engine shaft to create thrust.
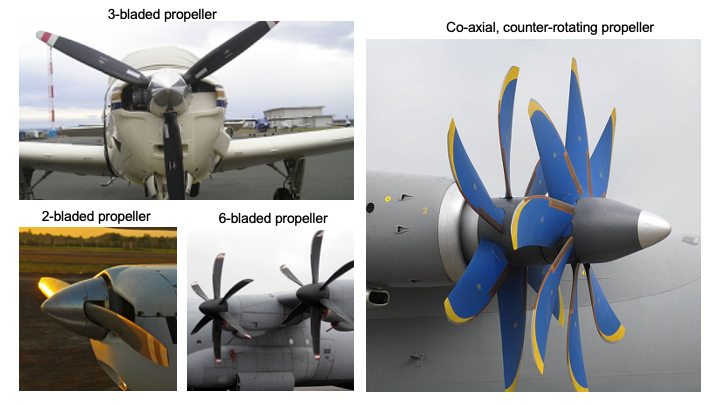
Propellers with larger numbers of blades also tend to be relatively more efficient (when compared based on the same thrust and total blade area) because they act increasingly like an actuator disk.[6] However, the propeller’s net efficiency depends on other factors, including operating speed (rpm or rps),[7] tip Mach number, blade section Reynolds numbers, diameter, and blade pitch. Today, there is an increasing emphasis on reducing propeller noise, which has driven engineers of modern designs (even on general aviation airplanes) to use more blades and smaller diameters with lower tip Mach numbers.
What is the difference between propeller power and torque?
Torque, given the symbol , is the rotational action applied to the propeller shaft, while angular velocity,
, is the speed at which the propeller rotates, measured in radians per second. Power,
, represents the rate at which energy is transferred and is given by the equation
. A high torque with low rotational speed results in lower power, whereas a lower torque with high rotational speed can still generate significant power. The key to efficient propeller performance is balancing
and
to achieve optimal thrust and efficiency across different flight conditions. For example, consider a propeller with high torque but lower rotational speed: if
= 50 Nm and
= 100 rad/s, then the power is
= 50
100 = 5000 W = 5 kW. Alternatively, if the propeller operates at lower torque but higher speed, say
= 25 Nm and
= 200 rad/s, the power remains the same at
= 25
200 = 5000 W = 5 kW.
Momentum Theory of the Single Propeller
The Rankine propeller theory,[8] also known as the Rankine-Froude theory,[9] provides a fundamental framework for understanding the performance of propellers. This theory models the propeller as an idealized actuator disk that imparts momentum to the air, creating a pressure jump across the disk. The physical problem is that the propeller does work on the air as it passes through the propeller disk, i.e., the propeller applies a force to the air in the downstream direction, changing the momentum and kinetic energy of the air. As a result, the force on the propeller, which is produced because of a pressure difference between one side of the propeller disk and the other, is in the opposite direction to the force on the fluid, i.e., the thrust is directed upstream.
Flow Model
The flow model for the single propeller problem is shown in the figure below. The upstream and downstream sections labeled 1 and 2 bound the control volume. Remember that when the integral form of the conservation equations is used, then points out of the control volume by convention. The upstream or freestream (undisturbed) velocity is
, and the pressure there is
. It will be assumed it is uniform, which is a reasonable assumption unless the propeller is affected by a wing or another part of the airframe. It will also be assumed that a one-dimensional, steady, incompressible flow applies throughout, so that the flow velocities change only with downstream distance.
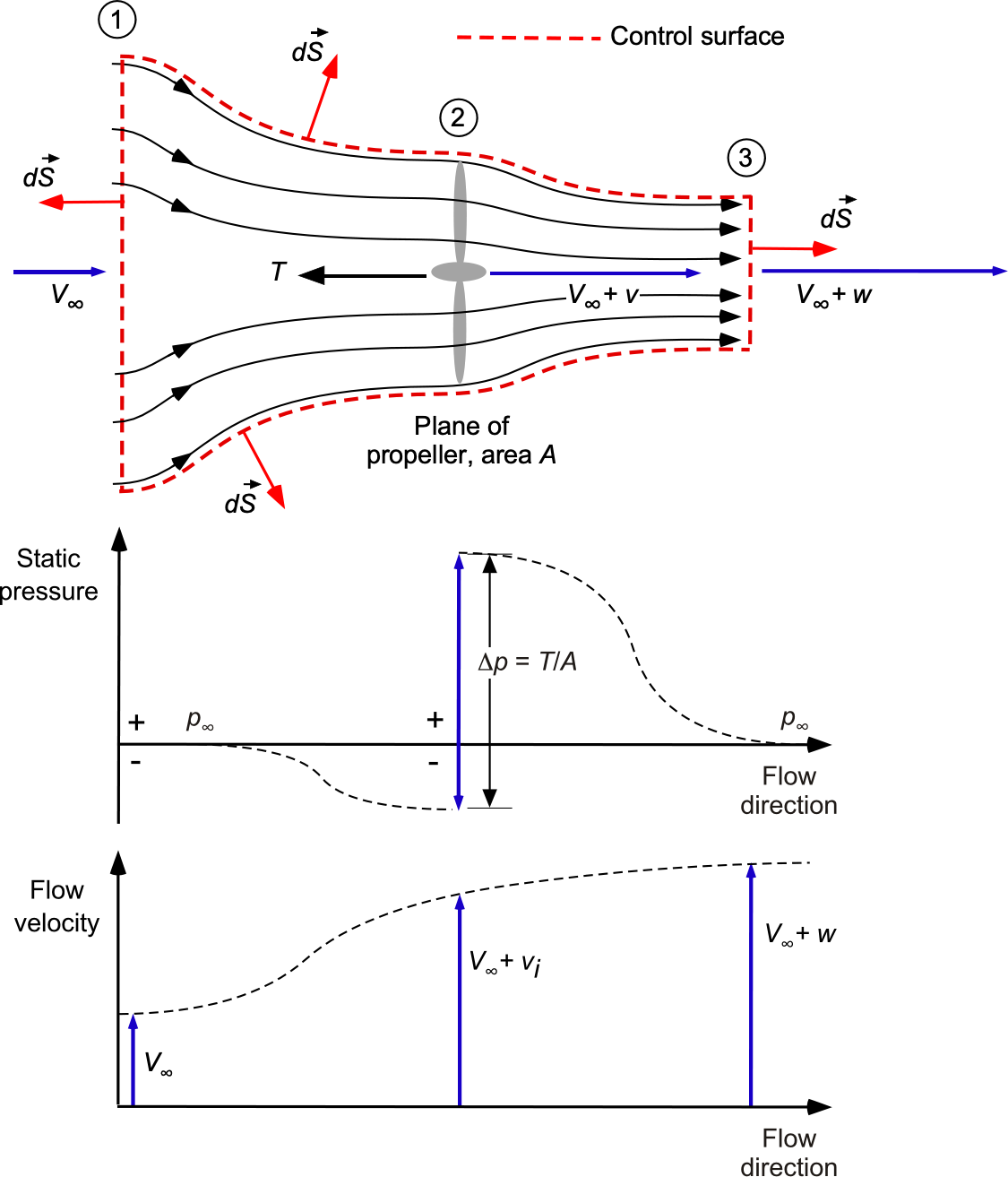
The flow is entrained and accelerated into the propeller, so at the plane of the propeller, the flow velocity is plus an increment
, i.e., the velocity there is
. The static pressure will also change there. The propeller works on the air to increase its momentum and kinetic energy, so the velocity downstream is
. In the slipstream, the static pressure will also recover to ambient conditions, i.e., the pressure downstream will slowly return to
.
Application of the Conservation Laws
The conservation of mass requires a constant mass flow through the control volume. The areas of the upstream and downstream parts of the streamtube (stations 1 and 2) are unknown, but the area of the propeller disk, , is known (
where
is the diameter of the propeller), so the mass flow rate through the propeller (and hence through the boundaries of the control volume) is
(8)
The conservation of momentum requires that the net change in momentum of the fluid (as applied by the propeller) is equal to the force on the fluid, i.e.,
(9)
where the direction of is downstream, and the thrust on the propeller,
, is in the opposite direction (pointing left in the figure) so that
(10)
The conservation of energy states that the work done on the propeller (to move it forward) plus the work done on the air (to create the aerodynamic force) must be equal to the gain in kinetic energy of the slipstream as it passes through the propeller, i.e.,
(11)
so that the power required for the propeller to produce the thrust
is
(12)
Notice that the term on the left-hand side of the above equation can be written as the sum of the
term, which is the work done to move the propeller forward, i.e., the useful work. The
term is the work done on the air or the induced power loss; the induced power is an irrecoverable power loss, which constitutes a loss in the propeller’s efficiency in producing useful work.
Induced Velocity
The induced velocity in the plane of the propeller can be found by substituting Eq. 10 into Eq. 12, which gives
(13)
Expanding out and simplifying gives
(14)
This result gives the relationship between and
, i.e.,
(15)
For the next step, take Eq. 10 and substitute in Eq. 8 (mass flow rate) and the connection between and
(
) to get
(16)
The induced velocity in the plane of the propeller can now be solved for in terms of thrust, i.e.,
(17)
and expanding out gives
(18)
The ratio is referred to as the propeller disk loading. It can be seen that the latter equation is quadratic in
, which can be solved to get
(19)
for which there are two roots, i.e., from the term. Only one can be a physical root (the other one violates the assumed flow model, where the flow is assumed to be through the propeller from left to right), which is
(20)
(21)
Therefore, it will be apparent that for any given propeller producing a thrust , its induced velocity
will decrease rapidly with increasing airspeeds, i.e.,
. In the design of a propeller in its normal propulsive state, the induced losses can often be ignored compared to the profile losses.
Power Required
The corresponding power required to drive the propeller and produce a thrust, , becomes
(22)
The useful power for propulsion is , and the second term is the irrecoverable induced loss. Rearranging the preceding equation gives
(23)
This power equation can also be written non-dimensionally as
(24)
Notice that in the limiting case when the airspeed, , becomes high, then
, meaning that the induced losses tend to zero. Therefore, a greater fraction of the power delivered to the propeller goes into useful work to propel the airplane forward. However, in practice, some additional profile (non-lifting) power,
, will be required to overcome the viscous (shear) drag of the blades so that the total power required,
, can be written as
(25)
Propulsive Efficiency
The propulsive efficiency of the propeller can also be derived from the preceding analysis. The useful power for propulsion is so the efficiency of the propeller,
, can be written as
(26)
which shows that the propeller becomes more efficient at higher airspeeds, where the induced velocity becomes a smaller fraction of the airspeed. This result also confirms that the efficiency of the propeller at higher airspeeds as will be dictated by the profile losses on the blades, i.e.,
(27)
The profile power losses, denoted by , depend on the blade sections and the net blade area, which is measured by the propeller’s solidity or activity factor.
Activity Factor & Solidity
A parameter called the activity factor, , was used in the earliest days of propeller design. Its definition has originated within the industry rather than in any scientific context. The
is considered a measure of the propeller’s “aerodynamic activity” or effectiveness in producing thrust and is defined as
(28)
where is the number of blades,
is the reference chord length of the blade, and
is the diameter of the propeller. A higher value of the activity factor indicates that the blades have more lifting area for generating thrust.
Definitions and Nomenclature
Notice that both and
have historically been used to denote the propeller diameter, and they may be used interchangeably. In the NACA literature, the preference is to use
for diameter. Similarly, the definition of the reference chord has been inconsistent, ranging from the local chord value at the 70%, 75%, or 80% radius station to a weighted chord based on the platform area distribution. So, it is essential to verify the specific definition used in any given context. Likewise, propeller efficiency may be denoted by
or
, with the latter preferred in modern usage.
Solidity, given the symbol , is a related dimensionless parameter, which is defined as the ratio of the total blade area to the disk area swept by the propeller, i.e.,
(29)
The scientific literature commonly uses total solidity for the entire propeller and “local” solidity for a specific blade section, which is more geometrically meaningful than the activity factor. The local solidity is given by
(30)
Notice that a reference or “mean” chord is used when considering total solidity. The most appropriate mean chord for a propeller is a “torque” weighted chord based on
(31)
where =
and
= 0.15
is a starting point or root cutout that accounts for the presence of the propeller hub or “boss.”[10] The reasoning for this
weighted chord equation is that the blade sections near the tips are much more aerodynamically effective than those near the root end and so more affect the propeller’s performance. In this regard, other definitions of the activity factor have been used, including
(32)
Therefore, verifying the definitions used when analyzing propeller data in a specific context is essential, especially before conducting any comparative analysis. To be meaningful, the performance characteristics of different propeller blade shapes or numbers of blades, for example, should be performed based on the same value of the activity factor or overall solidity.
Wake Swirl Effects
Incorporating the swirl in the momentum theory involves adding the in-plane tangential velocity component to the otherwise purely axial flow, i.e., a rotational flow component parallel to the rotor’s plane of rotation. Rankine’s actuator disk theory considers only the axial momentum balance, assuming the flow is steady, inviscid, and incompressible, although Glauert further considered this term. When swirl is included, the average angular velocity component of the downstream flow must be considered, as it affects the power requirements and propulsive efficiency. While the effects are best considered within the framework of the blade element theory, an approximation can be derived from the momentum theory.
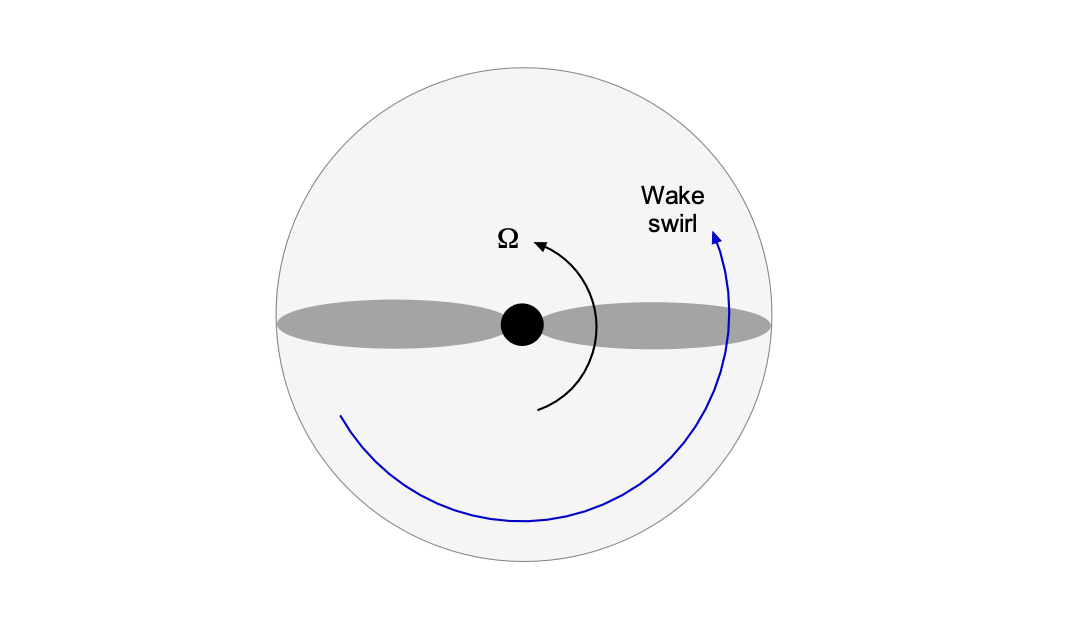
Propeller swirl refers to the rotational velocity of the air in the downstream wake behind the plane of a propeller, which, in effect, creates a spiral or corkscrew flow, as shown in the figure below. This effect occurs because of viscosity, where the propeller blades drag along a certain amount of fluid as they rotate. The upshot imparts a rotational velocity component to the fluid, , as a consequence of generating thrust. The higher the thrust and torque, the more significant his effect. Swirl causes efficiency losses because some kinetic energy is lost to rotational fluid motion, in addition to that from thrust generation.
Recall that the thrust generated by a propeller with a pure axial velocity component,
, is given by
(33)
where is the mass flow rate through the propeller. The power
required to generate this thrust, considering the presence of an average swirl velocity,
, is
(34)
where the effective velocity, , is given by
(35)
Therefore, the power required is
(36)
which is greater than the power required for a pure translational change in momentum through the propeller.
In the wake of a propeller, the tangential velocity component, , can be derived from the angular momentum imparted by the propeller blades. The torque,
, generated by the propeller can be calculated using the relationship between torque and power, i.e.,
(37)
where is the power absorbed by the propeller and
is the angular velocity of the propeller. The time rate of change of angular momentum, which is a torque, is given by
(38)
where is the angular momentum. By using the relationship between torque and angular momentum, the effective radius at which the torque acts can be considered as the mean aerodynamic radius of the propeller, which can be approximated by
(39)
where is the propeller’s radius (=
). Finally, the average tangential velocity,
, is
(40)
In practice, the effect of wake swirl on propeller performance is minimal, perhaps reducing the overall propulsive efficiency by 1% to 2%. However, for large propellers driven by engines delivering large amounts of torque, the effects may be as high as 5%. Coaxial counter-rotating propellers, ducted propellers, and swirl recovery vanes have been employed to mitigate wake swirl and enhance net propulsive efficiency.
Propeller Performance Coefficients
The thrust coefficient for a propeller is a non-dimensional thrust and is defined as
(41)
where is the thrust generated by the propeller,
is the propeller’s diameter, and
is the number of revolutions of the propeller per second (rps). If the rotational angular velocity of the propeller is
, then
. The rotational speed is often measured in revolutions per minute (rpm), so rpm = 60
rps = 60
.
For a propeller, a non-dimensional airspeed called a tip speed ratio or advance ratio, given the symbol , is defined by
(42)
Finally, the power coefficient for a propeller is defined as .
Notice that the definitions of propeller thrust coefficient, advance ratio, and power coefficient differ from those used for helicopter rotors. It is essential not to confuse them. The table below clarifies the differences, where = 2
.
| Rotating-wing system | Thrust coefficient, |
Power coefficient, |
Advance ratio |
| Helicopter rotor | |||
| Propeller |
The momentum theory results for a propeller can be written in coefficient form. For the induced velocity, then
(43)
and for the induced power, then
(44)
The corresponding propulsive efficiency is then
(45)
Momentum Theory of the Tandem Propeller
Tandem coaxial propellers are arranged on the same axis but rotate in opposite directions, allowing for a more compact design than separate, non-coaxial propellers. Additionally, the opposing rotation of the propellers cancels out torque reaction effects, which can be very significant when large amounts of power and torque are being transmitted from the engine. In a tandem propeller system, two propellers are aligned along the same axis but operate at different stations, effectively working on the same slipstream. The second propeller acts on the accelerated flow produced by the first propeller, leading to further acceleration and additional thrust generation.
There are two conditions of interest:
- The rear propeller is sufficiently separated to operate in the slipstream and induced velocity field of the front propeller.
- Both propellers are located so close that they share the same induced velocity.
Separated Tandem Propellers
The mass flow rate through both propellers must be conserved for the tandem system, as shown in the figure below. If the first propeller increases the velocity from to
, and the second propeller further increases it to
, the mass flow rates through each propeller are
(46)
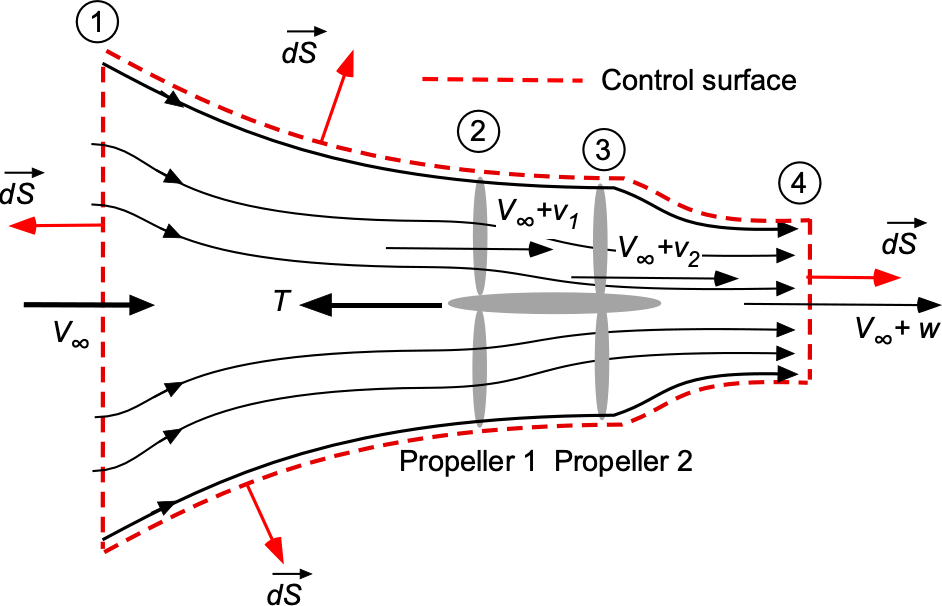
The thrust produced by each propeller can then be determined, i.e.,
(47)
The total thrust generated by the tandem system is then the sum of the thrusts produced by each propeller, i.e.,
(48)
The total power required is the sum of the power required by each propeller, i.e.,
(49)
The addition of the profile power is necessary to account for the drag associated with the propeller blades. It affects the overall efficiency of both single- and tandem-propeller systems. The efficiency of each propeller can be expressed as
(50)
For a tandem configuration with two propellers, the efficiency of each propeller is
(51)
Therefore, the overall efficiency of the tandem system can then be expressed as
(52)
Including profile power affects the efficiency calculation because both thrust production and the power required to overcome drag must be balanced. If the profile power becomes significant relative to the thrust generated, it will lower the overall efficiency, particularly at higher speeds where the profile losses will predominate. In this regard, it will be noted that
(53)
which is the same result as found for the single propeller, i.e., its net propulsive efficiency mostly depends on the profile power required.
Tandem Propellers with Shared Induced Velocity
Now consider two tandem propellers positioned closely enough to share the same induced velocity increment , as shown in the figure below. The flow velocity at the propeller planes is
. The analysis simplifies under the assumption that the induced velocity is the same for both propellers. The mass flow rate through the tandem propeller system is
(54)
where is the area of each propeller disk, which is assumed to be the same for both propellers.
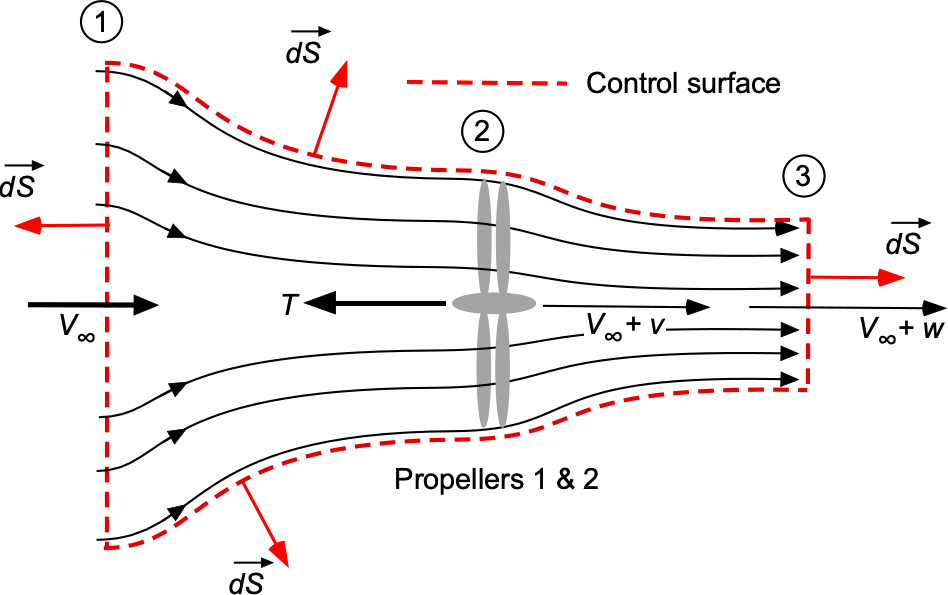
The thrust generated by each propeller can be expressed as
(55)
Because both propellers share the same induced velocity , the total thrust is
(56)
The power required for each propeller is given by
(57)
and
(58)
Therefore, the total power required is
(59)
The efficiency of the tandem propeller system can be analyzed by considering the ratio of useful work to the total power required. The useful work is related to the thrust produced and the forward velocity, . The efficiency of each propeller can be expressed as
(60)
Incorporating the profile power associated with the drag of the propeller blades, the overall efficiency of the tandem system can be expressed as
(61)
which again comes to
(62)
Comparing Efficiencies
A common issue in evaluating tandem versus single propeller designs is the basis for comparison, such as constant net thrust, equal power input, or equivalent solidity, the ratio of blade area to disk area. Within the framework of momentum theory and its inherent assumptions, no configuration is inherently superior. At higher airspeeds, the profile power required by the blades becomes the dominant factor in determining net propulsive efficiency. Although counter-rotating propellers can recover some energy from swirl in the wake, this effect contributes only marginally to overall efficiency. It is rarely significant enough to favor one configuration over the other.
When comparing propulsive efficiencies, it is essential to account for the added complexity of managing power distribution between propellers, as well as the overall weight efficiency of the propulsion system. Depending on the specific design choices and operating conditions, a tandem propeller system may outperform or underperform relative to a well-optimized single propeller. The primary advantage of a tandem configuration is its ability to absorb the same amount of power as a single large propeller while using a smaller overall diameter. Ultimately, the effectiveness of any propeller system depends on careful design and optimization to meet the required performance goals, subject to constraints such as size, weight, and cost. Generally, more blades result in higher weight and increased costs.
General Propeller Performance
A more accurate way to predict a propeller’s performance is by using the blade element theory, which can also aid in designing propeller blades to achieve optimal efficiency. Developing a computer program to calculate the performance of a propeller using the blade element method is a relatively straightforward task. The principle used here is calculating the angle of attack and the corresponding lift and drag at each propeller blade section, as shown in the figure below. All blades are twisted along their span from root to tip, which is done to achieve good aerodynamic efficiency. Therefore, the local value of blade pitch differs from point to point along the span.
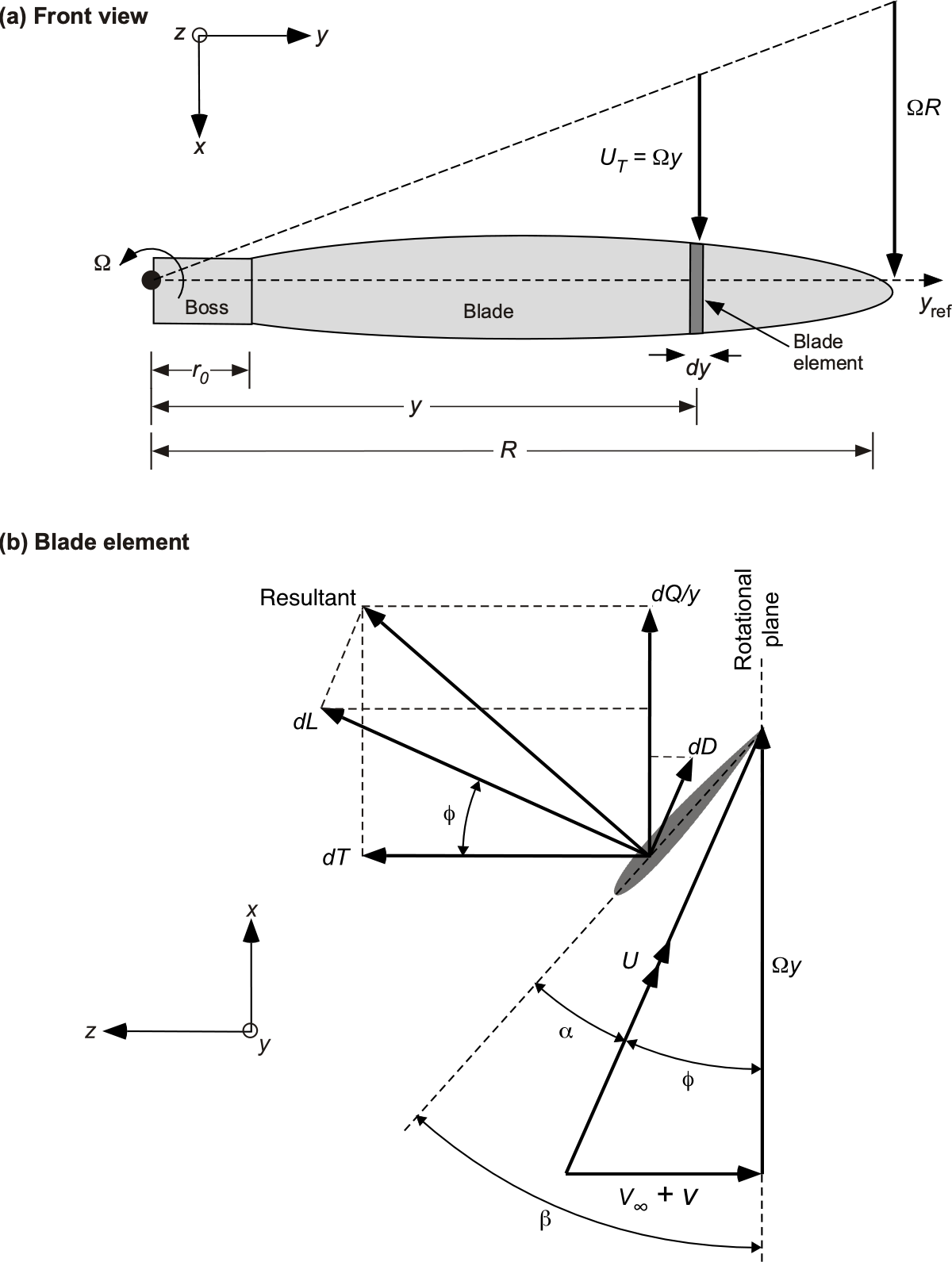
Notice that the relative flow angles and, hence, the angle of attack of the blade element are obtained by using the vector addition of the relative velocity components, i.e., the vector sum of the rotational velocity, , of the blade element as it rotates about the shaft and the freestream velocity,
, where the span position from the rotational axis is denoted by
. This combination yields what is typically referred to as the velocity triangle, which helps visualize and calculate the relative flow angles.
In the propeller theory, it is usually assumed that the induced velocity, , is small compared to the freestream (flight) velocity, i.e.,
, and can be neglected. However, at lower airspeeds, a good approximation for the average induced velocity over the propeller disk can be obtained using Eq. 20, i.e.,
(63)
Because is a function of thrust,
, and thrust is a function of
, including the induced inflow, an iterative approach is required.
Theoretical Development
The local pitch of the blade is as it varies along the span, so the angle of attack
of any blade section is
(64)
The lift coefficient on the blade element then follows as the product of the angle of attack of the blade and the local lift-curve slope of the airfoil section, i.e.,
(65)
where is measured from the zero-lift angle, recognizing that the lift-curve slope
will depend somewhat on the shape of the blade section used, as well as the local incident Mach number at the section, and to some extent, the Reynolds number too. The lift on a blade element will be
(66)
where is the local chord of the blade (
is the area of the blade element), and the resultant local velocity at the blade element,
, is
(67)
When the lift on all the sections of the propeller blade is obtained, then the net thrust of the propeller can be obtained by resolution of the local lift vectors in the direction of the thrust component, followed by spanwise integration, i.e.,
(68)
where is the number of propeller blades, and
is the propeller’s radius. The component of drag that affects thrust can be ignored. Additionally, it is reasonable to assume that the problem exhibits axisymmetry. Hence, the angles of attack at any given blade station are the same for any rotational angular position of the propeller blades. The integration process is usually performed numerically.
By analogous arguments, the power required to rotate the propeller will be
(69)
where is the local profile drag that again depends on the airfoil shape used on the blade and the incident Mach number. Notice the inclusion of the moment arm
to get to the torque (torque is a moment, so the product of a force times a distance or “arm”), and power is just the product of torque and angular velocity. In this case, however, the component of the lift
(which is the induced drag) will contribute to the net drag of the section and, hence, the torque and power required to rotate the blades.
If the section drag coefficient is known, then
(70)
However, the challenge with a propeller is to properly represent the section because it depends on the Mach number, in this case, the helical Mach number, i.e.,
(71)
where is the local speed of sound, i.e.,
). Of course, suppose the helical Mach number becomes too large and exceeds the drag divergence Mach number of the airfoil sections. In that case, the propeller’s efficiency will decrease rapidly.
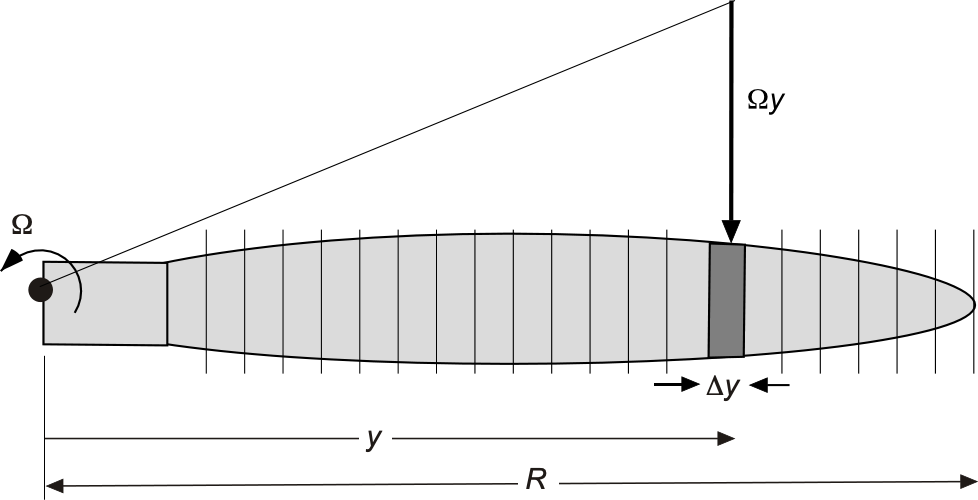
Numerical Implementation
The propeller blade can be discretized into segments, as shown in the figure below. To give any reasonable definition of the spanwise aerodynamic loads,
should be between 30 and 100. Each segment is then small enough to assume constant flow properties. Let
denote the spanwise position of the
-th segment, where
, and let
be the spanwise width of each segment.
For each segment, the resultant local velocity, , is computed using
(72)
and so the inflow angle is
(73)
The local angle of attack is then obtained using
(74)
The local lift coefficient, , is
(75)
If the drag coefficient is known or assumed, e.g.,
, it can be used directly; otherwise, the normal process is to interpolate from airfoil data tables (i.e., a table look-up approach) based on the local helical Mach number
. Reynolds number effects can also be included if such data are available.
Computing the lift force, , and the drag force,
, on each segment gives
(76)
Finally, the thrust and power contributions from all segments are then summed up using
(77)
which, for simplicity of illustration here, uses the trapezoidal rule of integration.
MATLAB code to implement the blade element theory analysis of a propeller. Including the inflow velocity is left as an exercise for the reader.
Show code/hide code.
% Constants and input parameters
rho = 1.225; % Air density (kg/m^3)
Omega = 200; % Rotational speed (rad/s)
V_inf = 50; % Free-stream velocity (m/s)
N_b = 3; % Number of blades
R = 1.5; % Propeller radius (m)
N = 100; % Number of segments
c = 0.1; % Chord length (m)
C_l_alpha = 5.7; % Lift-curve slope
C_d = 0.01; % Profile drag coefficient
% Discretize the blade span
y = linspace(0, R, N);
dy = y(2) – y(1);
% Initialize variables
J = V_inf / (Omega * R); % Advance ratio
beta_range = deg2rad(10:1:30); % Range of blade pitch angles in radians
C_T = zeros(size(beta_range));
C_P = zeros(size(beta_range));
for k = 1:length(beta_range)
beta = beta_range(k);
% Initialize variables for each pitch angle
V = sqrt((Omega * y).^2 + V_inf^2);
phi = atan(Omega * y / V_inf);
alpha = beta – phi;
% Lift coefficient
C_l = C_l_alpha * alpha;
% Calculate lift and drag forces on each segment
dL = 0.5 * rho * V.^2 * c .* C_l * dy;
dD = 0.5 * rho * V.^2 * c * C_d * dy;
% Thrust and Power calculation
T = N_b * sum(dL .* cos(phi));
P = Omega * N_b * sum((dL .* sin(phi) + dD .* y) * dy);
% Non-dimensional coefficients
C_T(k) = T / (rho * (Omega^2) * (R^4));
C_P(k) = P / (rho * (Omega^3) * (R^5));
end
% Plot the results
figure;
plot(J, C_T, ‘-o’);
xlabel(‘Advance Ratio, J’);
ylabel(‘Thrust Coefficient, C_T’);
title(‘Thrust Coefficient vs. Advance Ratio’);
figure;
plot(J, C_P, ‘-o’);
xlabel(‘Advance Ratio, J’);
ylabel(‘Power Coefficient, C_P’);
title(‘Power Coefficient vs. Advance Ratio’);
% Output results
fprintf(‘Advance Ratio (J): %.2f\n’, J);
fprintf(‘Thrust Coefficients (C_T):\n’);
disp(C_T);
fprintf(‘Power Coefficients (C_P):\n’);
disp(C_P);
Propeller Performance Charts
Propeller performance curves are presented in terms of the thrust coefficient , power coefficient
, and propulsive efficiency
, respectively, analogous to how airfoil and wing aerodynamic coefficients are used.[11] All propellers have their characteristics quantified in this manner, and there is a separate set of curves for each reference blade pitch angle. By convention, the reference pitch angle
is not the angle of attack of the blade sections of the propeller but the reference pitch at the 75% blade span (i.e., at
), which is a geometric quantity and can be measured.
Helical Pitch and Slip
Often, the pitch of a propeller is measured in units of length rather than an angle. This refers to the geometric helical pitch that the reference blade section traces out during one revolution, similar to a screw thread. Hence, the old name of a propeller is called an airscrew. As illustrated in the figure below, the helical pitch is the actual distance the propeller advances along its axis in one complete revolution, taking into account the effects of viscosity. If the propeller were moving through a perfectly rigid medium (like a screw in a solid material), it would advance precisely the distance of its geometric pitch per revolution, as shown in the figure below. However, the propeller actually covers a shorter distance because the air deforms under its action and traces out a helix with a lower pitch.

The helix angle, , describes the inclination of the path traced by a point on the propeller blade relative to the plane of rotation. A point on the propeller blade at radius
moves in a circular path with a circumferential distance per revolution given by
(78)
Simultaneously, the propeller advances axially by , which is given by
(79)
where is the airspeed and
is the rotational speed in revolutions per second. The helix angle
is given by
(80)
Substituting terms gives
(81)
For a reference span station, say , which is the conventional reference location for catalog data, the helix angle becomes
(82)
where is the propeller’s diameter.
A concept sometimes used to describe the efficiency of a propeller is its slip, which quantifies the difference between the geometric pitch (theoretical advance per revolution) and the actual advance through the air. The propeller slip ratio, , is defined as
(83)
where is the geometric pitch, i.e., the distance the propeller would ideally move forward in one revolution, and
is the actual advance, i.e., the distance the propeller actually progresses through the air per revolution.
A high slip ratio indicates that the propeller is inefficient in converting rotational motion into forward thrust, whereas a low slip ratio (near zero) indicates more efficient operation. Ideally, the actual advance per revolution would equal the geometric pitch, but in practice, some slip is inevitable because of wake deformations that occur under the action of the propeller. While the slip ratio is sometimes used as an indicator of propeller performance, it has limited quantitative aerodynamic significance and should not be confused with propulsive efficiency.
Propeller Dimensions
Propeller dimensions are typically given in inches because of historical conventions in U.S. aviation, where USC units remain the industry standard for aircraft manufacturing and maintenance. This ensures consistency with aircraft manuals, FAA regulations, and existing designs, making it easier for pilots, mechanics, and manufacturers to communicate and compare specifications. Diameter and pitch are expressed in inches. For example, the Sensenich 69CK propeller has a 69-inch diameter and is available in a helical pitch range of 42 to 58 inches, offering options such as 69 x 42, 69 x 44, and so on. While metric conversions are used internationally, general aviation favors inches for compatibility and ease of use.
Advance Ratio
The propeller advance ratio, , is a fundamental aerodynamic parameter that helps determine the efficiency and effectiveness of a propeller in producing thrust under different operating conditions. It connects forward motion, rotational speed, and propeller design, serving as a crucial non-dimensional parameter in propeller and rotor analysis. The advance ratio,
, is defined as
(84)
where is the airspeed (in units of m/s or ft/s),
is the rotational speed of the propeller (revolutions per second, fps), and
= diameter of the propeller (in units of m or ft). Physically, the advance ratio can be interpreted as the distance traveled by the propeller per revolution in terms of diameters, i.e., a non-dimensional distance or advance.
Thrust Coefficient
Recall that the thrust coefficient for a propeller is defined as
(85)
where is the thrust generated by the propeller,
is the propeller’s diameter, and
is the number of revolutions of the propeller per second. If the rotational angular velocity of the propeller is
, then
. Normally, the results for
and other propeller characteristics are plotted as a function of the advance ratio,
.
Representative versus
results for a propeller are shown in the figure below, with a separate curve for each reference blade pitch. Notice that the results from the blade element method discussed previously (i.e., the “theory”) agree well with the measurements.
Power Coefficient
Recall that the corresponding power coefficient for the propeller is defined as
(86)
where would be the brake power, i.e., the power delivered to the propeller through the driving shaft. Normally, the torque,
, would be measured, so then
=
. The corresponding
versus
results for a propeller are shown in the figure below. Again, notice that the theory is in good agreement with the measurements.

Propulsive Efficiency
Propeller efficiency measures how effectively the propeller converts available power into useful thrust. It is the ratio of useful power output (thrust power) to the total power input, i.e., engine power at the shaft or “brake” power. Propellers are typically designed for optimal performance at specific operating points. The propulsive efficiency of a propeller is defined as
(87)
which is just a non-dimensional statement that the propeller’s efficiency is the ratio of the useful power to the input power. Notice that the symbols or
are used to denote propeller efficiency, with
being preferred in most contexts to avoid confusion with the propulsion system’s overall efficiency.
Using the previous definitions of and
then
(88)
The corresponding representative versus
results for a propeller are shown in the figure below, again with one curve for each reference pitch angle.

Notice that for a given propeller operated with any given blade pitch and rotational speed
, its propulsive efficiency increases with increasing forward airspeed to reach a maximum and then diminishes rapidly. Consequently, a propeller of a given (fixed) blade pitch cannot operate with high propulsive efficiency over a wide range of values of
(or airspeed for a given rotational speed).
This latter outcome occurs because propeller blades are wings (rotating wings). All wings can only operate aerodynamically over relatively small ranges of the angle of attack, i.e., local blade section angles of attack between 2 and 14 degrees, depending on the local Mach number and Reynolds number. The local sectional angles of attack on the propeller depend not only on the rotational speed of the propeller and airspeed or advance ratio but also on how the propeller is twisted along its span. So, as airspeed changes, (propeller speed in terms of revolutions per second) is assumed constant. Then, the blade pitch must be increased to progressively maintain the angle of attack on the propeller. By gradually increasing the blade pitch, the best efficiency can be obtained over a much wider range of airspeeds, which is precisely the purpose of a continuously variable pitch or “constant speed” propeller.
Reference Blade Pitch
The aforementioned reference geometric blade pitch angle of a propeller, often denoted , is measured at the section located at the 3/4 radius from the rotational axis, i.e., at
. The pitch angle is taken between the local chord line of that section and the plane of rotation. It should be noted that it is a purely geometric quantity and does not represent the aerodynamic angle of attack. At that station, a material point on the blade traces a helix whose geometric helical pitch is given by
(89)
Given a reported helical pitch value at 3/4 radius, the corresponding geometric pitch angle is
(90)
The significance of the 3/4-radius station follows from the use of blade element theory. The power-weighted reference station is the radiuswise location at which an untwisted blade of constant angle
absorbs the same power as a hyperbolically twisted blade with
. Recall that the sectional velocity combines the rotational and axial components, i.e.,
(91)
where
(92)
Neglecting induced inflow corresponds to , so that
. The elemental torque is then
(93)
with
(94)
For small inflow angles, and
. Under an equal-thrust comparison, the induced torque satisfies
and cancels, so the profile torque provides the radiuswise weighting
.
For a blade with uniform chord with no root cutout, the power-weighted station is
(95)
Substituting gives the numerator as
(96)
and the denominator as
(97)
Forming the ratio and cancelling gives
(98)
In the small-inflow limit , then
(99)
which places the reference station at three-quarters radius. Increasing airspeed (larger ) moves the station slightly further inboard according to the closed-form expression above, but it remains close to
, which is why this location is used as a fixed reference. Once
is determined, the geometric helical pitch of the propeller can be reported as
(100)
Further Discussion of Propeller Performance
With this understanding of the flow at the blade section and the creation of thrust from the propeller, the various curves of ,
, and
, as shown previously, can now be explained in greater detail. At low values of
, the corresponding angles of attack of the blade sections are relatively high. So, the blade sections produce relatively high lift but are close to the point of stall. The propeller still produces thrust but requires high power and is inefficient. As airspeed and
increase, the blade sections operate at lower angles of attack and closer to their best section lift-to-drag ratios. As a result, the thrust is maintained, but the drag on the blades decreases, leading to a significant increase in propulsive efficiency.
The lowest angles of attack will produce little lift on the blades or thrust on the propeller. However, there is a range of airspeeds (assuming blade pitch does not change) for which good propulsive efficiency is obtained. Therefore, the best aerodynamic efficiencies will only be obtained when all or most blade sections operate at or near the angles of attack that yield their best lift-to-drag ratio, which is typically between 2 and 8 degrees, depending on the incident Mach number.
There is eventually a point at higher airspeeds (or high ) where the blade sections encounter diminished angles of attack and higher helical Mach numbers, simultaneously decreasing thrust and efficiency unless the blade pitch increases further. Eventually, the blade pitch cannot be mechanically increased to improve efficiency, and as a result, efficiency decreases.
Propulsive Efficiency
The results also explain the differences in propulsive efficiency of fixed-pitch versus constant-speed propellers, as shown in the figure below. Notice that if the propeller pitch is fixed, its propulsive efficiency increases slowly with airspeed, reaches a maximum, and then decreases rapidly. The relatively low efficiency of a fixed-pitch propeller at low airspeeds results in poor takeoff and climb performance for the aircraft. Further increases in airspeed beyond the airspeed for peak efficiency will cause propeller efficiency to decrease precipitously. This behavior reduces the power delivered to the airstream as useful work, effectively setting an upper barrier to the airspeed achievable by the airplane.
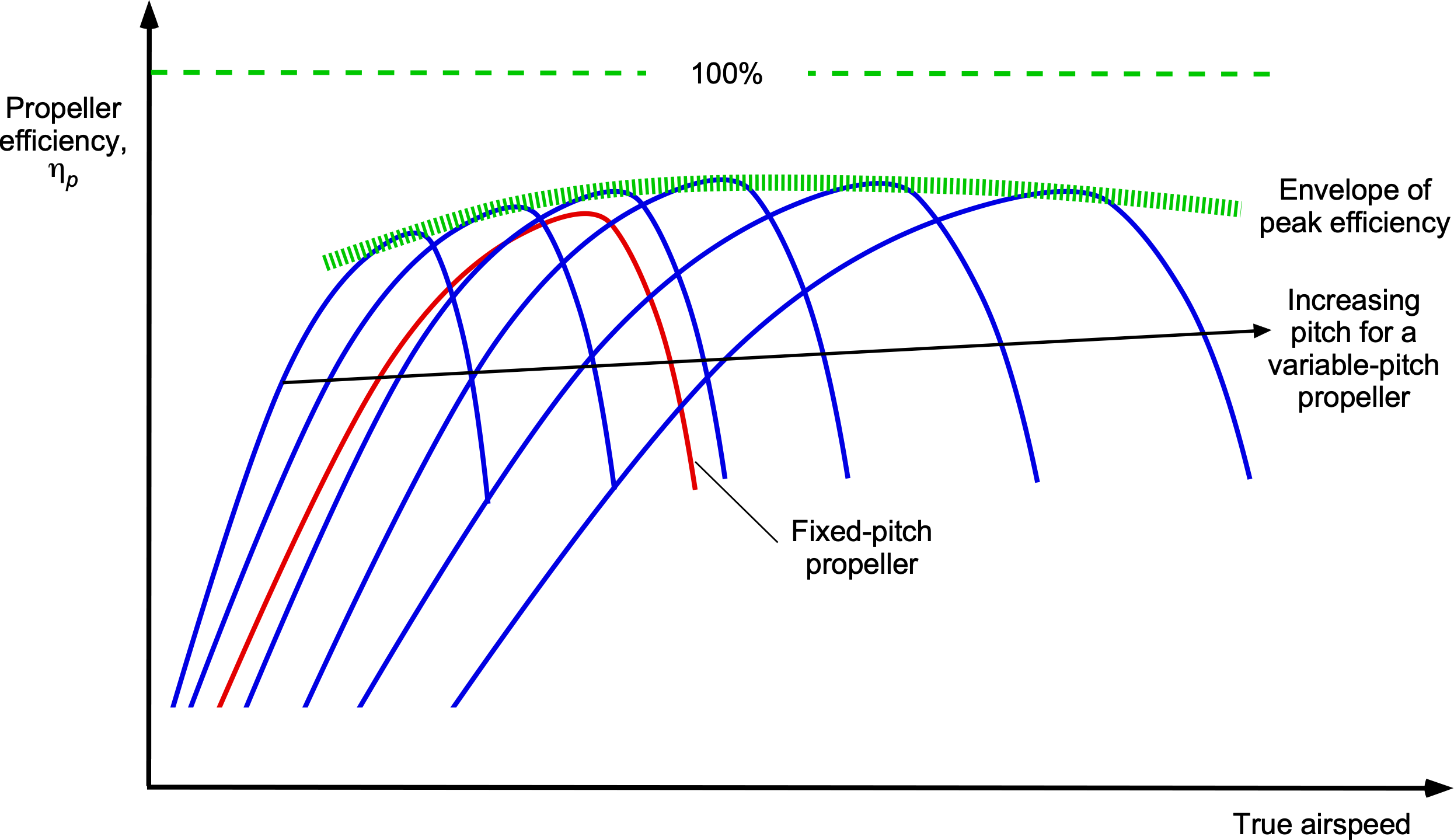
The preceding situation is very different for a variable-pitch or constant-speed propeller, which can be set to a fine pitch for takeoff. This provides good propulsive efficiency and low airspeed, resulting in markedly better takeoff and climb performance for the airplane. As airspeed increases, the blade pitch can be adjusted to maintain a constant rpm schedule, allowing the propeller efficiency to closely follow the envelope of peak efficiency. This reason is why airplanes with constant-speed propellers have much better overall flight performance and can cruise at much higher airspeeds, as shown in the figure below. A constant-speed propeller also maintains a steady load on the engine, which is essential for long engine life.
Worked Example #1 – Calculating propeller performance
Refer to the propeller charts below, which are a standard performance presentation for all propellers. Assume that an actual propeller has a diameter of 7 ft and is a constant-speed propeller with a rotational speed of 2,000 rpm. The propeller operates at an equivalent of 8,000 ft ISA density altitude.
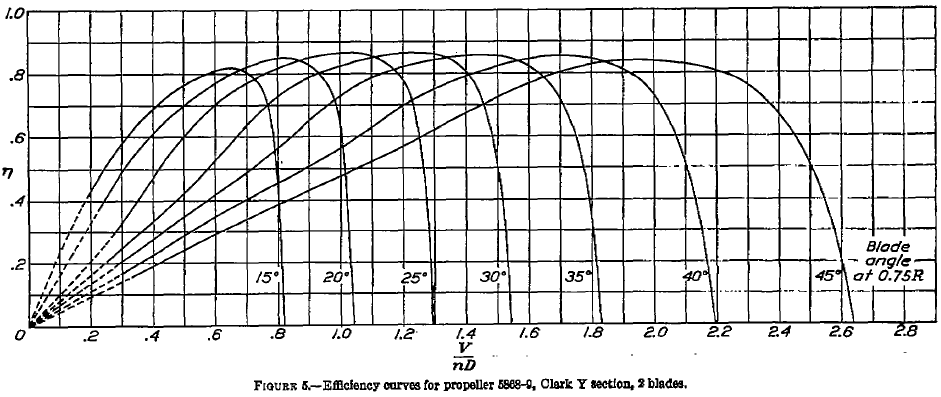
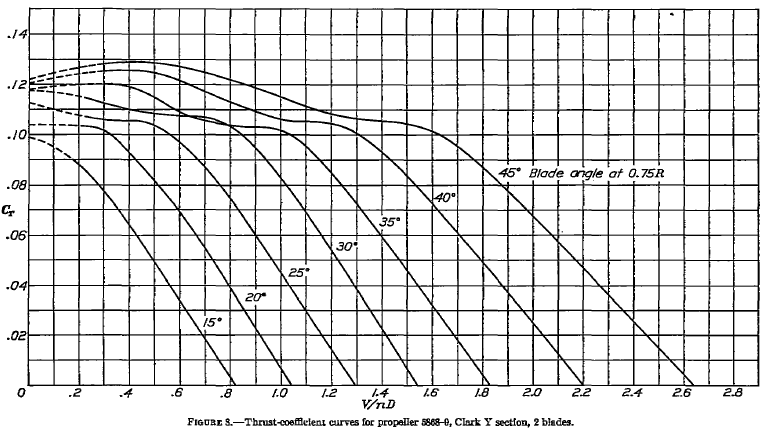
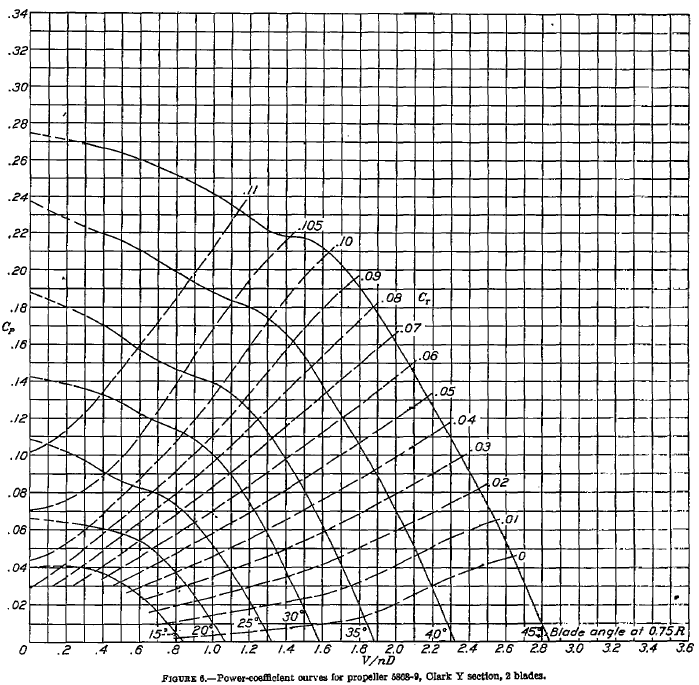 For each blade pitch angle measured at 75% radius and at the point of maximum propulsive efficiency in each case, estimate the following:
For each blade pitch angle measured at 75% radius and at the point of maximum propulsive efficiency in each case, estimate the following:
(a) What are the advance ratio values and corresponding airspeed values?
(b) What are the propeller thrust coefficient values and the propeller’s corresponding thrust?
(c) What are the values of the propeller power coefficient and the corresponding shaft torque and power required to rotate the propeller?
Show solution/hide solution.
(a) At the peak efficiency, the values of the advance ratio can be read off the first chart. We can easily do this to two decimal places; the chart can be digitized for better accuracy. We are also given information about the specific propeller, which is relatively small and would likely be for a general aviation aircraft, so in each case, we can calculate the corresponding airspeed for a given value of , i.e.,
so
It is best to use a table to show the results, i.e.,
| Blade pitch ( |
|||
| 15 | 0.82 | 0.65 | 151.7 |
| 20 | 0.85 | 0.82 | 191.3 |
| 25 | 0.87 | 1.04 | 242.7 |
| 30 | 0.87 | 1.25 | 292.7 |
| 35 | 0.86 | 1.45 | 338.3 |
| 40 | 0.86 | 1.70 | 398.7 |
| 45 | 0.84 | 1.95 | 455.0 |
(b) The propeller thrust coefficient can be read off the second chart for each value of the advance ratio, as was identified in the previous part. The thrust coefficient for a propeller is defined as
so the corresponding thrust (in units of force) from the propeller is
We are told that the propeller operates at the equivalent of 8,000 ft ISA density altitude. According to the ISA equations, the density at this altitude is 0.001869 slugs/ft. Inserting the information gives
Again, it is best to use a table to show the results, i.e.,
| Blade pitch ( |
|||
| 15 | 0.65 | 0.025 | 124.7 |
| 20 | 0.82 | 0.038 | 189.5 |
| 25 | 1.04 | 0.040 | 199.4 |
| 30 | 1.25 | 0.047 | 234.4 |
| 35 | 1.45 | 0.052 | 259.3 |
| 40 | 1.70 | 0.060 | 299.2 |
| 45 | 1.95 | 0.072 | 359.0 |
(c) The propeller power coefficient can be read off the third chart for each value of the advance ratio identified in the previous part. The power coefficient for a propeller is defined as
so the corresponding power needed to drive the propeller is
Inserting the known information gives
where the conversion to horsepower (hp) is obtained by dividing the result in ft-lb s by 550. Again, it is best to use a table to show the results, i.e.,
| Blade pitch ( |
|||
| 15 | 0.65 | 0.022 | 46.5 |
| 20 | 0.82 | 0.035 | 74.0 |
| 25 | 1.04 | 0.048 | 101.3 |
| 30 | 1.25 | 0.065 | 137.5 |
| 35 | 1.45 | 0.09 | 190.3 |
| 40 | 1.70 | 0.12 | 253.8 |
| 45 | 1.95 | 0.17 | 359.7 |
Helical Tip Mach Number
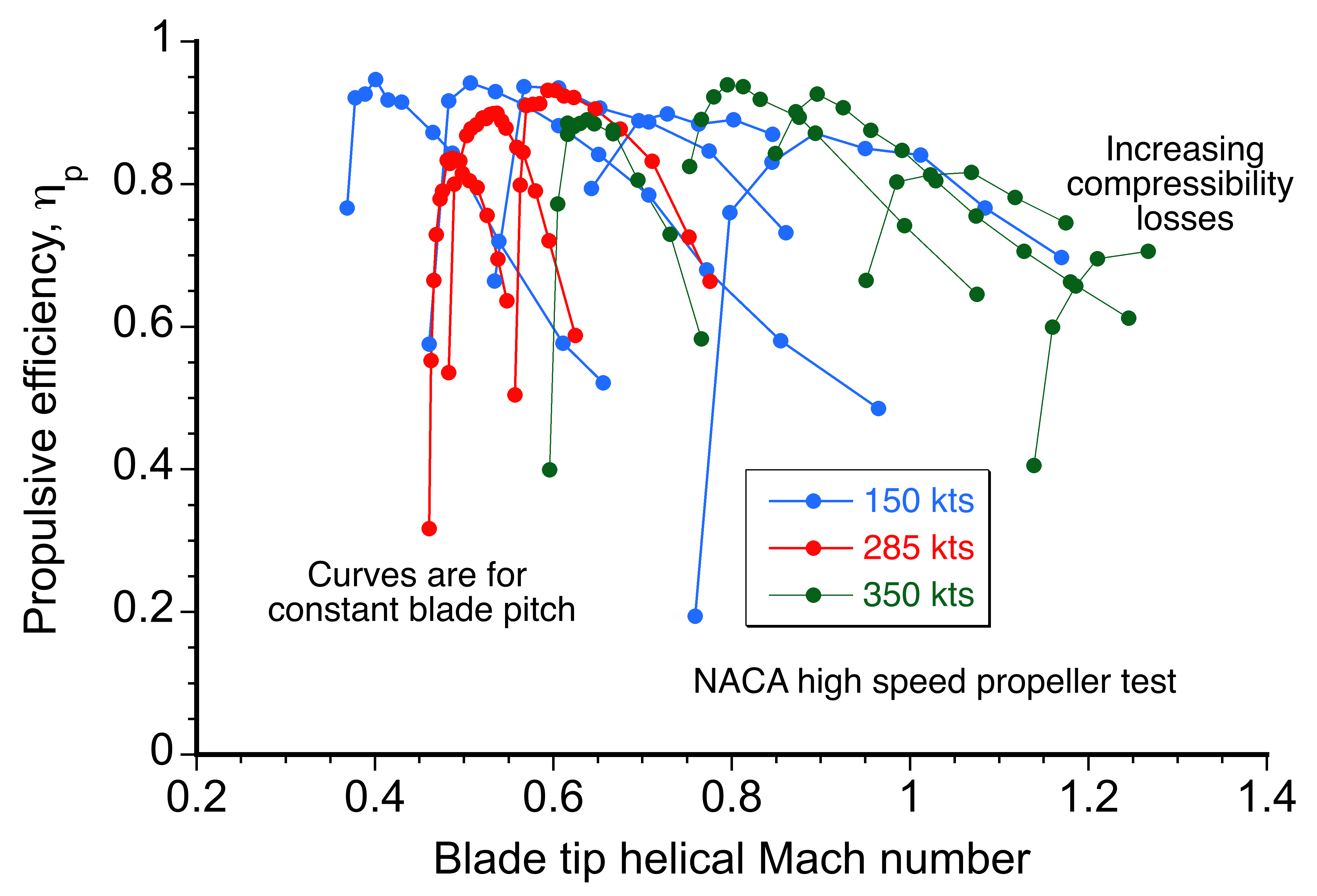
As airspeed and the values of increase, a concern is that the tip speed of the propeller can approach supersonic conditions. If this condition occurs, the propeller loses propulsive efficiency and produces more noise. This reduction in efficiency means that more power is required to make the same amount of thrust, which can adversely affect fuel consumption and overall flight performance. Supersonic airflow generates shock waves, resulting in a dramatic increase in noise levels from a propeller. A propeller’s noise is a significant concern in aviation, impacting passenger comfort, community acceptance, and regulatory compliance.
Let be the helical tip velocity based on the vector sum of the rotational and airspeed components at the blade elements. Therefore, the rotational tip speed of a propeller characterized by radius
or diameter
(= 2
) is
(101)
and so the helical tip speed of the propeller is
(102)
where is the forward airspeed. Therefore, the helical Mach number
will be
(103)
where is the speed of sound at the atmospheric conditions at which the propeller operates.
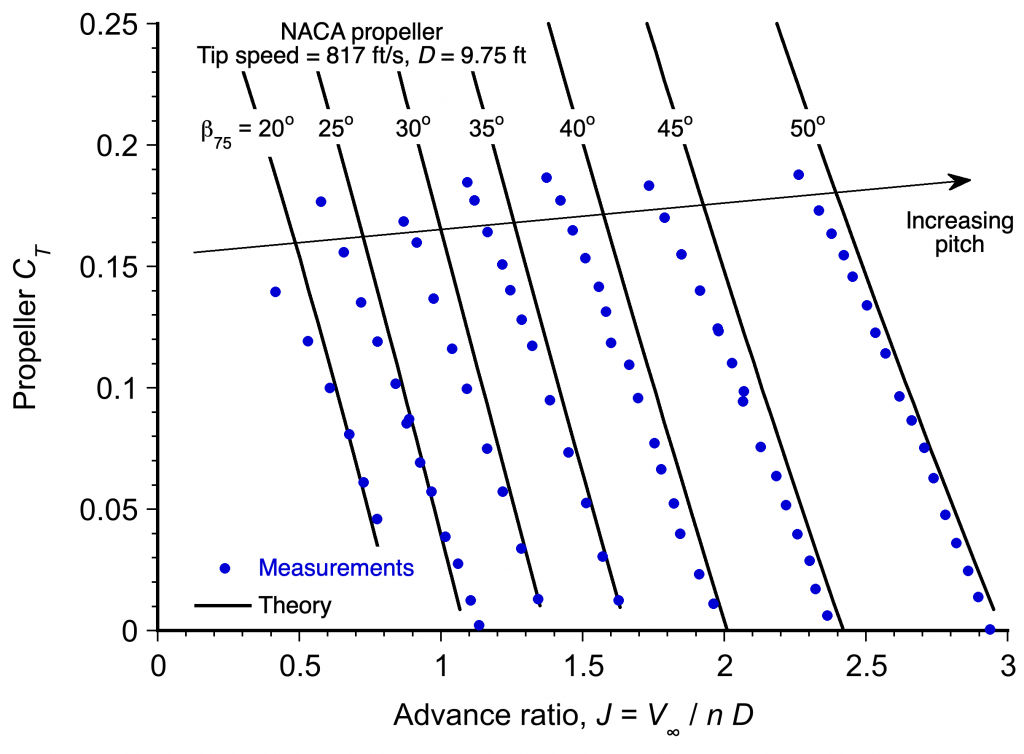
The measurements shown in the figure below, which are for a high-speed NACA propeller with thin blades, eventually reach supersonic helical tip Mach numbers. In this case, the measurements were taken at a constant blade pitch to examine variations in rotational speed. Notice that rapid losses in propulsive efficiency (from a peak of about 0.95) are produced at higher airspeeds if the rotational tip speeds are kept at high values. Increasing compressibility losses appear when helical tip Mach numbers of about 0.8 are reached. While every propeller will be different, exceeding some limiting critical tip Mach number at the blade tip, which may be around 0.7 for a standard blade and 0.8 for a thin airfoil section and swept leading edge at the tip, can be expected to decrease propulsive efficiency, increase noise, or, more usually, both.
Propeller Noise
Propeller noise is a significant contributor to the acoustic signature of propeller-driven aircraft, particularly during takeoff, climb, and high-speed cruise flight. It originates from unsteady aerodynamic forces acting on the blades and from compressibility effects at high tip speeds. Unlike rotorcraft, conventional single-rotor airplane propellers do not experience blade-wake or blade-vortex interaction noise. Instead, the dominant sources are thickness noise, loading noise, noise from compressibility effects, and shock waves that originate at the blade tips.
Thickness noise arises from the periodic displacement of air by the rotating blades. This type of noise radiates at the blade-passing frequency and integer multiples of its harmonics. The aerodynamic forces cause loading noise, primarily from the lift on the blades. It is most intense during high-thrust phases such as takeoff and climb. Both sources contribute to the prominent tonal peaks in the noise spectrum.
As the tip Mach number approaches or exceeds 0.85, compressibility effects become significant, and localized shock waves may form near the blade tips. This results in a significant increase in noise amplitude, harshness, and high-frequency content. The noise becomes impulsive in character and highly directional. The schlieren image below illustrates this phenomenon for , showing spiraling acoustic Mach waves forming at the blade tips. These waves compress and intertwine into a rotating analog of a Mach cone produced by an airplane flying near the speed of sound, similar in character to a forming sonic boom. Also visible are the tip vortex and the turbulent blade wake, which contribute additional high-frequency acoustic content.
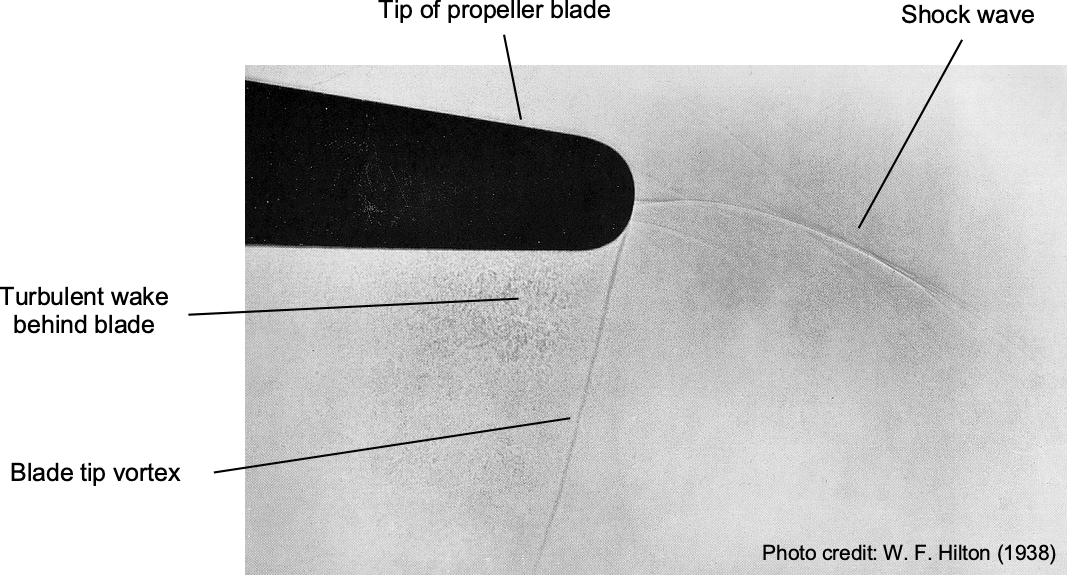
The dominant source of propeller noise in airplane applications is loading or monopole noise, generated by unsteady aerodynamic forces acting on the rotating blades. These effects can be modeled as a so-called dipole source, and the far-field acoustic power radiated by such a source term increases quickly with blade tip speed. From classical aeroacoustic theory, the acoustic power radiated by a rotating blade system can be approximated as
(104)
where is the radiated acoustic power,
is the freestream air density,
is the aerodynamic force on the blade,
is the angular velocity of the propeller,
is the blade radius, and
is the freestream speed of sound. The aerodynamic force scales with dynamic pressure, giving
. Therefore, substituting into the expression for
leads to
(105)
Furthermore, using the rotational tip Mach number gives
(106)
Because the sound pressure level (SPL) scales logarithmically with acoustic power, then
(107)
and finally leading to the propeller noise scaling law with tip Mach number, i.e., the sound pressure level is
(108)
In practice, empirical measurements often yield a slightly lower exponent because of effects other than ideal, such as non-uniform inflow, installation interference, and atmospheric absorption. A more commonly used representation is
(109)
The dominant frequency of propeller noise corresponds to the blade-passing frequency, defined as
(110)
where is the number of blades and
is the shaft rotational frequency. Tonal components, associated with thickness and loading noise, tend to dominate the acoustic spectrum and are most intense at the blade-passing frequency and its harmonics. Broadband noise arises from turbulence ingestion, unsteady blade loading, and distorted inflow conditions. The radiation pattern is highly directional, with tonal noise being strongest in the plane of rotation, while loading noise is primarily radiated in a direction parallel to the thrust axis.
Several strategies exist to reduce propeller noise. Limiting tip speed is essential to avoid shock-induced impulsive noise, which can be managed by selecting a larger diameter or reducing the rotational speed. Increasing the number of blades reduces the per-blade loading and shifts tonal energy to higher, more attenuated frequencies. Careful blade design, including sweep, twist, and taper, helps minimize the unsteady aerodynamic forces and delay compressibility effects. Finally, installation effects are critical; distorted inflow caused by fuselage or wing boundary layers can exacerbate noise, especially in pusher configurations. Tractor propeller arrangements, with cleaner inflow conditions, typically offer lower noise levels for a given thrust.
Example #2 – Calculating a propeller’s helical tip Mach number
Refer back to Worked Example #1. What are the propeller’s helical tip speed values and helical Mach number? Comment on your results. Note: The helical tip speed is the vector sum of the rotational speed at the tip of the propeller and the freestream (airspeed).
Show solution/hide solution.
Let be the helical tip velocity based on the vector sum of the rotational and airspeed components. The rotational tip speed of a propeller of diameter
is
where and so the helical tip speed of the propeller is
where is the forward airspeed. The helical Mach number
will be
where is the local speed of sound at the conditions at which the propeller operates. At 8,000 ft ISA density altitude,
1085.3 ft/s.As a final table to show the results, the helical tip speed and Mach number are:
| Blade pitch ( |
||||
| 15 | 0.65 | 151.7 | 748.6 | 0.69 |
| 20 | 0.82 | 191.3 | 757.6 | 0.70 |
| 25 | 1.04 | 242.7 | 772.2 | 0.71 |
| 30 | 1.25 | 292.7 | 788.9 | 0.73 |
| 35 | 1.45 | 338.3 | 807.3 | 0.74 |
| 40 | 1.70 | 398.7 | 833.5 | 0.77 |
| 45 | 1.95 | 455.0 | 862.8 | 0.79 |
We notice that for an airspeed above 400 ft/s, the propeller blade tips would likely begin to operate near or just beyond the critical Mach number (i.e., the onset of transonic flow), which for the thin tips of propeller blades is about 0.8. Under these conditions, the propeller will likely lose propulsive efficiency.
Propeller Selection Process
Propeller selection must match the aircraft’s mission, performance goals, and propulsion system. Key factors include thrust requirements across flight phases, powerplant type (piston, turboprop, or electric), cruise efficiency, and installation constraints. The diameter of the propeller has a significant impact on thrust, power consumption, and efficiency. Larger diameters enhance low-speed performance but may be limited by ground clearance, airframe geometry, or high tip speeds. At higher rpm, blade tips must remain subsonic to avoid compressibility losses and the rapid increase in noise associated with the tip Mach number.
The blade count affects the amount of thrust a propeller can produce for a given diameter. More blades allow the disk to absorb greater power, which is helpful for high-power engines or when the diameter is constrained by ground clearance or airframe layout. However, additional blades add weight, drag, and mechanical complexity, especially in variable-pitch systems. Blade geometry must also be optimized spanwise to maintain efficiency under varying inflow. Key factors include the airfoil section, chord distribution, and twist, which typically follow a hyperbolic pattern from the root to the tip.
Variable-pitch or constant-speed propellers maintain propulsive efficiency by adjusting blade angles in flight and are standard on high-performance piston and turboprop aircraft. Material selection also matters. Wood is lightweight and inexpensive, but it is limited to low-power use under 200 shp (149.4 kW). In contrast, aluminum is at least three times stronger but approximately six times heavier by volume. Composite blades combine low weight with high strength, allowing for thinner, more efficient shapes in high-speed turboprop applications.
The propeller must also match the engine’s power and torque characteristics. Piston engines operate efficiently at lower rpm (less than 3,000) and can often drive propellers directly. Turboshaft engines typically require a reduction gearbox, although free-turbine designs are also common and efficient.[12] Electric motors, with high torque at low rpm, can also drive large, slow-turning propellers directly, in the range 4,000 to 8,000 rpm. Additional concerns for propeller integration include vibration, noise, and inflow distortion, particularly for pusher configurations that are affected by an upstream wake. A successful design will strike a balance among these factors to deliver efficient, reliable, and quiet propulsion that suits the aircraft and its mission.
Steps for Propeller Selection
The following are the specific steps that are involved in the selection of a propeller:
- Define the mission profile and flight envelope. These requirements may include the maximum takeoff weight, cruise speed and altitude, climb performance, and powerplant characteristics and limitations such as available power and allowable rotational speed (rpm) range. Airframe installation constraints, such as ground clearance or nacelle position, must also be considered.
- Estimate thrust and power requirements. Use aircraft drag estimates and mission analysis to determine the required thrust during takeoff, climb, and cruise. Combine this with available shaft power from the powerplant to calculate the required propeller efficiency.
- Select a candidate propeller diameter and rotational speed. Select a diameter that provides sufficient disk area for thrust without exceeding the tip Mach number limit of approximately 0.85 at the maximum propeller rpm, thereby minimizing compressibility losses.
- Choose or design a blade geometry. For custom designs, one can use blade element theory (BET) or similar methods to determine the optimal twist and planform (chord) distribution. For off-the-shelf options, consult the manufacturer’s data sheets and performance characteristics (i.e.,
curves) to determine the thrust coefficient, power coefficient, and efficiency, which will help in selecting the propeller.
- Calculate the advance ratio and match it to the performance requirements. Use the
parameter to find the operating point of the aircraft, then compare it to the propeller performance curves. One generally aims to operate the propeller near its peak efficiency at cruise, while still ensuring sufficient thrust and propulsive efficiency at lower airspeeds, such as takeoff.
- Determine whether variable pitch is required. If the flight conditions span a wide range of flight speeds or power settings, a variable-pitch propeller may be needed to maintain the propulsive efficiency. Fixed-pitch or ground-adjustable designs are simpler but can be optimized for only one flight condition.
- Evaluate structural and material choices. Consider blade loads, centrifugal forces, vibration, and the possibilities of material fatigue. Common materials include wood, aluminum, and composite, each with its own trade-offs in terms of weight, strength, durability, and cost.
- Integrate with the engine and airframe. Ensure compatibility with the engine’s torque curve and power output. It may be necessary to account for airframe-induced inflow distortion, especially in pusher configurations. Validate hub mounting, spinner clearance, and “ram” airflow to the cooling system.
- Test and refine the selection. After the preliminary design or selection, the propeller’s performance must be verified through ground runs and flight testing. Normally, aircraft manufacturers will instrument the powerplant and aircraft to record enough parameters to verify or revise the prepropeller design as needed based on outcomes from the flight test data.
Speed-Power Coefficient (Weick) Method
The speed-power coefficient, , is a non-dimensional parameter introduced by Fred Weick at NACA to characterize propeller performance independently of physical dimensions, such as diameter or blade pitch. Weick originally used the
coefficient to develop empirical performance maps of propeller efficiencies, thereby reducing or eliminating the need for wind tunnel or shaft torque (i.e., dynamometer) measurements. This approach remains useful today, particularly when evaluating geometrically similar propellers or analyzing flight data from smaller propeller-driven aircraft. While the advance ratio
is central to the selection and aerodynamic design of propellers, the
coefficient often proves more practical for interpreting in-flight performance, particularly under less-than-ideal operating conditions. In the case of fixed-pitch propellers, which are efficient only over a small range of conditions, the value of
at the intended operating conditions helps identify the most appropriate propeller. This is because it links flight speed, power, and rpm to the known or predicted performance characteristics of the powerplant and the aircraft.
Definition and Derivation
Weick[13] noted that while the ,
, and
coefficients help compare the thrust, torque, and power of different propellers, “they are not particularly helpful, however, in selecting or designing propellers to fit certain specific requirements for driving some form of airplane.” Furthermore, Weick noted that “the parameter
is not a fair basis upon which to compare the efficiencies of various propellers that might fit the same aircraft at the same values of
and
but have different diameters,
, and, therefore, other values of
.”
To this end, Weick showed that a new dimensionless propeller coefficient involving ,
, and
without
may be obtained from
and
using
(111)
where is the air density,
is the true airspeed of the propeller through the air,
is the shaft power available to the propeller, and
is the propeller’s rotational speed in revolutions per second. An alternative form, which keeps the numerical values within a reasonable range, is in terms of the fifth root of the previous expression, where
(112)
Notice that the numerical values of must be calculated using consistent engineering units.
It should be apparent that is called a “speed-power” coefficient because it contains the rotational and forward speeds and the power that the propeller needs to absorb.[14] The diameter and pitch selection of a propeller is based on identifying the optimal balance between efficiency and operational constraints under specific design conditions for the aircraft. The power required for flight depends on the aircraft and its operating conditions. Because a fixed-pitch propeller operates most efficiently at a single point in its performance envelope, the
coefficient can help determine the best-matched propeller for the desired airspeed and power available at the propeller’s shaft.
Practical Usage and Application
The speed-power coefficient has several practical applications, including the selection of fixed-pitch propellers, in-flight performance testing, and the analysis of aircraft performance, particularly when complete aerodynamic data are unavailable. In many aircraft test programs, thrust is not directly measurable, but airspeed, rpm, shaft power, and air density can usually be obtained. Because the
coefficient incorporates both power and air density, it naturally accounts for variations in throttle setting and density altitude, allowing test data from different flight conditions to be reduced to a more consistent and useful form.
Wind Tunnel Testing
The value of the coefficient can first be explained with reference to propeller performance measurements made in the wind tunnel. Propeller testing in the tunnel often involves using a series of geometrically similar designs, with variations in pitch and airspeed that are applied in uniform increments. For example, the NACA tested propellers in their 20-foot wind tunnel using a system that allowed continuous adjustments in pitch over an extensive range of angles and at increasing airspeeds until the blade tips exceeded their critical Mach numbers. High helical tip Mach numbers typically set a limit on propeller operation in terms of both efficiency and noise, or sometimes both. For a fixed-pitch propeller of a given blade design, the range of pitch angles that are available is typically limited to only two or three.
If performance data are available for candidate propellers, the corresponding value of can also be calculated and compared to the efficiency curves. Start with the definition that
(113)
The propulsive efficiency is given by
(114)
Substituting the standard expressions for and
into the definition of
gives
(115)
and substituting into the expression for gives
(116)
Simplifying gives the result that
(117)
which can be evaluated from the data comprising the standard propeller “ curves.”
An example of this type of analysis is shown in the figure below. The curves in the plot show the values of as a function of advance ratio
for each blade pitch, along with the corresponding curves of propeller efficiency
. The “curve of best efficiency” traces a locus of operating points where each propeller configuration operates most efficiently. By computing
for a desired flight speed, shaft power, and rpm, one can find the corresponding
and so select a propeller diameter that places the powerplant and aircraft as a system near this optimal propeller curve. Alternatively, for a given propeller and
value, the use of
allows the power
and revolutions per second
available from a given powerplant to be matched to this propeller.
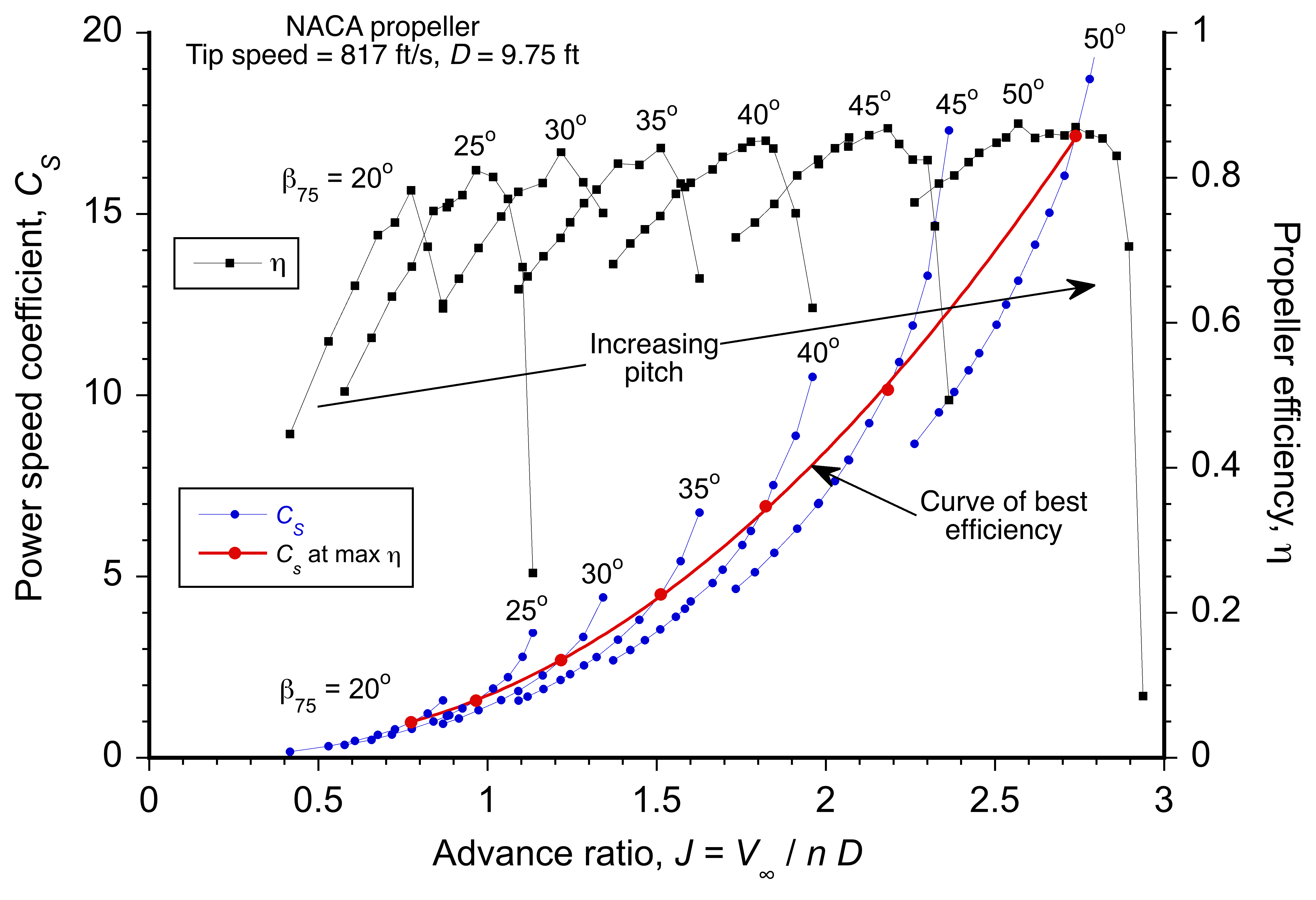
If the desired advance ratio is known or can be determined, then the corresponding propeller diameter is computed using
(118)
The blade pitch is then chosen so that the propeller exhibits its peak efficiency near the target , with some intentional bias toward lower values to avoid performance degradation at takeoff and climb. This trade-off is necessary because fixed-pitch propellers cannot be adjusted in flight. Alternatively, the propeller speed,
, can be chosen for a given propeller diameter, i.e.,
(119)
It should be noted that, regarding wind tunnel testing, the coefficient does not provide any additional information about propeller selection, as the diameter and pitch required to meet a given level of performance can be determined entirely from the “
-curves.” What it does do, however, is verify that the selected propeller matches the power available from the powerplant at a given airspeed and density altitude, and that the corresponding values of the
and
coefficients are within the normal operating range of that propeller and are close to the line of best propulsive efficiency. This approach ensures that the chosen propeller utilizes the available power efficiently, neither overloading nor underloading it.
Flight Testing
In flight testing, the speed-power coefficient provides a valuable means of assessing propeller performance when the propeller “
curves” are unavailable. Its utility lies in the fact that all of the constituent terms of
, i.e.,
(120)
can be obtained from basic flight instrumentation. The airspeed is measured using a Pitot-static system and corrected, if necessary, by local air density to provide the true airspeed, . The air density
is computed from measurements of ambient pressure and temperature using the equation of state, or by deriving it from measurements of pressure altitude and temperature with the ISA model.
The shaft power can be estimated from powerplant data in terms of performance charts or “decks” in the form of numerical data. For example, for piston engines, the shaft power required to compute
is estimated using engine performance data that correlates manifold pressure, engine rpm, pressure altitude, and outside air temperature with brake power (e.g., bhp) output. These values are measured from the aircraft’s engine instruments and, in post-flight analysis, are cross-referenced against charts or tables provided by the engine manufacturer. This indirect method, while approximate, is sufficiently accurate for trend analysis and performance comparison. For electric motors, the electrical input power, measured in volts and amps, can be measured during flight and corrected for by the motor’s electrical-to-mechanical efficiency, i.e., through a calibration.
By evaluating for a sequence of flight test points at different values of
, engineers can assess the suitability of a given propeller, evaluate off-design behavior, and ensure proper matching between the propeller and powerplant, all without needing detailed propeller “
-curves.” As shown in Fig.~??, which comprises notional flight test data, the most suitable propeller(s) for the aircraft will show data points that cluster tightly or trend smoothly. This outcome suggests the propeller is a good match for the powerplant and the aircraft. If the data points exhibit significant scatter and display inconsistent trends, this outcome suggests a less suitable or even an unsuitable propeller. More than one flight with each propeller should be conducted to establish definitive trends.
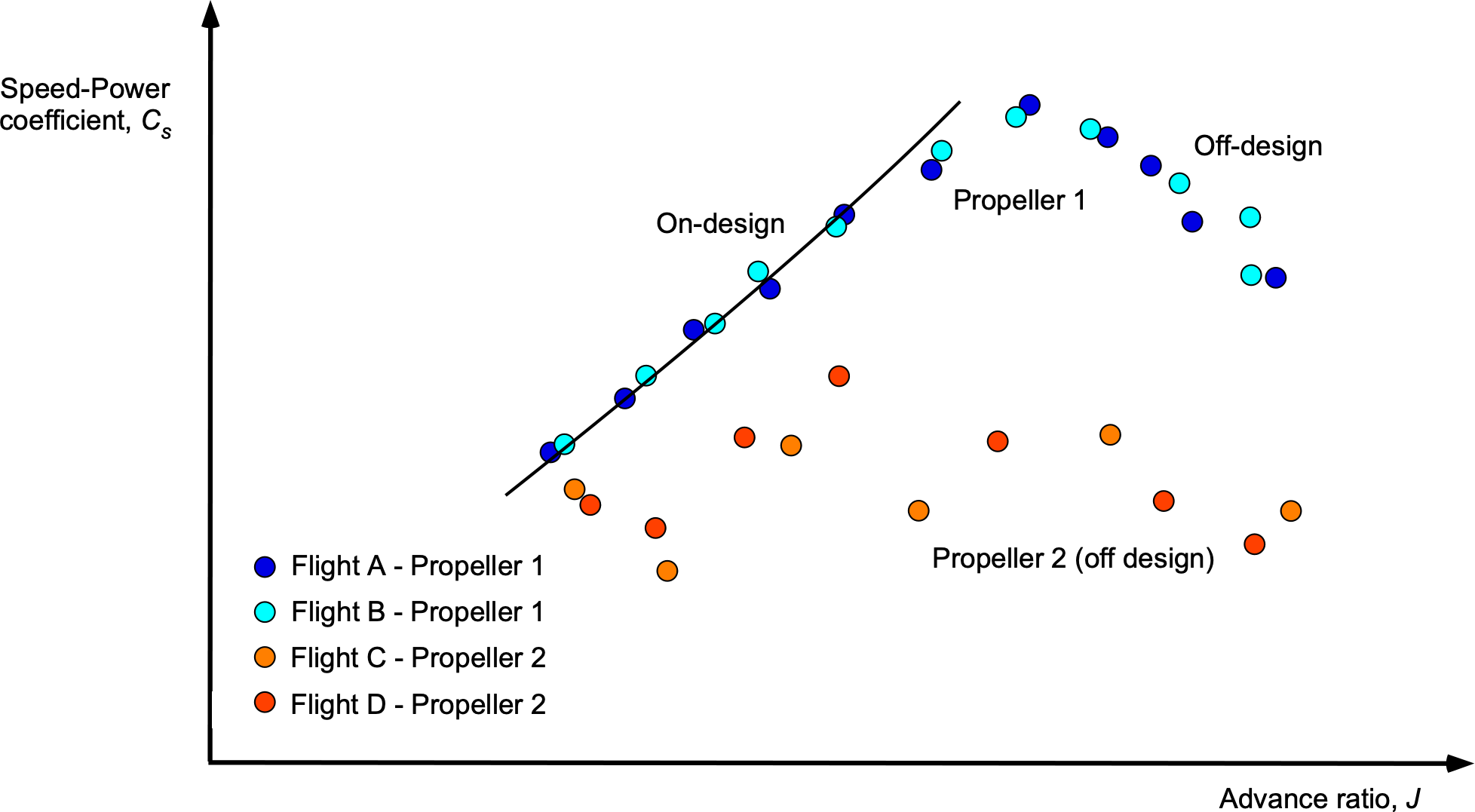
Pusher Propellers
Tractor propellers, which are mounted at the front of the aircraft and operate in undisturbed freestream flow, represent the most common configuration in aircraft design. However, numerous aircraft have been developed with pusher propellers, where the propeller is located aft of the fuselage or wing structure. In this arrangement, the propeller operates in the wake of the airframe, which can influence its aerodynamic performance. Today, pusher configurations are frequently employed in uncrewed aerial vehicles (UAVs) and drones, as illustrated in the photograph below.

The wake generated by the fuselage will influence the aerodynamic performance of a pusher propeller because it ingests air altered by upstream boundary-layer growth and, in some cases, regions of separated flow. The principal aerodynamic consequence is a distortion of the inflow profile across the propeller disk, with the inner region typically experiencing a significant velocity deficit. The upshot is a reduction in the effective mass flow rate through the propeller, along with changes to the local angle of attack on the blades. In severe cases, ingesting a fuselage wake can introduce unsteady turbulent structures into the flow, potentially degrading propeller performance and increasing noise.
There are few direct measurements that compare the performance of pusher propellers versus that of tractor propellers under the same or similar operating conditions. Fred Weick alludes to wind tunnel tests conducted by the Royal Aircraft Establishment (RAE) in 1922. The results, which are summarized in the figure below, suggest that even with relatively large fuselage sizes compared to the propeller diameter, the effects on maximum propulsive efficiency of a pusher versus a tractor propeller are minimal, perhaps on the order of 2%. Nevertheless, the wide range of potential fuselage shapes and downstream wake profiles that could be encountered in practical design indicates that some modeling effort is warranted better to understand the magnitude of the possible interference effects.
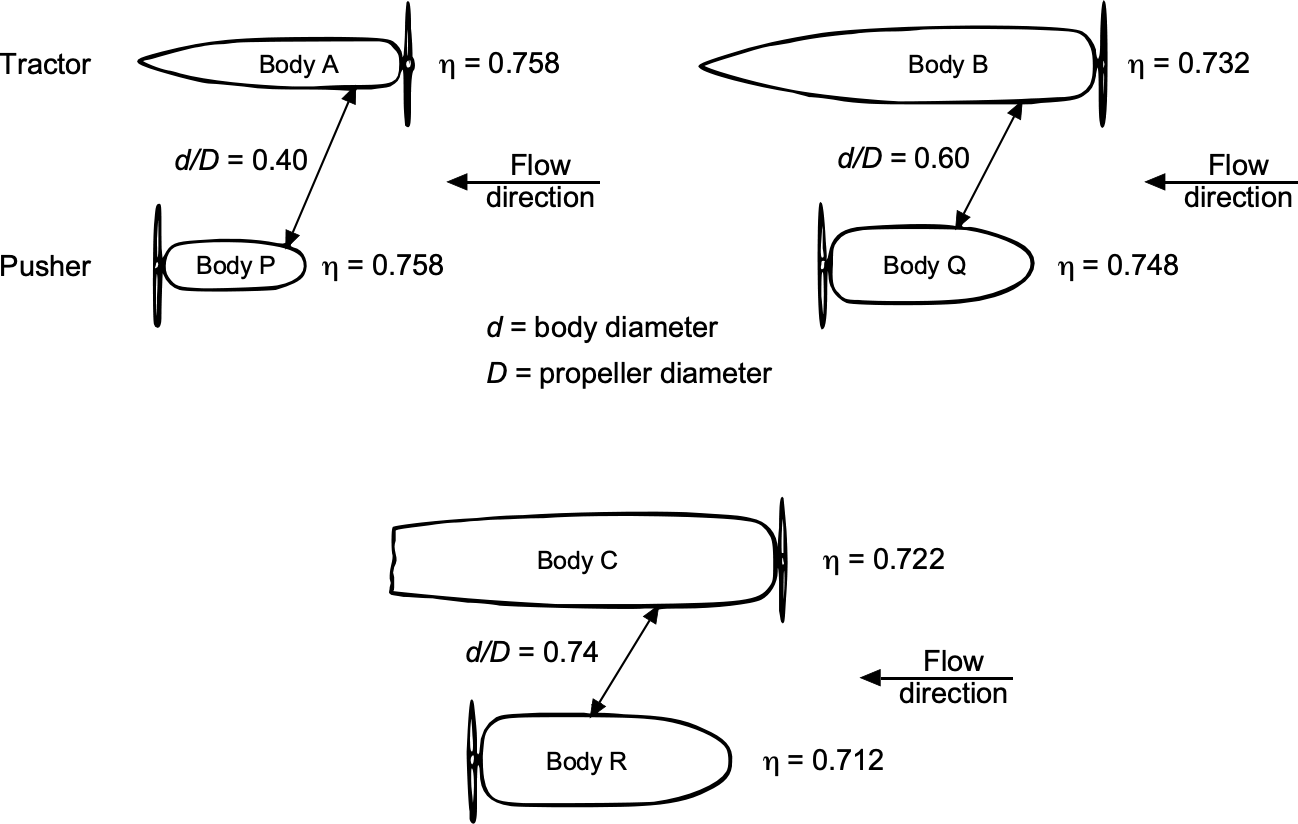
Flow Model
Consider a flow model of a pusher propeller, as shown in the figure below, where the inflow into the propeller disk is composed of two concentric zones:
- An inner disk of radius
that is subject to a velocity deficit
, i.e.,
. The parameter
is a characteristic wake width or spread parameter, typically comparable to the diameter of the fuselage.
- An outer annular region from
to
that is exposed to undisturbed freestream flow, i.e.,
.
In this model, each region is treated as an independent actuator disk segment, where and
denote the inner and outer areas, respectively. Assume both regions have the same average induced velocity
at the plane of the propeller.
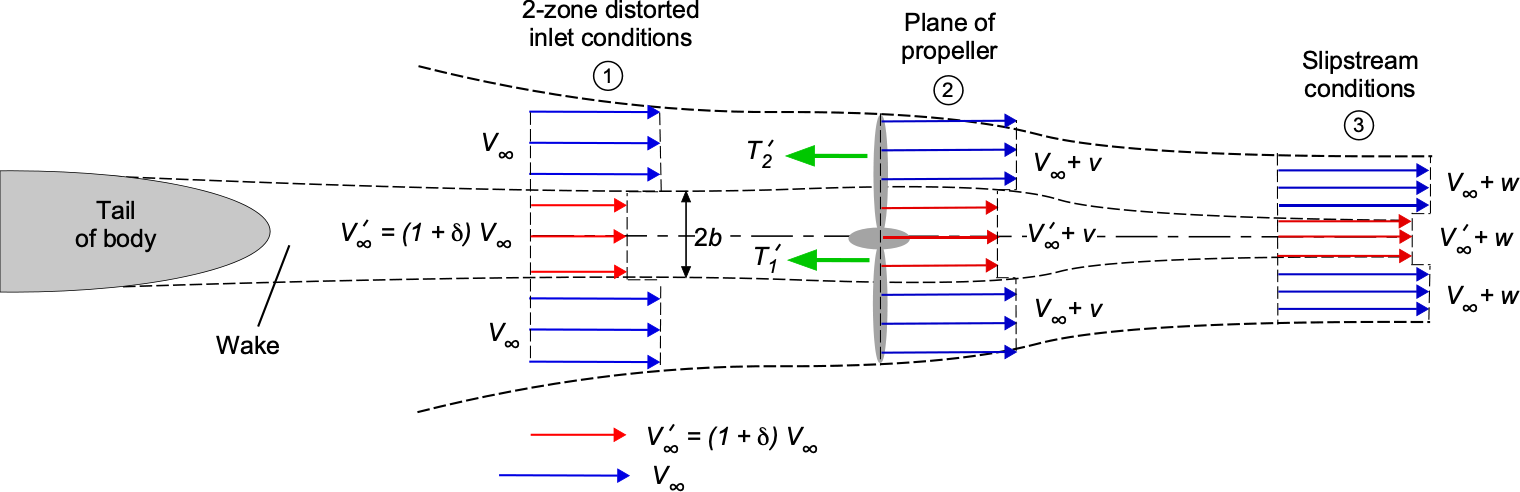
Thrust
(121)
Therefore, the total thrust is
(122)
Notice that =
, so this previous result becomes
(123)
The corresponding thrust in the clean inflow case (no velocity deficit) is
(124)
and so the thrust ratio (distorted inflow versus clean) is
(125)
which for , i.e., a propeller at typical thrust levels in the normal working state, becomes
(126)
Power
Now, consider the power required. The average velocity through each region of the disk is
(127)
The thrust in each region is given by Eq. 121, so the total power required becomes
(128)
Substituting and expanding gives
(129)
so that
(130)
In the limit as , the second term is small and may be neglected, giving
(131)
The ideal (clean inflow) power required is
(132)
so the power ratio (distorted inflow versus clean) is
(133)
Because and
, then the power ratio becomes
(134)
Efficiency
Finally, the propulsive efficiency with inflow distortion is defined in the conventional way using
(135)
From the previous results, then
(136)
Therefore,
(137)
Multiplying out and keeping only first-order terms gives
(138)
(139)
showing that the change in efficiency increases proportionately to the wake velocity deficit , and the area ratio
, i.e., the portion of the propeller disk immersed in the wake.
The apparent increase in propulsive efficiency given by Eq. 139 is a consequence of the idealized assumptions of the actuator disk model, including uniform induced velocity and no swirl. Physically, such an effect could occur because the inner part of the propeller, which generates little propulsive work, consumes less power because of wake deficiency and lower dynamic pressure. However, inflow distortion near the hub may introduce other losses not represented in this analysis.
Low Reynolds Number Propellers
Low Reynolds number propellers are designed to perform efficiently in flow regimes characterized by Reynolds numbers based on the blade sectional chord at 75% radius, typically below 105. Such operating conditions are generally found on small airplanes, UAVs, and drones. In this regime, the blade sectional drag values are higher than at chord Reynolds numbers above 106, which significantly impacts the thrust and lowers the overall propulsive efficiency of the propeller.
As the Reynolds number decreases below one million () and the effects of viscosity begin to outweigh inertia effects in the flow, the lift and drag characteristics of airfoil sections undergo profound changes. The data in the figure below shows the profound effects of operating airfoils at lower Reynolds numbers, which in this case range from as low as 20,000 up to 3,000,000 (three million). At the highest Reynolds number of
, the lift coefficient varies almost linearly with the angle of attack, which is typical of airfoil behavior at higher Reynolds numbers. However, as the Reynolds number decreases below a million, the lift and drag curves become significantly more rounded and eventually become nonlinear for variations in the angle of attack. Indeed, these effects start to become pronounced even at Reynolds numbers of 500,000.
The corresponding drag polar for these conditions provides another helpful way to summarize the effects of the Reynolds number on the aerodynamic characteristics. Notice again the profound effects of reducing the Reynolds number below 500,000, which deleteriously affects the lift-to-drag ratio, especially below values of 50,000. For extremely low Reynolds numbers of less than , lift-to-drag ratios of less than 5 are expected, at least with “conventional” airfoil sections.
Therefore, the impact of these airfoil section effects on propeller performance at low chord Reynolds numbers is profound. For example, the figure below illustrates the efficiencies of a two-bladed propeller at “full scale” and two smaller ones operating at lower tip Reynolds numbers based on average chord. Notice the marked drop in maximum efficiency at lower Reynolds numbers and the range over which the system can operate efficiently. Most of these effects on propulsive efficiency are primarily related to the higher sectional drag coefficient values at these low Reynolds numbers.[15] However, there is also a reduction in maximum sectional lift values, which limits thrust production. These considerations are essential for propeller applications to most UAVs and drones, which can expect maximum propulsion efficiencies of between 50% and 60% rather than 80% or higher for “full-scale” propellers.
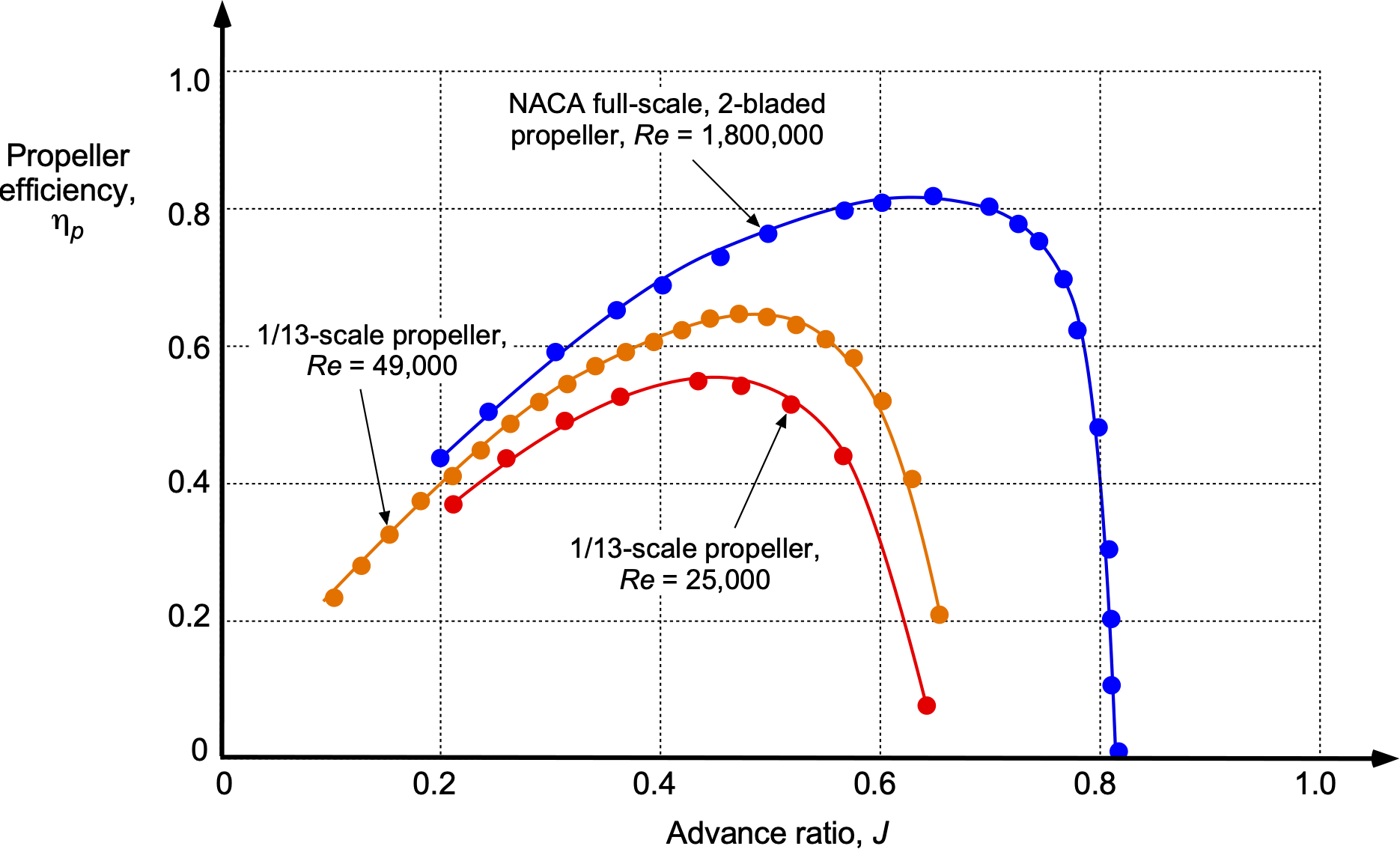
Unique low-Reynolds-number airfoil designs are typically employed to minimize sectional drag on smaller propellers, much like those used on fixed wings, but these must be carefully designed. A larger solidity (blade area) also assists in generating the necessary thrust under these conditions, perhaps at the expense of some other losses in propulsive efficiency. Designing low-Reynolds-number airfoils suitable for propellers typically involves utilizing advanced computational fluid dynamics (CFD) simulations to analyze and optimize their performance. These simulations enable engineers to understand the flow behavior around propeller blades and refine design parameters. Wind tunnel testing is essential for validating the design assumptions and performance predictions.
Selecting Propellers for a Drone
Selecting the correct propeller(s) for a UAV or drone involves several interrelated factors, including the drone’s size, weight, aerodynamic and motor characteristics, and the desired flight performance, such as airspeed, range, and endurance. A VTOL-capable drone may use a separate set of propellers for lifting and another for propulsion, i.e., a hybrid concept. In this setup, the lifting propellers are switched off in forward flight, and a conventional fixed wing generates the lift to overcome the drone’s weight. In some cases, propellers may be used for both lifting and propulsion, as in a tiltrotor or tilting wing concept, which requires further compromises in selecting the best propeller. The assumption is that such propellers are of fixed pitch only, so the selected propeller(s) will inevitably be a compromise.
Steps for Propeller Selection
The following are the basic steps to select the appropriate propeller(s) for low-Reynolds-number operation. Inevitably, without access to a test rig and a wind tunnel, the process will involve trial and error, as well as sound judgment and a degree of common sense. Fortunately, at this smaller scale, propellers are inexpensive, allowing the final decision to be made based on flight testing with a selection of different propellers until the best level of performance is achieved.
- Propeller diameter. To determine the propeller requirements, consider the drone’s weight, aerodynamic efficiency (e.g., lift-to-drag ratio), estimated motor specifications, battery type, voltage, and desired flight characteristics. Heavier drones need larger diameter and higher-pitch propellers to generate sufficient thrust to overcome their drag. A VTOL drone requires sufficient thrust from its lifting propellers to overcome its own weight and maintain lift.
- Calculate the thrust and power requirements. A thrust-to-weight ratio of about 2:1 is necessary for good hovering, forward flight, and maneuvering performance for a quadcopter drone. For example, if a drone has a mass of 1 kg, the design thrust must be capable of carrying 2 kg, e.g., 500 g per motor and propeller for a quadcopter. The power requirements can be estimated using the momentum theory, starting from an initial estimate of the needed propeller diameter.
- Match to motor. Match the initial size and pitch of the propeller to the motor’s recommended range, taking into account the motor’s power or “KV rating.” Different propellers can dramatically affect the drone’s speed, maneuverability, and other flight characteristics.
- Establish the type of propeller. A propulsive propeller will require significantly less thrust than the drone’s weight, depending on the drone’s lift-to-drag ratio. The goal is to achieve the highest level of propulsive efficiency at the propeller’s operating
value for a given combination of required thrust, propeller rpm or rps, and airspeed. To this end, propeller charts., i.e.,
versus
,
versus
and
versus
should be consulted. The speed-power coefficient,
, should be evaluated.
- Review small-scale propeller measurements. They are available in chart form and as data files. They cover a comprehensive range of propellers suitable for drones. Measurements are available in the static thrust condition (i.e., hover) and forward flight over a range of
values. Remember that small propellers have relatively low thrust-producing efficiencies, ranging from 50% to 60%, which impacts flight duration for a given battery charge.
- Consult the motor specifications. The motor’s datasheet may recommend a propeller size (diameter) and pitch. Manufacturers often provide a range of suitable propellers for their motors. It is usually wise to stay within the recommended ranges, although other propellers may also be appropriate.
- Decide on the propeller geometry. Propellers are specified in terms of diameter x pitch. For example, a propeller marked as 20 x 14 means it has a diameter of 20 inches and a helical pitch distance of 14 inches; the propeller could also be specified in cm units. Propellers come in quantum sizes such as 12 inches, 14 inches, etc. Lifting (hovering) propellers will have relatively low pitches, whereas propulsive propellers will have higher pitches. The faster the drone must fly, the higher the propeller pitch needed for good propulsive efficiency.
- Decide on the propeller material. Plastic propellers are lightweight and inexpensive but less durable. Carbon fiber propellers give better aerodynamic performance but are significantly more expensive. It is often a good choice to buy and test three inexpensive propellers initially, perhaps with higher and lower pitches than the first choice, before settling on a carbon fiber propeller.
Hovering (Static Thrust) Propeller
Propellers generally have lower static thrust or hovering efficiency compared to helicopter rotors, partly because of their typically higher disk loadings and non-optimal blade designs. The power requirements can be decomposed into an induced component, which depends on thrust, and a profile component, which is constant and independent of thrust.
Induced Power
From momentum theory, the induced velocity at the propeller’s disk is
(140)
where is the thrust required. The ideal induced power required is
(141)
Expressing this result in terms of the propeller thrust coefficient, i.e., , gives
(142)
Therefore, the ideal induced power coefficient using the propeller definition is
(143)
Accounting for induced losses with the induced power factor , the actual induced power coefficient is
(144)
Profile Power
The profile power is the power required to overcome the aerodynamic drag of the propeller blades as they rotate through the air, independent of any thrust generation. For a propeller in hover, assuming constant blade chord
, constant section drag coefficient
, the differential profile power on a blade segment at radius
is given by
(145)
Multiplying by the number of blades and integrating from the root (
) to the tip (
) yields the total profile power, i.e.,
(146)
The nondimensional profile power coefficient is defined as
(147)
and substituting and
, this becomes
(148)
The solidity of the propeller is
(149)
ans substituting into the expression for gives
(150)
Total Power
Finally, the total power coefficient for hovering flight using the modified momentum theory is
(151)
Alternatively, the power coefficient can be written in terms of the figure of merit, , as
(152)
As shown by the power polar in the figure below, with suitable values for and
or
, the modified momentum theory can give good predictions of hovering performance. The low Reynolds number, combined with the typical (and excessive) spanwise blade twist and non-optimal chord distribution used on these propellers, generally results in relatively poor hovering performance. In this case,
= 1.35 and
= 0.2 (
0.5) gives good agreement with these measurements. Notice that increasing propeller pitch produces more thrust for the same rpm; the resulting measurements then move up the curve to higher values of
and
. The effects of the Reynolds number, combined with the effects of rpm, slightly increase thrust and reduce power at higher rpm values.
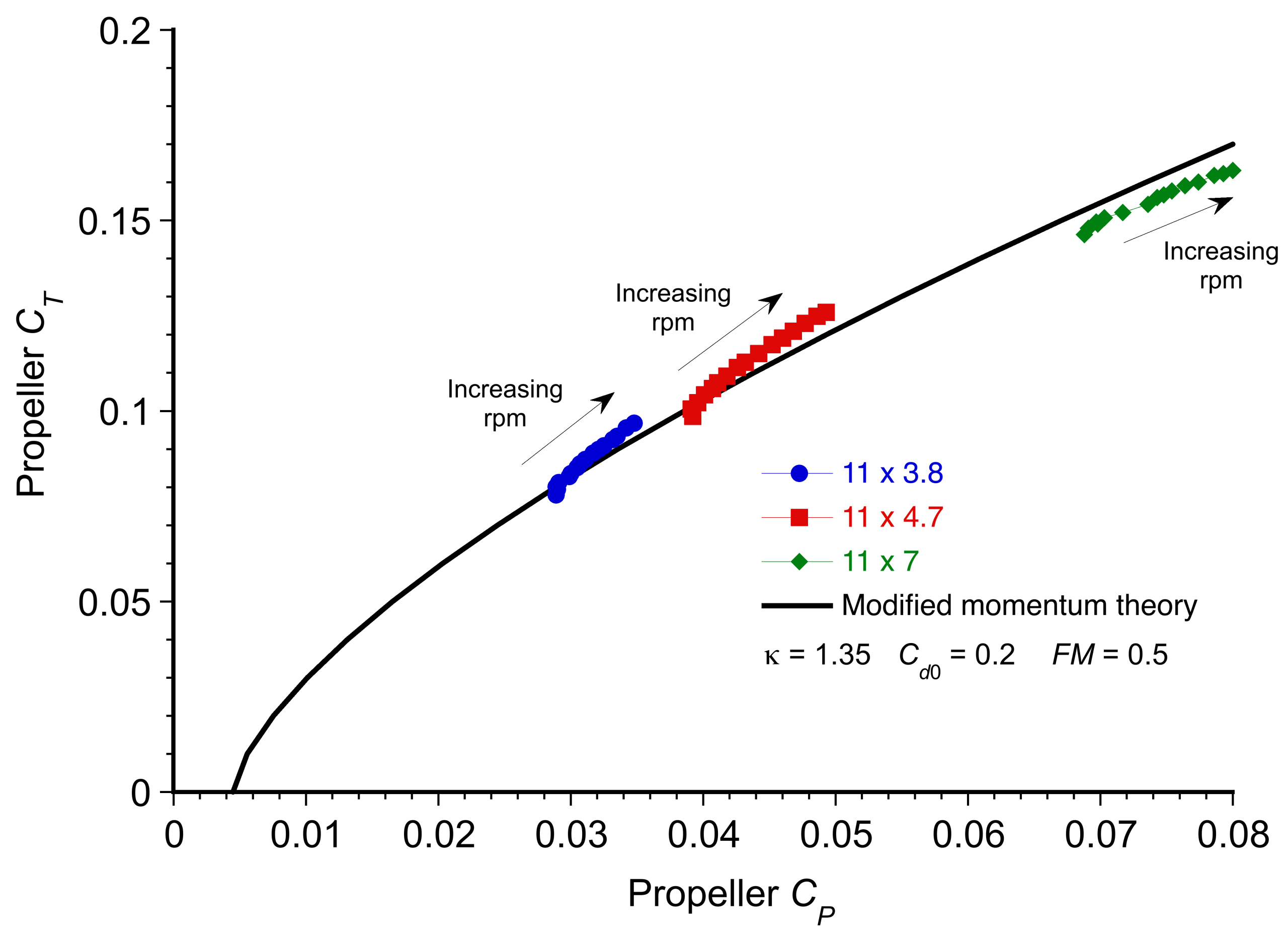
Worked Example #3 –Drone in hovering flight
A VTOL quad-rotor drone weighs 7.5 kg at takeoff, including the battery, camera, and other components. Estimate the size of the propellers and motors needed. Assume MSL ISA conditions.
Show solution/hide solution.
- As a general rule of thumb, the thrust required from the propeller(s) must be enough to carry 7.5 kg
2 = 15 kg to ensure sufficient takeoff and maneuver performance. For example, for a quadcopter, each motor must be attached to a propeller to provide enough thrust to overcome 3.75 kg, i.e., a weight of 36.8 N.
- Estimate the diameter and pitch of the propeller to produce the needed thrust. The thrust equation is
, where in this case
= 36.8 N (thrust per propeller),
= 0.12 (nominal thrust coefficient for a low pitch propeller as shown in the figure above),
= 1.225 kg/m
(standard sea-level air density),
= rpm/60 = 8,880/60 (rotations per second),
= propeller diameter (m). Rearranging to solve for
gives
and substituting the values gives
Propellers are usually only available in quantum sizes, so the next size up will be 13 or 14 inches.
- The momentum theory can be used to estimate the net power, which will be
where
is the number of rotors (= 4 in this case),
, and the figure of merit,
, for a small rotor can be assumed to be 0.6. Inserting the numbers gives
so about 800 W per motor.
- Select the motor in the 800 W power class. Motors are marketed using a kV (or KV) rating, and most also recommend a specific battery requirement. For example, a motor may have an 800 KV rating, which means it will spin at 800 rpm per Volt. If it is supported by an 11.1-Volt LiPo battery, it will spin up to 8,800 rpm. A 200-kV to 400-kV motor will likely be the most suitable choice in this case.
- The motor’s datasheet may also suggest a range of propeller sizes and helical pitches that fully absorb the motor’s power and give good propulsive efficiency. A propeller for good hovering performance typically has a helical pitch of about 3 to 4 inches, but this depends on the required thrust.
- Test the drone with the selected propeller(s) to ensure proper operation. If necessary, propellers of a slightly different pitch may be needed. Suppose a test stand with a load cell or even a digital spring balance is available. In that case, the thrust and battery energy consumption can be measured using different propellers to decide on the most suitable one before the first flight tests. Remember that the best lifting propellers will have low helical pitch values.
- While more detailed performance calculations may ultimately be required, the steps here outline a rapid method for sizing the propellers.
Propulsion Propeller
Selecting a propeller for a drone to meet its propulsion requirements must be performed more rigorously and requires access to propeller charts, specifically the thrust coefficient versus advance ratio
, the power coefficient
versus
, and the efficiency
versus
. Such charts may come from wind tunnel tests or calculations using a propeller model (code). Propulsive propellers for drones, with their high rpm values and lower airspeeds (compared to a conventional airplane), operate at relatively low
values, typically less than unity.
The figure below shows wind tunnel measurements for a small-scale propeller with three different pitch values that are being considered to meet propulsive requirements. At low values of , the propeller blades may experience stall because of excessive pitch relative to the incoming airflow. As airspeed builds and
increases, the blade angles of attack decrease, and the propeller starts to produce useful thrust, also with increasing propulsive efficiency. At some point, the propeller reaches its maximum efficiency. Beyond this, for a given rpm, the blade section angles of attack continue to decrease, which reduces both the lift produced by the blades and the net thrust from the propeller. Eventually, the thrust reduces to zero, and at sufficiently high
for a given blade pitch, the propeller starts to generate negative thrust, thereby creating drag.
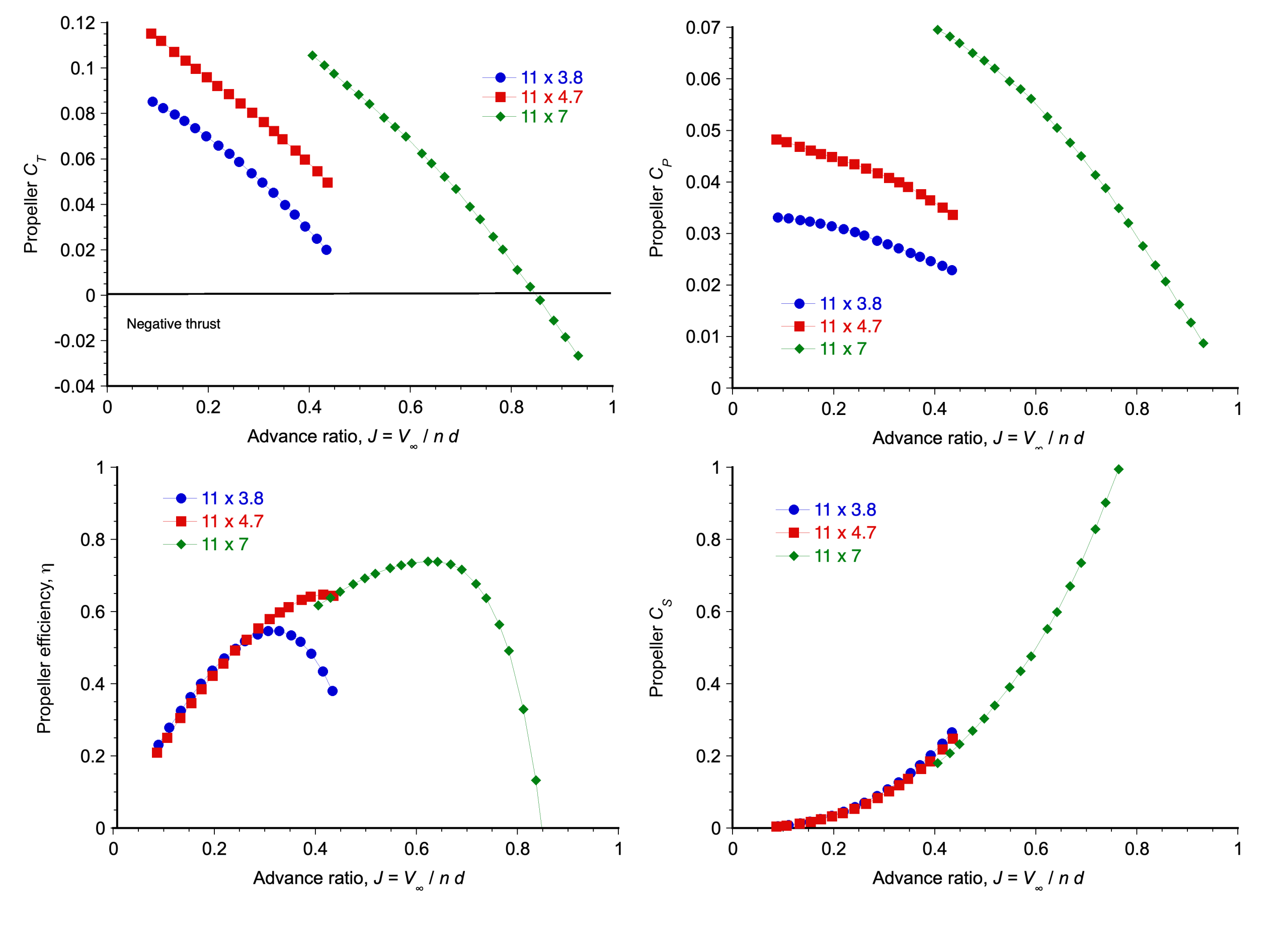
When selecting a specific fixed-pitch propeller, the goal is to operate it at or near its optimal propulsive efficiency. The advantage of non-dimensional coefficients, including the speed-power coefficient, is that the results can scale to different propeller diameters, provided the Reynolds numbers do not change significantly, possibly up to 20%. Inevitably, oversized propellers will have to operate at values of that are too low to be efficient. In contrast, smaller propellers will have to operate at much higher values of
to provide the required thrust. Higher blade pitch values will increase the value of
, at which the maximum propulsive efficiency is obtained. The process is inevitably iterative, based on the power required for flight (and hence the drag of the drone), with the final propeller pitch being a compromise. While it is a point design, it should also have some operating margins in terms of efficiency over a range of airspeeds. Besides
, the use of the
coefficient helps ensure that the selected propeller is matched to the powerplant and aircraft that it powers.
Worked Example #4 – Propeller selection for a drone in forward flight
Consider selecting a propeller for a drone with a motor turning at 7,000 rpm and a power of 8 kW. The required cruise airspeed is 45 mph. The initial choice is an 11 x 3.8-inch pitch propeller, with the 11-inch diameter based on a geometric constraint. Assume MSL ISA conditions and the availability of propeller performance data.
Show solution/hide solution.
The first step is to compute the speed-power coefficient, which is defined as
(153)
Substituting the known values, = 1.225 kg/m
,
= 20.12 m/s,
= 8,000 W, and
= 7,000/60 = 116.67 rps, gives
(154)
Using the curves and the propeller efficiency curves, a speed-power coefficient of approximately 0.52 corresponds to a 7-inch pitch propeller that would be operating near its peak propulsive efficiency. Notice that the shaded zones represent where the values of
and
are compatible.
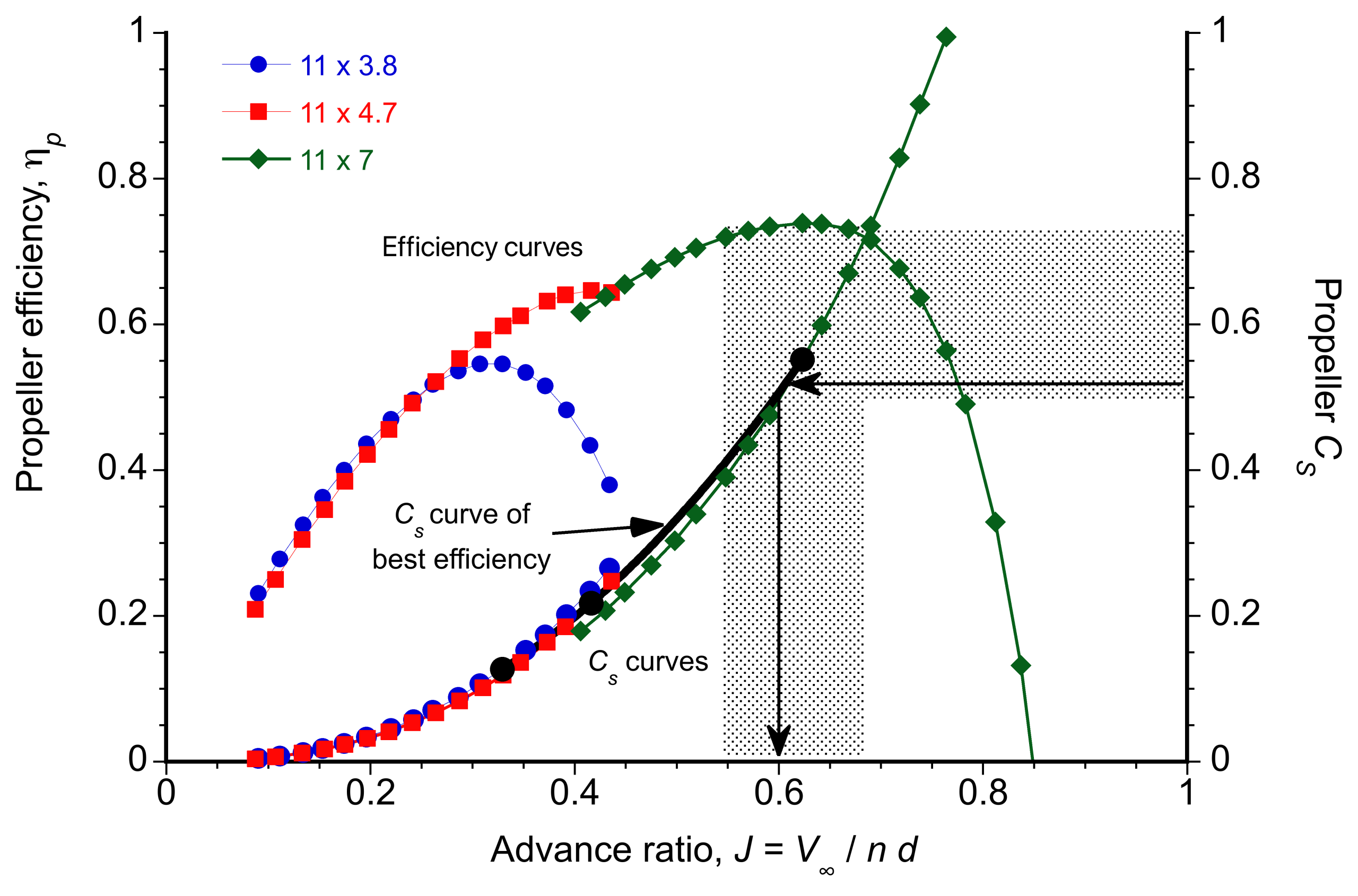
The associated advance ratio for best efficiency at this pitch is approximately = 0.60. The diameter can now be determined using
(155)
This result suggests that an 11.3 x 7-inch propeller would be a better match for this motor than the initially chosen 11 x 3.8-inch propeller. A standard 11 x 7-inch fixed-pitch propeller will closely meet this specification.
Proprotors
A proprotor is a unique aircraft propulsion system that functions as a propeller in forward flight and a helicopter rotor in hover. Unlike conventional propellers, which are optimized solely for producing thrust, a proprotor must also generate vertical lift, as required for vertical takeoff, landing, and low-speed maneuvering. This dual functionality makes a proprotor central to the operation of convertible rotor aircraft such as the V-22 Osprey, where the same rotating system transitions between generating lift and providing propulsion. A proprotor will typically have a larger diameter than a propeller but a lower diameter than a helicopter rotor, which is a compromise fundamental to its design.

The industry continues to explore convertible rotorcraft concepts in pursuit of aircraft that combine the vertical takeoff and hovering efficiency of helicopters with the cruise speed and range of fixed-wing airplanes. Several proposed concepts have emerged, including two large airliner-type concepts, as shown in the figure below. While other proposed configurations exist, most designs rely on one or two pairs of tilting proprotors to provide vertical lift in hover and propulsion in forward flight. Variants such as tiltwing and tiltrotor configurations exhibit distinct aerodynamic and operational trade-offs.
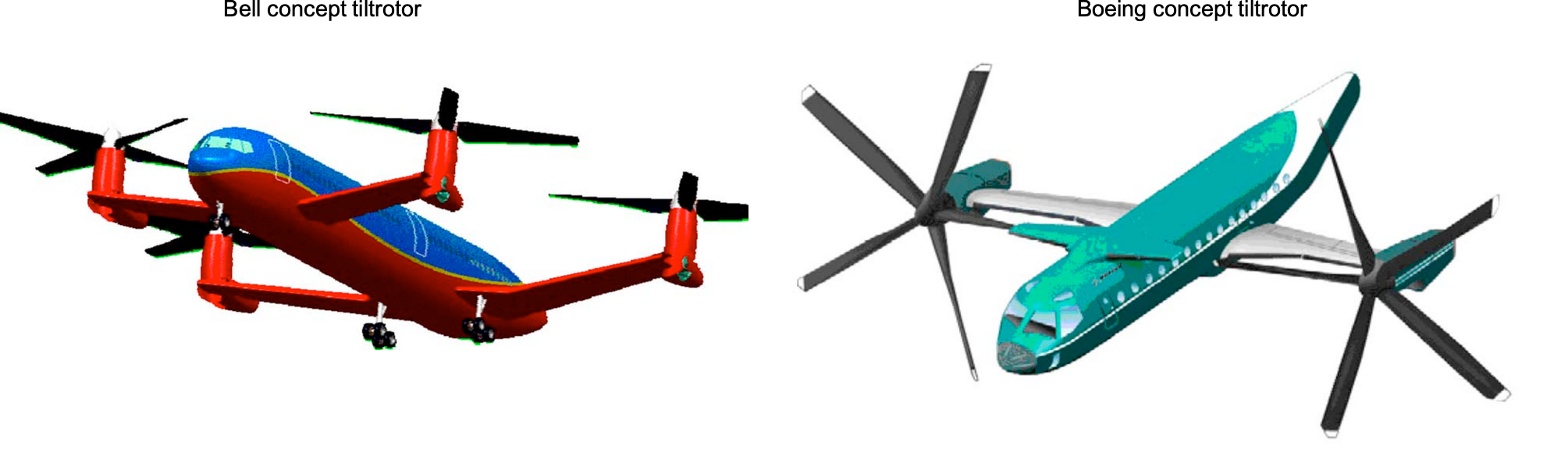
The performance and efficiency of a proprotor depend on numerous blade design and operational considerations. The proprotor must operate efficiently in two fundamentally different flight regimes, each with conflicting aerodynamic requirements, acting as a lifter like a helicopter rotor in vertical flight and as a propulsor like a propeller in forward flight. While some compromises in performance are unavoidable, a key challenge lies in ensuring these trade-offs do not limit the overall flight capabilities of the rotorcraft. This goal is crucial for eVTOL rotorcraft, two proposed concepts being shown in the figure below, which will rely heavily on the optimal efficiency of their proprotors to maximize flight time on each battery charge.
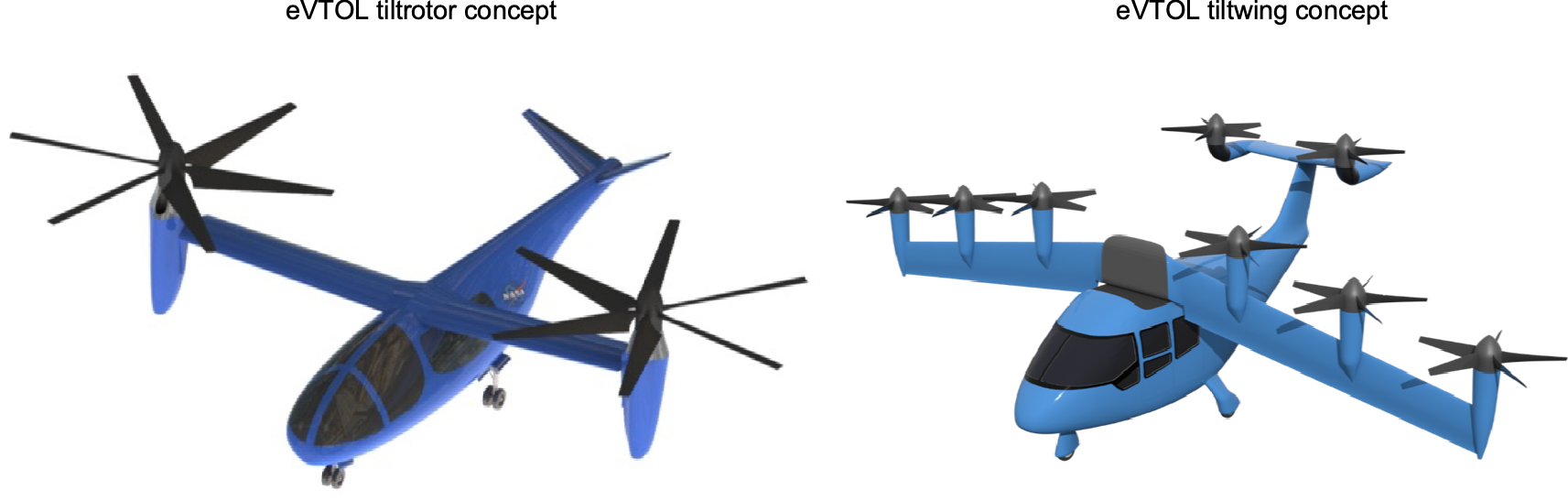
Despite their increased mechanical complexity and higher cost, convertible rotorcraft present engineering solutions for demanding military and civilian missions. With the potential to carry heavier payloads to longer ranges, they can bridge a critical gap between the vertical takeoff capability of helicopters and the speed and range of fixed-wing aircraft. This unique combination enables rapid response over longer distances without the need for runways, supporting operations such as heavy-lift logistics, offshore supply, disaster relief, humanitarian aid in remote regions, and expeditionary military missions. These capabilities are particularly valuable in scenarios where speed, flexibility, and access to unprepared landing zones are essential, conditions under which fixed-wing transports cannot operate. However, such convertible rotorcraft tend to be more expensive to acquire and operate (per unit weight) because they have components and systems common to both helicopters and airplanes.
Overall Design Challenges
A primary difficulty lies in designing proprotors that perform efficiently across a wide flight envelope encompassing diverse aerodynamic operating conditions. Unlike conventional helicopter rotors or propellers, which are optimized for specific situations, proprotors must maintain both good hovering efficiency and high-speed cruise performance. Addressing this dual requirement requires validated modeling tools that can predict rotor aerodynamic performance confidently across diverse operating conditions. At the very least, achieving hovering efficiency comparable to a helicopter, while also attaining cruise efficiency approaching the values typical of turboprop-powered airplanes, will be fundamental to the success of a convertible rotor concept.
Operating State
Thrust, disk loading, , (i.e., thrust per unit disk area), blade loading (thrust per unit blade area,
) or blade loading coefficient
, and tip speed,
, all affect the aerodynamic efficiency of a proprotor in both hovering and forward flight operations. In hover, the proprotors must carry the aircraft’s weight, i.e., for a pair of proprotors, each one will require a thrust equal to half the aircraft’s weight, i.e.,
.
In forward flight, the proprotor encounters higher blade Mach numbers but operates at lower thrust levels because it must only overcome the aircraft’s drag, i.e.,
(156)
where is the aircraft’s lift-to-drag ratio at a given operational condition. The design compromise will be, in part, based on the required disk area and blade area. A larger disk area is better for hovering flight, while a lower blade area is needed for good propulsive efficiency, as illustrated in the figure below.
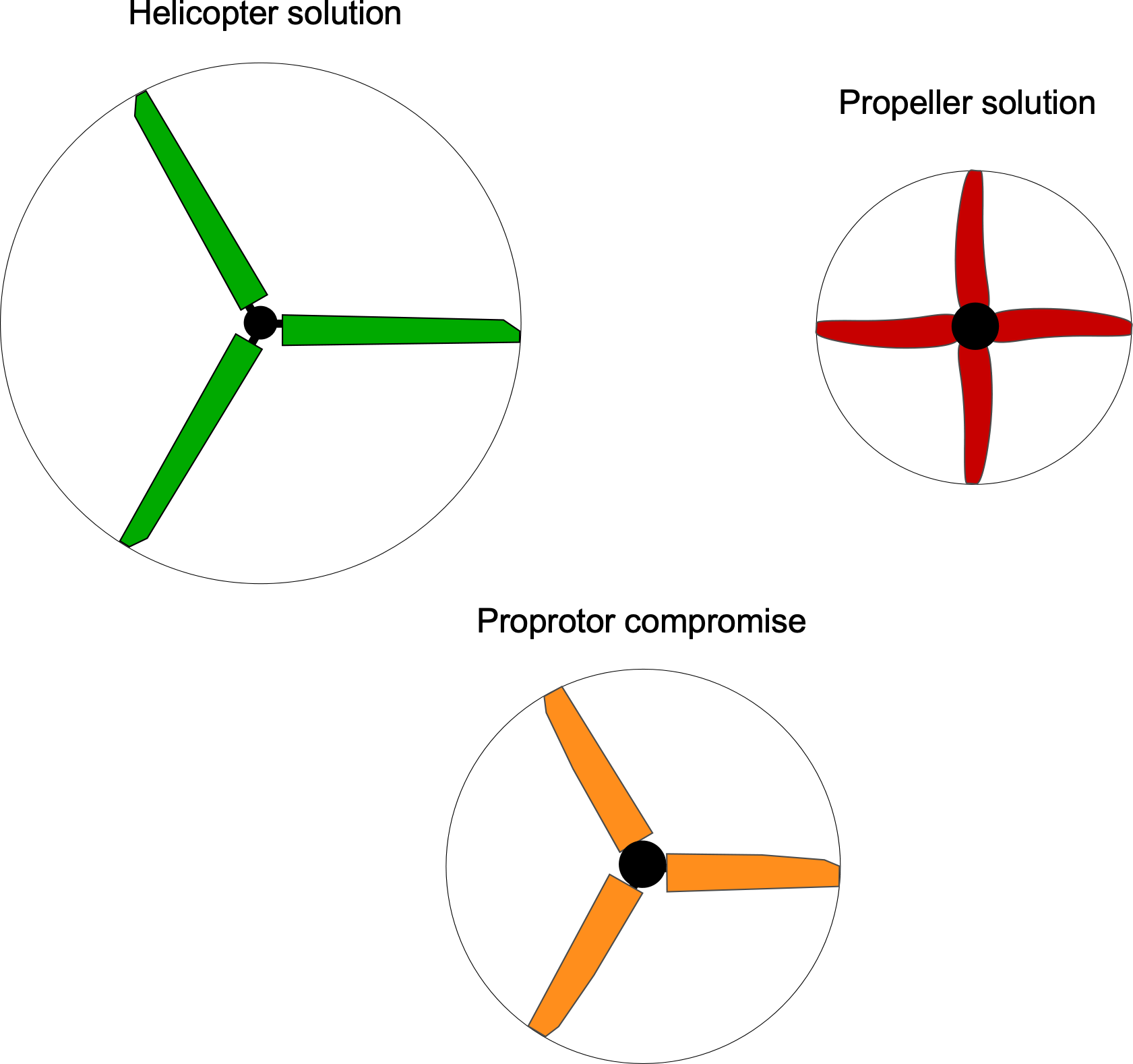
Blade Twist and Distribution
The blade twist required to maximize the hovering performance of a proprotor will not be the same as that needed to maximize the propulsive efficiency while retaining sufficient performance margins. As previously discussed, the twist distribution of a propeller blade is nominally hyperbolic, with less required twist for lower tip speed ratios[16] and hover. In practice, this compromise often results in a blade twist distribution that approximates the average of the optimal values for hover and cruise.
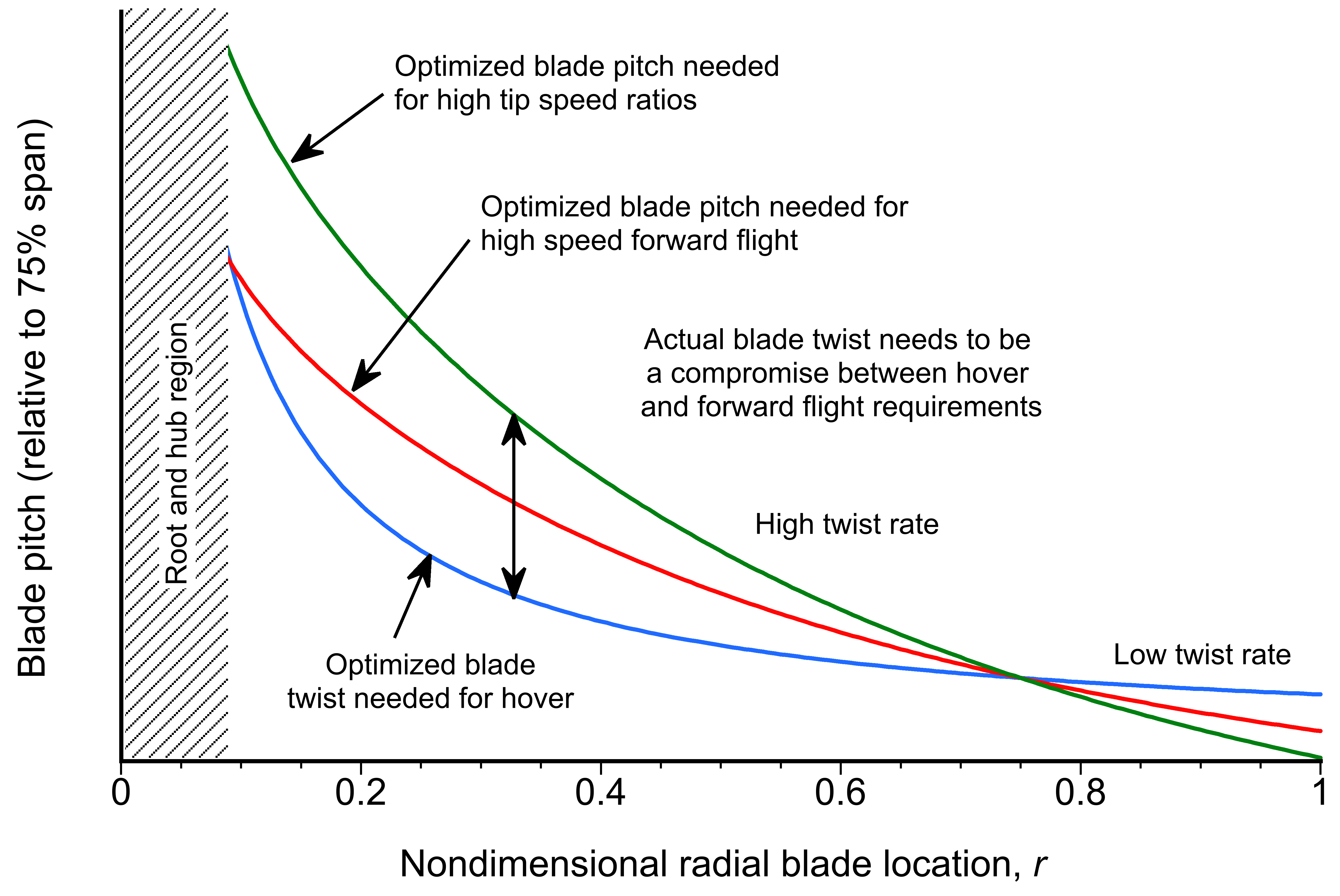
While in-flight blade morphing or twist adjustment is attractive for optimizing performance across flight regimes, current materials and actuation technologies are not yet mature enough for practical application. Smart materials, such as shape-memory alloys and piezoelectrics, show promise but face limitations in durability, actuation speed, and structural integrity under rotor loads. Integrating such systems without adding excessive weight or compromising safety remains a significant challenge. Consequently, proprotor designs rely on fixed-twist blades representing a carefully chosen compromise.
Solidity
Recall that the solidity of a rotating wing device is defined as
(157)
where is the average (or weighted average) blade chord. Notice that the solidity is affected by the number of blades,
. The value of the blade loading coefficient,
, has an upper limit of approximately 0.14 when
is defined based on that for a helicopter rotor, i.e.,
(158)
This limitation on the operating value of arises because of the maximum lift coefficient that the blade sections can realistically produce before stalling. Because of the need for sufficient stall margins for maneuvering flight and gusts in helicopter mode, the maximum value of
should be no more than 0.10. This value imposes a lower limit on the proprotor’s solidity,
, for any given thrust coefficient
, i.e.,
(159)
Therefore, to generate a specified amount of thrust with the lowest power requirements and without the blades stalling inside its expected flight envelope, the proprotor must have a minimum blade area, , and a high enough tip speed,
.
While induced losses dominate performance in hovering flight, which requires a low disk loading to achieve the maximum possible diameter for the proprotor, profile losses govern its propulsive efficiency, just as they do for a regular propeller. Therefore, a lower blade area will be needed for a proprotor when it operates as a propeller. However, this condition may not necessarily be achieved with a lower solidity because is inversely proportional to
. Airfoils with good lift-to-drag ratios at the anticipated operating lift coefficients will also be needed to minimize profile losses. Therefore, the selected disk and blade areas, as well as perhaps the airfoil sections, must be a compromise between the two flight regimes. The selected solidity will also dictate blade and hub weights, which are critical for any VTOL concept.
Blade Planform
Proper optimization of the blade chord is needed to maximize the lift-to-drag ratios of the airfoils along the blade span at their anticipated lift coefficients and over their operational ranges of Mach and chord Reynolds numbers. To this end, an optimal combination of twist, taper, and airfoil shape will be needed. These design principles are the same as those used for propellers and helicopter rotors, where a mild amount of taper from root to tip reduces profile power requirements and optimally distributes the angle of attack and lift coefficient over the blades. Thinning the blade will also be desirable, as it reduces the profile drag coefficient. However, the challenge is to use airfoils that are neither too thin to meet structural requirements nor those that result in higher drag, divergence Mach numbers, higher pitching moments, or reduced maximum lift coefficients. Sweepback on the blade, as done with a modern propeller, may be needed to delay the onset of compressibility effects when the proprotor operates as a propeller.
Tip Speed
While higher tip speeds are desirable to maximize lift on the proprotor and to give good control authority with acceptable stall margins, reduced tip speeds in forward flight may be needed to ensure that the blades do not exceed the local drag divergence Mach numbers of the airfoils, , to maintain propulsive efficiency. Thinning the airfoil section will increase the values of
, as shown in the figure below. While lowered tip speeds will reduce thrust and stall margins, they can also maintain efficiency by reducing the helical Mach numbers on the blade sections below the drag divergence Mach number.
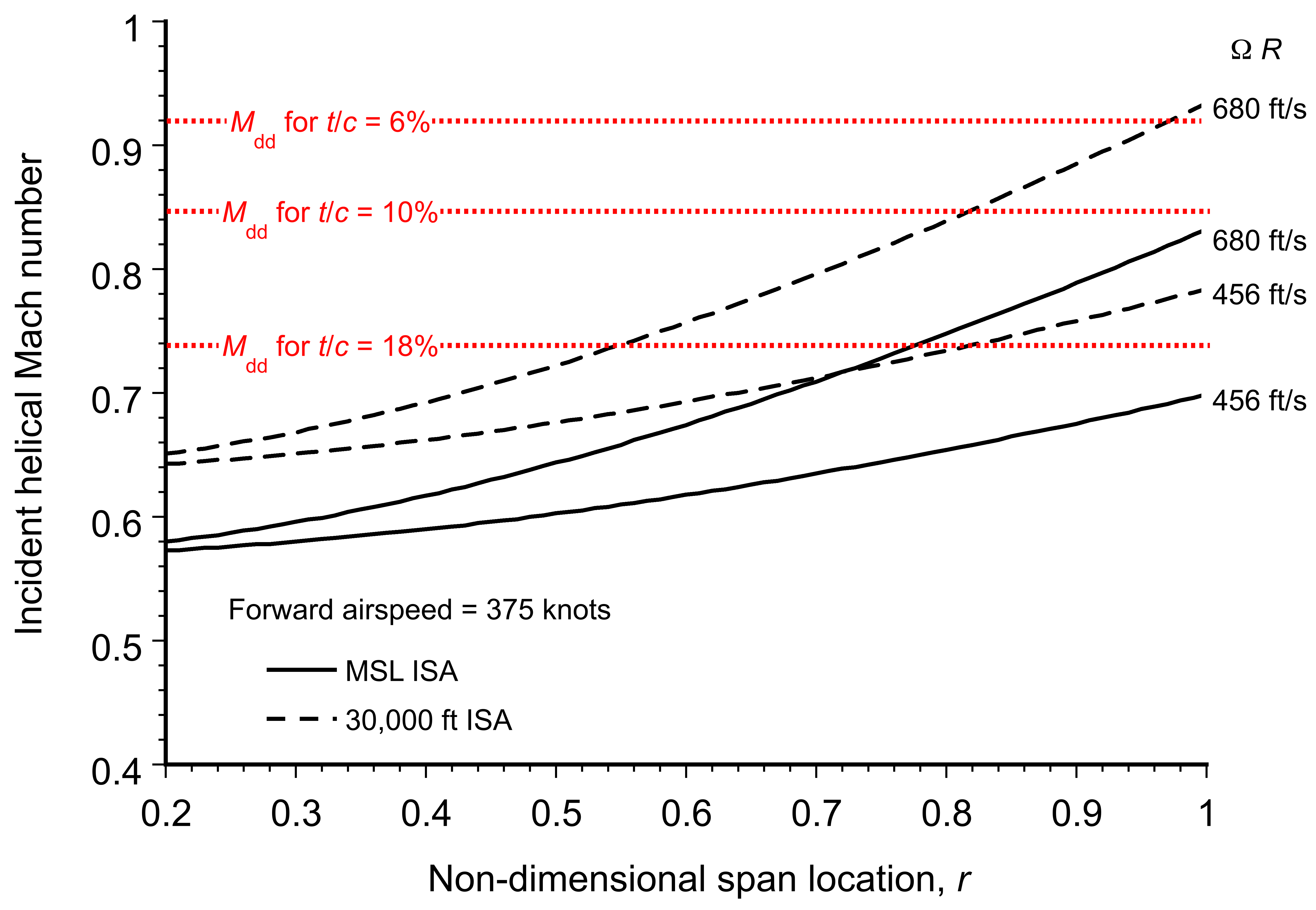
While modulating tip speed has clear advantages, this assumes that stall or gust margins are not compromised and that aeroelastic stability will not become an issue. Future proprotor designs may benefit substantially by continuously optimizing the blade tip speed to produce the needed thrust while maximizing propulsive efficiency and maintaining acceptable performance margins.
Airfoil Sections
Appropriate airfoils with high aerodynamic efficiency in hovering flight, i.e., high lift-to-drag ratios at the design lift coefficients and high maximum lift coefficients, must be used on proprotors while retaining high drag divergence Mach numbers along the blade for forward flight. The figure below illustrates that the section thickness-to-chord ratio has a significant impact on the drag divergence Mach number.
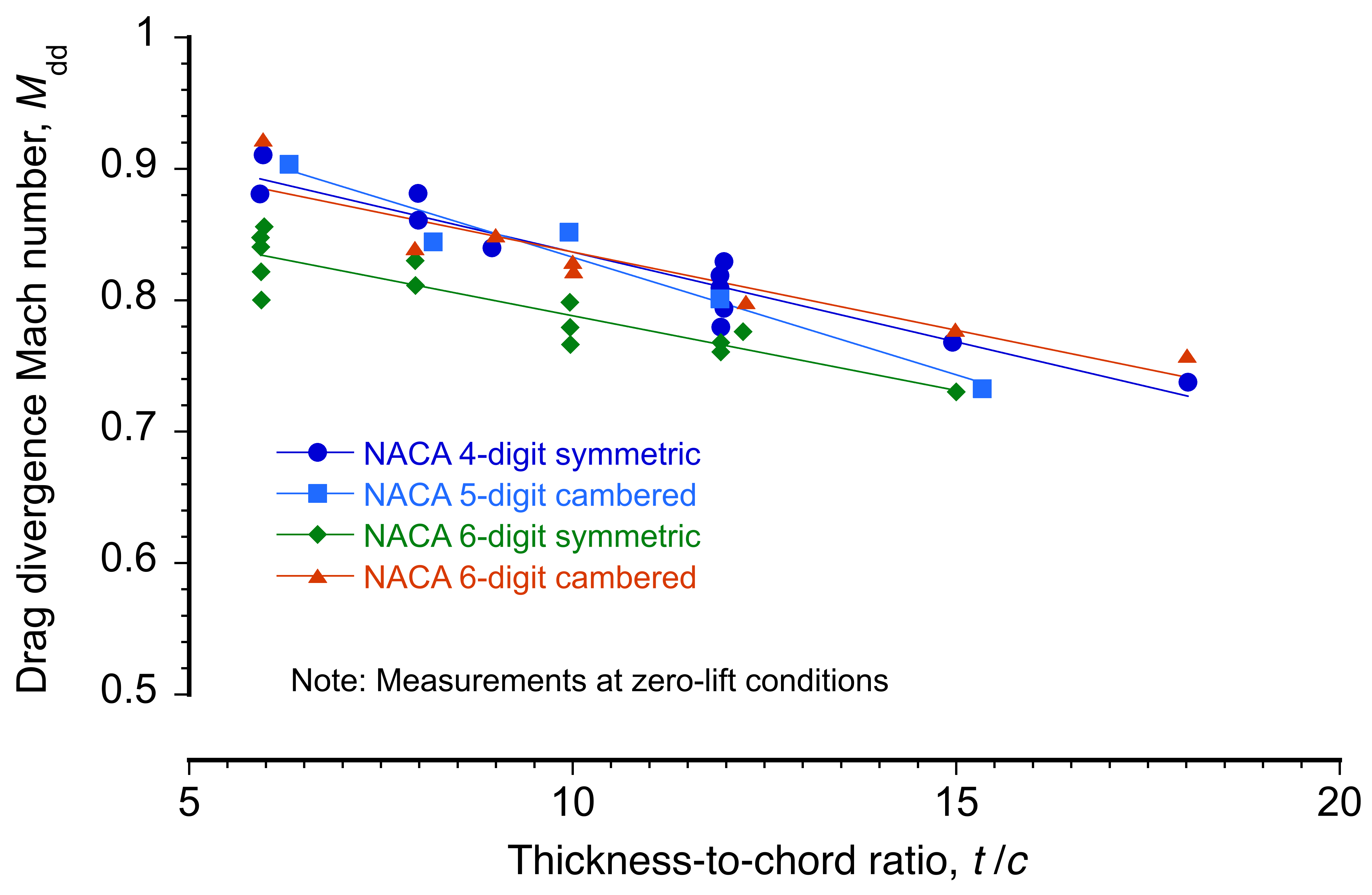
The aerodynamic diversity found on proprotor blades poses additional challenges in airfoil selection and/or design compared to those for helicopter rotors. In high-speed forward flight, more significant parts of the blades will operate at higher subsonic and transonic Mach numbers than in hovering flight. Profile power losses dominate proprotors at higher airspeeds, so accurate estimates of the onset of compressibility losses will be fundamental to predicting their anticipated performance and selecting the most appropriate airfoils.
The proprotor blades on the XV-15 experimental tiltrotor used NACA 64-series airfoil sections. These airfoils were designed for low drag at specific lift coefficients, primarily for subsonic applications. The “64” designation indicates that the airfoil maintains minimum pressure at 40% chord and is tailored for efficient performance within a limited range of lift coefficients. These airfoils are characterized by relatively thin profiles and extended laminar flow regions, making them ideal for proprotors. However, their efficiency comes at the cost of reduced tolerance to off-design conditions and potential sensitivity to surface contamination or roughness. The V-22 uses NASA XN-series airfoils (Experimental-NASA), which are considered proprietary.
In the future, the ability to fully integrate airfoil design methods (i.e., the inverse design of an airfoil and blade shape to meet a given set of requirements) into the overall design of the proprotor may be needed to realize the best efficiency of the proprotor over the broadest possible range of operating conditions.
Design for Hovering Flight
In hovering flight, the proprotors must support the entire weight of the aircraft plus an increment to counter the vertical download on the wings and airframe. While such download effects are relatively smaller on tiltwing concepts, for tiltrotors, this download can comprise a significant fraction of the rotorcraft’s weight. The requirement of a significant vertical force from the proprotor system demands the highest possible values of power loading if the resulting rotorcraft is to achieve good performance.
In hover, the power loading, , is defined as the ratio of the thrust produced by the proprotor to the power required at the shaft to produce that thrust. The
can be written in terms of the disk loading and figure of merit as
(160)
where =
is the disk loading for any one of the proprotors. Power loading is a measure of absolute aerodynamic efficiency because it determines the vertical thrusting capability of the propeller relative to the shaft power delivered, which can be correlated with the fuel expended, given knowledge of the brake-specific fuel consumption (BSFC) characteristics of the engine.
Equation 160 shows that a high value of the figure of merit, , is a necessary but insufficient condition to achieve efficient hovering flight. Low values of
are also required to minimize the induced losses. However, using a large disk area on a proprotor will be incompatible with propulsive efficiency goals in forward flight, where induced losses are relatively small, thrust demands are much lower, and a lower solidity will be required to minimize the more dominant profile losses in this flight condition.
For a typical large-scale rotor system, the maximum achievable value of will typically be between 0.6 and 0.8. Suggestions have been made that proprotors are more efficient than helicopter rotors because they can reach relatively higher values of
before stalling. However, Eq. 160 shows that such claims are misleading because proprotors typically operate at higher values of
as a consequence of their design compromises. Therefore, even with marginally higher values of
, they will still operate at lower power loadings.
Using the figure of merit as a design metric requires further explanation, as its application is not without limitations. The figure of merit is defined relative to the datum “ideal” performance provided by the simple momentum theory, which can be written as
(161)
where (
1) is an induced power factor that depends on the blade design (i.e., blade twist and planform, tip shape, and the number of blades) and proprotor operating state (i.e., thrust and disk loading), and
represents the profile power losses, which depends primarily on airfoil section characteristics and planform shape. Through cumulative design improvements to the blade, profound impacts on overall hovering performance can be achieved.
However, a complicating factor in aerodynamic design is the number of interdependent factors that can influence the figure of merit. In particular, the attainable value of the figure of merit depends on the operating value of , which can be better exposed if the
is rewritten as
(162)
where , in this case, is the weight carried per proprotor. Theoretically, it can be shown that the best power loading of the proprotor is obtained when
. However, this condition is generally achieved at values of
that are too low to be practical (i.e., the rotor size becomes unrealistically big). Therefore, a design goal will be to achieve the highest figure of merit by using the most appropriate blade shape at any value of
and aircraft weight.
Considerable payoffs can result from minor improvements. Only a 1% increase in the value of may lead to a 2–3% increase in aircraft payload and perhaps a 5% increase in range, depending on the proprotor design and the rotorcraft’s anticipated mission(s). However, it is essential to recognize that the selection of disk loading, and ultimately the highest achievable figure of merit of the proprotor, may also be constrained by factors other than those related to its aerodynamics. Trades in the design space, based on the need to attain specific values of propulsive efficiency in forward flight (for speed, cruise performance, and flight range), may also constrain the values of
and
that can be practically achieved. In this respect, power loading remains the most helpful metric for comparing different proprotor concepts because it provides an absolute measure of aerodynamic efficiency.
Design for Forward Flight
In forward flight, the wings of a convertible rotor aircraft carry its entire weight, and the proprotor operates as a pure propulsor, similar to a conventional propeller. Therefore, producing excellent levels of propulsive efficiency will be fundamental to achieving the highest flight speeds and maximizing payload and range. In forward flight, the proprotor operates at lower thrust but with higher inflow, and the induced losses become a progressively smaller fraction of the total power as the airspeed increases. The induced losses, however, will not be negligible, and the blade shape must still be carefully optimized to minimize these losses and achieve the best levels of efficiency. Furthermore, minimizing the fuel load requires that the proprotors be driven by engines with excellent specific fuel consumption. The airframe must also have excellent aerodynamic efficiency with low levels of drag if the aircraft is to meet higher speed and longer-range goals.
However, the highest levels of propulsive efficiency may not be attainable without compromising, in some way, the ability to reach low values of and high values of
in hovering flight. Any significantly compromised levels of hovering and/or propulsive efficiency will reduce the attainable flight range and/or increase fuel requirements, and/or reduce payload. Overcoming this problem remains a significant design challenge. However, the compromises needed may not be severe if certain advances in aerodynamic capability can be realized and/or certain performance margins are relaxed.
Propulsive efficiency, , can be defined in the same manner as conventional propellers, i.e.,
(163)
where is the thrust produced by any one proprotor,
is the propulsive power,
is the induced power, and
is the profile power. The total power required can be written as
(164)
where , and
is the propulsive (useful) power expended. According to the modified momentum theory for a rotor in axial flight, its induced velocity is
(165)
which reduces to the correct result in the static thrust condition (i.e., hover) that
(166)
Notice that in practice, will depend on
. Furthermore, in the limit as
becomes large, then
becomes increasingly small and approaches the limit
(167)
Using Eq. 167, the efficiency can be rewritten as
(168)
where it is apparent that the induced losses still depend on disk loading as they do in hover, but in this case, they diminish relatively quickly with increasing . It is apparent, therefore, that in high-speed forward flight, the efficiency of the proprotor is determined by the ratio of the relative profile power losses to the useful power expended; the equation for propulsive efficiency becomes
(169)
Therefore, minimizing profile losses becomes critical if the goal is to maintain the best values of propulsive efficiency at the highest airspeeds.
Net profile losses must be obtained by radial integration of the drag produced on each blade element, i.e.,
(170)
where is the resultant (helical) velocity at the blade element. With some further assumptions, the profile power required with a given value of profile power coefficient
can be shown to be approximately proportional to the cube of the helical tip speed of the blade, i.e.,
(171)
where is the helical tip velocity based on the vector sum of the rotational and axial flow components at the blade elements, i.e.,
. The value of
depends on a combination of proprotor solidity, planform shape, airfoil section(s), and their operating angles of attack, Mach numbers, etc. Unless stall becomes an issue, profile losses have a smaller dependency on thrust through the variations in local lift coefficients along the blade from their design values. However, as previously explained, compressibility effects, as they contribute to
, become significant when the local helical Mach number begins to approach the drag divergence Mach number(s) of the blade section(s).
The minimization of overall profile losses on the proprotor depends on the ability to design for a smaller disk area (i.e., a lower blade radius), a lower tip speed,
, and the lowest achievable value of
at the operating lift coefficients. The primary challenge is to maximize the drag divergence Mach numbers and lift-to-drag ratios of the blade sections at appropriate combinations of helical Mach numbers and chord Reynolds numbers. The interdependent effects of Reynolds number and Mach number on airfoil performance depend on flight altitude. However, such effects are too airfoil-specific to be readily generalized.
Proprotor/Aircraft Performance Goals
It will now be apparent that achieving balanced hover and forward flight performance requires clearly defined proprotor design requirements. Multiple approaches may be necessary to determine the design requirements in relation to the desired mission performance. One approach is illustrated in the figure below, which compares the propulsive efficiency of a proprotor versus its hovering figure of merit. All things being equal, high values of both and
will be necessary for good overall efficiency. However, because both
and
are only relative measures of efficiency, these parameters do not fully expose the requirements needed for the rotorcraft as a whole.
An alternative presentation in the figure below also summarizes a way of setting proprotor efficiency goals[17] for future generations of convertible rotor aircraft in terms of hover power loading. Power loading, , is an absolute measure of hovering efficiency because it represents the ratio of thrust produced to the power required to produce that thrust, as well as fuel burn. Recall from Eq.161 that in hover
(172)
so good hovering efficiency is only obtained by maximizing the figure of merit and minimizing disk loading. The requirements would be set at the density altitudes appropriate to the intended mission profile(s).
Conventional helicopters have hover power loadings in the range of 9 to 11 lb /hp (5.47 to 6.69 kg/kW). In contrast, current tiltrotors such as the V-22 (because of their smaller diameter rotors and higher disk loadings) have power loadings of only about 6 to 7 lb/hp (3.6 to 4.26 kg/kW), even with the claimed higher figure of merit (0.82) relative to a helicopter. Therefore, the design goal for any future convertible rotor concept is to approach the hovering power loading of a helicopter while also being capable of producing power loadings and propulsive efficiencies comparable to those obtained with conventional propellers. As previously mentioned, this will require the judicious selection of the diameter, blade twist, planform, airfoils, tip shape, and tip speed of the proprotor.
Yet another presentation of proprotor design requirements is shown in the figure below in terms of the propulsive power loading in forward flight versus the power loading in hover. As previously mentioned, the currently flying incarnation of the tiltrotor, the V-22, has substantially lower power loadings in hovering flight than a helicopter. So, it is only about half as efficient as a helicopter in hover per unit of payload. Recall also that the power loading in forward flight is
(173)
which depends on both and
. The V-22 also has a lower power loading in forward flight relative to a turboprop airplane. Based on its estimated
, the V-22 can only be about half as efficient as a turboprop airplane in transporting payload. It would seem there is much scope for improvement from an engineering perspective.
In conclusion, it can be stated that while maximizing both and
must be a prerequisite for any proprotor design, this must be done by also balancing the requirements of the design against the need for low disk loading and high cruise lift-to-drag ratio to achieve the highest possible values of power loading, i.e., any proprotor design must ultimately be matched to the rotorcraft as a whole. Achieving high power loadings in hovering and forward flight becomes even more critical for convertible rotor concepts that carry heavier payloads to longer flight ranges.
Summary & Closure
Propellers are an effective and robust means of generating thrust, making them widely used in aviation across various propulsion systems. Fixed-pitch propellers, while straightforward and lightweight, have inherent limitations in their efficiency across different flight conditions. Variable-pitch and constant-speed propellers expand the operational range, allowing for better efficiency at various speeds and altitudes, though they come with increased mechanical complexity, weight, and cost. Whether paired with piston engines, turboshaft engines, or electric motors, correctly matching the propeller to its specific powerplant is crucial for achieving optimal performance.
A key trend in the design of modern airplanes is the shift toward propellers with smaller diameters and more blades. This configuration helps reduce blade tip speeds, minimize noise, and improve efficiency. Advances in composite materials, such as Kevlar and carbon fiber, have further transformed propeller design. These materials enable thinner, swept blades that enhance aerodynamic efficiency at higher tip Mach numbers and flight speeds while reducing weight, vibration, and acoustic footprint. Despite these innovations, wooden propellers are still used for low-performance airplanes because of their cost-effectiveness and ease of manufacturing.
For UAVs and drones, propeller selection plays a crucial role in overall efficiency, endurance, and mission effectiveness. Fixed-pitch propellers are typically preferred because of their simplicity and reliability; however, they require careful optimization to achieve optimal performance. The relationship between blade pitch, advance ratio, and rotational speed must be fine-tuned to ensure efficient thrust production across various flight conditions. Non-dimensional coefficients, such as the power-speed coefficient, assist in scaling performance across different propeller sizes, but trade-offs are inevitable. The iterative nature of propeller design means that achieving the right balance between thrust production and power consumption is essential. Ultimately, the goal is to develop propellers that maintain high efficiency across various operating conditions while meeting noise, weight, and performance constraints. As UAV and drone technology advances, so will propeller innovations, pushing the boundaries of aerodynamic efficiency, power optimization, and operational versatility.
Proprotors represent a critical enabling technology for VTOL aircraft that must transition seamlessly between hover and forward flight. Their unique dual-role function introduces more complex aerodynamic challenges, requiring careful trade-offs in design to meet competing performance demands. As research continues to refine airfoil selection, blade geometry, and rotor control strategies, proprotor systems will remain central to advancing efficient, versatile convertible-rotor airplane concepts that meet civil and military mission requirements.
5-Question Self-Assessment Quickquiz
For Further Thought or Discussion
- Is using a fixed-pitch or constant-speed propeller for a UAV desirable? Discuss.
- Why does using a swept blade on a propeller generally help give the propeller better efficiency?
- Discuss the factors that may influence the design of a propeller blade, including material selection, shape, and angle of attack.
- Explain the concept of propeller slipstream and its influence on the overall aerodynamic performance of an aircraft. How does the slipstream interact with other aircraft components?
- Explain how tip speed affects propeller noise and efficiency. What design considerations are made to optimize tip speed within acceptable limits?
- Explain the phenomenon of propeller torque and its effect on aircraft handling. How do pilots and designers compensate for torque effects during flight?
Other Useful Online Resources
To learn more about propellers, check out these helpful online resources:
- Ever wonder how an airplane propeller is made? Take a tour of Hartzell Propeller to learn how propellers work and how they are made.
- “The Propeller Explained” is a documentary film.
- World’s Largest Wooden Propeller Factory: How It’s Made.
- Props to Alaina: The Art of Airplane Propellers.
- How Sensenich Wooden Props are made.
- Apparently, this is the only video you need to understand airplane propellers. It’s good, but there is more to learn.
- “Airplane Propellers: Principles and Types” is a World War II-era U.S. War Department training film.
- In 2022, students from the University of Maryland made a flying quadrotor drone based on the aerial screw design. Still, it only had a novelty value compared to modern quadrotor designs. ↵
- A steam engine of the era would have been far too heavy (boiler, engine, condenser, fuel, water, etc.) and with too little power to be of any aeronautical use. ↵
- Glauert, H. (1935) "Airplane Propellers." In: Durand, W.F., Ed., Aerodynamic Theory, Vol. IV, Division L, Springer, New York, 169–360. ↵
- An excellent analogy to this concept would be a continuously variable transmission on an automobile. ↵
- The blade twist distribution is fixed and cannot be varied. ↵
- The actuator disk is equivalent to an infinite number of blades. ↵
- rpm = revolutions per second; rps = revolutions per minute. ↵
- Aspects of Rankine's propeller theory were published in various sources, including Rankine, W. J. M., "On the Mechanical Principles of the Action of Propellers," Transactions of the Institute of Naval Architects, Vol. 6, pp. 13-39, 1865. ↵
- Froude, R. "On the Part Played in Propulsion by Differences in Fluid Pressure," Transactions of the Royal Institution of Naval Architects, 30, 390, 1889. ↵
- The boss is simply the part of the propeller that is used to attach it to the engine shaft or gearbox. ↵
- However, it should be noted that the definitions of
and
are different from those used for helicopter rotors. ↵
- In a free-turbine turboprop, the propeller is driven by a power turbine that is not mechanically connected to the gas generator. ↵
- Weick, F. E., Aircraft Propeller Design, McGraw-Hill Book Co., Inc., New York and London, 1930. ↵
- The reciprocal of the coefficient was used by Drzewiecki. See: Drzewiecki, S., Théorie Générale de l'Hélice Propulsive, Gauthier-Villars, Paris, 1920. This form, however, covers an extensive range of values, making its use impractical. ↵
- Although some additional losses come from the wake, which experiences a higher fraction of losses from the thicker boundary layers trailed into the wake and increased turbulence from shear. ↵
- Meaning lower values of
. ↵
- This form of presentation has been suggested by Dr. Kenneth Rosen, former VP of Engineering for Sikorsky Aircraft. ↵

